Attribute-Based Conditional Proxy Re-encryption in the Standard Model Under LWE

Abstract

Attribute-based conditional proxy re-encryption (AB-CPRE) allows delegators to carry out attribute-based control on the delegation of decryption by setting policies and attribute vectors. The fine-grained control of AB-CPRE makes it suitable for a variety of applications, such as cloud storage and distributed file systems. However, all existing AB-CPRE schemes are constructed under classical number-theoretic assumptions, which are vulnerable to quantum cryptoanalysis. Therefore, we propose the first AB-CPRE scheme based on the learning with errors (LWE) assumption. Constructed from fully key-homomorphic encryption (FKHE) and key-switching techniques, our scheme is unidirectional, single-hop, and enables a polynomial-depth boolean circuit as its policy. Furthermore, we split the ciphertext into two independent parts to avoid two-level or multi-level encryption/decryption mechanisms. Taking advantage of it, we then extend our single-hop AB-CPRE into an efficient and concise multi-hop one. No matter how many transformations are performed, the re-encrypted ciphertext is in constant size, and only one encryption/decryption algorithm is needed. Both of our schemes are proved to be selective secure against chosen-plaintext attacks (CPA) in the standard model.

Appendices

A Proof for Single-hop AB-CPRE

All details of proof can be found in full version [20]. Due to space limitations, we only present the simulator algorithms used in our proof.

• $\mathbf { Setup }_{SIM}(n , \mathbf{x}^* )$: Let $\mathbf{x}^* = \lbrace x_i^* \rbrace _{i \in [\ell ]}$ to be the attribute vector selected by adversary $\mathcal {A}$. Sample a uniform matrix $\mathbf{D}_{\theta } \leftarrow \mathbb {Z}_q^{n \times m}$ and generate a random identity’s public key $\mathbf{A}_{\theta } \leftarrow \mathbb {Z}_q^{n \times m}$ , then choose $\ell$ random matrices $\mathbf{S}_1^* , ... , \mathbf{S}_\ell ^* \leftarrow \lbrace -1 , 1 \rbrace ^{m \times m}$. Set $\mathbf{B}_i = \mathbf{A}_{\theta } \mathbf{S}_i^* - x_i^* \mathbf{G}$ for all $i \in [\ell ]$. Select an error sampling algorithm $\chi$, which is a $B-$bounded distribution. Keep matrices $\lbrace \mathbf{S}_i^* \rbrace _{ i \in [\ell ] }$ as secret and output public parameters $pp := ( \lbrace \mathbf{B}_i \rbrace _{i \in [\ell ]}, \chi )$ and specific public key $pk_{\theta } := ( \mathbf{A}_{\theta } , \mathbf{D}_{\theta } )$.

• $\mathbf { Enc }_{SIM}(pp , pk_\theta ,\boldsymbol{\mu }_b , \mathbf{x}^* )$: Let $pp = ( \lbrace \mathbf{B}_i \rbrace _{i \in [\ell ]}, \chi )$, $pk_\theta = \mathbf ( \mathbf{A}_{\theta } , \mathbf{D}_{\theta } )$, a challenge message $\boldsymbol{\mu }_b \in \lbrace 0 , 1 \rbrace ^{m}$, and a selected attribute vector $\mathbf{x}^* = ( \lbrace x_i^* \rbrace _{ i \in [\ell ] } )$. Choose a random vector $\mathbf{s} \leftarrow \mathbb {Z}_q^n$ and two error vectors $\mathbf{e}_{in} , \mathbf{e}_{out} \leftarrow \chi ^{m}$. Compute $ct = ( \mathbf{c}_{in} , \mathbf{c}_{out} )$ as

  $$\mathbf{c}_{in} = ( \mathbf { A_{\theta } } )^T \mathbf{s} + \mathbf{e}_{in} , \mathbf{c}_{out} = ( \mathbf { D_{\theta } } )^T \mathbf{s} + \mathbf{e}_{out} + \lfloor q/2 \rfloor \boldsymbol{\mu }_b.$$

  Use $\lbrace \mathbf{S}_i^* \rbrace _{ i \in [\ell ] }$ chosen in $Setup_{SIM}$ instead of uniform matrices in $\lbrace -1 , 1 \rbrace ^{m \times m}$ and then assemble $cc^* = ( \lbrace \mathbf{c}_i = (x_i^* \mathbf{G} + \mathbf{B}_i )^T \mathbf{s} + (\mathbf{S}_i^*)^T \mathbf{e}_{in} \rbrace _{ i \in [ \ell ] } ) \in \mathbb {Z}_q^{ \ell m }$. Output a challenge ciphertext $CT^* = (ct^* , cc^* )$.

• $\mathbf { ReKeyGen }_{SIM}( pp , pk_{\beta } , f )$: Parse $pp = (\lbrace \mathbf{B}_i \rbrace _{i \in [\ell ]}, \chi )$ , $pk_{\beta } = ( \mathbf{A}_{\beta } , \mathbf{D}_{\beta } )$, and a policy $f \in \mathcal {F}_{\ell , d}$. Let $\mathbf{S}_f^* = \mathbf {Eval}_{ \mathrm {sim} }( f , (x_i^* , \mathbf{S}_i^*)_{i \in [\ell ] } , \mathbf{A}_{\theta })$.

  • 1) In the case that $f( \mathbf{x}^* ) \ne 0$, let $\mathbf{B}_f = \mathbf{A}_{\theta } \mathbf{S}_f^* - f( \mathbf{x}^* ) \mathbf{G}$ and set $\mathbf{F} = [ \mathbf{A}_{\theta } | \mathbf{B}_f - f( \mathbf{x}^* ) \mathbf{G} ] \in \mathbb {Z}^{n \times 2m}$. Compute the basis $\mathbf{T}_{ \theta , f}$ for $\mathbf{F}$ as $\mathbf{T}_{ \theta , f} \leftarrow \mathbf {ExtendLeft}( \mathbf{A}_{\theta } , \mathbf{G} , \mathbf{T}_{\mathbf{G}}, \mathbf{S}_f )$. Then generate a matrix $\mathbf{R}_{ \theta , f } \in \mathbb {Z}^{2m \times m}$ such that $\mathbf{F} \mathbf{R}_{ \theta , f } = - \mathbf{D}_{\theta }$ by executing $\mathbf {SamplePre}( \mathbf{F} , \mathbf{T}_{ \theta , f} , - \mathbf{D}_{\theta } , \sigma )$, set $\overline{\mathbf{R}}_{\alpha ,f } = \mathbf {Power2}_q( \mathbf{R}_{ \alpha , f } )$, sample $\mathbf{E}_1 \leftarrow \chi ^{2km \times n} , \mathbf{E}_2 , \mathbf{E}_3 \leftarrow \chi ^{2 km \times m}$ and compute

    $$\mathbf{Q} = \begin{bmatrix} \mathbf{E}_1 \mathbf{A}_{\beta } + \mathbf{E}_2 &{} \mathbf{E}_1 \mathbf{D}_{\beta } + \mathbf{E}_3 + \overline{ \mathbf{R} }_{ \theta , f } \\ \mathbf{0}_{m \times m} &{} \mathbf{I}_{m \times m} \end{bmatrix} \in \mathbb {Z}_q^{ (2km + m ) \times 2m}.$$

  • 2) In the case that $f( \mathbf{x}^* ) = 0$, sample two matrices $\mathbf{E}_1 \leftarrow \chi ^{2km \times n} , \mathbf{E}_2 \leftarrow \chi ^{2 km \times m}$, choose a matrices $\mathbf{M}' \leftarrow \mathbb {Z}^{ 2km \times m }$ uniformly at random, and compute

    $$\mathbf{Q} = \begin{bmatrix} \mathbf{E}_1 \mathbf{A}_{\beta } + \mathbf{E}_2 &{} \mathbf{M}' \\ \mathbf{0}_{m \times m} &{} \mathbf{I}_{m \times m} \end{bmatrix} \in \mathbb {Z}_q^{ (2km + m ) \times 2m}.$$

  Output $rk_{ \theta , f \rightarrow \beta } = \mathbf{Q}$ as re-encryption key.

B Correctness for Multi-hop AB-CPRE

The correctness of original ciphertext is the same as the correctness in Sect. 4. Then the correctness of transformed ciphertext is presented as follows.

Transformed Ciphertext. $(ct^{(t-1)} = (\mathbf{c}_{in}^{(t-1)} , \mathbf{c}_{out}^{(t-1)} ) , cc^{(t-1)} = \lbrace \mathbf{c}_i^{(t-1)} \rbrace _{i \in [\ell ] } )$ is the ciphertext which has been transformed $t-1$ times and associated with attribute vector $\mathbf{x}$ under $pk_\alpha$. For convenience, $rk_{\alpha , f \rightarrow \beta , \mathbf{y}} = (\mathbf{Q} , \mathbf{P})$ is the re-encryption key, where $f( \mathbf{x} ) = 0$. Set $\widetilde{ \mathbf{c} }_{in,f}^{(t-1)} = \mathbf {Bits}_q( [\mathbf{c}_{in}^{(t-1)} ; \mathbf{c}_f^{(t-1)} ] )$ and $\overline{ \mathbf{R} }_{\alpha ,f } =\mathbf {Power2}_q( \mathbf{R}_{\alpha , f } )$. Then the t times transformed ciphertext $( ct^{(t)} , cc^{(t)} )$ is showed as following;

$$\begin{aligned} \mathbf{c}_{in}^{(t)}&= (\mathbf {s}^{(t)})^T \mathbf{A}_\beta + \mathbf{e}_{in}^{(t)} = ( \widetilde{ \mathbf{c} }_{in,f}^{(t-1)} )^T \mathbf{E}_1 \mathbf{A}_\beta + ( \widetilde{ \mathbf{c} }_{in,f}^{(t-1)} )^T \mathbf{E}_2, \\ \mathbf{c}_{out}^{(t)}&= ( \widetilde{ \mathbf{c} }_{in,f}^{(t-1)} )^T \mathbf{E}_1 \mathbf{D}_\beta + ( \widetilde{ \mathbf{c} }_{in,f}^{(t-1)} )^T \mathbf{E}_3 + [ \mathbf {e}_{in}^{(t-1)} ; \mathbf {e}_{f}^{(t-1)} ]^T \mathbf{R}_{\alpha , f } + ( \mathbf{e}_{out}^{(t-1)} )^T + \lfloor q/2 \rfloor \boldsymbol{\mu }, \\ \lbrace \mathbf{c}_{i}^{(t)}&= ( \mathbf {s}^{(t)} )^T( y_i \mathbf{G} + \mathbf{B}_i ) + ( \mathbf{e}_i^{(t)} )^T = ( \widetilde{ \mathbf{c} }_{in,f}^{(t-1)} )^T \mathbf{E}_1 ( y_i \mathbf{G} + \mathbf{B}_i ) + ( \widetilde{ \mathbf{c} }_{in,f}^{(t-1)} )^T \mathbf{E}_{B_i} \rbrace _{ i \in [\ell ] }. \end{aligned}$$

For any $t > 0$, we can learn that, $\Vert \mathbf {e}_{in}^{(t)} \Vert \le 2 k m \sqrt{m} B$ and $\Vert \mathbf {e}_i^{(t)} \Vert \le 2 k m \sqrt{m} B$. Because $\Vert \mathbf{e}_{out}^{(0)} \Vert \le \sqrt{m}B$ and $\Vert \mathbf{e}_f^{(t)} \Vert \le \sqrt{2} m k B (m+1)^d$, we have,

$$\Vert \mathbf {e}_{out}^{(t)} \Vert \le \sqrt{m}B + 2 k m \sqrt{m} B t + 2 \sqrt{2}km^2(m + 1)^d \sigma Bt.$$

Therefore, for the t times transformed ciphertext $( ct^{(t)} , cc^{(t)} )$,

$$\begin{bmatrix} ( \mathbf {c}_{in}^{(t)} )^T \quad ( \mathbf {c}_{out}^{(t)} )^T \end{bmatrix} \cdot \begin{bmatrix} \mathbf{R}_{\alpha } \\ \mathbf{I}_{m \times m} \end{bmatrix} = ( \mathbf {e}_{in}^{(t)} )^T \mathbf{R}_{\alpha } + ( \mathbf {e}_{out}^{(t)} )^T + \lfloor q/2 \rfloor \boldsymbol{\mu }^T. $$

where $\Vert ( \mathbf {e}_{in}^{(t)} )^T \mathbf{R}_{\alpha } + ( \mathbf {e}_{out}^{(t)} )^T \Vert \le \Vert ( \mathbf {e}_{in}^{(t)} )^T \Vert \cdot \Vert \mathbf{R}_{\alpha } \Vert + \Vert ( \mathbf {e}_{out}^{(t)} )^T \Vert \le 2 k m^2 \sqrt{m} \sigma B + \sqrt{m} B + 2 k m \sqrt{m} B t + 2 \sqrt{2} k m^2(m + 1)^d \sigma B t \le B \cdot (m+1)^{O(d)} \le q/4$ with overwhelming probability, which ensures the correctness.

C Simulator Algorithms for Multi-hop AB-CPRE

We only present $\mathbf { ReKeyGen }_{SIM}$ for multi-hop scheme and the other simulator algorithms can be found in the full version [20].

• $\mathbf { ReKeyGen }_{SIM}( pp , pk_{\beta } , f ,\mathbf{y} )$: Parse $pp = (\lbrace \mathbf{B}_i \rbrace _{i \in [\ell ]}, \chi )$, $pk_{\beta } = ( \mathbf{A}_{\beta } , \mathbf{D}_{\beta } )$, a policy $f \in \mathcal {F}_{\ell , d}$ and an attribute vector $\mathbf{y} = \lbrace y_i \rbrace _{i \in [\ell ]}$. Compute $\mathbf{S}_f^*$ by executing $\mathbf {Eval}_{ \mathrm {sim} }( f , (x_i^* , \mathbf{S}_i^*)_{i \in [\ell ] } , \mathbf{A}_{\theta })$, sample matrices $\mathbf{E}_1 \leftarrow \chi ^{2km \times n}$, $\mathbf{E}_2 , \mathbf{E}_3 \leftarrow \chi ^{2 km \times m}$.

  • 1) In the case that $f( \mathbf{x}^* ) \ne 0$, let $\mathbf{B}_f = \mathbf{A}_{\theta } \mathbf{S}_f^* - f( \mathbf{x}^* ) \mathbf{G}$ and set $\mathbf{F} = [ \mathbf{A}_{\theta } | \mathbf{B}_f - f( \mathbf{x}^* ) \mathbf{G} ] \in \mathbb {Z}^{n \times 2m}$. Compute the basis $\mathbf{T}_{ \theta , f}$ for $\mathbf{F}$ as $\mathbf{T}_{ \theta , f} \leftarrow \mathbf {ExtendLeft}( \mathbf{A}_{\theta } , \mathbf{G} , \mathbf{T}_{\mathbf{G}}, \mathbf{S}_f )$. Then generate a matrix $\mathbf{R}_{ \theta , f } \in \mathbb {Z}^{2m \times m}$ such that $\mathbf{F} \mathbf{R}_{ \theta , f } = - \mathbf{D}_{\theta }$ by executing $\mathbf {SamplePre}( \mathbf{F} , \mathbf{T}_{ \theta , f} , - \mathbf{D}_{\theta } , \sigma )$, set $\overline{\mathbf{R}}_{\alpha ,f } = \mathbf {Power2}_q( \mathbf{R}_{ \alpha , f } )$, and compute

    $$\mathbf{Q} = \begin{bmatrix} \mathbf{E}_1 \mathbf{A}_{\beta } + \mathbf{E}_2 &{} \mathbf{E}_1 \mathbf{D}_{\beta } + \mathbf{E}_3 + \overline{ \mathbf{R} }_{ \theta , f } \\ \mathbf{0}_{m \times m} &{} \mathbf{I}_{m \times m} \end{bmatrix} \in \mathbb {Z}_q^{ (2km + m ) \times 2m}.$$

  • 2) In the case that $f( \mathbf{x}^* ) = 0$, choose a matrices $\mathbf{M} \leftarrow \mathbb {Z}^{ 2km \times m }$ uniformly at random, and compute

    $$\mathbf{Q} = \begin{bmatrix} \mathbf{E}_1 \mathbf{A}_{\beta } + \mathbf{E}_2 &{} \mathbf{M} \\ \mathbf{0}_{m \times m} &{} \mathbf{I}_{m \times m} \end{bmatrix} \in \mathbb {Z}_q^{ (2km + m ) \times 2m}.$$

  If $\mathbf{y}$ is none or null, then set $\mathbf{P}$ as a null matrix. Otherwise, samples $\ell$ matrices $\mathbf{E}_{B_i}$ from error distribution $\chi ^{2km \times m}$ and compute,

  

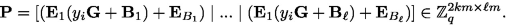
  Output $rk_{ \theta ,f \rightarrow \beta , \mathbf{y} } = ( \mathbf{Q} , \mathbf{P} )$ as re-encryption key.