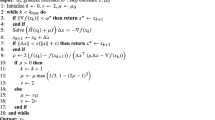Abstract
We present an improved variant of the matrix-triangularization subresultant prs method [1] for the computation of a greatest common divisor of two polynomialsA andB (of degreesm andn, respectively) along with their polynomial remainder sequence. It is improved in the sense that we obtain complete theoretical results, independent of Van Vleck’s theorem [13] (which is not always true [2, 6]), and, instead of transforming a matrix of order 2·max(m, n) [1], we are now transforming a matrix of orderm+n. An example is also included to clarify the concepts.
Abstract
Представлен улучшенный вариант матрично-грианіулярннационноіо субрезультантного метода пояиномиальных носледовательностей остатков (ППО) [1] для вычисления наибольшего общеіо дедителя двух мноточленовA иB (стеиенейm иn соответственно) с одновременным нахождением нх ПОП. Улучшение заключается в том, что нолучены законченные теоретические результаты, независимые от теоремы Ван Влека [13] (которая нэ всегда снраведлива, см [2, 6]). Кроме того, вместо преобразования матрицы норядка 2 · мах (m, n) [1] тенерь нреобразуется матрица норядкаm+n. Представлен численный нример для иллюстрации этих ноложений.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Akritas, A. G.A new method for computing polynomial greatest common divisors and polynomial remainder sequences. Numerische Mathematik52 (1988), pp. 119–127.
Akritas, A. G.,Elements of computer algebra with applications. J. Wiley Interscience, New York, 1989.
Akritas, A. G.Exact algorithms for the matrix-triangularization subresultant prs method. In: “Proceedings of the Conference on Computers and Mathematics”, Boston, Massachusetts, June 1989, pp. 145–155.
Bareiss, E. H.Sylvester’s identity and multistep integer-preserving Gaussian elimination. Mathematics of Computation22 (1968), pp. 565–578.
Dodgson, C. L.,Condensation of determinants. Proceedings of the Royal Society of London15, (1866), pp. 150–155.
González, L., Lombardi, H., Recio, T., and Roy, M-F.Spécialization de la suite de Sturm et sousrésultants. University of Cantabria, Department of Mathematics, Statistics and Computation, Technical Report 8-1990, S-39071, Santander, Spain.
Habicht, W.,Eine Verallgemeinerung des Sturmschen Wurzelzaelverfahrens, Commentarii Mathematici Helvetici21 (1948), pp. 99–116.
Kowalewski, G.,Einfürung in die Determinantentheorie. Chelsea, New York, 1948.
Malaschonok, G. I.Solution of a system of linear equations in an integral domain. Journal of Computational Mathematics and Mathematical Physics23 (1983), pp. 1497–1500 (in Russian).
Malaschonok, G. I.,System of linear equations over a commutative ring. Academy of Sciences of Ukraine, Lvov, 1986 (in Russian).
Sylvester, J. J.On the relation between the minor determinants of linearly equivalent quadratic functions. Philosophical Magazine1 (Fourth Series) (1851), pp. 259–305.
Sylvester, J. J.On a theory of the syzygetic relations of two rational integral functions, comprising an application to the theory of Sturm’s functions, and that of the greatest common measure. Philosophical Transactions143 (1853), pp. 407–548.
Van Vleck, E. B.On the determination of a series of Sturm’s functions by the calculation of a single determinant. Annals of Mathematics1 (1899–1900), Second Series, pp. 1–13.
Waugh, F. V. and Dwyer, P. S.Compact computation of the inverse of a matrix. Annals of Mathematical Statistics16, (1945), pp. 259–271.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Akritas, A.G., Akritas, E.K. & Malaschonok, G.I. Matrix computation of subresultant polynomial remainder sequences in integral domains. Reliable Comput 1, 375–381 (1995). https://doi.org/10.1007/BF02391682
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF02391682