Design of Low-redundant Cosine-modulated Nonuniform Filter Bank with Flexible Frequency Division

Abstract

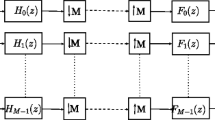
In this paper, a low-redundant cosine-modulated nonuniform filter bank (LR-CMNFB) and its design method are proposed. The proposed LR-CMNFB consists of two parallel subsystems which have the same frequency division scheme. The nonuniform analysis and synthesis filters of the first subsystem are formed by directly merging the consecutive cosine-modulated versions of a lowpass prototype filter, and the filters of the second subsystem are derived to meet the aliasing cancelation condition. Since the aliasing of the two subsystems is structurally canceled without guardband constraint on the filter location, the shift-invariance and flexible nonuniform frequency division, two important properties in many signal and image processing applications, can be achieved simultaneously at the cost of low redundancy (that is less than 2). From a particular analysis on the filter characteristic and the reconstruction error of the whole system, it is found that the good characteristics of filters and the good reconstruction performance of LR-CMNFB can be jointly obtained by constraining the prototype filter to be the linear-phase spectral factor of a 2 Mth band filter. Several design examples are given to illustrate the performance of the proposed LR-CMNFB and its potential in practical applications.

Appendices

Appendix A

In this appendix, we give the detailed explanation of (13). Based on (12), $z^{ - N} H_{k} (z^{ - 1} )$ has the following expression,

$$ z^{ - N} H_{k} (z^{ - 1} ) = z^{ - N} \sqrt {\frac{{d_{k} }}{2}} \sum\limits_{{i = m_{k - 1} p_{k - 1} }}^{{m_{k} p_{k} - 1}} {\left[ {e^{{ - j\beta_{i} }} U_{i}^{*} (z^{ - 1} ) + e^{{j\beta_{i} }} U_{i} (z^{ - 1} )} \right]} . $$

(37)

$U_{i}^{*} (z^{ - 1} )$ has the same magnitude response with $U_{i} (z)$, and $U_{i} (z^{ - 1} )$ has the same magnitude response with $U_{i}^{*} (z)$. Observing (12) and (37), it can be seen that, in order for $F_{k} (z)$ to be the time-reversed version of $H_{k} (z)$, that is, $F_{k} (z) = z^{ - N} H_{k} (z^{ - 1} )$, the following condition should be satisfied,

$$ e^{{j\gamma_{i} }} U_{i} (z) = z^{ - N} e^{{ - j\beta_{i} }} U_{i}^{*} (z^{ - 1} ). $$

(38)

Substituting (9) into (38) yields

$$ e^{{j\gamma_{i} }} P\left( {zW_{2M}^{(i + 0.5)} } \right) = z^{ - N} e^{{ - j\beta_{i} }} P\left( {z^{ - 1} W_{2M}^{ - (i + 0.5)} } \right). $$

(39)

Since $P(z)$ is a linear-phase lowpass filter, it is related as

$$ P(z) = z^{ - N} P(z^{ - 1} ). $$

(40)

Replacing z with $zW_{2M}^{(i + 0.5)}$ in (40), we have

$$ P\left( {zW_{2M}^{i + 0.5} } \right) = z^{ - N} W_{2M}^{ - (i + 0.5)N} P\left( {z^{ - 1} W_{2M}^{ - (i + 0.5)} } \right). $$

(41)

Substituting (41) into (39), the condition (37) finally becomes

$$ e^{{j(\beta_{i} + \gamma_{i} )}} = W_{2M}^{(i + 0.5)N} . $$

(42)

Appendix B

This appendix gives the derivation of (33). Since $P(e^{j\omega } )$ is a linear-phase lowpass filter and has the order of $2L$, $G(e^{j\omega } ) = |P(e^{j\omega } )|^{2}$ has zero phase and the order of $4L$. Substituting (31), $G(e^{j\omega } )$ can be written as,

$$ \begin{aligned} G(e^{j\omega } ) & = P(e^{j\omega } ) \cdot P^{*} (e^{ - j\omega } ) \\ & = e^{ - j\omega L} {\mathbf{b}}^{T} {\boldsymbol{\upvarphi }}(\omega ) \cdot {\boldsymbol{\upvarphi }}^{T} ( - \omega ){\mathbf{b}}e^{j\omega L} \\ & = {\mathbf{b}}^{T} \underbrace {{{\boldsymbol{\upvarphi }}(\omega ){\boldsymbol{\upvarphi }}^{T} ( - \omega )}}_{{{\varvec{\Phi}}(\omega )}}{\mathbf{b}}, \\ \end{aligned} , $$

(43)

where ${\varvec{\Phi}}(\omega )$ is a $(L + 1) \times (L + 1)$ matrix. Since ${\boldsymbol{\upvarphi }}(\omega ) = [1,\cos \omega , \ldots ,\cos (L\omega )]^{T} ,$ the $(i,j)$ th element of ${\varvec{\Phi}}(\omega )$ is computed as

$$ \begin{aligned} {\varvec{\Phi}}_{i,j} (\omega ) & = \cos (i\omega )\cos (j\omega ) \\ & = \frac{1}{2}\left[ {\cos \left( {(i + j)\omega } \right) + \cos \left( {(i - j)\omega } \right)} \right], \\ \end{aligned} $$

(44)

which indicates that the position $(i,j)$ of the term $\cos (n\omega )$ for $n = 0,1, \ldots ,2L$ in ${\varvec{\Phi}}(\omega )$ satisfies $i + j = n$ or $|i - j| = n$. Accordingly, it is straightforward to get the following expression,

$$ {\varvec{\Phi}}(\omega ) = \sum\limits_{n = 0}^{2L} {{\mathbf{S}}_{n} \cos (n\omega )} , $$

(45)

where ${\mathbf{S}}_{n}$ is a $(L + 1) \times (L + 1)$ matrix with the $(i,j)$ th element ${\mathbf{S}}_{n} (i,j) = 0.5\left[ {\delta (i + j - n) + \delta (|i - j| - n)} \right]$. Substituting (45) into (43), we have

$$ \begin{aligned} G\left( {e^{j\omega } } \right) & = {\mathbf{b}}^{T} \left[ {\sum\limits_{n = 0}^{2L} {{\mathbf{S}}_{n} \cdot \cos (n\omega )} } \right]{\mathbf{b}} \\ & = \sum\limits_{n = 0}^{2L} {{\mathbf{b}}^{T} {\mathbf{S}}_{n} {\mathbf{b}} \cdot \cos (n\omega )} , \\ \end{aligned} $$

(46)

which is just the expression of (33).