Abstract
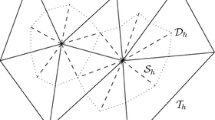
We present a patch-based equilibrated flux recovery procedure for the conforming finite element approximation to diffusion problems. The recovered flux is computed as the solution to a local constraint-free minimization problem on each patch. The approach is valid for higher order conforming elements in both two and three dimensions. The resulting estimator admits guaranteed reliability and the robust local efficiency is proved under the quasi-monotonicity condition of the diffusion coefficient. Numerical experiments are given to confirm the theoretical results.




Similar content being viewed by others
References
Ainsworth, M., Oden, J.T.: A Posteriori Error Estimation in Finite Element Analysis. Wiley, Wiley (2000)
Bank, R., Xu, J., Zheng, B.: Superconvergent derivative recovery for Lagrange triangular elements of degree p on unstructured grids. SIAM J. Numer. Anal. 45(5), 2032–2046 (2007)
Bernardi, C., Verfürth, R.: Adaptive finite element methods for elliptic equations with non-smooth coefficients. Numer. Math. 85(4), 579–608 (2000)
Boffi, D., Fortin, M., Brezzi, F.: Mixed Finite Element Methods and Applications. Springer Series in Computational Mathematics. Springer, Berlin (2013)
Braess, D.: Finite Elements: Theory, Fast Solvers, and Applications in Solid Mechanics. Cambridge University Press, Cambridge (2007)
Braess, D., Pillwein, V., Schöberl, J.: Equilibrated residual error estimates are p-robust. Comput. Methods Appl. Mech. Eng. 198(13), 1189–1197 (2009)
Braess, D., Schöberl, J.: Equilibrated residual error estimator for edge elements. Math. Comput. 77(262), 651–672 (2008)
Braess, D., Verfürth, R.: A posteriori error estimators for the Raviart–Thomas element. SIAM J. Numer. Anal. 33(6), 2431–2444 (1996)
Cai, D., Cai, Z.: A hybrid a posteriori error estimator for conforming finite element approximations. Comput. Methods Appl. Mech. Eng. 339, 320–340 (2018)
Cai, Z., Zhang, S.: Recovery-based error estimator for interface problems: conforming linear elements. SIAM J. Numer. Anal. 47(3), 2132–2156 (2009)
Cai, Z., Zhang, S.: Flux recovery and a posteriori error estimators: conforming elements for scalar elliptic equations. SIAM J. Numer. Anal. 48(2), 578–602 (2010)
Cai, Z., Zhang, S.: Robust equilibrated residual error estimator for diffusion problems: conforming elements. SIAM J. Numer. Anal. 50(1), 151–170 (2012)
Cochez-Dhondt, S., Nicaise, S.: Equilibrated error estimators for discontinuous Galerkin methods. Numer. Methods Partial Differ. Equ. 24(5), 1236–1252 (2008)
Dörfler, W.: A convergent adaptive algorithm for Poisson’s equation. SIAM J. Numer. Anal. 33(3), 1106–1124 (1996)
Ern, A., Vohralík, M.: Stable broken $H^1$ and ${\bf H}({\rm div})$ polynomial extensions for polynomial-degree-robust potential and flux reconstruction in three space dimensions. ArXiv e-prints (2017)
Kellogg, R.B.: On the Poisson equation with intersecting interfaces. Appl. Anal. 4(2), 101–129 (1974)
Ladeveze, P., Leguillon, D.: Error estimate procedure in the finite element method and applications. SIAM J. Numer. Anal. 20(3), 485–509 (1983)
Luce, R., Wohlmuth, B.: A local a posteriori error estimator based on equilibrated fluxes. SIAM J. Numer. Anal. 42(4), 1394–1414 (2004)
Morin, P., Nochetto, R.H., Siebert, K.G.: Convergence of adaptive finite element methods. SIAM Rev. 44(4), 631–658 (2002)
Naga, A., Zhang, Z.: The polynomial-preserving recovery for higher order finite element methods in 2D and 3D. Discrete Contin. Dyn. Syst. Ser. B 5(3), 769 (2005)
Petzoldt, M.: A posteriori error estimators for elliptic equations with discontinuous coefficients. Adv. Comput. Math. 16(1), 47–75 (2002)
Prager, W., Synge, J.L.: Approximations in elasticity based on the concept of function space. Q. Appl. Math. 5, 241–269 (1947)
Sewell, E.G.: Automatic generation of triangulations for piecewise polynomial approximation. Ph.D. thesis, Purdue University, West Lafayette, IN (1972)
Verfürth, R.: A note on constant-free a posteriori error estimates. SIAM J. Numer. Anal. 47(4), 3180–3194 (2009)
Verfürth, R.: A Posteriori Error Estimation Techniques for Finite Element Methods. Numerical Mathematics and Scientific Computation. OUP, Oxford (2013)
Ziegler, G.M.: Lectures on Polytopes, vol. 152. Springer, New York (2012)
Zienkiewicz, O.C., Zhu, J.Z.: A simple error estimator and adaptive procedure for practical engineerng analysis. Int. J. Numer. Methods Eng. 24(2), 337–357 (1987)
Zienkiewicz, O.C., Zhu, J.Z.: The superconvergent patch recovery and a posteriori error estimates. Part 1. The recovery technique. Int. J. Numer. Methods Eng. 33(7), 1331–1364 (1992)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Zhiqiang Cai: This work was supported in part by the National Science Foundation under Grant DMS-1522707. Shun Zhang: This work was supported in part by Hong Kong Research Grants Council under the GRF Grant Project No. 11305319, CityU 9042090.
Rights and permissions
About this article
Cite this article
Cai, D., Cai, Z. & Zhang, S. Robust equilibrated a posteriori error estimator for higher order finite element approximations to diffusion problems. Numer. Math. 144, 1–21 (2020). https://doi.org/10.1007/s00211-019-01075-1
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00211-019-01075-1