Smallest tournaments not realizable by ${\frac{2}{3}}$-majority voting

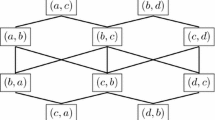
Abstract

Define the predictability number α(T) of a tournament T to be the largest supermajority threshold ${\frac{1}{2} < \alpha\leq 1}$ for which T could represent the pairwise voting outcomes from some population of voter preference orders. We establish that the predictability number always exists and is rational. Only acyclic tournaments have predictability 1; the Condorcet voting paradox tournament has predictability ${\frac{2}{3}}$; Gilboa has found a tournament on 54 alternatives (i.e. vertices) that has predictability less than ${\frac{2}{3}}$ , and has asked whether a smaller such tournament exists. We exhibit an 8-vertex tournament that has predictability ${\frac{13}{20}}$ , and prove that it is the smallest tournament with predictability < ${\frac{2}{3}}$ . Our methodology is to formulate the problem as a finite set of two-person zero-sum games, employ the minimax duality and linear programming basic solution theorems, and solve using rational arithmetic.