A static boundary element solution for Bickford–Reddy beam

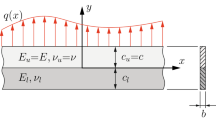
Abstract.

Bickford–Reddy beam theory is a refined model in which it is assumed that axial displacements vary cubically across the height of the cross section. Consequently, quadratic distribution for transverse shear stresses is automatically satisfied instead of Timoshenko beam model which predicts a constant distribution for those stresses, requiring the incorporation of a shear correction factor into the model. This article shows a new static solution which is based on direct boundary element method (BEM) for Bickford–Reddy beam theory. Mathematical steps required by this BEM technique are adequately addressed, for instance, a) integral equations are derived using Betti’s reciprocal theorem, b) fundamental solutions are obtained from the fundamental problem which has direct relationship to the real Bickford–Reddy beam problem; c) explicit influence matrices and load vectors are derived from source collocation at boundary points, and then at domain points, and d) BEM solutions are obtained for structures containing domain discontinuities such as stepped beams and continuous beams. Numerical results are presented for uniform beams having rectangular and circular cross sections and for problems having domain discontinuities.

Appendices

Appendix 1

The higher derivatives of function $\psi$ used in Eqs. (27) and (28) are

$$\begin{aligned} {\text{d}}\psi /{\text{d}}x & = \left[ {C_{1} + 3C_{2} r^{2} - C_{3} \sqrt y e^{ - r\sqrt y } } \right]sgn\left( {x,\hat{x}} \right), \\ {\text{d}}^{2} \psi /{\text{d}}x^{2} & = 6C_{2} r + C_{3} ye^{ - r\sqrt y } , \\ {\text{d}}^{3} \psi /{\text{d}}x^{3} & = \left[ {6C_{2} - C_{3} y\sqrt y e^{ - r\sqrt y } } \right]sgn\left( {x,\hat{x}} \right), \\ {\text{d}}^{4} \psi /{\text{d}}x^{4} & = C_{3} y^{2} e^{ - r\sqrt y } , \\ {\text{d}}^{5} \psi /{\text{d}}x^{5} & = \left[ { - C_{3} y^{2} \sqrt y e^{ - r\sqrt y } } \right]sgn\left( {x,\hat{x}} \right), \\ {\text{d}}^{6} \psi /{\text{d}}x^{6} & = C_{3} y^{3} e^{ - r\sqrt y } - 2\delta \left( {x,\hat{x}} \right)C_{3} y^{2} \sqrt y e^{ - r\sqrt y } , \\ \end{aligned}$$

and ${\text{sgn}}\left( {x,\hat{x}} \right)$ is the sign function defined by

$${\text{sgn}}\left( {x,\hat{x}} \right) = \left\{ {\begin{array}{*{20}l} {1, \quad {\text{if}} \quad x > \hat{x}} \\ { - 1, \quad {\text{if}} \quad x < \hat{x}} \\ \end{array} } \right..$$

Appendix 2

The functions $\eta ij\left( r \right)$ and βij(r) used in Eqs. (39) and (40) are given by

$$\begin{aligned} \eta_{11} \left( r \right) & = \left( {\alpha^{2} \hat{F}_{x}^{2} - \alpha^{2} \bar{D}_{x} H_{x} } \right){\text{d}}^{5} \psi \left( r \right)/{\text{d}}r^{5} + \left( {2\alpha \bar{A}_{xz} \hat{F}_{x} + \alpha^{2} \bar{A}_{xz} H_{x} + \bar{A}_{xz} \bar{D}_{x} } \right){\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} , \\ \eta_{21} \left( r \right) & = - \left( {\alpha^{2} \hat{F}_{x}^{2} - \alpha^{2} \bar{D}_{x} H_{x} } \right){\text{d}}^{6} \psi \left( r \right)/{\text{d}}r^{6} - \left( {2\alpha \bar{A}_{xz} \hat{F}_{x} + \alpha^{2} \bar{A}_{xz} H_{x} + \bar{A}_{xz} \bar{D}_{x} } \right){\text{d}}^{4} \psi \left( r \right)/{\text{d}}r^{4} , \\ \eta_{31} \left( r \right) & = 0, \\ \eta_{12} \left( r \right) & = \left( {\alpha^{2} \hat{F}_{x}^{2} - \alpha^{2} \bar{D}_{x} H_{x} } \right)d^{4} \psi \left( r \right)/{\text{d}}r^{4} + \left( {\alpha \bar{A}_{xz} \hat{F}_{x} + \alpha^{2} \bar{A}_{xz} H_{x} } \right){\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} , \\ \eta_{22} \left( r \right) & = - \left( {\alpha^{2} \hat{F}_{x}^{2} - \alpha^{2} \bar{D}_{x} \cdot H_{x} } \right){\text{d}}^{5} \psi \left( r \right)/{\text{d}}r^{5} - \left( {\alpha \bar{A}_{xz} \hat{F}_{x} + \alpha^{2} \bar{A}_{xz} H_{x} } \right){\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} , \\ \eta_{32} \left( r \right) & = \left( {\bar{A}_{xz} \hat{F}_{x} + \alpha^{2} \bar{A}_{xz} H_{x} } \right){\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} , \\ \eta_{13} \left( r \right) & = \left( {\bar{D}_{x} \bar{A}_{xz} + \alpha \bar{A}_{xz} \hat{F}_{x} } \right){\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} , \\ \eta_{23} \left( r \right) & = - \left( {\bar{D}_{x} \cdot \bar{A}_{xz} + \alpha \cdot \bar{A}_{xz} \cdot \hat{F}_{x} } \right){\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} , \\ \eta_{33} \left( r \right) & = \left( { - \alpha^{2} \bar{D}_{x} H_{x} + \alpha^{2} \hat{F}_{x}^{2} } \right){\text{d}}^{5} \psi \left( r \right)/{\text{d}}r^{5} + \left( {\bar{A}_{xz} \bar{D}_{x} + \alpha \bar{A}_{xz} \hat{F}_{x} } \right){\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} , \\ \beta_{11} \left( {\text{r}} \right) & = \bar{D}_{x} {\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} - \bar{A}_{xz} \psi \left( r \right), \\ \beta_{21} \left( {\text{r}} \right) & = - \bar{D}_{x} {\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} + \bar{A}_{xz} {\text{d}}\psi \left( r \right)/{\text{d}}r, \\ \beta_{31} \left( r \right) & = - \hat{F}_{x} \alpha {\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} - \bar{A}_{xz} {\text{d}}\psi \left( r \right)/{\text{d}}r, \\ \beta_{12} \left( {\text{r}} \right) & = \bar{D}_{x} {\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} - \bar{A}_{xz} {\text{d}}\psi \left( r \right)/{\text{d}}r, \\ \beta_{22} \left( {\text{r}} \right) & = - \bar{D}_{x} {\text{d}}^{4} \psi \left( r \right)/{\text{d}}r^{4} + \bar{A}_{xz} {\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} , \\ \beta_{32} \left( {\text{r}} \right) & = - \hat{F}_{x} \cdot \alpha {\text{d}}^{4} \psi \left( r \right)/{\text{d}}r^{4} - \bar{A}_{xz} {\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} , \\ \beta_{13} \left( {\text{r}} \right) & = \bar{A}_{xz} {\text{d}}\psi \left( r \right)/{\text{d}}r + \hat{F}_{x} \alpha {\text{d}}^{3} \psi \left( r \right)/{\text{d}}r^{3} , \\ \beta_{23} \left( {\text{r}} \right) & = - \bar{A}_{xz} {\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} - \hat{F}_{x} \cdot \alpha {\text{d}}^{4} \psi \left( r \right)/{\text{d}}r^{4} , \\ \beta_{33} \left( {\text{r}} \right) & = - H_{x} \alpha^{2} {\text{d}}^{4} \psi \left( r \right)/{\text{d}}r^{4} + \bar{A}_{xz} {\text{d}}^{2} \psi \left( r \right)/{\text{d}}r^{2} . \\ \end{aligned}$$

Appendix 3

The results of the integrations in Eqs. (41) and (42) are depending on a relative position between $\hat{x}$ and x. If $\hat{x} > x$

$$\begin{aligned} {\text{g}}_{{0{\text{a}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{0}^{{\hat{x}}} \psi \left( {r = \hat{x} - x} \right){\text{d}}x = C_{3} /\sqrt y \left( {1 - e^{{ - \hat{x}\sqrt y }} } \right) + C_{1} \hat{x}^{2} /2 + C_{2} \hat{x}^{4} /4, \\ {\text{g}}_{{1{\text{a}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{0}^{{\hat{x}}} \left[ {{\text{d}}\psi \left( {r = \hat{x} - x} \right)/{\text{d}}r} \right]{\text{d}}x = C_{3} (e^{{ - \hat{x}\sqrt y }} - 1) + \hat{x}^{3} C_{2} + \hat{x}C_{1} , \\ {\text{g}}_{{2{\text{a}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{0}^{{\hat{x}}} \left[ {{\text{d}}^{2} \psi \left( {r = \hat{x} - x} \right)/{\text{d}}r^{2} } \right]{\text{d}}x = 3\hat{x}^{2} C_{2} + C_{3} \sqrt y \left( {1 - e^{{ - \hat{x}\sqrt y }} } \right), \\ {\text{g}}_{{3{\text{a}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{0}^{{\hat{x}}} \left[ {{\text{d}}^{3} \psi \left( {r = \hat{x} - x} \right)/{\text{d}}r^{3} } \right]{\text{d}}x = 6C_{2} \hat{x} + C_{3} y\left( {e^{{ - \hat{x}\sqrt y }} - 1} \right), \\ {\text{g}}_{{4{\text{a}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{0}^{{\hat{x}}} \left[ {{\text{d}}^{4} \psi \left( {r = \hat{x} - x} \right)/{\text{d}}r^{4} } \right]{\text{d}}x = - C_{3} \cdot \sqrt {y^{3} } \left( {e^{{ - \hat{x}\sqrt y }} - 1} \right) . \\ \end{aligned}$$

Otherwise,

$$\begin{aligned} {\text{g}}_{{0{\text{d}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{{\hat{x}}}^{L} \psi \left( {r = - \hat{x} + x} \right){\text{d}}x = C_{3} /\sqrt y \left( {1 - e^{{ - \left( {L - \hat{x}} \right)\sqrt y }} } \right) + C_{1} \left( {L - \hat{x}} \right)^{2} /2 + C_{2} \left( {L - \hat{x}} \right)^{4} /4, \\ {\text{g}}_{{1{\text{d}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{{\hat{x}}}^{L} \left[ {{\text{d}}\psi \left( {r = - \hat{x} + x} \right)/{\text{d}}r} \right]{\text{d}}x = \left( {e^{{ - \left( {L - \hat{x}} \right)\sqrt y }} - 1} \right)C_{3} + \left( {L - \hat{x}} \right)^{3} C_{2} + \left( {L - \hat{x}} \right)C_{1} , \\ {\text{g}}_{{2{\text{d}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{{\hat{x}}}^{L} \left[ {{\text{d}}^{2} \psi \left( {r = - \hat{x} + x} \right)/{\text{d}}r^{2} } \right]{\text{d}}x = 3\left( {L - \hat{x}} \right)^{2} C_{2} + C_{3} \sqrt y \left( {1 - e^{{ - \left( {L - \hat{x}} \right)\sqrt y }} } \right), \\ {\text{g}}_{{3{\text{d}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{{\hat{x}}}^{L} \left[ {{\text{d}}^{3} \psi \left( {r = - \hat{x} + x} \right)/{\text{d}}r^{3} } \right]{\text{d}}x = 6 \cdot C_{2} \cdot \left( {L - \hat{x}} \right) + C_{3} \cdot y\left( {e^{{ - \left( {L - \hat{x}} \right)\sqrt y }} - 1} \right), \\ {\text{g}}_{{4{\text{d}}}} \left( {\hat{x}} \right) & = \mathop \smallint \limits_{{\hat{x}}}^{L} \left[ {{\text{d}}^{4} \psi \left( {r = - \hat{x} + x} \right)/{\text{d}}r^{4} } \right]{\text{d}}x, \\ & = - C_{3} \sqrt {y^{3} } \left( {e^{{ - \left( {L - \hat{x}} \right)\sqrt y }} - 1} \right). \\ \end{aligned}$$

Appendix 4

Consider a cantilever beam under concentrated load ${\text{Q}}_{\text{L}}$ at its tip. Four distinct analytical solutions for transverse displacement are discussed based on beam theories and elasticity. For Euler and Timoshenko beam models, displacements $w_{E} \left( x \right)$and $w_{T} \left( x \right)$ are [11]

$$w_{E} \left( x \right) = {\text{Q}}_{\text{L}} x^{2} \left( {3L - x} \right)/\left( {6D_{x} } \right),$$

(50)

$$w_{T} \left( x \right) = w_{E} \left( x \right) + {\text{Q}}_{\text{L}} x/\left( {kA_{xz} } \right).$$

(51)

According to Hutchinson [25], the shear correction factors of rectangular and circular cross sections are, respectively, given by $k = 5\left( {1 + \nu } \right)/\left( {6 + 5\nu } \right)$ and $k = 6\left( {1 + \nu } \right)^{2} /\left( {7 + 12\nu + 4\nu^{2} } \right)$. For a rectangular cross section, transverse displacement $w_{B} \left( x \right)$ of the Bickford beam is [16]

$$w_{B} \left( x \right) = {\text{Q}}_{\text{L}} \cdot {\text{L}}^{3} \left( {1 + \nu } \right)\left( {{\text{h}}/{\text{L}}} \right)^{2} \left\{ {{\text{x}}/{\text{L}} - \left[ {\sinh \left( {\eta _{1} \cdot {\text{L}}} \right) - \sinh \left( {\eta _{1} \cdot \left( {{\text{L}} - {\text{x}}} \right)} \right)} \right]} \right\}/ \propto_{1} + {\text{Q}}_{\text{L}} \cdot {\text{L}}^{3} \left( {{\text{x}}/{\text{L}}} \right)^{2} \left[ {3/2 - {\text{x}}/\left( {2{\text{L}}} \right)} \right]/\left( {3D_{x} } \right) ,$$

(52)

with $\eta_{1} = \left( {1/h} \right)\left( {420/\left( {1 + \nu } \right)} \right)^{{{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} ,$

$$\propto_{1} = \left[ {\eta_{1} .{\text{L}}.{ \cosh }\left( {\eta _{1} .{\text{L}}} \right)} \right]\} /\left( {5D_{x} } \right).$$

Different from beam solutions given in Eq. (50), (51), (52), plane stress elasticity solutions are now discussed assuming the right end fixed, see Fig. 5c. According to Timoshenko and Goodier [26], axial and transverse displacements for a rectangular cross-sectional beam after setting $u\left( {L,0} \right) = w\left( {L,0} \right) = 0$ are given as follows:

$$u\left( {x,z_{1} } \right) = - {\text{Q}}_{\text{L}} x^{2} z_{1} /\left( {2D_{x} } \right) - \nu {\text{Q}}_{\text{L}} z_{1}^{3} /\left( {6D_{x} } \right) + {\text{Q}}_{\text{L}} z_{1}^{3} /\left( {6GI} \right) + B_{2} z_{1} ,$$

(53)

$$\begin{aligned} w\left( {x,z_{1} } \right) & = \nu {\text{Q}}_{\text{L}} xz_{1}^{2} /\left( {2D_{x} } \right) + {\text{Q}}_{\text{L}} x^{3} /\left( {6D_{x} } \right) \\ & \quad + B_{1} \left( {{\text{x}} - {\text{L}}} \right) - {\text{Q}}_{\text{L}} L^{2} /\left( {6D_{x} } \right). \\ \end{aligned}$$

(54)

The constants $B_{1}$ and $B_{2}$ can be determined using a third constraint condition to eliminate rotation of the $xz_{1}$ plane about centroidal fixed point. In Ref. [26], a condition $\partial u/\partial z_{{1\left( {x = L,,z = 0} \right)}} = 0$ is set, resulting $B_{2} = - {\text{Q}}_{\text{L}} L^{2} /\left( {2D_{x} } \right)$ and $B_{1} = - {\text{Q}}_{\text{L}} \left[ {L^{2} /(2D_{x} } \right) + h^{2} /\left( {8{\text{GI}}} \right)]$. So, assuming $\nu = 0$ and using Eq. (54) at centroid and $x = 0$ gives

$${\text{w}}\left( {0,0} \right) = {\text{Q}}_{\text{L}} \left[ {L^{3} + 2{\text{L}}\left( {h/2} \right)^{2} } \right]/\left( {3D_{x} } \right).$$

(55)

By back-substituting $B_{2}$ into Eq. (53), axial displacement at fixed end with $z_{1} = \pm h/2$ gives $u\left( {L, \pm h/2} \right) \ne 0.$

If an alternative third constraint condition given by Kaliakin [27], $u\left( {L,z_{1} = h/2} \right) = 0$ or $u\left( {L,z_{1} = - h/2} \right) = 0$ is used instead, the values of $B_{1}$ and $B_{2}$ using Eq. (53) and Eq. (54) yields $B_{2} = {\text{Q}}_{\text{L}} {\text{h}}\left[ {12L^{2} - \left( {2 + \nu } \right)h^{2} } \right]/(48D_{x} )$ and $B_{1} = - {\text{Q}}_{\text{L}} {\text{h}}\left[ {12L^{2} - \left( {2 + \nu } \right)h^{2} } \right]/(48D_{x} ) - \left( {1 + \nu } \right){\text{Q}}_{\text{L}} /\left( {4D_{x} } \right)$. Consequently, Eq. (54) at centroid with $x = L$ and assuming $\nu = 0$ gives

$${\text{w}}\left( {0,0} \right) = {\text{Q}}_{\text{L}} \left[ {L^{3} + 3{\text{L}}\left( {h/2} \right)^{2} } \right]/\left( {3D_{x} } \right).$$

(56)

For cylindrical beam problems, the left end is considered fixed for both beam and elasticity solutions. Transverse displacements of Bickford model is given by

$$w_{E} \left( x \right) = {\text{Q}}_{\text{L}} x^{2} \left( {3L - x} \right)/\left( {6D_{x} } \right) + \propto_{2} \left\{ {\lambda x - [\sinh \left( {\lambda x} \right) - \sinh \lambda \left( {L - x} \right)]/[\lambda \cosh \left( {\lambda x} \right)]} \right\},$$

(57)

where

$$\lambda = \sqrt {\bar{A}_{xz} \cdot D_{x} /\left[ {\alpha \left( {F_{x} \cdot \hat{D}_{x} - \hat{F}_{x} \cdot Dx} \right)} \right]} ,$$

$$\mu = \hat{A}_{xz} \hat{D}_{x} /[\alpha \left( {\hat{D}_{x} \cdot F_{x} - \hat{F}_{x} \cdot D_{x} } \right),$$

$$\propto_{2} = {\text{Q}}_{\text{L}} \hat{D}_{x}^{2} /\left( {\bar{A}_{xz} D_{x}^{2} } \right).$$

According to Ref. [26], non-zero stresses of a cylindrical beam with radius R under plane stress conditions are given, respectively, by

$$\begin{aligned} & \sigma_{x} = - {\text{Q}}_{\text{L}} \left( {{\text{L}} - {\text{x}}} \right)z_{1} /{\text{I,}} \hfill \\ & \sigma_{y} = \sigma_{{z_{1} }} = \tau_{{yz_{1} }} = 0, \hfill \\ & \tau_{xy} = - \left( {1 + 2\nu } \right){\text{Q}}_{\text{L}} yz_{1} /\left[ {4\left( {1 + \nu } \right)I} \right], \hfill \\ & \tau_{xz} = \propto_{7} {\text{Q}}_{\text{L}} \left[ {R^{2} - z_{1}^{2} - \left( {1 - 2\nu } \right)y^{2} / \propto_{7} } \right]/ \propto_{8} , \hfill \\ \end{aligned}$$

(58)

with $I = \pi R^{4} /4$ or $I = D_{x} /E,$

$$\propto_{7} = 3 + 2\nu ,$$

$$\propto_{8} = 8\left( {1 + \nu } \right)I.$$

Assuming $\nu = 0$ and using strain–displacement relations, Hooke’s law, and Eq. (58) gives

$$\begin{aligned} \partial u/\partial x = \varepsilon_{x} = \sigma_{x} /E = - {\text{Q}}_{\text{L}} z_{1} \left( {{\text{L}} - {\text{x}}} \right)/D_{x} , \hfill \\ \partial v/\partial y = \varepsilon_{y} = \sigma_{y} /E = 0, \hfill \\ \partial w/\partial z_{1} = \varepsilon_{{z_{1} }} = \sigma_{{z_{1} }} /E = 0, \hfill \\ \end{aligned}$$

(59)

$$\begin{aligned} \partial u/\partial z_{1} + \partial w/\partial x & = \gamma_{{xz_{1} }} = \tau_{{xz_{1} }} /\left( {E/2} \right) \\ & = 3{\text{Q}}_{\text{L}} \left( {R^{2} - z_{1}^{2} - y^{2} /3} \right)/\left( {4D_{x} } \right), \\ \partial v/\partial z_{1} + \partial w/\partial y & = \gamma_{{yz_{1} }} = \tau_{{yz_{1} }} /\left( {E/2} \right) = 0, \\ \partial u/\partial y + \partial v/\partial x & = \gamma_{xy} = \tau_{xy} /\left( {E/2} \right) = - {\text{Q}}_{\text{L}} yz_{1} /\left( {2D_{x} } \right). \\ \end{aligned}$$

(60)

After integrating properly Eq. (59), displacements can be given as follows:

$$\begin{aligned} v\left( {x,z_{1} } \right) = b\left( {x,z_{1} } \right), \hfill \\ w\left( {x,y} \right) = c\left( {x,y} \right),\,\,\,{\text{and}} \hfill \\u \left( {x,y,z_{1} } \right) = - {\text{Q}}_{\text{L}} z_{1} \left( {Lx - x^{2} /2} \right) + a\left( {y,z_{1} } \right). \hfill \\ \end{aligned}$$

(61)

If the resulting expressions obtained by substituting Eq. (61) into Eq. (60) are conveniently integrated, functions a, b, and c can be written as follows:

$$\begin{aligned} a\left( {y,z_{1} } \right) & = - C_{4} z_{1} + C_{6} z_{1} + C_{5} + \\ & \quad {\text{Q}}_{\text{L}} z\left( {R^{2} - y^{2} } \right)/\left( {4D_{x} } \right), \\ b\left( {x,z_{1} } \right) & = C_{1} z_{1} - C_{6} x + C_{2} , \\ c\left( {x,y} \right) & = - {\text{Q}}_{\text{L}} z_{1} x^{2} \left( {x - 3L} \right)/\left( {6D_{x} } \right) \\ & \quad - C_{1} y + C_{4} x + C_{3} . \\ \end{aligned}$$

(62)

Assuming no translational displacements at origin, three constants in Eqs. (61) and (62) can be determined, giving $C_{2} = C_{3} = C_{5} = 0.$ If the rigid body rotation components $\omega_{x} = \partial v/\partial z_{1} - \partial w/\partial y,$$\omega_{y} = \partial u/\partial z_{1} - \partial w/\partial x$, and $\omega_{{z_{1} }} = \partial u/\partial y - \partial v/\partial x$ are also assumed to be zero at origin, the remaining constants give $C_{1} = C_{6} = 0$ and $C_{4} = 3{\text{Q}}_{\text{L}} R^{2} /\left( {8D_{x} } \right)$. Consequently, the maximum transverse displacement at $x = L$ is

$$w_{max} = L{\text{Q}}_{\text{L}} \left[ {4L^{2} + 3R^{2} } \right]/(12D_{x} ).$$

(63)

By back-substituting the constants $C_{5} ,C_{6}$ and $C_{4}$ into Eq. (61), axial displacements at fixed end with $z_{1} = \pm R$ give non-zero values, which is, $u\left( {0,0, \pm R} \right) = \pm 3{\text{Q}}_{\text{L}} R^{3} /\left( {8D_{x} } \right)$.

If the constraint of rotation about the y-axis is set using $u = 0$ at point $\left( {x = 0, y = 0, z_{1} = R} \right)$, all values of the constants are maintained, except the fourth constant which gives $C_{4} = {\text{Q}}_{\text{L}} R^{2} /\left( {4D_{x} } \right)$. So, the maximum transverse displacement at $x = L$ is

$$w_{max} = L{\text{Q}}_{\text{L}} \left[ {4L^{2} + 6R^{2} } \right]/(12D_{x} ).$$

(64)

Appendix 5

Considering a cantilever beam under a uniformly distributed load $q_{0}$. In Ref. [11], analytical solutions of transverse displacement for Euler, Timoshenko, and Bickford beam models are

$$w_{E} \left( {\text{x}} \right) = {\text{q}}_{0} {\text{L}}^{4} \left( {6{\text{x}}^{2} /{\text{L}}^{2} - 4{\text{x}}^{3} /{\text{L}}^{3} + {\text{x}}^{4} /{\text{L}}^{4} } \right)/\left( {24{\text{D}}_{\text{x}} } \right),$$

(65)

$$w_{T} \left( {\text{x}} \right) = w_{E} \left( {\text{x}} \right) + {\text{q}}_{0} {\text{Lx}}\left( {2 - {\text{x}}/{\text{L}}} \right)/\left( {2{\text{kA}}_{\text{xz}} } \right),$$

(66)

$$\begin{aligned} w_{B} \left( x \right) & = \propto_{3} \left[ {\cosh \left( {\lambda x} \right) + \lambda L\sinh \left( {\lambda \left( {L - x} \right)} \right)} \right] \\ & \quad + w_{E} \left( x \right) + c, \\ \end{aligned}$$

(67)

where

$$c = - q_{0} \mu \hat{D}_{x} \left( {1 + \lambda \cdot L \cdot \sinh \left( {\lambda \cdot L} \right)} \right)/ \propto_{3} + \left( {2Lx - x^{2} } \right)/\left( {2\lambda^{2} \hat{A}_{xz} D_{x} } \right),$$

and coefficients $\lambda ,$$\mu ,$ and $\propto_{3}$ are given by

$$\begin{aligned} \propto_{3} & = q_{0} \mu \hat{D}_{x} /\left[ {\hat{A}_{xz} D_{x} \lambda^{4} \cosh \left( {\lambda L} \right)} \right], \\ \lambda & = \sqrt {\bar{A}_{xz} \cdot D_{x} /\left[ {\alpha \left( {F_{x} \cdot \hat{D}_{x} - \hat{F}_{x} \cdot Dx} \right)} \right]} , \\ \mu & = \hat{A}_{xz} \hat{D}_{x} /\left[ {\alpha \left( {\hat{D}_{x} \cdot F_{x} - \hat{F}_{x} \cdot D_{x} } \right)} \right]. \\ \end{aligned}$$

(68)

The coefficient $\mu$ given in Eq. (68) contains a slight correction from the expression found in Ref. [11, p.32].

Analogous to Appendix 4, plane stress elasticity solutions are discussed in this section assuming the right end fixed, see Fig. 5c. The analytical solutions given by Ding et al. [28] for axial and transverse displacements written in terms of set of the undetermined coefficients are

$$\begin{aligned} u\left( {x,z_{1} } \right) & = \left[ {2a\left( {2 + \nu } \right)xz_{1}^{3} + 2dx - 2\nu gx} \right]/E \\ & \quad - \left( {2ax^{3} - 3bx^{2} - 6cx + 2\nu ex} \right)z_{1} /E \\ & \quad - \left[ {b\left( {2 + \nu } \right)z_{1}^{3} + 2\left( {1 + \nu } \right)fz_{1} } \right]/E + \omega z_{1} + u_{0} \\ \end{aligned}$$

(69)

$$\begin{aligned} w\left( {x,z_{1} } \right) & = - \left[ {a\left( {1 + 2\nu } \right)z_{1}^{4} /2 + 2\nu dz_{1} + 2gz_{1} } \right]/E \\ & \quad - \left[ {ax^{4} /2 - bx^{3} - 3cx^{2} - \left( {2 + \nu } \right)ex^{2} } \right]/E \\ & \quad + \left( {3a\nu x^{2} - 3b\nu x - 3\nu c + e} \right)z_{1}^{2} /E + \omega x + w_{0} , \\ \end{aligned}$$

(70)

where

(a, b, c, d, e, f, g) and ($u_{0}$, $w_{0,}$$\omega )$ are the sets of undetermined coefficients associated with displacements generated by body deformation and rigid body motions, respectively.

Using boundary conditions for stresses and stress resultants given in Lekhnitskii [29], and by setting constraint conditions $u\left( {L,0} \right) = w\left( {L,0} \right) = 0$ and $\partial u/\partial z_{{\left( {x = L,,z_{1} = 0} \right)}} = 0$, Ding et al. [28] show that the values of the coefficients in Eqs. (69) and (70) are $a = q_{0} /h^{3}$, b$= d = f = 0$, c$= - q_{0} /\left( {10h} \right)$, $e = 3q_{0} /\left( {4h} \right)$, q$= - q_{0} /4$, $u_{0} = - \nu q_{0} L/\left( {2E} \right)$, $w_{0} = q_{0} L^{2} \left( {30L^{2} - \left( {24 + 15\nu } \right)h^{2} } \right)/(240D_{x}$), and $\omega = q_{0} L\left[ {20L^{2} - 3\left( {5\nu + 8} \right)h^{2} } \right]/(120D_{x}$).

Consequently, Eq. (70) at centroid with $x = 0$ and assuming $\nu = 0$ gives

$$w\left( {0,0} \right) = q_{0} \left( {L - x} \right)\left[ {15L^{3} + \left( {12h^{2} - 5L^{2} } \right)x + 18Lh^{2} - 5Lx^{2} - 5x^{3} } \right]/\left( {120D_{x} } \right).$$

(71)

By back-substituting the constants $u_{0}$, $w_{0,}$ and $\omega$ into Eq. (69), axial displacement at the fixed end with $z_{1} = \pm h/2$ gives $u\left( {L, \pm h/2} \right) \ne 0$, implying on unwanted values in a physical sense.

If constraint conditions given in Ref. [29] are changed to $u\left( {L,0} \right) = w\left( {L,0} \right) = 0$ and $u\left( {L, \pm h/2} \right) = 0$, only the values of $w_{0}$ and $\omega$ in Eqs. (69) and (70) are modified, giving $w_{0} = q_{0} L^{2} \left[ {30L^{2} + \left( {16 + 35\nu } \right)h^{2} } \right]/(240D_{x}$), and $\omega = q_{0} L\left[ {20L^{2} + 2\left( {5\nu - 2} \right)h^{2} } \right]/(120D_{x}$). Consequently, Eq. (70) at centroid with $x = 0$ and assuming $\nu = 0$ gives

$$\begin{aligned} w\left( {0,0} \right) & = q_{0} \left( {L - x} \right)\{ 15L^{3} + \left( {12h^{2} - 5L^{2} } \right)x \\ & \quad + 8Lh^{2} - 5Lx^{2} - 5x^{3} \} /\left( {120D_{x} } \right). \\ \end{aligned}$$

(72)