Pythagorean fuzzy linguistic decision support model based on consistency-adjustment strategy and consensus reaching process

Abstract

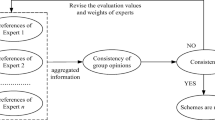
This study designs a novel decision support model to address group decision-making (GDM) problems with Pythagorean fuzzy linguistic information. To do so, a new concept of Pythagorean fuzzy linguistic preference relations (PFLPRs) is first introduced to describe fuzzy and uncertain information, where the Pythagorean fuzzy linguistic values (PFLVs) are represented by the linguistic membership degree and linguistic non-membership degree. Then, we also give the definitions of multiplicative consistency of PFLPRs, consistency index (CI), individual consensus degree (IGD) and group consensus degree (GCD). Subsequently, a consistency-adjustment approach is proposed to convert unacceptable multiplicative consistent PFLPRs into acceptable ones, as well as, derive the optimal normalized Pythagorean fuzzy priority weight vector (PFPWV) for alternatives. Furthermore, we design two algorithms in group decision support model. The first algorithm is used to check the multiplicative consistency of original PFLPRs and transform the unacceptable multiplicative consistent PFLPRs into the acceptable ones. The second algorithm is designed to aid the GCD to achieve the predefined level. The most innovative features of the proposed decision support model are following two points. One is that the GCD reaches the predefined level, while each PFLPR still keeps multiplicative consistency. The other is that it can preserve decision makers’ original preference information as much as possible. Finally, we give a numerical example to illustrate validity and practicality of this proposed approach.

Appendix

Proof of Theorem 1

At first, we demonstrate the necessary part. If PFLPR $A = \left( {a_{ij} } \right)_{n \times n}$ is multiplicative consistent, based on Definition 4, we can get

$$ I\left( {a_{ij\mu } } \right)I\left( {a_{jk\mu } } \right)I\left( {a_{ki\mu } } \right) = I\left( {a_{ik\mu } } \right)I\left( {a_{kj\mu } } \right)I\left( {a_{ji\mu } } \right). $$

(30)

As $a_{ij\mu } = a_{ji\upsilon } ,\;a_{ij\upsilon } = a_{ji\mu },$ we can get $I\left( {a_{ij\mu } } \right)I\left( {a_{kj\upsilon } } \right)I\left( {a_{ik\upsilon } } \right) = I\left( {a_{ik\mu } } \right)I\left( {a_{kj\mu } } \right)I\left( {a_{ij\upsilon } } \right),\;i,j,k \in N,$ then

$$ \frac{{I\left( {a_{ij\mu } } \right)}}{{I\left( {a_{ij\upsilon } } \right)}} = \frac{{I\left( {a_{ik\mu } } \right)}}{{I\left( {a_{ik\upsilon } } \right)}} \cdot \frac{{I\left( {a_{kj\mu } } \right)}}{{I\left( {a_{kj\upsilon } } \right)}},\quad \forall i,j,k \in N. $$

(31)

Thus, it can deduce the following result on the basis of Eq. (31), $\Phi \left( {a_{ij} } \right) = \Phi \left( {a_{ik} } \right) \cdot \Phi \left( {a_{kj} } \right),\quad i,j,k \in N$. Then, we prove the sufficient section.

As $\Phi \left( {a{}_{ij}} \right) = \Phi \left( {a_{ik} } \right) \cdot \Phi \left( {a_{kj} } \right),\;i,j,k \in N$ and $\Phi \left( {a_{ij} } \right) = \frac{{I\left( {a_{ij\mu } } \right)}}{{I\left( {a_{ij\upsilon } } \right)}}$, we can get

$$ \frac{{I\left( {a_{ij\mu } } \right)}}{{I\left( {a_{ij\upsilon } } \right)}} = \frac{{I\left( {a_{ik\mu } } \right)}}{{I\left( {a_{ik\upsilon } } \right)}} \cdot \frac{{I\left( {a_{kj\mu } } \right)}}{{I\left( {a_{kj\upsilon } } \right)}}. $$

(32)

Next, we obtain the new formula $I\left( {a_{ij\mu } } \right)I\left( {a_{ik\upsilon } } \right)I\left( {a_{kj\upsilon } } \right) = I\left( {a_{ij\upsilon } } \right)I\left( {a_{ik\mu } } \right)I\left( {a_{kj\mu } } \right),\;i,j,k \in N.$ As $a_{ij\mu } = a_{ji\upsilon } ,\;a_{ij\upsilon } = a_{ji\mu } ,\;i,j \in N,$ we have $I\left( {a_{ij\mu } } \right)I\left( {a_{jk\mu } } \right)I\left( {a_{ki\mu } } \right) = I\left( {a_{ik\mu } } \right)I\left( {a_{kj\mu } } \right)I\left( {a_{ji\mu } } \right),\;i,j,k \in N,$ which proves that PFLPR $A = \left( {a_{ij} } \right)_{n \times n}$ is multiplicative consistent.

Proof of Theorem 2

As PFLPR $A = \left( {a_{ij} } \right)_{n \times n}$ is multiplicative consistent, then we get $\Phi \left( {a_{ij} } \right) = \Phi \left( {a_{ik} } \right) \cdot \Phi \left( {a_{kj} } \right),\;i,j,k \in N$. If $\Phi \left( {a_{ik} } \right) \ge 1$ and $\Phi \left( {a_{kj} } \right) \ge 1$, then $\Phi \left( {a_{ij} } \right) = \Phi \left( {a_{ik} } \right) \cdot \Phi \left( {a_{kj} } \right) \ge 1$, for any $i,j,k \in N$. Based on Definition 5, the proof of Theorem 2 is accomplished.

Proof of Theorem 3

The optimal deviation values are $\tilde{s}_{ij}^{ + } ,\tilde{s}_{ij}^{ - } ,\tilde{r}_{ij}^{ + } ,\tilde{r}_{ij}^{ - } ,i,j \in N$ and $\tilde{\omega } = \left( {\tilde{\omega }_{1} ,\tilde{\omega }_{2} , \ldots ,\tilde{\omega }_{n} } \right)^{T}$ is the optimal normalized PFPWV in Model 2, then for any $i,j,k \in N$, we get

$$ \tilde{s}_{ij}^{ - } - \tilde{s}_{ij}^{ + } = 0.5\left( {\ln \omega_{i\mu } + \ln \omega_{j\upsilon } } \right) - \ln I\left( {a_{ij\mu } } \right),\quad \tilde{r}_{ij}^{ - } - \tilde{r}_{ij}^{ + } = 0.5\left( {\ln \omega_{i\upsilon } + \ln \omega_{j\mu } } \right) - \ln I\left( {a_{ij\upsilon } } \right), $$

(33)

$$ \ln I\left( {a_{ij\mu } } \right) + \tilde{s}_{ij}^{ - } - \tilde{s}_{ij}^{ + } = 0.5\left( {\ln \omega_{i\mu } + \ln \omega_{j\upsilon } } \right),\quad \ln I\left( {a_{ij\upsilon } } \right) + \tilde{r}_{ij}^{ - } - \tilde{r}_{ij}^{ + } = 0.5\left( {\ln \omega_{i\upsilon } + \ln \omega_{j\mu } } \right). $$

(34)

Based on Eqs. (33) and (34),we can get

$$ I\left( {a_{ij\mu } } \right) \cdot \exp \left( {\tilde{s}_{ij}^{ - } - \tilde{s}_{ij}^{ + } } \right) = \sqrt {\tilde{\omega }_{i\mu } \tilde{\omega }_{j\upsilon } } ,\quad I\left( {a_{ij\upsilon } } \right) \cdot \exp \left( {\tilde{r}_{ij}^{ - } - \tilde{r}_{ij}^{ + } } \right) = \sqrt {\tilde{\omega }_{i\upsilon } \tilde{\omega }_{j\mu } } ,\quad i \ne j, $$

(35)

$$ I^{ - 1} \left( {I\left( {a_{ij\mu } } \right) \cdot \exp \left( {\tilde{s}_{ij}^{ - } - \tilde{s}_{ij}^{ + } } \right)} \right) = I^{ - 1} \left( {\sqrt {\tilde{\omega }_{i\mu } \tilde{\omega }_{j\upsilon } } } \right),I^{ - 1} \left( {I\left( {a_{ij\upsilon } } \right) \cdot \exp \left( {\tilde{r}_{ij}^{ - } - \tilde{r}_{ij}^{ + } } \right)} \right) = I^{ - 1} \left( {\sqrt {\tilde{\omega }_{i\upsilon } \tilde{\omega }_{j\mu } } } \right),\;i \ne j, $$

(36)

therefore, according to Eq. (17), we can obtain that $\tilde{a}_{ij\mu } = I^{ - 1} \left( {\sqrt {\tilde{\omega }_{i\mu } \tilde{\omega }_{j\upsilon } } } \right)$ and $\tilde{a}_{ij\upsilon } = \sqrt {\omega_{i\upsilon } \omega_{j\mu } } ,\quad i \ne j.$

According to Corollary 1, it can certified that the PFLPR $A = \left( {a_{ij} } \right)_{n \times n} = \left( {\left\langle {a_{ij\mu } ,a_{ij\upsilon } } \right\rangle } \right)_{n \times n}$ is multiplicative consistent. The proof of Theorem 3 is accomplished.

Proof of Theorem 5

It is obvious that (i) and (iii) are valid and correct. Next, we can present the proof of (ii) and (iv).

As $a_{ij\mu } = a_{ji\upsilon }$, $a_{ij\upsilon } = a_{ji\mu }$, $b_{ij\mu } = b_{ij\upsilon }$, $b_{ij\upsilon } = b_{ji\mu }$, $i,j \in N$, then

$$ \begin{aligned} d\left( {A,B} \right) = 0 & \Leftrightarrow \left| {\ln I\left( {a_{{ij\mu }} } \right) - \ln I\left( {b_{{ij\mu }} } \right)} \right| + \left| {\ln I\left( {a_{{ij\upsilon }} } \right) - \ln I\left( {b_{{ij\upsilon }} } \right)} \right| = 0,\quad i < j \\ & \Leftrightarrow I\left( {a_{{ij\mu }} } \right) = I\left( {b_{{ij\mu }} } \right),\;I\left( {a_{{ij\upsilon }} } \right) = I\left( {b_{{ij\upsilon }} } \right),\quad i < j \\ & \Leftrightarrow I\left( {a_{{ij\mu }} } \right) = I\left( {b_{{ij\mu }} } \right),\;I\left( {a_{{ij\upsilon }} } \right) = I\left( {b_{{ij\upsilon }} } \right),\quad i,j \in N. \\ & \Leftrightarrow A = B \\ \end{aligned} $$

(37)

Based on Eq. (18), we can get

$$ \begin{aligned} d\left( {A,B} \right) = & \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{i < j} {\left( {\left| {\ln I\left( {a_{ij\mu } } \right) - \ln I\left( {b_{ij\mu } } \right)} \right| + \left| {\ln I\left( {a_{ij\upsilon } } \right) - \ln I\left( {b_{ij\upsilon } } \right)} \right|} \right)} \\ & = \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{i < j} {\left( {\left| {\left( {\ln I\left( {a_{ij\mu } } \right) - \ln I\left( {c_{ij\mu } } \right)} \right) + \left( {\ln I\left( {c_{ij\mu } } \right) - \ln I\left( {b_{ij\mu } } \right)} \right)} \right|} \right.} \\ & + \left| {\left( {\ln I\left( {a_{ij\upsilon } } \right) - \ln I\left( {c_{ij\upsilon } } \right)} \right) + \left( {\ln I\left( {c_{ij\upsilon } } \right) - \ln I\left( {b_{ij\upsilon } } \right)} \right)} \right|) \\ & \le \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{i < j} {\left( {\left| {\ln I\left( {a_{ij\mu } } \right) - \ln I\left( {c_{ij\mu } } \right)} \right|} \right.} + \left| {\ln I\left( {c_{ij\mu } } \right) - \ln I\left( {b_{ij\mu } } \right)} \right|, \\ & + \left| {\ln I\left( {a_{ij\upsilon } } \right) - \ln I\left( {c{}_{ij\upsilon }} \right)} \right| + \left| {\ln I\left( {c_{ij\upsilon } } \right) - \ln I\left( {b_{ij\upsilon } } \right)} \right|) \\ & = \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{i < j} {\left( {\left| {\ln I\left( {a_{ij\mu } } \right) - \ln I\left( {c_{ij\mu } } \right)} \right| + \left| {\ln I\left( {c_{ij\mu } } \right) - \ln I\left( {b_{ij\mu } } \right)} \right|} \right)} \\ & + \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{i < j} {\left( {\left| {\ln I\left( {a_{ij\upsilon } } \right) - \ln I\left( {c_{ij\upsilon } } \right)} \right| + \left| {\ln I\left( {c_{ij\upsilon } } \right) - \ln I\left( {b_{ij\upsilon } } \right)} \right|} \right)} \\ & = d\left( {A,C} \right) + d\left( {C,B} \right) \\ \end{aligned} $$

(38)

then the proof of Theorem 5 is accomplished.

Proof of Theorem 6

As $A_{k} = \left( {a_{ij,k} } \right)_{n \times n} \left( {k \in M} \right)$ be a collection of PFLPRs, based on Definition 1, we can get $a_{ij\mu ,k} = a_{ji\upsilon ,k}$,$a_{ij\upsilon ,k} = a_{ji\mu ,k}$,$I^{2} \left( {a_{ij\mu ,k} } \right) + I^{2} \left( {a_{ij\upsilon ,k} } \right) \le 1\;,\quad \forall i,j \in N,k \in M$, then $a_{ij\mu ,c} = I^{ - 1} \left( {\prod\nolimits_{k = 1}^{m} {\left( {I\left( {a_{ij\mu ,k} } \right)} \right)^{{\lambda_{k} }} } } \right) = I^{ - 1} \left( {\prod\nolimits_{k = 1}^{m} {\left( {I\left( {a_{ji\upsilon ,k} } \right)} \right)^{{\lambda_{k} }} } } \right) = a_{ji\upsilon ,c}$. According to Eq. (25), we can get $I\left( {a_{ij\mu ,c} } \right) = \prod\nolimits_{k = 1}^{m} {\left( {I\left( {a_{ij\mu ,k} } \right)} \right)}^{{\lambda_{k} }},$ $I\left( {a_{ij\upsilon ,c} } \right) = \prod\nolimits_{k = 1}^{m} {\left( {I\left( {a_{ij\upsilon ,k} } \right)} \right)}^{{\lambda_{k} }} ,\quad i,j \in N.$ Since $I\left( {a_{ij\mu ,k} } \right),I\left( {a_{ij\upsilon ,k} } \right) \in [0,1],$ $i,j \in N,\;k \in M,$ by Lemma 1, we get

$0 \le I\left( {a_{ij\mu ,c} } \right) = \prod\nolimits_{k = 1}^{m} {\left( {I\left( {a_{ij\mu ,k} } \right)} \right)}^{{\lambda_{k} }} \le \sum\nolimits_{k = 1}^{m} {\lambda_{k} I\left( {a_{ij\mu ,k} } \right)} \le 1 \cdot \sum\nolimits_{k = 1}^{m} {\lambda_{k} } = 1,$

$0 \le I\left( {a_{ij\upsilon ,c} } \right) = \prod\nolimits_{k = 1}^{m} {\left( {I\left( {a_{ij\upsilon ,k} } \right)} \right)}^{{\lambda_{k} }} \le \sum\nolimits_{k = 1}^{m} {\lambda_{k} I\left( {a_{ij\upsilon ,k} } \right)} \le 1 \cdot \sum\nolimits_{k = 1}^{m} {\lambda_{k} } = 1,$ it can obtain that $s_{0} \le a_{ij\mu ,c} \le s_{2\tau }$ and $s_{0} \le a_{ij\upsilon ,c} \le s_{2\tau } ,\quad i,j \in N.$ Furthermore, as $I^{2} \left( {a_{ij\mu ,k} } \right) + I^{2} \left( {a_{ij\upsilon ,k} } \right) \in [0,1],i,j \in N,k \in M$, according to Lemma 1, we have

$$ \begin{aligned} I^{2} \left( {a_{ij\mu ,c} } \right) + I^{2} \left( {a_{ij\upsilon ,c} } \right) = & \left( {\prod\limits_{k = 1}^{m} {\left( {I\left( {a_{ij\mu ,k} } \right)} \right)^{{\lambda_{k} }} } } \right)^{2} + \left( {\prod\limits_{k = 1}^{m} {\left( {I\left( {a_{ij\upsilon ,k} } \right)} \right)^{{\lambda_{k} }} } } \right)^{2} \\ & \le \left( {\sum\limits_{k = 1}^{m} {\lambda_{k} } I\left( {a_{ij\mu ,k} } \right)} \right)^{2} + \left( {\sum\limits_{k = 1}^{m} {\lambda_{k} } I\left( {a_{ij\upsilon ,k} } \right)} \right)^{2} \\ & = \left( {\sum\limits_{k = 1}^{m} {\lambda_{k} } } \right)^{2} I^{2} \left( {a_{ij\mu ,k} } \right) + \left( {\sum\limits_{k = 1}^{m} {\lambda_{k} } } \right)^{2} I^{2} \left( {a_{ij\upsilon ,k} } \right), \\ & = \left( {\sum\limits_{k = 1}^{m} {\lambda_{k} } } \right)^{2} \left( {I^{2} \left( {a_{ij\mu ,k} } \right) + I^{2} \left( {a_{ij\upsilon ,k} } \right)} \right) \\ & = \left( {\sum\limits_{k = 1}^{m} {\lambda_{k} } } \right)^{2} \cdot 1 = 1 \\ \end{aligned} $$

(39)

then $I^{2} \left( {a_{ij\mu ,c} } \right) + I^{2} \left( {a_{ij\upsilon ,c} } \right) \le 1,\quad i,j \in N$.

Based on Definition 2, it is proved that $A_{c} = \left( {a_{ij,c} } \right)_{n \times n}$ is a PFLPR. The proof of Theorem 6 is accomplished.

Proof of Theorem 7

Suppose that $\tilde{s}_{ij,k}^{ - } ,\tilde{s}_{ij,k}^{ + } ,\tilde{r}_{ij,k}^{ - } ,\tilde{r}_{ij,k}^{ + } ,i,j \in N,k \in M$ be the most desirable deviation values, as well as, $\tilde{s}_{ij,c}^{ + } ,\tilde{s}_{ij,c}^{ - } ,\tilde{r}_{ij,c}^{ + } ,\tilde{r}_{ij,c}^{ - } ,i,j \in N,k \in M$ be the optimal deviation values, and then we have

$$ \tilde{s}_{ij,c}^{ - } = \sum\limits_{k = 1}^{m} {\lambda_{k} } \tilde{s}_{ij,k}^{ - } ,\tilde{s}_{ij,c}^{ + } = \sum\limits_{k = 1}^{m} {\lambda_{k} \tilde{s}_{ij,k}^{ + } } ,\tilde{r}_{ij,c}^{ - } = \sum\limits_{k = 1}^{m} {\lambda_{k} } \tilde{r}_{ij,k}^{ - } ,\tilde{r}_{ij,c}^{ + } = \sum\limits_{k = 1}^{m} {\lambda_{k} \tilde{r}_{ij,k}^{ + } } . $$

(40)

Let $\tilde{A}_{c} = \left( {\tilde{a}_{ij,c} } \right)_{n \times n} = \left( {\left\langle {\tilde{a}_{ij\mu ,c} ,\tilde{a}_{ij\upsilon ,c} } \right\rangle } \right)_{n \times n}$ and $\tilde{A}_{k} = \left( {\tilde{a}_{ij,k} } \right)_{n \times n} = \left( {\left\langle {\tilde{a}_{ij\mu ,k} ,\tilde{a}_{ij\upsilon ,k} } \right\rangle } \right)_{n \times n}$, for $\forall i,j \in N,k \in M$, we can get

$$ \tilde{a}_{ij\mu ,k} = I^{ - 1} \left( {I\left( {a_{ij\mu ,k} } \right) \cdot \exp \left( {\tilde{s}_{ij,k}^{ - } - \tilde{s}_{ij,k}^{ + } } \right)} \right),\tilde{a}_{ij\upsilon ,k} = I^{ - 1} \left( {I\left( {a_{ij\upsilon ,k} } \right) \cdot \exp \left( {\tilde{r}_{ij,k}^{ - } - \tilde{r}_{ij,k}^{ + } } \right)} \right), $$

(41)

$$ \tilde{a}_{ij\mu ,c} = I^{ - 1} \left( {I\left( {a_{ij\mu ,c} } \right) \cdot \exp \left( {\tilde{s}_{ij,c}^{ - } - \tilde{s}_{ij,c}^{ + } } \right)} \right),\tilde{a}_{ij\upsilon ,c} = I^{ - 1} \left( {I\left( {a_{ij\upsilon ,c} } \right) \cdot \exp \left( {\tilde{r}_{ij,c}^{ - } - \tilde{r}_{ij,c}^{ + } } \right)} \right). $$

(42)

Based on Theorem 3, it is notable that $\tilde{A}_{k} \left( {k \in M} \right)$ and $A_{c}$ are the multiplicative consistent PFLPRs.

According to Eqs. (41) and (42), we get $I\left( {\tilde{a}_{ij\mu ,k} } \right) = I\left( {a_{ij\mu ,k} } \right) \cdot \exp \left( {\tilde{s}_{ij,k}^{ - } - \tilde{s}{}_{ij,k}^{ + } } \right),$ $I\left( {\tilde{a}_{ij\upsilon ,k} } \right) = I\left( {a_{ij\upsilon ,k} } \right) \cdot \exp \left( {\tilde{r}_{ij,k}^{ - } - \tilde{r}_{ij,k}^{ + } } \right),\quad i,j \in N,k \in M,$ $I\left( {\tilde{a}_{ij\mu ,c} } \right) = I\left( {a_{ij\mu ,c} } \right) \cdot \exp \left( {\tilde{s}_{ij,c}^{ - } - \tilde{s}_{ij,c}^{ + } } \right),$ $I\left( {\tilde{a}_{ij\upsilon ,c} } \right) = I\left( {a_{ij\upsilon ,c} } \right) \cdot \exp \left( {\tilde{r}_{ij,c}^{ - } - \tilde{r}_{ij,c}^{ + } } \right),\quad i,j \in N,$ it can obtain that

$$ \ln I\left( {\tilde{a}_{ij\mu ,k} } \right) - \ln I\left( {a_{ij\mu ,k} } \right) = \tilde{s}_{ij,k}^{ - } - s_{ij,k}^{ + } ,\quad \ln I\left( {\tilde{a}_{ij\upsilon ,k} } \right) - \ln I\left( {a_{ij\upsilon ,k} } \right) = \tilde{r}_{ij,k}^{ - } - \tilde{r}_{ij,k}^{ + } ,\;i,j \in N,\;k \in M, $$

(43)

$$ \ln I\left( {\tilde{a}_{ij\mu ,c} } \right) - \ln I\left( {a_{ij\mu ,c} } \right) = \tilde{s}_{ij,c}^{ - } - \tilde{s}_{ij,c}^{ + } ,\ln I\left( {\tilde{a}_{ij\upsilon ,c} } \right) - \ln I\left( {a_{ij\upsilon ,c} } \right) = \tilde{r}_{ij,c}^{ - } - \tilde{r}_{ij,c}^{ + } ,i,j \in N. $$

(44)

Based on $CI\left( {A_{k} } \right) < \overline{CI} ,k \in M$, we can obtain the following formula by Eqs. (39), (42) and (43),

$$ \begin{aligned} {\text{CI}}\left( {A_{c} } \right) & = \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{{i\, < j}} {\left( {\left| {\ln I\left( {\tilde{a}_{{ij\mu ,c}} } \right) - \ln I\left( {a_{{ij\mu ,c}} } \right)} \right| + \left| {\ln I\left( {\tilde{a}_{{ij\upsilon ,c}} } \right) - \ln I\left( {a_{{ij\upsilon ,c}} } \right)} \right|} \right)} \\ & = \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{{i\, < j}} {\left( {\left| {\tilde{s}_{{ij,c}}^{ - } - \tilde{s}_{{ij,c}}^{ + } } \right| + \left| {\tilde{r}_{{ij,c}}^{ - } - \tilde{r}_{{ij,c}}^{ + } } \right|} \right)} \\ & = \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{{i\, < j}} {\left( {\left| {\sum\limits_{{k = 1}}^{m} {\lambda _{k} \tilde{s}_{{ij,k}}^{ - } - \sum\limits_{{k = 1}}^{m} {\lambda _{k} } \tilde{s}_{{ij,k}}^{ + } } } \right| + \left| {\sum\limits_{{k = 1}}^{m} {\lambda _{k} \tilde{r}_{{ij,k}}^{ - } - \sum\limits_{{k = 1}}^{m} {\lambda _{k} \tilde{r}_{{ij,k}}^{ + } } } } \right|} \right)} \\ & = \frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{{i\, < j}} {\left( {\left| {\sum\limits_{{k = 1}}^{m} {\lambda _{k} \left( {\tilde{s}_{{ij,k}}^{ - } - \tilde{s}_{{ij,k}}^{ + } } \right)} } \right| + \left| {\sum\limits_{{k = 1}}^{m} {\lambda _{k} \left( {\tilde{r}_{{ij,k}}^{ - } - \tilde{r}_{{ij,k}}^{ + } } \right)} } \right|} \right)} , \\ & \le \sum\limits_{{k = 1}}^{m} {\lambda _{k} } \left( {\frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{{i\, < j}} {\left( {\left| {\tilde{s}_{{ij,k}}^{ - } - \tilde{s}_{{ij,k}}^{ + } } \right| + \left| {\tilde{r}_{{ij,k}}^{ - } - \tilde{r}_{{ij,k}}^{ + } } \right|} \right)} } \right) \\ & = \sum\limits_{{k = 1}}^{m} {\lambda _{k} } \left( {\frac{1}{{n\left( {n - 1} \right)\ln 2\tau }}\sum\limits_{{i\, < j}} {\left( {\left| {\ln I\left( {\tilde{a}_{{ij\mu ,k}} } \right) - \ln I\left( {a_{{ij\mu ,k}} } \right)} \right| + \left| {\ln I\left( {\tilde{a}_{{ij\upsilon ,k}} } \right) - \ln I\left( {a_{{ij\upsilon ,k}} } \right)} \right|} \right)} } \right) \\ & = \sum\limits_{{k = 1}}^{m} {\lambda _{k} \cdot } CI\left( {A_{k} } \right) \le \sum\limits_{{k = 1}}^{m} {\lambda _{k} \cdot \mathop {\max }\limits_{{1 \le k \le m}} } \left\{ {CI\left( {A_{k} } \right)} \right\} = \mathop {\max }\limits_{{1 \le k \le m}} \left\{ {CI\left( {A_{K} } \right)} \right\} \\ \end{aligned} $$

then the proof of Theorem 7 is completed.