Solutions of Bagley–Torvik and Painlevé equations of fractional order using iterative reproducing kernel algorithm with error estimates

Abstract

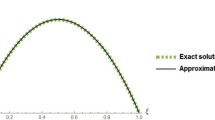
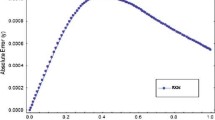
This paper presents iterative reproducing kernel algorithm for obtaining the numerical solutions of Bagley–Torvik and Painlevé equations of fractional order. The representation of the exact and the numerical solutions is given in the $\hat{W}_{2}^{3} \left[ {0,1} \right]$, $W_{2}^{3} \left[ {0,1} \right]$, and $W_{2}^{1} \left[ {0,1} \right]$ inner product spaces. The computation of the required grid points is relying on the $\hat{R}_{t}^{{\left\{ 3 \right\}}} \left( s \right)$, $R_{t}^{{\left\{ 3 \right\}}} \left( s \right)$, and $R_{t}^{{\left\{ 1 \right\}}} \left( s \right)$ reproducing kernel functions. An efficient construction is given to obtain the numerical solutions for the equations together with an existence proof of the exact solutions based upon the reproducing kernel theory. Numerical solutions of such fractional equations are acquired by interrupting the $n$-term of the exact solutions. In this approach, numerical examples were analyzed to illustrate the design procedure and confirm the performance of the proposed algorithm in the form of tabulate data, numerical comparisons, and graphical results. Finally, the utilized results show the significant improvement in the algorithm while saving the convergence accuracy and time.