Abstract
Consignment contracts are widely implemented in virtual market. We consider a supplier produces product and sells it to market through a retailer by consignment contract. In a unified model assumption, considering random demand and customers’ uncertain return behavior, we focus on two popular consignment contracts: vendor managed consignment inventory contract and consignment contract with revenue sharing. Comparing analytical results between the two contracts, we find that (1) the retailer and whole supply chain will benefit more from the consignment contract with revenue sharing, (2) the supplier will prefer to vendor managed consignment inventory contract, (3) both the retailer and supply chain will share a higher risk under the consignment contract with revenue sharing, the supplier’s shares of risk and profit highly depend on his share of the total expected cost, (4) all the profit and risk functions are decrease in uncertainty of customer return. We believe that these managerial insights can advance the understanding about use of the two consignment contracts in supply chain management with both benefit and risk considerations.







Similar content being viewed by others
Notes
Similar analysis can be conducted for the linear price-dependent demand function.
If \(b \le 1,\) the optimal price for the optimization problem to be considered late goes to infinity.
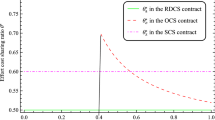
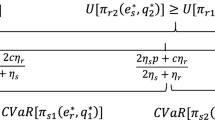
As the retailer almost represents the whole supply chain when \(\alpha \rightarrow 1\), the retailer should also bear all the risk of the supply chain.
References
Adida, E., & Ratisoontorn, N. (2011). Consignment contracts with retail competition. European Journal of Operational Research, 215(1), 136–148.
Ai, X., Chen, J., & Ma, J. (2012). Contracting with demand uncertainty under supply chain competition. Annals of Operations Research, 201, 17–38.
Bassok, Y., & Nagarajan, M. (2004). Contracting under risk preferences. Working paper, University of Southern California.
Chen, S. L., & Liu, C. L. (2008). The optimal consignment policy for the manufacturer under supply chain co-ordination. International Journal of Production Research, 46(18), 5121–5143.
Chen, Y. J., & Seshadri, S. (2006). Supply chain structure and demand risk. Automatica, 42, 1291–1299.
Chiu, C. H., & Choi, T. M. (2013). Supply chain risk analysis with mean-variance models: A technical review. Annals of Operations Research. doi:10.1007/s10479-013-1386-4
Choi, T. M. (2013). Multi-period risk minimization purchasing models for fashion products with interest rate, budget, and profit target considerations. Annals of Operations Research. doi:10.1007/s10479-013-1453-x
Choi, T. M. (2011). Coordination and risk analysis of VMI supply chains with RFID technology. IEEE Transactions on Industrial Informatics, 7, 497–504.
Choi, T. M., & Chow, P. S. (2008). Mean-variance analysis of quick response programme. International Journal of Production Economics, 114, 456–475.
DeCarlo, S., & Anderson, A. C. (2002). The Super 500: America’s Top Companies. Forbes, 15 April, p. 168.
Gan, X., Sethi, S., & Yan, H. (2005). Channel coordination with a risk neutral supplier and a downside risk-averse retailer. Production and Operations Management, 14, 80–89.
Lariviere, M. A., & Porteus, E. L. (2001). Selling to the newsvendor: An analysis of price-only contracts. Manufacturing and Service Operations Management, 3(4), 293–305.
Lau, H. S. (1980). The newsboy problem under alternative optimization objectives. Journal of the Operational Research Society, 31, 525–535.
Lau, H. S., & Lau, A. H. L. (1999). Manufacturer’s pricing strategy and returns policy for a single-period commodity. European Journal of Operational Research, 116, 291–304.
Li, S., & Hua, Z. (2008). A note on channel performance under consignment contract with revenue sharing. European Journal of Operational Research, 184, 793–796.
Markowitz, H. M. (1959). Portfolio selection: Efficient diversification of investment. New York: Wiley.
Mostard, J., & Teunter, R. (2006). The newsboy problem with resalable returns: A single model and case study. European Journal of Operational Researc h, 16, 81–96.
Ogryczak, W., & Ruszczynski, A. (2001). On consistency of stochastic dominance and mean-semideviation models. Mathematical Programming, Series B, 89, 217–232.
Petruzzi, N., & Dada, M. (1999). Pricing and the newsvendor problem: A review with extensions. Operations Research, 47, 184–194.
Ru, J., & Wang, Y. (2010). Consignment contracting: Who should control inventory in the supply chain? European Journal of Operational Research, 201, 760–769.
Wang, Y. (2006). Joint pricing-production decisions in supply chains of complementary products with uncertain demand. Operations Research, 54, 1110–1127.
Wang, Y., Jiang, L., & Shen, Z. (2004). Channel performance under consignment contract with revenue sharing. Management Science, 50(1), 34–47.
Wang, C. X., & Webster, S. (2007). Channel coordination for a supply chain with a risk-neutral manufacturer and a loss-averse retailer. Decision Sciences, 38, 361–389.
Webster, S., & Weng, Z. K. (2000). A risk-free perishable item returns policy. Manufacturing & Service Operations Management, 2(1), 100–106.
Zhang, D., Matta, R. D., & Lowe, T. J. (2010). Channel coordination in a consignment contract. European Journal of Operational Research, 207(2), 897–905.
Zhao, Y., Choi, T. M., Cheng, T. C. E., & Wang, S. (2014). Mean-risk analysis of wholesale price contracts with stochastic price-dependent demand. Annals of Operations Research. doi:10.1007/s10479-014-1689-0
Acknowledgments
The authors sincerely thank the editor and the anonymous reviewers for their constructive and important comments on the paper. We acknowledge the support of (i) National Natural Science Foundation of China, grant no. 71202162, for Wei Hu; (ii) the Fundamental Research Funds for the Central Universities of China No. NKZXZD1103, and The Major Program of the National Social Science Fund of China (Grant No. 13&ZD147), for YongjianLi; and (iii) National Natural Science Foundation of China (NSFC) No. 71372100, for Wen Wang.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
Proof of Proposition 1
For any given w and z, we take the partial derivative of (1) with respect to p as
Since \((1-k)ap^{-b-1}>0, {\partial E\Pi _R^{vc}(p|w,z)}/{\partial p}=0\) implies that (2). Moreover, for any given w and \(z, {\partial E\Pi _R^{vc}(p|w,z)}/{\partial p}>0\) for all \(p<p^{vc*}(w,z)\) and \({\partial E\Pi _R^{vc}(p|w,z)}/{\partial p}<0\) for all \(p>p^{vc*}(w,z),\) so \(p^{vc*}(w,z)\) is the unique maximizer of \(E\Pi _R^{vc}(p|w,z).\) \(\square \)
Proof of Proposition 2
Taking the first derivative of the supplier’s expected profit \(E\Pi _S^{vc}(w,z)\) with respect to w gets the optimal \(w^{vc*}(z)\) as expressed in (3). Then after substituting (3) back into \(E\Pi _S^{vc}(w,z)\), we have \(E\Pi _S^{vc}(w^{vc*}(z),z)=(1-k)\frac{acz}{b-1}\bigg \{\frac{b^2c}{(b-1)^2} \frac{z}{z-\Lambda (z)}\bigg \}^{-b},\) next we have to confirm that if \(d[xh(x)]/dx=h(x)+x dh(x)/dx>0,\) the optimal \(z^{vc*}\) is uniquely determined by (4).
where \(G(z)=z-bzF(z)+(b-1)\Lambda (z).\) The optimal \(z^{vc*}\) satisfy \(G(z)=0,\) and such a \(z^{vc*}\) always exists in the interval (A, B) of \(F(\cdot ).\) As G(z) is continuous in \((A,B), G(A)=A>0,\) and \(G(B)=-(b-1)\mu <0.\) Next, we go on to verify the uniqueness of \(z^{vc*}.\) We have \(G'(z)=[1-F(z)][1-bzh(z)]\) and \(G''(z)=-h(z)G'(z)-b[1-F(z)][h(z)+zh'(z)].\) So if \(d[xh(x)]/dx=h(x)+x dh(x)/dx>0, G''(z)<0\) at \(G'(z)=0,\) which indicates that G(z) is a unimodal in (A, B). Combining with \(G(A)>0\) and \(G(B)<0,\) then uniqueness of \(z^{vc*}\) is guaranteed. \(\square \)
Proof of Proposition 3
(i) We can easily get these results from (3), (4), (5), (10), (11) and (12).
(ii) we can rewrite \(E\Pi ^{rc*}\) in (15) as
where \(\frac{2b-b\alpha -1}{2b-1}\left( \frac{b}{b-\alpha }\right) ^b\) is increasing in \(\alpha ,\) and is equal to 1 when \(\alpha \) is equal to 0. So \(E\Pi ^{rc*} \ge E\Pi ^{vc*}.\)
Further, from (6) and (14), we get
where \([(b/(b-\alpha )]^{b-1}\) is increasing in \(\alpha .\) Especially when \(\alpha =0,\) the term \([(b/(b-\alpha )]^{b-1}\) turns out to be 1, and \(E\Pi _R^{rc*}=E\Pi _R^{vc*}\).
It is easy to verify that \((1-\alpha )[b/(b-\alpha )]^{b}\) is decreasing in \(\alpha ,\) which reaches its maximum at \(\alpha =0\).
(iii) to prove \({\beta }^{rc} \ge {\beta }^{vc},\) we have
(iv) Taking the first derivative with \(\alpha ,\) we have \(d {\beta }^{rc}/d \alpha =(b-1)^2/(2b-b \alpha -1)^2 \ge 0,\) and \(\lim _{\alpha \rightarrow 1}{\beta }^{rc}=(b-1)/(b-1)=1.\) \(\square \)
Proof of Proposition 4
(i) We can easily get this result from proposition 2, (11) and (12).
(ii) and (iii) As \(c=C/(1-k),\) it is easy to verify \(w^{vc*},\) \(p^{vc*}\) and \(p^{rc*}\) are increase in c, then they are increase in \(k. E\Pi _R^{vc*},\) \(E\Pi _S^{vc*}, E\Pi ^{vc*}, E\Pi _R^{rc*},\) \(E\Pi _S^{rc*},\) and \(E\Pi ^{rc*}\) are decrease in c, so they are decrease in k. \(\square \)
Proof of Lemma 1
Part (i): Taking the first derivative with \(\alpha \) of \(\lambda (\alpha ),\) we have
Let
we have \(\gamma '(\alpha )=-(b-1)/[b(1-\alpha )+\alpha ]^2-b(b-1)/(b-\alpha )^2<0,\) and \(\gamma (0)=1-1/b>0, \gamma (1)=-1<0.\) Then \(\gamma (\alpha )\) is decreasing from positive to negative in [0, 1]. So \(\lambda (\alpha )\) first increasing and then decreasing in [0, 1]. Thus \(\lambda (\alpha )\) is a unimodal function of \(\alpha .\)
Part (ii): The results can be easily derived by substituting \(\alpha =1\) and \(\alpha =0\) into (17).
Part (iii): From (i), we know that \(\lambda (\alpha )\) is first strictly increasing and then strictly decreasing in the interval [0, 1]. Due to the continuity of \(\lambda (\alpha )\) and \(\lambda (1)=0,\) \(\lambda (0)=1\) from part (ii), there exists a unique \(\hat{\alpha }\in (0,1),\) which satisfies \(\lambda (\hat{\alpha })=1.\)
Part (iv): When \(\alpha \in [0,\hat{\alpha }], \lambda (\alpha )\) is first increasing and then decreasing in \(\alpha \), which reaches its minimum 1 at the boundary 0 and \(\hat{\alpha }\); when \(\alpha \in (\hat{\alpha },1], \lambda (\alpha )\) is decreasing in \(\alpha \) and \(0 \le \lambda (\alpha ) <1.\) \(\square \)
Proof of Proposition 5
(i) From proposition 3 (i), we have \(z^{rc*}=z^{vc*},\)
Furthermore, py(p) is a decreasing function of p, and \(p^{vc*}\ge p^{rc*},\) then \(\delta ^{rc*}\ge \delta ^{vc*}\) is obvious.
Part (ii)
We can rearrange the above two expressions as:
When \(\alpha =0, \frac{b[\alpha (b-2)+1]}{\alpha (b-1)^2+b}\left( \frac{b}{b-\alpha }\right) ^b\) turns out to be 1, and taking the first derivative with \(\alpha ,\) we have
then \(\delta _R^{rc*} \ge \delta _R^{vc*}\) is obvious.
Part (iii): Similarly, we have
We can rewrite the above two expressions as:
From lemma 1 (iv), we can easily derive when \(\alpha \in [0,\hat{\alpha }], \delta _S^{rc*}\ge \delta _S^{vc*};\) when \(\alpha \in (\hat{\alpha },1], \delta _S^{rc*}< \delta _S^{vc*}\). \(\square \)
Proof of Proposition 6
(i)
and
To prove \({\beta }^{rc'}\ge {\beta }^{vc'},\) we have
(ii) We have
and
(iii) Taking the first derivative with \(\alpha \) for given b, we have \(d {\beta }^{rc'}/d \alpha =(b-1)^2/(b-\alpha )^2 \ge 0,\) and we get \(\lim _{\alpha \rightarrow 1}{\beta }^{rc'}=(b-1)/(b-1)=1.\) \(\square \)
Proof of Proposition 7
From (18), we know \((1-k)p^{rc*}\) and \(z^{rc*}\) are irrelevant with k, while y(p) is decrease in p and \(p^{rc*}\) is increase in k, then \(\delta ^{rc*}\) is decrease in k. The same procedure can be applied to \(\delta ^{vc*}.\)
From (20) and (22), we know \(r^{rc*}, (1-k)p^{rc*}\) and \(z^{rc*}\) are irrelevant with k, with y(p) is decrease in p and \(p^{rc*}\) is increase in k, then \(\delta _R^{rc*}\) and \(\delta _S^{rc*}\) are decrease in k.
From (21) and (23), we know \((1-k) [p^{vc*}-w^{vc*}], (1-k)w^{vc*}\) and \(z^{vc*}\) are irrelevant with k, combining with y(p) is decrease in p and \(p^{vc*}\) is increase in k, then \(\delta _R^{vc*}\) and \(\delta _S^{vc*}\) are decrease in k. \(\square \)
Rights and permissions
About this article
Cite this article
Hu, W., Li, Y. & Wang, W. Benefit and risk analysis of consignment contracts. Ann Oper Res 257, 641–659 (2017). https://doi.org/10.1007/s10479-015-1919-0
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-015-1919-0