Skip to main content
SpringerLink
Log in

On a Kohn-Vogelius like formulation of free boundary problems

  • Published:
Computational Optimization and Applications Aims and scope Submit manuscript

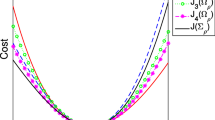
Abstract

The present paper is concerned with the solution of a Bernoulli type free boundary problem by means of shape optimization. Two state functions are introduced, namely one which satisfies the mixed boundary value problem, whereas the second one satisfies the pure Dirichlet problem. The shape problem under consideration is the minimization of the L 2-distance of the gradients of the state functions. We compute the corresponding shape gradient and Hessian. By the investigation of sufficient second order conditions we prove algebraic ill-posedness of the present formulation. Our theoretical findings are supported by numerical experiments.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. Acker, A.: On the geometric form of Bernoulli configurations. Math. Methods Appl. Sci. 10, 1–14 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  2. Afraites, L., Dambrine, M., Eppler, K., Kateb, D.: Detecting perfectly insulated obstacles by shape optimization techniques of order two. Discrete Contin. Dyn. Syst., Sér. B 8(2), 389–416 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  3. Afraites, L., Dambrine, M., Kateb, D.: On second order shape optimization methods for electrical impedance tomography. SIAM J. Control Optim. 47(3), 1556–1590 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  4. Alt, H.W., Caffarelli, L.A.: Existence and regularity for a minimum problem with free boundary. J. Reine Angew. Math. 325, 105–144 (1981)

    MathSciNet  MATH  Google Scholar 

  5. Dambrine, M.: On variations of the shape Hessian and sufficient conditions for the stability of critical shapes. Rev. R. Acad. Cienc. Exactas Fis. Nat. Ser. A. Mat. 96(1), 95–121 (2002)

    MathSciNet  MATH  Google Scholar 

  6. Dambrine, M., Pierre, M.: About stability of equilibrium shapes. Model Math. Anal. Numer. 34(4), 811–834 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  7. Delfour, M., Zolesio, J.-P.: Shapes and Geometries. SIAM, Philadelphia (2001)

    MATH  Google Scholar 

  8. Dennis, J.E., Schnabel, R.B.: Numerical Methods for Nonlinear Equations and Unconstrained Optimization Techniques. Prentice-Hall, Englewood Cliffs (1983)

    Google Scholar 

  9. Eppler, K.: Boundary integral representations of second derivatives in shape optimization. Discuss. Math. Differ. Incl. Control Optim. 20, 63–78 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  10. Eppler, K.: Optimal shape design for elliptic equations via BIE-methods. J. Appl. Math. Comput. Sci. 10, 487–516 (2000)

    MathSciNet  MATH  Google Scholar 

  11. Eppler, K.: On the local stability of the n-sphere for the n-dimensional Dido problem. Preprint SPP 1253-069, DFG Priority Program 1253, Universität Erlangen-Nürnberg (2009, submitted)

  12. Eppler, K., Harbrecht, H.: A regularized Newton method in electrical impedance tomography using shape Hessian information. Control Cybern. 34, 203–225 (2005)

    MathSciNet  MATH  Google Scholar 

  13. Eppler, K., Harbrecht, H.: Efficient treatment of stationary free boundary problems. Appl. Numer. Math. 56, 1326–1339 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  14. Eppler, K., Harbrecht, H.: Tracking Neumann data for stationary free boundary problems. SIAM J. Control Optim. 48, 2901–2916 (2009)

    Article  MathSciNet  Google Scholar 

  15. Eppler, K., Harbrecht, H.: Tracking Dirichlet data in L 2 is an ill-posed problem. J. Optim. Theory Appl. 145, 17–35 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  16. Eppler, K., Harbrecht, H., Schneider, R.: On convergence in elliptic shape optimization. SIAM J. Control Optim. 45, 61–83 (2007)

    Article  MathSciNet  Google Scholar 

  17. Flucher, M., Rumpf, M.: Bernoulli’s free-boundary problem, qualitative theory and numerical approximation. J. Reine Angew. Math. 486, 165–204 (1997)

    MathSciNet  MATH  Google Scholar 

  18. Grossmann, Ch., Terno, J.: Numerik der Optimierung. Teubner, Stuttgart (1993)

    MATH  Google Scholar 

  19. Harbrecht, H.: Analytical and numerical methods in shape optimization. Math. Methods Appl. Sci. 31(18), 2095–2114 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  20. Haslinger, J., Kozubek, T., Kunisch, K., Peichl, G.: Shape optimization and fictitious domain approach for solving free boundary problems of Bernoulli type. Comput. Optim. Appl. 26, 231–251 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  21. Haslinger, J., Kozubek, T., Kunisch, K., Peichl, G.: An embedding domain approach for a class of 2-d shape optimization problems: mathematical analysis. J. Math. Anal. Appl. 290, 665–685 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  22. Haslinger, J., Ito, K., Kozubek, T., Kunisch, K., Peichl, G.: On the shape derivative for problems of Bernoulli type. Interfaces Free Bound. 11, 317–330 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  23. Hörmander, L.: The Analysis of Linear Partial Differential Operators. Springer, New York (1983–1985)

    Book  Google Scholar 

  24. Ito, K., Kunisch, K., Peichl, G.: Variational approach to shape derivatives for a class of Bernoulli problems. J. Math. Anal. Appl. 314, 126–149 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  25. Kohn, R., Vogelius, M.: Determining conductivity by boundary measurements. Commun. Pure Appl. Math. 37, 289–298 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  26. Murat, F., Simon, J.: Étude de problèmes d’optimal design. In: Céa, J. (ed.) Optimization Techniques, Modeling and Optimization in the Service of Man. Lect. Notes Comput. Sci., vol. 41, pp. 54–62. Springer, Berlin (1976)

    Chapter  Google Scholar 

  27. Pironneau, O.: Optimal Shape Design for Elliptic Systems. Springer, New York (1983)

    Google Scholar 

  28. Simon, J.: Differentiation with respect to the domain in boundary value problems. Numer. Funct. Anal. Optim. 2, 649–687 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  29. Sokolowski, J., Zolesio, J.-P.: Introduction to Shape Optimization. Springer, Berlin (1992)

    Book  MATH  Google Scholar 

  30. Steinbach, O.: Numerical Approximation Methods for Elliptic Boundary Value Problems. Finite and Boundary Elements. Springer, New York (2008)

    Book  MATH  Google Scholar 

  31. Tepper, D.E.: On a free boundary value problem, the starlike case. SIAM J. Math. Anal. 6, 503–505 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  32. Tiihonen, T.: Shape optimization and trial methods for free-boundary problems. RAIRO Model. Math. Anal. Numér. 31(7), 805–825 (1997)

    MathSciNet  MATH  Google Scholar 

  33. Zolesio, J.-P.: Numerical algorithms and existence result for a Bernoulli-like steady free boundary problem. Large Scale Syst. 6, 263–278 (1984)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut für Numerische Mathematik, Technische Universität Dresden, Zellescher Weg 12–14, 01062, Dresden, Germany

    Karsten Eppler

  2. Institut für Angewandte Analysis und Numerische Simulation, Universität Stuttgart, Pfaffenwaldring 57, 70569, Stuttgart, Germany

    Helmut Harbrecht

Authors
  1. Karsten Eppler
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Helmut Harbrecht
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Helmut Harbrecht.

Additional information

This work was supported by the DFG Priority Program “Optimierung mit partiellen Differentialgleichungen” (PDE constrained optimization).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Eppler, K., Harbrecht, H. On a Kohn-Vogelius like formulation of free boundary problems. Comput Optim Appl 52, 69–85 (2012). https://doi.org/10.1007/s10589-010-9345-3

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10589-010-9345-3

Keywords

Search

Navigation