A choice of selling format in the online marketplace with cross-sales supply chain: Platform selling or traditional reselling?

Abstract

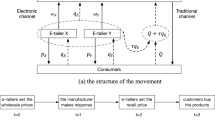
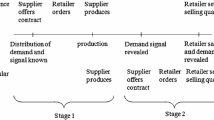
In the online retail market, how to work with upstream suppliers is a key issue for downstream online retailers (e-retailers). Online retailers can choose between functioning either as the “two-sided platforms” (e.g., Taobao.com or eBay.com) allowing suppliers to sell directly to customers by paying a revenue-sharing fee, or as the “resellers” (e.g., Wal-mart.com or JingDong.com) that purchase products from suppliers, and then resell them to customers. Given the rapid growth of e-commerce over past few years, this choice, which is the focus of this article, has become an important practice-based decision. We develop a game-theoretic model for a cross-sales supply chain in which two suppliers deal with two common online retailers. As Stackelberg leaders, online retailers can operate either as a two-sided platform (serving both suppliers and customers) or as a reseller (ordering from suppliers and selling competing products on its own platform). Each supplier adopts either an exclusive-sales strategy, selling products through an exclusive e-retailer, or a cross-sales strategy, selling products through two e-retailers. We analyze the optimal decisions for both e-retailers and suppliers in competing supply chains and describe the system equilibrium for the online marketplace.

Appendix

1.1 Proof of demand function for Eq. (2)

First, use the first order conditions (FOCs) of the the utility function of the consumer Eq. (1):

$${{\partial U} \mathord{\left/ {\vphantom {{\partial U} {\partial q_{ij} }}} \right. \kern-0pt} {\partial q_{ij} }} = a_{ij} - q_{ij} - b(q_{3 - i,j} + q_{i,3 - j} + q_{3 - i,3 - j} ) - p_{ij} = 0$$

we can find the optimal values of $q_{ij}$:

$$q_{11} = a_{11} - bq_{12} - bq_{21} - bq_{22} - p_{11} ,$$

(11)

$$q_{12} = a_{12} - bq_{11} - bq_{21} - bq_{22} - p_{12} ,$$

(12)

$$q_{21} = a_{21} - bq_{12} - bq_{11} - bq_{22} - p_{21} ,\,{\text{and}}$$

(13)

$$q_{22} = a_{22} - bq_{12} - bq_{21} - bq_{11} - p_{22} .$$

(14)

Then, solving Eqs. (11)–(14), we can get:

$$q_{ij} = (a - ab - p_{ij} - 2bp_{ij} + bp_{i,3 - j} + bp_{3 - i,j} + bp_{3 - i,3 - j} )/( - 3b^{2} + 2b + 1).$$

(15)

Simplifying (15), we can get:

$$q_{ij} = A_{ij} - \beta p_{ij} + \theta \sum\limits_{mn \ne ij} {p_{mn} } ,\,{\text{and}}\,A_{ij} = a/(1 + 3b),\beta = (1 + 2b)/[(1 - b)(1 + 3b)],\theta = b/[(1 - b)(1 + 3b)].$$

Proof of Lemma 1–3

First, we insert the (inverse) demand Eq. (2) into the retailers’ profit functions (4). That gives us the following updated retailers’ profit functions:

$$R_{j}^{EDD} = (p_{ij} - w_{ij}^{{}} )(a_{ij} - ba_{3 - i,3 - j} - p_{ij} + bp_{3 - i,3 - j} )/(1 - b^{2} ),\quad i,j = \{ 1,2\}$$

(16)

Then use the first order conditions (FOCs) of the profit functions (16) to find the optimal values of $p_{ij}$, and it can easily be proven that the FOCs guarantee optimality.

$$p_{ij}^{{}} = w_{ij} /2 - b/2 + bp_{3 - i,3 - j} /2 + 1/2\quad i,j = \{ 1,2\}$$

(17)

Second, inserting Eq. (17) into the suppliers’ profit functions (3) and using the first order conditions (FOCs), we can find the optimal values of $w_{ij}$:

$$w_{11}^{EDD} { = }w_{22}^{EDD} { = }2(1 - b)/(4 - 3b)$$

(5)

It can easily be proven that the FOCs guarantee optimality.

Third, inserting Eq. (5) into Eq. (17), we can derive the optimal values of $p_{ij}$:

$$p_{11}^{EDD} = p_{22}^{EDD} = 3(1 - b)/(4 - 3b)$$

(6)

The Proof of Lemma 1 is completed.□

The Proof of Lemmas 2 and 3 is similar to the Proof of Lemma 3.

Proof of Proposition 1

$$\begin{aligned} \frac{{p_{11}^{EDD} }}{{p_{11}^{EDP} }} & = \frac{3(1 - b)/(4 - 3b)}{{3(1 - b)(2 + b)/(8 - 3b^{2} )}} = \frac{{(8 - 3b^{2} )}}{(2 + b)(4 - 3b)} = \frac{{8 - 3b^{2} }}{{8 - 3b^{2} - 2b}} > 1, \\ \frac{{p_{11}^{EDP} }}{{p_{11}^{EPD} }} & = \frac{{3(1 - b)(2 + b)/(8 - 3b^{2} )}}{{(1 - b)(4 + 3b)/(8 - 3b^{2} )}} = \frac{3(2 + b)}{(4 + 3b)} > 1,\,{\text{and}} \\ \frac{{p_{22}^{EDP} }}{{p_{11}^{EPP} }} & = \frac{{(1 - b)(4 + 3b)/(8 - 3b^{2} )}}{(1 - b)/(2 - b)} = \frac{(4 + 3b)(2 - b)}{{(8 - 3b^{2} )}} = \frac{{8 - 3b^{2} + 2b}}{{8 - 3b^{2} }} > 1. \\ \end{aligned}$$

Then, we can derive that $p_{11}^{EDD} > p_{11}^{EDP} > p_{11}^{EPD} (p_{22}^{EDP} ) > p_{11}^{EPP}$.

The proof of Proposition 1 is completed.□

Proof of Proposition 2

(i) $S_{i}^{EDD} /S_{i}^{EPP} { = }\frac{(1 - r)(1 - b)}{{(1 + b)(2 - b)^{2} }}/\frac{2(1 - b)}{{(1 + b)(4 - 3b)^{2} }} = \frac{{(1 - r)(4 - 3b)^{2} }}{{2(2 - b)^{2} }}$, and we can get

• if $r = r_{1} = (8 - 16b + 7b^{2} )/(3b - 4)^{2}$, then $S_{i}^{EDD} { = }S_{i}^{EPP}$;

• if $r > r_{1} = (8 - 16b + 7b^{2} )/(3b - 4)^{2}$, then $S_{i}^{EDD} > S_{i}^{EPP}$;

• if $r < r_{1} = (8 - 16b + 7b^{2} )/(3b - 4)^{2}$, then $S_{i}^{EDD} > S_{i}^{EPP}$

• $R_{i}^{EDD} /R_{i}^{EPP} { = }\frac{r(1 - b)}{{(1 + b)(2 - b)^{2} }}/\frac{(1 - b)}{{(1 + b)(4 - 3b)^{2} }} = \frac{{r(4 - 3b)^{2} }}{{(2 - b)^{2} }}$, and we can get

• if $r = r_{2} = (2 - b)^{2} /(4 - 3b)^{2}$, then $R_{j}^{EDD} { = }R_{j}^{EPP}$;

• if $r < r_{2} = (2 - b)^{2} /(4 - 3b)^{2}$, then $R_{j}^{EDD} > R_{j}^{EPP}$;

• if $r > r_{2} = (2 - b)^{2} /(4 - 3b)^{2}$, then $R_{j}^{EDD} < R_{j}^{EPP}$.

Therefore, $S_{i}^{EDD} \le S_{i}^{EPP} , \quad R_{j}^{EDD} \le R_{j}^{EPP} , \quad for \, i,j = \{ 1,2\} ; \, if \, r_{2} (b) \le r \le r_{1} (b).$

Similarly, we can derive

(ii) $S_{1}^{EDD} \le S_{1}^{EPD} , \quad R_{1}^{EDD} \le R_{1}^{EPD} , \quad if\, r_{4} (b) \le r \le r_{3} (b).$

(iii) $S_{2}^{EPD} \le S_{2}^{EPP} ,\quad R_{2}^{EPD} \le R_{2}^{EPP} ,\quad if\, r_{6} (b) \le r \le r_{5} (b).$

The Proof of Proposition 2 is completed.□

Proof of Corollary 1

$$\begin{aligned} r_{3}^{{}} - r_{1}^{{}} & = (128 - 192b^{2} + 63b^{4} )/((4 - 3b)^{2} (4 + 3b)^{2} ) - (8 - 16b + 7b^{2} )/(3b - 4)^{2} > 0, \\ r_{5}^{{}} - r_{3}^{{}} & = (32 - 32b^{2} + 7b^{4} )^{2} /(8 - 3b^{2} )^{2} - (128 - 192b^{2} + 63b^{4} )/((4 - 3b)^{2} (4 + 3b)^{2} ) > 0, \\ r_{6}^{{}} - r_{4}^{{}} & = (2 - b)^{2} (2 + b)^{2} /(8 - 3b^{2} )^{2} - (8 - 3b^{2} )^{2} /((4 - 3b)^{2} (4 + 3b)^{2} ) > 0, \\ r_{4}^{{}} - r_{2}^{{}} & = (8 - 3b^{2} )^{2} /((4 - 3b)^{2} (4 + 3b)^{2} ) - (2 - b)^{2} /(4 - 3b)^{2} > 0. \\ \end{aligned}$$

And by the expression of $r_{1} ,r_{2} ,r_{3} ,r_{4} ,r_{5} ,r_{6}$, we can attain

• If $0 < b < 0.4226$, then $r_{1} (b) > r_{2} (b)$; otherwise, $r_{1} (b) \le r_{2} (b)$.

• If $0 < b < 0.7506$, then $r_{3} (b) > r_{4} (b)$; otherwise, $r_{3} (b) \le r_{4} (b)$.

• If $0 < b < 0.9194$, then $r_{5} (b) > r_{6} (b)$; otherwise, $r_{5} (b) \le r_{6} (b)$.

• If $0 < b < 0.5021$, then $r_{1} (b) > r_{4} (b)$; otherwise, $r_{1} (b) \le r_{4} (b)$.

• If $0 < b < 0.5144$, then $r_{1} (b) > r_{6} (b)$; otherwise, $r_{1} (b) \le r_{6} (b)$.

• If $0 < b < 0.6074$, then $r_{3} (b) > r_{2} (b)$; otherwise, $r_{3} (b) \le r_{2} (b)$.

• If $0 < b < 0.7899$, then $r_{3} (b) > r_{6} (b)$; otherwise, $r_{3} (b) \le r_{6} (b)$.

• If $0 < b < 0.6518$, then $r_{5} (b) > r_{2} (b)$; otherwise, $r_{5} (b) \le r_{2} (b)$.

• If $0 < b < 0.8312$, then $r_{5} (b) > r_{4} (b)$; otherwise, $r_{5} (b) \le r_{4} (b)$.

Therefore, if $b \in (0,0.4026)$, then $r_{6} (b) \le r_{4} (b) \le r_{2} (b) \le r_{1} (b) \le r_{3} (b) \le r_{5} (b)$. Based on the above results, we can derive the first Corollary 1.

The Proof of Corollary 1 is completed.□

Proof of Theorem 1

From Corollary 1, we can derive that:

• (1) If $b \in (0,0.4226 )$, then $S_{i}^{EDD} < S_{i}^{EPP}$, $S_{2}^{EDD} < S_{2}^{EDP}$, and $S_{1}^{EDP} < S_{1}^{EPP}$; $R_{1}^{EDD} < R_{1}^{EPP}$, $R_{1}^{EDD} < R_{1}^{EDP}$, and $R_{1}^{EDP} < R_{1}^{EPP}$; $R_{2}^{EDD} < R_{2}^{EPP}$, $R_{2}^{EDD} < R_{2}^{EDP}$, and $R_{2}^{EDP} < R_{2}^{EPP}$.

• (2) If $b \in (0,0.9194 )$, then $S_{i}^{EDD} > S_{i}^{EPP}$, $S_{2}^{EDD} > S_{2}^{EDP}$, and $S_{1}^{EDP} > S_{1}^{EPP}$; $R_{1}^{EDD} > R_{1}^{EPP}$, $R_{1}^{EDD} > R_{1}^{EDP}$, and $R_{1}^{EDP} > R_{1}^{EPP}$; $R_{2}^{EDD} > R_{2}^{EPP}$, $R_{2}^{EDD} > R_{2}^{EDP}$, and $R_{2}^{EDP} > R_{2}^{EPP}$.

Therefore, we can derive that

• (i) for any given $b \in (0,0.2037)$, there exist $r_{1}^{{}}$ and $r_{2}^{{}}$ such that a platform selling format is the unique Nash equilibrium for all supply chain members, as long as $r_{2}^{{}} < r < r_{1}^{{}}$.

• (ii) for any given $b \in (0.9194,1)$, there exists $r_{5}^{{}}$ and $r_{6}^{{}}$ such that a reselling format is the unique Nash equilibrium for all supply chain members, as long as $r_{5}^{{}} < r < r_{6}^{{}}$.

The Proof of Theorem 1 is completed.□

Proof of Lemmas 4–7

For the case CDD, take the first derivative of Eq. (8) w.r.t. $p_{11}^{{}}$ and $p_{12}^{{}}$:

$$\frac{{\partial R_{1}^{CDD} }}{{\partial p_{11}^{CDD} }} = a - \beta (2p_{11} - w_{11} ) + \theta (p_{21} - w_{21} ) + \theta (p_{12} + \, p_{21} + \, p_{22} ) = 0,\,{\text{and}}$$

(16)

$$\frac{{\partial R_{2}^{CDD} }}{{\partial p_{12}^{CDD} }} = a - \beta (2p_{12} - w_{12} ) + \theta (p_{22} - w_{22} ) + \theta (p_{11} + \, p_{21} + \, p_{22} ) = 0.$$

(17)

The Hessian matrix is:

$$H = \left[ {\begin{array}{*{20}c} {\frac{{\partial^{2} R_{1}^{CDD} }}{{\partial p_{11}^{CDD2} }}} & {\frac{{\partial^{2} R_{1}^{CDD} }}{{\partial p_{11}^{CDD} \partial p_{21}^{CDD} }}} \\ {\frac{{\partial^{2} R_{1}^{CDD} }}{{\partial p_{21}^{CDD} \partial p_{11}^{CDD} }}} & {\frac{{\partial^{2} R_{1}^{CDD} }}{{\partial p_{21}^{CDD2} }}} \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} { - 2\beta } & \theta \\ \theta & { - 2\beta } \\ \end{array} } \right],\quad {\text{and}}\,{\text{then}}\,\left| H \right| > 0.$$

With (16) and (17), we have:

$$p_{11} = (a - w_{11} - ab + bp_{12} + 2bp_{21} + bp_{22} + 2bw_{11} - bw_{21} )/(2b + 2),\,{\text{and}}$$

(18)

$$p_{12} = (a - w_{12} - ab + bp_{11} + 2bp_{22} + bp_{21} + 2bw_{12} - bw_{22} )/(2b + 2),$$

(19)

Inserting Eqs. (18) and (19) into the suppliers’ profit functions (7) and using the first order conditions (FOCs), we can get the optimal values of $w_{ij}^{CDD}$,which is shown as Eq. (10). Putting Eq. (10) into Eqs. (18) and (19), and then solving them, we can attain the optimal values of $p_{ij}^{CDD}$ shown as Eq. (9).

The Hessian matrix is following as:

$$\begin{aligned} H & = \left[ {\begin{array}{*{20}c} {\frac{{\partial^{2} S_{1}^{CDD} }}{{\partial w_{11}^{CDD2} }}} & {\frac{{\partial^{2} S_{1}^{CDD} }}{{\partial w_{11}^{CDD} \partial w_{21}^{CDD} }}} \\ {\frac{{\partial^{2} S_{1}^{CDD} }}{{\partial w_{21}^{CDD} \partial w_{11}^{CDD} }}} & {\frac{{\partial^{2} S_{1}^{CDD} }}{{\partial w_{21}^{CDD2} }}} \\ \end{array} } \right],\,{\text{and}} \\ \left| H \right| & = (3311b^{6} + 10736b^{5} + 14204b^{4} + 9856b^{3} \\ & \quad + 3792b^{2} + 768b + 64)/[64(1 + 2b)^{4} (1 + 2b - 3b^{2} )] > 0. \\ \end{aligned}$$

The Proof of Lemma 4 is completed.□

The Proof of Lemma 5–7 is similar to the Proof of Lemma 4.

Proof of Proposition 3

From $p_{11}^{CDD}$ and $p_{11}^{CDD}$’s expression, we can get

• If $0 < b < (\sqrt {20 - 4r + r^{2} } + r)/(10 - 2r)$, and then $\frac{{p_{11}^{CDD} }}{{p_{11}^{CDP} }} = \frac{3(1 - b)}{2(2 - b)}/\frac{{4 + (11 + r)b - 2b^{2} - (r + 13)b^{3} }}{{2(2 + 8b + (1 - r + 6)b^{2} - (1 + r)b^{3} )}} > 1$;

• if $(\sqrt {20 - 4r + r^{2} } + r)/(10 - 2r) \le b < 1$, and then $\frac{{p_{11}^{CDD} }}{{p_{11}^{CDP} }} = \frac{3(1 - b)}{2(2 - b)}/\frac{{4 + (11 + r)b - 2b^{2} - (r + 13)b^{3} }}{{2(2 + 8b + (1 - r + 6)b^{2} - (1 + r)b^{3} )}} \le 1$;

In addition,

$$\begin{aligned} \frac{{p_{11}^{CDP} }}{{p_{11}^{C(DP)(DP)} }} & = \frac{{\left( {1 - b} \right)\left( {15b + br + b^{2} r + 13b^{2} + 4} \right)\left( {30b - 2b^{2} r - 2b^{3} r + 24b^{2} - 6b^{3} + 8} \right)}}{{\left( { - \, 17b^{3} + 13b + 4} \right)\left( {16b - 2b^{2} r - 2b^{3} r + 14b^{2} - 2b^{3} + 4} \right)}} > 1, \\ \frac{{p_{11}^{C(DP)(DP)} }}{{p_{11}^{C(PD)(PD)} }} & = \frac{{\left( {22b + br + b^{2} r + 20b^{2} + 6} \right)}}{{\left( {4 - \, 17b^{3} + 17b} \right)}} > 1, \\ \frac{{p_{11}^{C(DP)(DP)} }}{{p_{11}^{CPD} }} & = \frac{{\left( { - \, 17b^{3} + 13b + 4} \right)\left( {16b - 2b^{2} r - 2b^{3} r + 14b^{2} - 2b^{3} + 4} \right)}}{{\left( { - { 9}b^{3} + 7b + 2} \right)\left( {30b - 2b^{2} r - 2b^{3} r + 24b^{2} - 6b^{3} + 8} \right)}} > 1, \\ \frac{{p_{11}^{CPD} }}{{p_{11}^{CPP} }} & = \frac{{\left( { 9b^{3} + 9b + 2} \right)}}{{\left( {8b - b^{2} r - b^{3} r + 7b^{2} - 6b^{3} + 2} \right)}} > 1. \\ \end{aligned}$$

Then, we can derive Proposition 3.

The proof of Proposition 3 is completed.□

Proof of Proposition 4

Let r₇ be the threshold of $G_{1}^{CPP} = G_{1}^{C(DP)(DP)}$, yielding,

$$r_{ 7} (b){ = }\frac{{\sqrt {\Delta_{ 1} } - 44b^{ 6} + 123b^{ 5} + 183b^{ 4} + 32b^{ 3} - 28b^{ 2} - 8b}}{{9b^{ 6} + 4b^{ 5} + 3b^{ 4} + 12b^{ 3} + 4b^{ 2} }}.$$

Let r₈ be the threshold of $G_{1}^{CDD} = G_{1}^{C(DP)(DP)}$, yielding,

$$r_{ 8} (b){ = }\frac{{ - 6b^{ 5} { + }52b^{ 4} { + }79b^{ 3} { + }28b^{ 2} - 3b - 2}}{{2b^{ 5} + 4b^{ 4} + 5b^{ 3} + 4b^{ 2} + b}},$$

where

$$\begin{aligned} \Delta_{ 1} & = 5491b^{ 1 2} - 13294b^{ 1 1} - 15045b^{ 1 0} + 31620b^{ 9} { + }31482b^{ 8} - 16372b^{ 7} \\ & \quad - 27526b^{ 6} - 8616b^{ 5} + 1064b^{ 4} + 960b^{ 3} + 128b^{ 2} \\ \end{aligned}$$

The rectangular area $\{ (b,r )\left| {(0 < b < 1,0 < r < 1)} \right.\}$ is divided into four regions (IV, V, VI and VII) by three curves: $G_{1}^{CDD} (b,r) = G_{1}^{CPP} (b,r)$, $G_{1}^{CDD} (b,r) = G_{1}^{C(DP)(DP)} (b,r)$ and $G_{1}^{CPP} (b,r) = G_{1}^{C(DP)(DP)} (b,r)$. then, we can get,

• If $(b,r) \in IV$, and then $G_{1}^{CDD} (b,r) < G_{1}^{C(DP)(DP)} (b,r) < G_{1}^{CPP} (b,r)$;

• If $(b,r) \in V$, and then $G_{1}^{CDD} (b,r) < G_{1}^{CPP} (b,r) < G_{1}^{C(DP)(DP)} (b,r)$;

• If $(b,r) \in VI$, and then $G_{1}^{CPP} (b,r) < G_{1}^{CDD} (b,r) < G_{1}^{C(DP)(DP)} (b,r)$;

• If $(b,r) \in VII$, and then $G_{1}^{CPP} (b,r) < G_{1}^{C(DP)(DP)} (b,r) < G_{1}^{CDD} (b,r)$.

Therefore, we can derive Proposition 4.

The proof of Proposition 4 is completed.□

Proof of Proposition 5

$$G_{1}^{CDD} - G_{1}^{CPP} = \frac{{3(1 - b^{2} )}}{{2(1 + 3b)(2 - b)^{2} }} - \frac{{(1 - b^{2} )}}{2(1 + 3b)} = \frac{{(1 - b^{2} )[3 - 2(2 - b)^{2} ]}}{{2(1 + 3b)(2 - b)^{2} }},$$

Then, we can get that when $0 < b < 0.2679$, there exists $G_{1}^{CDD} < G_{1}^{CPP}$.

The proof of Proposition 5 is completed.□

Proof of Proposition 6

$$G_{1}^{CDD} - G_{1}^{CPD} = \frac{{3(1 - b^{2} )}}{{2(1 + 3b)(2 - b)^{2} }} - \frac{{(1 + 3b)(2 + 3b)(2 + 5b)(1 - b^{2} )}}{{2(2 + 8b + (1 - r + 6)b^{2} - (1 + r)b^{3} )^{2} }}$$

Let r₉ be the threshold of $G_{1}^{CPD} = G_{1}^{CDD}$, yielding,

$$r_{ 9} = \frac{{\sqrt {b(16 - 5\Delta_{ 2} )} + (3b^{2} - 2)\sqrt {\Delta_{ 2} } - 2b^{3} + 14b^{2} + 4}}{{2b^{2} (1 + b)}},\quad \Delta_{ 2} = 4(15b^{2} + 16b + 4)/3.$$

In addition,

• when $0 < b < 0. 7 6 2 4$, there exists $0 > r_{ 9} (b)$;

• when $0. 7 6 2 4< b < 0. 9 5 7 4$, there exists $1 > r > r_{ 9} (b)$.

Therefore, when one e-retailer in the cross-sales supply chain is using a traditional reselling format, if (i) $0 < b < 0. 7 6 2 4$, or (ii) $0. 7 6 2 4< b < 0. 9 5 7 4$ and $1 > r > r_{ 9} (b)$, the other e-retailer is incentivized to move from a traditional reselling format to a platform selling format,

$${\text{where}}\,r_{ 9} = \frac{{\sqrt {b(16 - 5\Delta_{ 2} )} + (3b^{2} - 2)\sqrt {\Delta_{ 2} } - 2b^{3} + 14b^{2} + 4}}{{2b^{2} (1 + b)}},\quad \Delta_{ 2} = 4(15b^{2} + 16b + 4)/3.$$

The proof of Proposition 6 is completed.□

Proof of Proposition 7

$$G_{1}^{CPP} - G_{1}^{CDP} = \frac{{(1 - b^{2} )}}{2(1 + 3b)} - \frac{{(1 - r)^{2} (1 + 3b)(1 - b^{2} )b^{2} }}{{(2 + 8b + (1 - r + 6)b^{2} - (1 + r)b^{3} )^{2} }} > 0,$$

Then, when one e-retailer in the cross-sales supply chain is using a platform selling format, the other e-retailer is incentivized to move from a traditional reselling format to a platform selling format, as $G_{1}^{CDP} < G_{1}^{CPP}$.

The proof of Proposition 7 is completed.□

Proof of Theorem 2

The rectangular area $\{ (b,r )\left| {(0 < b < 1,0 < r < 1)} \right.\}$ is divided into four regions (I, II and III) by two curves: $G_{1}^{CDD} (b,r) = G_{1}^{CPP} (b,r)$, $G_{1}^{CDD} (b,r) = G_{1}^{CDP} (b,r)$ and $G_{1}^{CPP} (b,r) = G_{1}^{CDP} (b,r)$.

Then, we can get if $(b,r) \in I$ (i.e. $0 < b < 0.2679$), then $G_{1}^{CDD} (b,r) < G_{1}^{CDP} (b,r) < G_{1}^{CPP} (b,r);$

• If $(b,r) \in II$, (i.e. $02679 < b < 0. 7 6 2 4$, or $0. 7 6 2 4< b < 0. 9 5 7 4$ and $1 > r > r_{ 9} (b)$),

• then $G_{1}^{CDD} (b,r) < G_{1}^{CPP} (b,r) < G_{1}^{CDP} (b,r)$;

• If $(b,r) \in III$,(i.e. $0. 9 5 7 4< b < 1$, or $0. 7 6 2 4< b < 0. 9 5 7 4$ and $0 < r < r_{ 9} (b)$),

• then $G_{1}^{CDD} (b,r) < G_{1}^{C(DP)(DP)} (b,r),G_{1}^{CPP} (b,r) < G_{1}^{C(DP)(DP)} (b,r)$.

In the other words, under a cross-sales supply chain, b can be found such that platform selling is the unique Nash equilibrium for all groups as $0 < b < 0.2679$.

The proof of Theorem 2 is completed.□