Scaled Selection Combining for Adaptive Decode-and-Forward Relaying in Dissimilar Rayleigh Fading Channels

Abstract

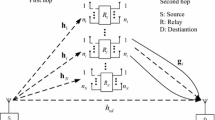
This paper enhances the conventional scaled selection combiner (SSC) for decode-and-forward (DF) relay networks using adaptive M-ary quadrature amplitude modulation (M-QAM) to improve the spectral efficiency. Compared with the conventional SSC designed for the combining of identically distributed diversity branches using the same modulation level, the improved SSC allows all diversity branches to choose different modulation levels according to the dissimilar channel conditions. Different scale factors are used for all diversity branches to reflect not only the performance degradation caused by possible erroneous relaying but also different error-resistance abilities of different levels QAM. We derive the bit-error-rate (BER) expressions for DF relay networks using SSC in a recursive way, with all channels conforming to independently and non-identically distributed (i.ni.d.) Rayleigh fading. Newton’s method is employed to obtain the numerical solutions of the optimal scale factors minimizing the BER, and the approximations of the optimal scale factors are derived in closed form for high SNRs. Theoretical analysis and simulation results show that the improved SSC can effectively combine diversity branches with different modulation levels, and for a DF cooperative network with N relay nodes, SSC achieves the full diversity gain of N+1 if for each branch its source-to-relay SNR is proportional to the (N+1)th power of its relay-to-destination SNR.

Appendices

Appendix 1: Proof of Proposition 1

First, we give the Nth order Taylor series expansion of $g(x)$ at the point $x=0$ as

$$\begin{aligned} g(x) = \frac{1}{{Z + x}} \mathop {\approx }\limits ^{x \rightarrow 0} \frac{1}{Z}\sum \limits _{n = 0}^N \left( \frac{{ - x}}{Z}\right) ^{n} = \frac{1}{Z} - \frac{x}{Z^{2}} + \frac{x^{2}}{Z^{3}} - \frac{x^{3}}{Z^{4}} + \cdots \end{aligned}$$

(38)

where $Z$ denotes a constant. And we give the Nth order Taylor series expansion of $h(x)$ at the point $x=0$ as

$$\begin{aligned} h(x)= 1 - \sqrt{\frac{1}{{1 + x}}} \mathop {\approx }\limits ^{x \rightarrow 0}\sum \limits _{n = 0}^N \frac{{h^{(n)}}(0)}{{n!}}x^{n} = \frac{1}{2}x - \frac{3}{8}x^{2} + \frac{5}{16}x^{3} - \frac{35}{128}x^{4} + \cdots \end{aligned}$$

(39)

Then when $\Gamma _{\mathrm{SD}} \rightarrow \infty$ and thus $\alpha _{0,1} = \beta _{1}/\Gamma _{\mathrm{SD}}$ approach zeros, by using the second-order Taylor series expansion of $g(x), \varPhi _{\mathrm{SR}1\mathrm{D}}(2)$ given by (7) can be approximated as

$$\begin{aligned}&{\varPhi _{{\mathrm{SR}}1{\mathrm{D}}}}(2) = \frac{1}{{{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}}}\left( {\frac{1}{{{\alpha _{1,1}}}} - \frac{1}{{{\alpha _{1,1}} + {\alpha _{0,1}}}}} \right) \nonumber \\&\quad \mathop {\approx }\limits ^{{\alpha _{0,1}} \rightarrow 0} \frac{1}{{{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}}}\left( {\frac{1}{{{\alpha _{1,1}}}} - \frac{1}{{{\alpha _{1,1}}}} + \frac{{{\alpha _{0,1}}}}{{{{\left( {{\alpha _{1,1}}} \right) }^2}}}} \right) = \frac{1}{{{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}}}\frac{{{\alpha _{0,1}}}}{{{{\left( {{\alpha _{1,1}}} \right) }^2}}} \end{aligned}$$

(40)

And when $X \rightarrow 0$ in (10), by using the second-order Taylor series expansion of $h(x)$, ${P_{Ray}}(M,\Gamma ,X)$ given by (10) can be approximated by

$$\begin{aligned} {P_{Ray}}(M,\Gamma ,X) = \frac{{{c_M}}}{{2\Gamma X}}\left( {1 - \sqrt{\frac{{d_M^2}}{{d_M^2 + X}}} } \right) \mathop {\approx }\limits ^{X \rightarrow 0} \frac{{{c_M}}}{{4\Gamma d_M^2}} - \frac{{3{c_M}X}}{{16\Gamma d_M^4}} \end{aligned}$$

(41)

Therefore, when ${\Gamma _{{\mathrm{SD}}}},\;{\Gamma _{{\mathrm{R1D}}}} \rightarrow \infty$ and thus ${\alpha _{n,i}} \rightarrow 0$ ($n=0, 1$, and $i=0, 1$), by substituting (40) and (41), ${P_{Ray(2)}}$ given by (12) can be written as

$$\begin{aligned} {P_{Ray(2)}}&\approx \frac{{3{c_{M{\mathrm{S}}}}}}{{16d_{M{\mathrm{S}}}^4}}{\alpha _{0,0}}{\alpha _{1,0}} + P_{Ray}^{{\mathrm{SR}}1}\frac{1}{{{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}}}\frac{{{\alpha _{0,1}}}}{{{{\left( {{\alpha _{1,1}}} \right) }^2}}}\nonumber \\& \quad + \left( {1 - 2P_{Ray}^{{\mathrm{SR}}1}} \right) \frac{{3{c_{M{\mathrm{S}}}}}}{{16d_{M{\mathrm{S}}}^4}}{\alpha _{0,1}}{\alpha _{1,1}}. \end{aligned}$$

(42)

When $P_{Ray}^{{\mathrm{SR}}1} \ll 1$, $P_{Ray}^{{\mathrm{SR}}1}$ in the third term of (42) can be ignored. Then by substituting (3), (42) turns into

$$\begin{aligned} {P_{Ray(2)}}&\approx {\left( {{\Gamma _{{\mathrm{SD}}}}{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}} \right) ^{ - 1}}\frac{3}{{16}}\left( {\frac{{{c_{M{\mathrm{S}}}}}}{{d_{M{\mathrm{S}}}^4{\beta _1}}} + \frac{{{c_{M{\mathrm{R}}i}}{\beta _1}}}{{d_{M{\mathrm{R}}i}^4}}} \right) + P_{Ray}^{{\mathrm{SR}}1}\frac{{{\beta _1}{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}}}{{{\Gamma _{{\mathrm{SD}}}}}}\nonumber \\&= {\left( {{\Gamma _{{\mathrm{SD}}}}{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}} \right) ^{ - 1}}{k_1} + P_{Ray}^{{\mathrm{SR}}1}\frac{{{\beta _1}{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}}}{{{\Gamma _{{\mathrm{SD}}}}}}, \end{aligned}$$

(43)

where $k_1$ is a positive constant.

When ${\Gamma _{{\mathrm{SR}}i}} \rightarrow \infty , i=1,\ldots ,N$, by using the first-order Taylor series expansion of $h(x)$, $P_{Ray}^{{\mathrm{SR}}i}$ can be approximated as

$$\begin{aligned} P_{Ray}^{{\mathrm{SR}}i} = {P_{Ray}}\left( {{M_{\mathrm{S}}},{\Gamma _{{\mathrm{SR}}i}},\frac{1}{{{\Gamma _{{\mathrm{SR}}i}}}}} \right) \approx \frac{{{c_{M{\mathrm{S}}}}}}{{4d_{M{\mathrm{S}}}^2{\Gamma _{{\mathrm{SR}}i}}}}, \end{aligned}$$

(44)

$i = 1,\ldots ,N$. By substituting (44), (43) turns into

$$\begin{aligned} {P_{Ray(2)}} \approx {\left( {{\Gamma _{{\mathrm{SD}}}}{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}} \right) ^{- 1}}\left( {{k_1} + \frac{{{{\left( {{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}} \right) }^2}}}{{{\Gamma _{{\mathrm{SR}}1}}}}\frac{{{c_{M{\mathrm{S}}}}}}{{4d_{M{\mathrm{S}}}^2}}} \right) = {\left( {{\Gamma _{{\mathrm{SD}}}}{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}} \right) ^{ - 1}}\left( {{k_1} + \frac{{{{\left( {{\Gamma _{{\mathrm{R}}1{\mathrm{D}}}}} \right) }^2}}}{{{\Gamma _{{\mathrm{SR}}1}}}}{k_2}} \right) , \end{aligned}$$

(45)

with $k_2$ denoting a positive constant.

Appendix 2: Proof of Proposition 2

When ${\Gamma _{{\mathrm{SD}}}},\;{\Gamma _{{\mathrm{R}}n{\mathrm{D}}}} \rightarrow \infty \;(n = 1,\ldots ,N)$ and thus ${\alpha _{n,i}} \rightarrow 0\;(n = 0,\ldots ,N$ and $i = 0,\ldots ,N)$, by using the (N+1)th order Taylor series expansion of $h(x)$, ${P_{Ray(N + 1)}}$ given by (21) can be approximated by

$$\begin{aligned} {P_{Ray(N + 1)}}&\approx \frac{{\left| {{h^{(N + 1)}}(0)} \right| }}{{2(N + 1)}}\frac{{{c_{M{\mathrm{S}}}}}}{{d_{M{\mathrm{S}}}^{2(N + 1)}}}\prod \limits _{n = 0}^N {{\alpha _{n,0}}} + \sum \limits _{i = 1}^N {(P_{Ray}^{{\mathrm{SR}}i}{\varPhi _{{\mathrm{SR}}i{\mathrm{D}}}}(N + 1)}\nonumber \\& \quad + \left( {1 - 2P_{Ray}^{{\mathrm{SR}}i}} \right) \frac{{\left| {{h^{(N + 1)}}(0)} \right| }}{{2(N + 1)}}\frac{{{c_{M{\mathrm{R}}i}}}}{{d_{M{\mathrm{R}}i}^{2(N + 1)}}}\prod \limits _{n = 0}^N {{\alpha _{n,i}}}). \end{aligned}$$

(46)

When $P_{Ray}^{{\mathrm{SR}}i} \ll 1,\;i=1,\ldots ,N$, $P_{Ray}^{{\mathrm{SR}}i}$ in the third term of (46) can be ignored. Then by substituting (3), (46) turns into

$$\begin{aligned} {P_{Ray(N + 1)}}&\approx {\left( {{\Gamma _{{\mathrm{SD}}}}}\right) ^{-1}} \prod \limits _{n = 1}^N {{{\left( {{\Gamma _{{\mathrm{R}}n{\mathrm{D}}}}} \right) }^{-1}}}\frac{{\left| {{h^{(N+1)}}(0)}\right| }}{{2(N+1)}}\nonumber \\&\cdot \left[ {\frac{{{c_{M{\mathrm{S}}}}}}{{d_{M{\mathrm{S}}}^{2(N + 1)}}}\prod \limits _{n = 0}^N {\frac{1}{{{\beta _n}}}} + \sum \limits _{i = 1}^N {\left( {\frac{{{c_{M{\mathrm{R}}i}}}}{{d_{M{\mathrm{R}}i}^{2(N + 1)}}}\prod \limits _{n = 0}^N{\frac{{{\beta _i}}}{{{\beta _n}}}}}\right) }}\right] \nonumber \\&\quad + \sum \limits _{i = 1}^N {P_{Ray}^{{\mathrm{SR}}i}{\varPhi _{{\mathrm{SR}}i{\mathrm{D}}}}(N + 1)}\nonumber \\&= {\left( {{\Gamma _{{\mathrm{SD}}}}} \right) ^{-1}}\prod \limits _{n = 1}^N {{{\left( {{\Gamma _{{\mathrm{R}}n{\mathrm{D}}}}} \right) }^{ - 1}}} {m_0} + \sum \limits _{i = 1}^N {P_{Ray}^{{\mathrm{SR}}i}{\varPhi _{{\mathrm{SR}}i{\mathrm{D}}}}(N + 1)} \end{aligned}$$

(47)

where $m_{0}$ is a positive constant.

By using the (N+1)th-order Taylor series expansion of $g(x)$, ${\varPhi _{{\mathrm{SR}}i{\mathrm{D}}}}(N + 1)$ given by (7) can be approximated by

$$\begin{aligned} {\varPhi _{{\mathrm{SR}}i{\mathrm{D}}}}(N + 1)&\approx \frac{1}{{ {\Gamma _{{\mathrm{R}}i{\mathrm{D}}}}}}\frac{{N!}}{{{{ \left( {{\alpha _{i,i}}} \right) }^{N +1}}}}\prod \limits _{n = 0,n \ne i}^N {{\alpha _{n,i}}}\nonumber \\&= {\left( {{\Gamma _{{\mathrm{SD}}}}}\right) ^{-1}}\prod \limits _{n = 1}^N {{{\left( {{\Gamma _{{\mathrm{R}}n{\mathrm{D}}}}} \right) }^{-1}}} \left( {N!{{\left( {{\Gamma _{{\mathrm{R}}i{\mathrm{D}}}}} \right) }^{N + 1}}\prod \limits _{n = 0}^N {\frac{{{\beta _i}}}{{{\beta _n}}}}}\right) . \end{aligned}$$

(48)

By substituting (44) and (48), ${P_{Ray(N + 1)}}$ can be written as

$$\begin{aligned} {P_{Ray(N + 1)}}&= {\left( {{\Gamma _{{\mathrm{SD}}}}} \right) ^{ - 1}}\prod \limits _{n = 1}^N {{{\left( {{\Gamma _{{\mathrm{R}}n{\mathrm{D}}}}} \right) }^{-1}}}\nonumber \\&\quad \cdot \left( {{m_{0}} + \sum \limits _{i = 1}^{N} {\left( {\frac{{{{\left( {{\Gamma _{{\mathrm{R}}i{\mathrm{D}}}}}\right) }^{N + 1}}}}{{{\Gamma _{{\mathrm{SR}}i}}}}\frac{{{c_{M{\mathrm{S}}}}}}{{4d_{M{\mathrm{S}}}^2}}N!\prod \limits _{n = 0}^N {\frac{{{\beta _i}}}{{{\beta _n}}}}}\right) }}\right) \nonumber \\&= {\left( {{\Gamma _{{\mathrm{SD}}}}} \right) ^{-1}}\prod \limits _{n = 1}^N {{{\left( {{\Gamma _{{\mathrm{R}}n{\mathrm{D}}}}} \right) }^{-1}}} \left( {{m_0} + \sum \limits _{i = 1}^N {\frac{{{{\left( {{\Gamma _{{\mathrm{R}}i{\mathrm{D}}}}} \right) }^{N + 1}}}}{{{\Gamma _{{\mathrm{SR}}i}}}}{m_i}}}\right) , \end{aligned}$$

(49)

with $m_i,\,i=1,\ldots ,N$, denoting positive constants.