Skip to main content
SpringerLink
Log in

Accurate Implicit–Explicit General Linear Methods with Inherent Runge–Kutta Stability

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

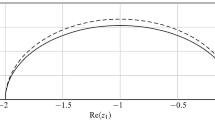
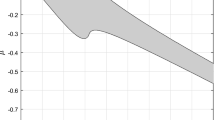
Abstract

We investigate implicit–explicit (IMEX) general linear methods (GLMs) with inherent Runge–Kutta stability (IRKS) for differential systems with non-stiff and stiff processes. The construction of such formulas starts with implicit GLMs with IRKS which are A- and L-stable, and then we ‘remove’ implicitness in non-stiff terms by extrapolating unknown stage derivatives by stage derivatives which are already computed by the method. Then we search for IMEX schemes with large regions of absolute stability of the ‘explicit part’ of the method assuming that the ‘implicit part’ of the scheme is \(A(\alpha )\)-stable for some \(\alpha \in (0,\pi /2]\). Examples of highly stable IMEX GLMs are provided of order \(1\le p\le 4\). Numerical examples are also given which illustrate good performance of these schemes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24

Similar content being viewed by others

References

  1. Abdulle, A., Vilmart, G.: PIROCK: a swiss-knife partitioned implicit–explicit orthogonal Runge–Kutta–Chebyshev integrator for stiff diffusion–advection–reaction problems with or without noise. J. Comput. Phys. 242, 869–888 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  2. Ascher, U.M., Ruuth, S.J., Spiteri, R.J.: Implicit–explicit Runge–Kutta methods for time-dependent partial differential equations. Appl. Numer. Math. 25, 151–167 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  3. Ascher, U.M., Ruuth, S.J., Wetton, B.: Implicit–explicit methods for time dependent PDE’s. SIAM J. Numer. Anal. 32, 797–823 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  4. Beck, S., Weiner, R., Podhaisky, H., Schmitt, B.A.: Implicit peer methods for large stiff ODE systems. J. Appl. Math. Comput. 38, 389–406 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  5. Boscarino, S.: Error analysis of IMEX Runge–Kutta methods derived from differential–algebraic systems. SIAM J. Numer. Anal. 45, 1600–1621 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  6. Boscarino, S.: On the accurate third order implicit–explicit Runge–Kutta methods for stiff problems. Appl. Numer. Math. 59, 1515–1528.37 (2009), B305–B331 (2015)

  7. Boscarino, S., Bürger, R., Mulet, P., Russo, G., Villada, M.L.: Linearly implicit IMEX Runge–Kutta methods for a class of degenerate convection–diffusion problems. SIAM J. Sci. Comput. 37, B305–B331 (2015). doi:10.1137/140967544

  8. Boscarino, S., Russo, G.: On a class of uniformly accurate IMEX Runge–Kutta schemes and applications to hyperbolic systems with relaxation. SIAM J. Sci. Comput. 31, 1926–1945 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  9. Braś, M., Cardone, A., Jackiewicz, Z., Welfert, B.: Order reduction phenomenon for general linear methods. SIAM J. Number. Anal. (submitted)

  10. Butcher, J.C.: The Numerical Analysis of Ordinary Differential Equations. Runge–Kutta and General Linear Methods. Wiley, Chichester (1987)

    MATH  Google Scholar 

  11. Butcher, J.C.: Diagonally-implicit multi-stage integration methods. Appl. Numer. Math. 11, 347–363 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  12. Butcher, J.C.: Numerical Methods for Ordinary Differential Equations. Wiley, Chichester (2003)

    Book  MATH  Google Scholar 

  13. Butcher, J.C.: General linear methods. Acta Numer. 15, 157–256 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  14. Butcher, J.C., Jackiewicz, Z.: Diagonally implicit general linear methods for ordinary differential equations. BIT 33, 452–472 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  15. Butcher, J.C., Jackiewicz, Z.: Construction of general linear methods with Runge–Kutta stability properties. Numer. Algorithms 36, 53–72 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  16. Butcher, J.C., Jackiewicz, Z., Wright, W.M.: Error propagation for general linear methods for ordinary differential equations. J. Complex. 23, 560–580 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  17. Butcher, J.C., Wright, W.M.: The construction of practical general linear methods. BIT 43, 695–721 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  18. Calvo, M.P., de Frutos, J., Novo, J.: Linearly implicit Runge–Kutta methods for advection–diffusion–reaction problems. Appl. Numer. Math. 37, 535–549 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  19. Cardone, A., Jackiewicz, Z., Sandu, A., Zhang, H.: Extrapolation-based implicit–explicit general linear methods (2013), arXiv:1304.2276

  20. Cardone, A., Jackiewicz, Z., Sandu, A., Zhang, H.: Extrapolation-based implicit–explicit general linear methods. Numer. Algorithms 65, 377–399 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  21. Cardone, A., Jackiewicz, Z., Sandu, A., Zhang, H.: Extrapolated implicit–explicit Runge–Kutta methods. Math. Model. Anal. 19, 18–43 (2014)

    Article  MathSciNet  Google Scholar 

  22. Cardone, A., Jackiewicz, Z., Sandu, A., Zhang, H.: Construction of highly stable implicit-explicit general linear methods. In: Discrete and Continuous Dynamical Systems. Series S, vol. 2015, pp. 185–194 (2015)

  23. Crouzeix, M.: Une méthode multipas implicite-explicite pour l’approximation des équations d’évolution paraboliques. Numer. Math. 35, 257–276 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  24. Dym, H., McKean, H.P.: Fourier Series and Integrals. Academic Press, New York (1972)

    MATH  Google Scholar 

  25. Frank, J., Hundsdorfer, W., Verwer, J.G.: On the stability of implicit–explicit linear multistep methods. Appl. Numer. Math. 25, 193–205 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  26. Hairer, E., Nørsett, S.P., Wanner, G.: Solving Ordinary Differential Equations I: Nonstiff Problems. Springer, New York (1993)

    MATH  Google Scholar 

  27. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations II. Stiff and Differential–Algebraic Problems. Springer, Berlin (1996)

    Book  MATH  Google Scholar 

  28. Hundsdorfer, W., Ruuth, S.J.: IMEX extensions of linear multistep methods with general monotonicity and boundedness properties. J. Comput. Phys. 225, 2016–2042 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  29. Hundsdorfer, W., Verwer, J.G.: Numerical Solution of Time-Dependent Advection–Diffusion–Reaction Equations. Springer, Berlin, Heidelberg, New York (2003)

    Book  MATH  Google Scholar 

  30. Izzo, G., Jackiewicz, Z.: Highly stable implicit–explicit Runge–Kutta methods. Appl. Number. Math. (submitted)

  31. Jackiewicz, Z.: Implementation of DIMSIMs for stiff differential systems. Appl. Numer. Math. 42, 251–267 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  32. Jackiewicz, Z.: Construction and implementation of general linear methods for ordinary differential equations. A review. J. Sci. Comput. 25, 29–49 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  33. Jackiewicz, Z.: General Linear Methods for Ordinary Differential Equations. Wiley, Hoboken (2009)

    Book  MATH  Google Scholar 

  34. Jebens, S., Knoth, O., Weiner, R.: Partially implicit peer methods for the compressible Euler equations. J. Comput. Phys. 230, 4955–4974 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  35. Kennedy, C.A., Carpenter, M.H.: Additive Runge–Kutta schemes for convection–diffusion–reaction equations. Appl. Numer. Math. 44, 139–181 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  36. Layton, A.T., Minion, M.L.: Implications of the choice of quadrature nodes for Picard integral deferred correction methods for ordinary differential equations. BIT 45, 341–373 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  37. Minion, M.L.: Semi-implicit projection methods for incompressible flow based on spectral deferred corrections. Appl. Numer. Math. 48, 369–387 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  38. Pareschi, L., Russo, G.: Implicit–explicit Runge–Kutta schemes for stiff systems of differential equations. Recent trends in numerical analysis, 269–288, Adv. Theory Comput. Math., 3, Nova Science Publishers, Huntington, NY (2001)

  39. Pareschi, L., Russo, G.: Implicit–explicit Runge–Kutta schemes and applications to hyperbolic systems with relaxation. J. Sci. Comput. 25, 129–155 (2005)

    MathSciNet  MATH  Google Scholar 

  40. Pearson, J.E.: Complex patterns in a simple systems. Science 261, 189–192 (1993)

    Article  Google Scholar 

  41. Prothero, A., Robinson, A.: On the stability and accuracy of one-step methods for solving stiff systems of ordinary differential equations. Math. Comput. 28, 145–162 (1974)

    Article  MathSciNet  MATH  Google Scholar 

  42. Shampine, L.F., Sommeijer, B.P., Verwer, J.G.: IRKC: an IMEX solver for stiff diffusion–reaction PDEs. J. Comput. Appl. Math. 196, 485–497 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  43. Schnakenberg, J.: Simple chemical reaction systems with limiting cycle behaviour. J. Theor. Biol. 81, 389–400 (1979)

    Article  MathSciNet  Google Scholar 

  44. Shu, C.-W.: High order ENO and WENO schemes for computational fluid dynamics. In: Barth, T.J., Deconinck, H. (eds.) High-Order Methods for Computational Physics. Lecture Notes in Computational Science and Engineering, vol. 9, pp. 439–582. Springer, Berlin (1999)

    Google Scholar 

  45. Wang, R., Spiteri, R.J.: Linear instability of the fifth-order WENO method. SIAM J. Numer. Anal. 45, 1871–1901 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  46. Wright, W.: General linear methods with inherent Runge–Kutta stability, Ph.D. thesis, The University of Auckland, New Zealand (2002)

  47. Wright, W.: Explicit general linear methods with inherent Runge–Kutta stability. Numer. Algorithms 31, 381–399 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  48. Zeeman, E.C.: Differential Equations for the Heartbeat and Nerve Impulse. In: Waddington, C.H. (ed.) Towards a Theoretical Biology, vol. 4, pp. 4–67. Edinburgh University Press, Edinburgh (1972)

    Google Scholar 

  49. Zhang, H., Sandu, A., Blaise, S.: High order implicit–explicit general linear methods with optimized stability regions (2014), arXiv:1407.2337

  50. Zhang, H., Sandu, A., Blaise, S.: Partitioned and implicit–explicit general linear methods for ordinary differential equations. J. Sci. Comput. 61, 119–144 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  51. Zharovsky, E., Sandu, A., Zhang, H.: A class of implicit–explicit two-step Runge–Kutta methods. SIAM J. Numer. Anal. 53, 321–341 (2015)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

The research reported in this paper was started during the visit of the first author (MB) to the Arizona State University in November 2014. This author wish to express his gratitude to the School of Mathematical and Statistical Sciences for hospitality during this visit.

Author information

Authors and Affiliations

  1. Faculty of Applied Mathematics, AGH University of Science and Technology, Kraków, Poland

    Michał Braś & Zdzisław Jackiewicz

  2. Dipartimento di Matematica e Applicazioni, Università di Napoli-“Federico II”, 80126, Naples, Italy

    Giuseppe Izzo

  3. School of Mathematical and Statistical Sciences, Arizona State University, Tempe, AZ, 85287, USA

    Zdzisław Jackiewicz

Authors
  1. Michał Braś
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Giuseppe Izzo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Zdzisław Jackiewicz
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Giuseppe Izzo.

Additional information

The work of Michał Braś was supported by the National Science Center under Grant DEC-2011/01/N/ST1/02672 and the Polish Ministry of Science and Higher Education.

The work of Giuseppe Izzo was partially supported by GNCS-INdAM.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Braś, M., Izzo, G. & Jackiewicz, Z. Accurate Implicit–Explicit General Linear Methods with Inherent Runge–Kutta Stability. J Sci Comput 70, 1105–1143 (2017). https://doi.org/10.1007/s10915-016-0273-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-016-0273-y

Keywords

Search

Navigation