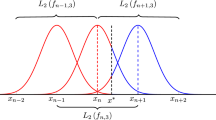
Abstract
In this paper, we develop the function, derivative and high-order derivatives recovery methods for the piecewise \(L^2\) projection and piecewise Lagrange interpolation. The presented recovery methods fully exploit the symmetry property to obtain the superconvergent recovered quantities. The analysis given here is based on Taylor expansion and to identify a symmetry sub-domain for superconvergence. Numerical examples are provided which demonstrate the superconvergence properties of the proposed recovery methods and its performance when applying to finite element method.
Similar content being viewed by others
Notes
The results of derivative recovery is the same as in [14], we present here for the reader’s convenience.
References
Agouzal, A., Vassilevski, Y.: On a discrete Hessian recovery for \(P^1\) finite elements. J. Numer. Math. 10, 1–12 (2002)
Bank, R.E., Xu, J.: Asymptotically exact a posteriori error estimators, part I: grids with superconvergence. SIAM J. Numer. Anal. 41, 2294–2312 (2003)
Bramble, J.H., Schatz, A.H.: Higher order local accuracy by averaging in the finite element method. Math. Comput. 31, 74–111 (1977)
Carstensen, C., Bartels, A.: Each averaging technique yields reliable a posteriori error control in FEM on unstructured grids. Part I: Low order conforming, nonconforming, and mixed FEM. Math. Comput. 71, 945–969 (2002)
Chen, C.M., Huang, Y.Q.: High Accuracy Theory of Finite Element Methods. Hunan Science Press, Hunan (1995). (in Chinese)
Chen, H., Guo, H., Zhang, Z., Zou, Q.: A \(C^0\) linear fintie element method for two fourth-order eigenvalue problems. IMA J. Numer. Anal. (2016). doi:10.1093/imanum/drw051
Chen, H., Zhang, Z., Zou, Q.: A recovery based linear finite element method for 1D bi-harmonic problems. J. Sci. Comput. 68, 375–394 (2016)
Gan, X., Akin, J.E.: Superconvergent second derivative recovery technieque and its application in a nonlocal damage mechanics model. Finite Elem. Anal. Des. 70, 27–35 (2013)
Guo, H., Zhang, Z., Zhao, R.: Hessian recovery for finite element methods. Math. Comput. 86, 1671–1692 (2017)
Guo, H., Zhang, Z., Zhao, R., Zou, Q.: Polynomial preserving recovery on boundary. J. Comput. Appl. Math. 307, 119–133 (2016)
Heimsund, B., Tai, X., Wang, J.: Superconvergence for the gradient of the finite element approximations by \(L^2\) projections. SIAM J. Numer. Anal. 40, 1538–1560 (2002)
Huang, Y.Q., Jiang, K., Yi, N.Y.: Some weighted averaging methods for gradient recovery. Adv. Appl. Math. Mech. 4, 131–155 (2014)
Huang, Y.Q., Liang, Q., Yi, N.Y.: High order compact schemes for gradient approximation. Sci. China Math. 53, 1903–1918 (2010)
Huang, Y.Q., Liu, H.L., Yi, N.Y.: Recovery of normal derivatives from the piecewise \(L^2\) projection. J. Comput. Phys. 231, 1230–1243 (2012)
Huang, Y.Q., Yang, W., Yi, N.Y.: Superconvergence analysis for the explicit polynomial recovery method. J. Comput. Appl. Math. 265, 187–198 (2014)
Huang, Y.Q., Yi, N.Y.: The superconvergent cluster recovery method. J. Sci. Comput. 44, 301–322 (2010)
Lakhany, A.M., Whiteman, J.R.: Superconvergent recovery operators: derivative recovery techniques. In: Krizek, M., Neittaanmaki, P., Stenberg, R. (eds.) Finite Element Methods: Superconvergence, Post-processing, and a Posteriori Estimates. Marcel Dekker INC, Newyork (1998)
Lamichhane, B.: A finite element method for a biharmonic equation based on gradient recovery operators. BIT Numer. Math. 54, 469–484 (2014)
Naga, A., Zhang, Z.: Function value recovery and its application in eigenvalue problems. SIAM J. Numer. Anal. 50, 272–286 (2012)
Ovall, J.: Function, gradient, and Hessian recovery using quadratic edge-bump functions. SIAM J. Numer. Anal. 45, 1064–1080 (2007)
Picasso, M., Alauzet, F., Borouchaki, H., George, P.: A numerical study of some Hessian recovery techniques on isotropic and anisotropic meshes. SIAM J. Sci. Comput. 33, 1058–1076 (2011)
van Leer, B., Nomura, S.: Discontinuous Galerkin for diffusion. In: Proceedings of 17th AIAA Computational Fluid Dynamics Conference, AIAA-2005-5108 (2005)
van Raalte, M., van Leer, B.: Bilinear forms for the recovery-based discontinuous Galerkin method for diffusion. Commun. Comput. Phys. 5, 683–693 (2009)
Vallet, M.G., Manole, C.M., Dompierre, J., Dufour, S., Guibault, F.: Numerical comparsion of some Hessian techniques. Int. J. Numer. Methods Eng. 72, 987–1007 (2007)
Yan, N.: Superconvergence Analysis and A Posteriori Error Estimation in Finite Element Methods. Science Press, Beijing (2008)
Zhang, Z., Naga, A.: A new finite element gradient recovery method: superconvergence property. SIAM J. Sci. Comput. 26, 1192–1213 (2005)
Zhu, J.Z., Zienkiewicz, O.C.: Superconvergence recovery technique and a posteriori error estimates. Int. J. Numer. Methods Eng. 30, 1321–1339 (1990)
Zienkiewicz, O.C., Zhu, J.Z.: The superconvergent patch recovery and a posteriori error estimates. Int. J. Numer. Methods Eng. 33, 1331–1382 (1992)
Acknowledgements
Yi’s research was partially supported by NSFC Project (11671341), Hunan Provincial NSF Project (2015JJ2145) and Hunan Education Department Project (16A206). Huang’s research was partially supported by NSFC Project (91430213).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Yi, N., Huang, Y. & Yang, W. Function, Derivative and High-Order Derivatives Recovery Methods Using the Local Symmetry Projection. J Sci Comput 74, 536–572 (2018). https://doi.org/10.1007/s10915-017-0451-6
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10915-017-0451-6