Skip to main content
SpringerLink
Log in

A High-Order Kernel-Free Boundary Integral Method for the Biharmonic Equation on Irregular Domains

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

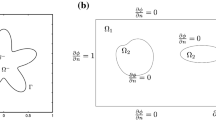
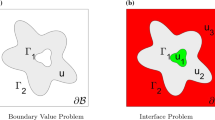
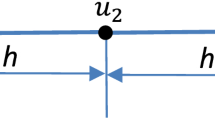
This work proposes second-order and fourth-order versions of a Cartesian grid based kernel-free boundary integral (KFBI) method for the biharmonic equation on both bounded irregular domains and singly periodic irregular domains. It is further development of the previous KFBI method for second-order elliptic PDEs. It reformulates boundary value problems of the fourth-order PDE as boundary integral equations of the first kind but the solution never needs to know the fundamental solution or Green’s function of the elliptic operator. Evaluation of boundary or volume integrals in the solution of boundary integral equations is made by solving equivalent interface problems on Cartesian grids with standard finite difference methods and fast Fourier transform based solvers. The work decomposes the biharmonic equation into two Poisson equations. It assumes the solution to one Poisson equation, which has no boundary conditions, as the sum of a volume integral with a double layer boundary integral, and applies Green’s third identity to derive a scalar boundary integral equation from the other Poisson equation that are subject to two boundary conditions. In the solution of the scalar boundary integral equation, each volume or boundary integral is evaluated with the KFBI method. Numerical examples are presented to demonstrate the solution accuracy and algorithm efficiency. A remarkable point of the work is that the nine-point compact difference scheme in dealing with each split second-order elliptic interface problem on irregular domains yields fourth-order accurate solution for the biharmonic equation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Adini, A., Clough, R.W.: Analysis of Plate Bending by the Finite Element Method. University of California, Berkeley (1960)

    Google Scholar 

  2. Arad, M., Yakhot, A., Ben-Dor, G.: A highly accurate numerical solution of a biharmonic equation. Numer. Methods Partial Differ. Equ. 13(4), 375–391 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  3. Argyris, J.H., Dunne, P.C.: The finite element method applied to fluid dynamics. In: Hewitt, B.L., Illingworth, C.R., Lock, R.C., Mangler, K.W., McDonnel, J.H., Richards, C., Walkden, F. (eds.) Computational Methods and Problems in Aeronautical Fluid Dynamics, pp. 158–197. Academic Press, London (1976)

    Google Scholar 

  4. Baker, G.A.: Finite element methods for elliptic equations using nonconforming elements. Math. Comput. 31, 45–59 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bhargava, R.: Solution of a biharmonic equation. Nature 201(4918), 530 (1964)

    Article  MATH  Google Scholar 

  6. Bialecki, B., Karageorghis, A.: Spectral chebyshev collocation for the Poisson and biharmonic equations. SIAM J. Sci. Comput. 32(5), 2995–3019 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bjørstad, P.: Fast numerical solution of the biharmonic Dirichlet problem on rectangles. SIAM J. Numer. Anal. 20(1), 59–71 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  8. Brebbia, C.A., Telles, J.C.F., Wrobel, L.C.: Boundary Element Techniques: Theory and Applications in Engineering. Springer, New York (2012)

    MATH  Google Scholar 

  9. Brenner, S., Sung, L.: C0 interior penalty methods for fourth order elliptic boundary value problems on polygonal domains. J. Sci. Comput. 22/23(1–3), 83–118 (2005)

    Article  MATH  Google Scholar 

  10. Brezzi, F., Fortin, M.: Mixed and Hybrid Finite Element Methods. Springer, Berlin (1991)

    Book  MATH  Google Scholar 

  11. Buzbee, B.L., Golub, G.H., Nielson, C.W.: On direct methods for solving Poisson’s equations. SIAM J. Numer. Anal. 7(4), 627–656 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  12. Camp, C.V.: Solution of the nonhomogeneous biharmonic equation by the boundary element method. Ph.D. thesis, Oklahoma State University (1987)

  13. Chan, R.H., DeLillo, T.K., Horn, M.A.: The numerical solution of the biharmonic equation by conformal mapping. SIAM J. Sci. Comput. 18(6), 1571–1582 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  14. Chen, G., Li, Z., Lin, P.: A fast finite difference method for biharmonic equations on irregular domains and its application to an incompressible Stokes flow. Adv. Comput. Math. 29(2), 113–133 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  15. Chen, J.T., Wu, C.S., Lee, Y.T., Chen, K.H.: On the equivalence of the Trefftz method and method of fundamental solutions for Laplace and biharmonic equations. Comput. Math. Appl. 53, 851–879 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  16. Cheng, X.L., Han, W., Huang, H.C.: Some mixed finite element methods for biharmonic equation. J. Comput. Appl. Math. 126, 91–109 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  17. Christiansen, S.: Integral equations without a unique solution can be made useful for solving some plane harmonic problems. IMA J. Appl. Math. 16(2), 143–159 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  18. Christiansen, S.: Derivation and analytical investigation of three direct boundary integral equations for the fundamental biharmonic problem. J. Comput. Appl. Math. 91(2), 231–247 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  19. Christiansen, S., Hougaard, P.: An investigation of a pair of integral equations for the biharmonic problem. IMA J. Appl. Math. 22(1), 15–27 (1978)

    Article  MathSciNet  MATH  Google Scholar 

  20. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. North-Holland, Amsterdam (1978)

    MATH  Google Scholar 

  21. Ciarlet, P.G., Raviart, P.A.: A mixed finite element method for the biharmonic equation. In: Mathematical Aspects of Finite Elements in Partial Differential Equations, pp. 125–145 (1974)

  22. Cockburn, B., Dong, B., Guzmán, J.: A hybridizable and superconvergent discontinuous Galerkin method for biharmonic problems. J. Sci. Comput. 40(1–3), 141–187 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  23. Collatz, L.: The Numerical Treatment of Differential Equations, vol. 60. Springer, New York (2012)

    Google Scholar 

  24. Conte, S.D., Dames, R.T.: An alternating direction method for solving the biharmonic equation. Math. Tables Other Aids Comput. 12(63), 198–205 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  25. Costabel, M., Lusikka, I., Saranen, J.: Comparison of three boundary element approaches for the solution of the clamped plate problem. In: Ciarlet, P.G., Lions, J.L. (eds.) Boundary Elements IX, vol. 2, pp. 19–34. Springer, Berlin (1987)

    Google Scholar 

  26. Costabel, M., Saranen, J.: Boundary element analysis of a direct method for the biharmonic Dirichlet problem. In: The Gohberg anniversary collection, pp. 569–587 (1989)

  27. Davini, C., Pitacco, I.: An unconstrained mixed method for the biharmonic problem. SIAM J. Numer. Anal. 38(3), 820–836 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  28. Ehrlich, L.W.: Solving the biharmonic equation as coupled finite difference equations. SIAM J. Numer. Anal. 8(2), 278–287 (1971)

    Article  MathSciNet  MATH  Google Scholar 

  29. Ehrlich, L.W., Gupta, M.M.: Some difference schemes for the biharmonic equation. SIAM J. Numer. Anal. 12(5), 773–790 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  30. Fairweather, G., Gourlay, A., Mitchell, A.: Some high accuracy difference schemes with a splitting operator for equations of parabolic and elliptic type. Numer. Math. 10(1), 56–66 (1967)

    Article  MathSciNet  MATH  Google Scholar 

  31. Fairweather, G., Karageorghis, A.: The method of fundamental solutions for elliptic boundary value problems. Adv. Comput. Math. 9(1–2), 69 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  32. Fornberg, B.: A pseudospectral approach for polar and spherical geometries. SIAM J. Sci. Comput. 16(5), 1071–1081 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  33. Fuglede, B.: On a direct method of integral equations for solving the biharmonic Dirichlet problem. Zeitschrift für Angewandte Mathematik und Mechanik 61(9), 449–459 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  34. Glowinski, R., Pironneau, O.: Numerical methods for the first biharmonic equation and for the two-dimensional Stokes problem. SIAM Rev. 21(2), 167–212 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  35. Greenbaum, A., Greengard, L., Mayo, A.: On the numerical solution of the biharmonic equation in the plane. Physica D 60, 216–225 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  36. Greengard, L., Kropinski, M.C.: An integral equation approach to the incompressible Navier–Stokes equations in two dimensions. SIAM J. Sci. Comput. 20(1), 318–336 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  37. Hadjidimos, A.: The numerical solution of a model problem biharmonic equation by using extrapolated alternating direction implicit methods. Numer. Math. 17(4), 301–317 (1971)

    Article  MathSciNet  MATH  Google Scholar 

  38. Heinrichs, W.: A stabilized treatment of the biharmonic operator with spectral methods. SIAM J. Sci. Stat. Comput. 12(5), 1162–1172 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  39. Hockney, R.W.: A fast direct solution of Poisson’s equation using fourier analysis. JACM 12(1), 95–113 (1965)

    Article  MathSciNet  MATH  Google Scholar 

  40. Hsiao, G.C., Wendland, W.L.: Boundary Integral Equations. Springer, New York (2008)

    Book  MATH  Google Scholar 

  41. Huang, S., Liu, Y.: A fast multipole boundary element method for solving the thin plate bending problem. Eng. Anal. Bound. Elem. 37(6), 967–976 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  42. Huang, W., Tang, T.: Pseudospectral solutions for steady motion of a viscous fluid inside a circular boundary. Appl. Numer. Math. 33(1–4), 167–173 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  43. Jaswon, M., Maiti, M.: An integral equation formulation of plate bending problems. J. Eng. Math. 2(1), 83–93 (1968)

    Article  MATH  Google Scholar 

  44. Jaswon, M.A., Symm, G.T.: Integral Equation Methods in Potential Theory and Elastostatics, vol. 132. Academic Press, London (1977)

    MATH  Google Scholar 

  45. Jeon, Y.: An indirect boundary integral equation method for the biharmonic equation. SIAM J. Numer. Anal. 31(2), 461–476 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  46. Jeon, Y.: New boundary element formulas for the biharmonic equation. Adv. Comput. Math. 9(1–2), 97–115 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  47. Jeon, Y.: New indirect scalar boundary integral equation formulas for the biharmonic equation. J. Comput. Appl. Math. 135(2), 313–324 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  48. Jeon, Y., McLean, W.: A new boundary element method for the biharmonic equation with Dirichlet boundary conditions. Adv. Comput. Math. 19(4), 339–354 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  49. Jiang, S., Ren, R., Tsuji, P., Ying, L.: Second kind integral equations for the first kind Dirichlet problem of the biharmonic equation in three dimensions. J. Comput. Phys. 230(19), 7488–7501 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  50. Jiang, Y., Wang, B., Xu, Y.: A fast Fourier–Galerkin method solving a boundary integral equation for the biharmonic equation. SIAM J. Numer. Anal. 52(5), 2530–2554 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  51. Karageorghis, A.: Modified methods of fundamental solutions for harmonic and biharmonic problems with boundary singularities. Numer. Methods Partial Differ. Equ. 8(1), 1–19 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  52. Karageorghis, A., Fairweather, G.: The method of fundamental solutions for the numerical solution of the biharmonic equation. J. Comput. Phys. 69(2), 434–459 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  53. Karageorghis, A., Fairweather, G.: The Almansi method of fundamental solutions for solving biharmonic problems. Int. J. Numer. Methods Eng. 26(7), 1665–1682 (1988)

    Article  MATH  Google Scholar 

  54. Karageorghis, A., Fairweather, G.: The simple layer potential method of fundamental solutions for certain biharmonic problems. Int. J. Numer. Methods Fluids 9(10), 1221–1234 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  55. Katsikadelis, J., Massalas, C., Tzivanidis, G.: An integral equation of the plane problem of the theory of elasticity. Mech. Res. Commun. 4(3), 199–208 (1977)

    Article  MATH  Google Scholar 

  56. Katsikadelis, J.T.: Boundary Elements: Theory and Applications. Elsevier, Amsterdam (2002)

    Google Scholar 

  57. Kupradze, V.D.: A method for the approximate solution of limiting problems in mathematical physics. USSR Comput. Math. Math. Phys. 4(6), 199–205 (1964)

    Article  MATH  MathSciNet  Google Scholar 

  58. Lai, M.C., Liu, H.C.: Fast direct solver for the biharmonic equation on a disk and its application to incompressible flows. Appl. Math. Comput. 164(3), 679–695 (2005)

    MathSciNet  MATH  Google Scholar 

  59. Lascaux, P., Lesaint, P.: Some nonconforming finite elements for the plate bending problem (Revue francaise d’automatique, informatique, recherche opérationnelle). Analyse numérique 9(R1), 9–53 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  60. Li, Z.: A fast iterative algorithm for elliptic interface problems. SIAM J. Numer. Anal. 35(1), 230–254 (1998). Please confirm the paper title for the reference [59]

    Article  MathSciNet  MATH  Google Scholar 

  61. Li, Z.C., Lee, M.G., Chiang, J.Y., Liu, Y.P.: The Trefftz method using fundamental solutions for biharmonic equations. J. Comput. Appl. Math. 235(15), 4350–4367 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  62. Maiti, M., Chakrabarty, S.: Integral equation solutions for simply supported polygonal plates. Int. J. Eng. Sci. 12(10), 793–806 (1974)

    Article  MATH  Google Scholar 

  63. Mayo, A.: The fast solution of Poisson’s and the biharmonic equations on irregular regions. SIAM J. Numer. Anal. 21(2), 285–299 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  64. Morley, L.S.D.: The triangular equilibrium element in the solution of plate bending problems. Aeronat. Q. 19, 149–169 (1968)

    Article  Google Scholar 

  65. Mu, L., Wang, J., Wang, Y., Ye, X.: A weak Galerkin mixed finite element method for biharmonic equations. In: Numerical Solution of Partial Differential Equations: Theory, Algorithms, and Their Applications, pp. 247–277. Springer, New York (2013)

  66. Mu, L., Wang, J., Ye, X.: Weak Galerkin finite element methods for the biharmonic equation on polytopal meshes. Numer. Methods Partial Differ. Equ. 30(3), 1003–1029 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  67. Poullikkas, A., Karageorghis, A., Georgiou, G.: Methods of fundamental solutions for harmonic and biharmonic boundary value problems. Comput. Mech. 21(4–5), 416–423 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  68. Rim, K., Henry, A.S.: An Integral Equation Method in Plane Elasticity, vol. 779. National Aeronautics and Space Administration, Washington (1967)

    Google Scholar 

  69. Roache, P.J.: Computational Fluid Dynamics. Hermosa Publishers, Albuquerque (1976)

    MATH  Google Scholar 

  70. Roberts, J., Thomas, J.M.: Mixed and hybrid methods. In: Ciarlet, P.G., Lions, J.L. (eds.) Handbook of Numerical Analysis, vol. 2. North-Holland, Amsterdam (1991)

    Google Scholar 

  71. Rosser, J.B.: Nine-point difference solutions for Poisson’s equation. Comput. Math. Appl. 1(3–4), 351–360 (1975)

    Article  MATH  Google Scholar 

  72. Saad, Y., Schultz, M.H.: GMRES: a generalized minimal residual algorithm for solving nonsymmetric linear systems. SIAM J. Sci. Stat. Comput. 7(3), 856–869 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  73. Sakakibara, K.: Method of fundamental solutions for biharmonic equation based on Almansi-type decomposition. Appl. Math. 62(4), 297–317 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  74. Samarskii, A.A.: The Theory of Difference Schemes, vol. 240. CRC Press, Boca Raton (2001)

    Book  MATH  Google Scholar 

  75. Sokolnikoff, I.S.: Mathematical Theory of Elasticity. McGraw-Hill Book Company, New York (1956)

    MATH  Google Scholar 

  76. Steinbach, O.: Numerical Approximation Methods for Elliptic Boundary Value Problems: Finite and Boundary Elements. Springer, New York (2007)

    Google Scholar 

  77. Stephenson, J.: Single cell discretizations of order two and four for biharmonic problems. J. Comput. Phys. 55(1), 65–80 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  78. Süli, E., Mozolevski, I.: hp-Version interior penalty DGFEMs for the biharmonic equation. Comput. Methods Appl. Mech. Eng. 196(13–16), 1851–1863 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  79. Timoshenko, S., Woinowsky-Krieger, S.: Theory of Plates and Shells, 2nd edn. McGraw-Hill, New York (1987)

    MATH  Google Scholar 

  80. Wong, Y.S., Jiang, H.: Approximate polynomial preconditioning applied to biharmonic equations. J. Supercomput. 3, 125–145 (1989)

    Article  MATH  Google Scholar 

  81. Xie, Y., Ying, W.: A fourth-order kernel-free boundary integral method for the modified Helmholtz equation. J. Sci. Comput. (2018). https://doi.org/10.1007/s10915-018-0821-8

    Article  MATH  Google Scholar 

  82. Ying, W.: A Cartesian grid-based boundary integral method for an elliptic interface problem on closely packed cells. Commun. Comput. Phys. 24(4), 1196–1220 (2018)

    Article  MathSciNet  Google Scholar 

  83. Ying, W., Henriquez, C.S.: A kernel-free boundary integral method for elliptic boundary value problems. J. Comput. Phys. 227(2), 1046–1074 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  84. Ying, W., Wang, W.C.: A kernel-free boundary integral method for implicitly defined surfaces. J. Comput. Phys. 252, 606–624 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  85. Ying, W., Wang, W.C.: A kernel-free boundary integral method for variable coefficients elliptic PDEs. Commun. Comput. Phys. 15(4), 1108–1140 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  86. Zhang, R., Zhai, Q.: A weak Galerkin finite element scheme for the biharmonic equations by using polynomials of reduced order. J. Sci. Comput. 64(2), 559–585 (2015)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Shanghai Jiao Tong University, Minhang, 200240, Shanghai, People’s Republic of China

    Yaning Xie

  2. School of Mathematical Sciences, MOE-LSC and Institute of Natural Sciences, Shanghai Jiao Tong University, Minhang, 200240, Shanghai, People’s Republic of China

    Wenjun Ying

  3. Department of Mathematics, National Tsing Hua University and National Center for Theoretical Sciences, Hsinchu, 300, Taiwan

    Wei-Cheng Wang

Authors
  1. Yaning Xie
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Wenjun Ying
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Wei-Cheng Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wenjun Ying.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Research of the second author is supported by the Science Challenge Project of China under Grant No. TZ2016002 and the National Natural Science Foundation of China under Grant DMS-11771290. Research of the second author was also supported by the Young Thousand Talents Program of China.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Xie, Y., Ying, W. & Wang, WC. A High-Order Kernel-Free Boundary Integral Method for the Biharmonic Equation on Irregular Domains. J Sci Comput 80, 1681–1699 (2019). https://doi.org/10.1007/s10915-019-01000-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-019-01000-6

Keywords

Search

Navigation