Skip to main content
SpringerLink
Log in

Convergence Analysis of the Localized Orthogonal Decomposition Method for the Semiclassical Schrödinger Equations with Multiscale Potentials

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

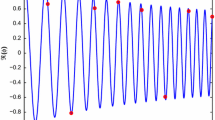
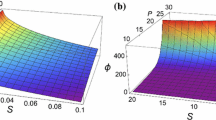
Abstract

We provide a convergence analysis of the localized orthogonal decomposition (LOD) method for Schrödinger equations with general multiscale potentials in the semiclassical regime. We focus on the influence of the semiclassical parameter and the multiscale structure of the potential on the numerical scheme. We construct localized multiscale basis functions by solving a constrained energy minimization problem in the framework of the LOD method. We show that localized multiscale basis functions with a smaller support can be constructed as the semiclassical parameter approaches zero. We obtain the first-order convergence in the energy norm and second-order convergence in the \(L^2\) norm for the LOD method and super convergence rates can be obtained if the solution possesses sufficiently high regularity. We analyse the temporal and spatial regularity of the solution and find that the spatial derivatives are more oscillatory than the time derivatives in the presence of a multiscale potential. By combining the regularity analysis, we are able to derive the dependence of the error estimates on the small parameters of the Schrödinger equation. Moreover, we find that the LOD method outperforms the finite element method in the presence of a multiscale potential due to the super convergence for high-regularity solutions and more relaxed dependence of the error estimates on the small parameters for low-regularity solutions. Finally, we present numerical results to demonstrate the accuracy and robustness of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Availability of data and materials

The data generated and analysed during the current study are available from the corresponding author on reasonable request.

Code Availability

Custom code was written in MATLAB.

References

  1. Abdulle, A., Henning, P.: Localized orthogonal decomposition method for the wave equation with a continuum of scales. Math. Comput. 86, 549–587 (2017)

  2. Altmann, R., Henning, P., Peterseim, D.: Quantitative Anderson localization of Schrödinger eigenstates under disorder potentials. Math. Models Methods Appl. Sci. 30, 917–955 (2020)

    MathSciNet  MATH  Google Scholar 

  3. Altmann, R., Henning, P., Peterseim, D.: Numerical homogenization beyond scale separation. Acta Numer. 30, 1–86 (2021)

    MathSciNet  Google Scholar 

  4. Altmann, R., Peterseim, D.: Localized computation of eigenstates of random Schrödinger operators. SIAM J. Sci. Comput. 41, B1211–B1227 (2019)

    MATH  Google Scholar 

  5. Babuska, I., Lipton, R.: Optimal local approximation spaces for generalized finite element methods with application to multiscale problems. Multiscale Model. Simul. 9, 373–406 (2011)

    MathSciNet  MATH  Google Scholar 

  6. Bao, W., Jin, S., Markowich, P.A.: On time-splitting spectral approximations for the Schrödinger equation in the semiclassical regime. J. Comput. Phys. 175, 487–524 (2002)

    MathSciNet  MATH  Google Scholar 

  7. Bebendorf, M.: Hierarchical Matrices. Springer (2008)

  8. Brenner, S., Scott, R.: The Mathematical Theory of Finite Element Methods, vol. 15. Springer (2007)

  9. Brezzi, F., Franca, L., Hughes, T., Russo, A.: \(b = \int {G}\). Comput. Methods Appl. Mech. Eng. 145, 329–339 (1997)

    MATH  Google Scholar 

  10. Carstensen, C., Verfürth, R.: Edge residuals dominate a posteriori error estimates for low order finite element methods. SIAM J. Numer. Anal. 36, 1571–1587 (1999)

    MathSciNet  MATH  Google Scholar 

  11. Chen, J., Li, S., Zhang, Z.: Efficient multiscale methods for the semiclassical Schrödinger equation with time-dependent potentials. Comput. Methods Appl. Mech. Engrg. 369, 113232 (2020)

    MathSciNet  MATH  Google Scholar 

  12. Chen, J., Ma, D., Zhang, Z.: A multiscale finite element method for the Schrödinger equation with multiscale potentials. SIAM J. Sci. Comput. 41, B1115–B1136 (2019)

    MATH  Google Scholar 

  13. Chen, J., Ma, D., Zhang, Z.: A multiscale reduced basis method for the Schrödinger equation with multiscale and random potentials. Multiscale Model. Simul. 18, 1409–1434 (2020)

    MathSciNet  MATH  Google Scholar 

  14. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. SIAM (2002)

  15. Clément, P.: Approximation by finite element functions using local regularization. RAIRO Anal. Numér. 9, 77–84 (1975)

    MathSciNet  MATH  Google Scholar 

  16. Delgadillo, R., Lu, J., Yang, X.: Gauge-invariant frozen Gaussian approximation method for the Schrödinger equation with periodic potentials. SIAM J. Sci. Comput. 38, A2440–A2463 (2016)

    MATH  Google Scholar 

  17. Dörfler, W.: A time- and space-adaptive algorithm for the linear time-dependent Schrödinger equation. Numer. Math. 73, 419–448 (1996)

    MathSciNet  MATH  Google Scholar 

  18. Weinan, E., Engquist, B.: The heterogeneous multiscale methods. Commun. Math. Sci. 1, 87–133 (2003)

    MathSciNet  MATH  Google Scholar 

  19. Efendiev, Y., Galvis, J., Hou, T.Y.: Generalized multiscale finite element methods (GMSFEM). J. Comput. Phys. 251, 116–135 (2013)

    MathSciNet  MATH  Google Scholar 

  20. Evans, L.: Partial Differential Equations, vol. 19. American Mathematical Society (1998)

  21. Hackbusch, W.: Hierarchical Matrices: Algorithms and Analysis, vol. 49. Springer (2015)

  22. Hackbusch, W.: Elliptic Differential Equations: Theory and Numerical Treatment, vol. 18. Springer (2017)

  23. Han, H., Zhang, Z.: Multiscale tailored finite point method for second order elliptic equations with rough or highly oscillatory coefficients. Commun. Math. Sci. 10, 945–976 (2012)

    MathSciNet  MATH  Google Scholar 

  24. Henning, P., Målqvist, A.: Localized orthogonal decomposition techniques for boundary value problems. SIAM J. Sci. Comput. 36, A1609–A1634 (2014)

    MathSciNet  MATH  Google Scholar 

  25. Henning, P., Målqvist, A., Peterseim, D.: Two-level discretization techniques for ground state computations of Bose–Einstein condensates. SIAM J. Numer. Anal. 52, 1525–1550 (2014)

    MathSciNet  MATH  Google Scholar 

  26. Henning, P., Morgenstern, P., Peterseim, D.: Multiscale partition of unity. In: Meshfree Methods for Partial Differential Equations, vol. VII, pp. 185–204. Springer (2015)

  27. Henning, P., Peterseim, D.: Oversampling for the multiscale finite element method. Multiscale Model. Simul. 11, 1149–1175 (2013)

    MathSciNet  MATH  Google Scholar 

  28. Henning, P., Wärnegård, J.: Superconvergence of time invariants for the Gross–Pitaevskii equation. Math. Comput. 91, 509–555 (2022)

    MathSciNet  MATH  Google Scholar 

  29. Hoang, V.H., Schwab, C.: High-dimensional finite elements for elliptic problems with multiple scales. Multiscale Model. Simul. 3, 168–194 (2005)

    MathSciNet  MATH  Google Scholar 

  30. Hou, T.Y., Wu, X., Cai, Z.: Convergence of a multiscale finite element method for elliptic problems with rapidly oscillating coefficients. Math. Comput. 68, 913–943 (1999)

    MathSciNet  MATH  Google Scholar 

  31. Hou, T.Y., Wu, X.-H.: A multiscale finite element method for elliptic problems in composite materials and porous media. J. Comput. Phys. 134, 169–189 (1997)

    MathSciNet  MATH  Google Scholar 

  32. Hou, T.Y., Zhang, P.: Sparse operator compression of higher-order elliptic operators with rough coefficients. Res. Math. Sci. 4, 1–49 (2017)

    MathSciNet  Google Scholar 

  33. Huang, Z., Jin, S., Markowich, P., Sparber, C.: A Bloch decomposition-based split-step pseudospectral method for quantum dynamics with periodic potentials. SIAM J. Sci. Comput. 29, 515–538 (2007)

    MathSciNet  MATH  Google Scholar 

  34. Huang, Z., Jin, S., Markowich, P., Sparber, C.: Numerical simulation of the nonlinear Schrödinger equation with multidimensional periodic potentials. Multiscale Model. Simul. 7, 539–564 (2008)

    MathSciNet  MATH  Google Scholar 

  35. Hughes, T.J.: Multiscale phenomena: Green’s functions, the Dirichlet-to-Neumann formulation, subgrid scale models, bubbles and the origins of stabilized methods. Comput. Methods Appl. Mech. Eng. 127, 387–401 (1995)

    MathSciNet  MATH  Google Scholar 

  36. Hughes, T.J., Feijoo, G.R., Mazzei, L., Quincy, J.B.: The variational multiscale method-a paradigm for computational mechanics. Comput. Methods Appl. Mech. Eng. 166, 3–24 (1998)

    MathSciNet  MATH  Google Scholar 

  37. Hughes, T.J., Sangalli, G.: Variational multiscale analysis: the fine-scale Green’s function, projection, optimization, localization, and stabilized methods. SIAM J. Numer. Anal. 45, 539–557 (2007)

    MathSciNet  MATH  Google Scholar 

  38. Jin, S., Markowich, P., Sparber, C.: Mathematical and computational methods for semiclassical Schrödinger equation. Acta Numer. 20, 121–209 (2011)

    MathSciNet  MATH  Google Scholar 

  39. Jin, S., Wu, H., Yang, X.: Gaussian beam methods for the Schrödinger equation in the semi-classical regime: Lagrangian and Eulerian formulations. Commun. Math. Sci. 6, 995–1020 (2008)

    MathSciNet  MATH  Google Scholar 

  40. Jin, S., Wu, H., Yang, X., Huang, Z.: Bloch decomposition-based Gaussian beam method for the Schrödinger equation with periodic potentials. J. Comput. Phys. 229, 4869–4883 (2010)

    MathSciNet  MATH  Google Scholar 

  41. Li, S., Zhang, Z.: Computing eigenvalues and eigenfunctions of Schrödinger equations using a model reduction approach. Commun. Comput. Phys. 24, 1073–1100 (2018)

    MathSciNet  MATH  Google Scholar 

  42. Louwen, A., van Sark, W., Schropp, R., Faaij, A.: A cost roadmap for silicon heterojunction solar cells. Sol. Energy Mater. Sol. Cells 147, 295–314 (2016)

    Google Scholar 

  43. Maier, R.: Computational multiscale methods in unstructured heterogeneous media. Ph.D. Thesis, University of Augsburg (2020)

  44. Maier, R.: A high-order approach to elliptic multiscale problems with general unstructured coefficients. SIAM J. Numer. Anal. 59, 1067–1089 (2021)

    MathSciNet  MATH  Google Scholar 

  45. Målqvist, A.: Multiscale methods for elliptic problems. Multiscale Model. Simul. 9, 1064–1086 (2011)

    MathSciNet  MATH  Google Scholar 

  46. Målqvist, A., Persson, A.: Multiscale techniques for parabolic equations. Numer. Math. 138, 191–217 (2018)

    MathSciNet  MATH  Google Scholar 

  47. Målqvist, A., Peterseim, D.: Localization of elliptic multiscale problems. Math. Comput. 83, 2583–2603 (2014)

    MathSciNet  MATH  Google Scholar 

  48. Målqvist, A., Peterseim, D.: Computation of eigenvalues by numerical upscaling. Numer. Math. 130, 337–361 (2015)

    MathSciNet  MATH  Google Scholar 

  49. Målqvist, A., Peterseim, D.: Numerical Homogenization by Localized Orthogonal Decomposition. SIAM (2020)

  50. Markowich, P.A., Pietra, P., Pohl, C.: Numerical approximation of quadratic observables of Schrödinger-type equations in the semi-classical limit. Numer. Math. 81, 595–630 (1999)

    MathSciNet  MATH  Google Scholar 

  51. Markowich, P.A., Pietra, P., Pohl, C., Stimming, H.P.: A Wigner-measure analysis of the Dufort–Frankel scheme for the Schrödinger equation. SIAM J. Numer. Anal. 40, 1281–1310 (2002)

    MathSciNet  MATH  Google Scholar 

  52. Miller, P.D.: Applied Asymptotic Analysis, vol. 75. American Mathematical Society (2006)

  53. Murray, J.D.: Asymptotic Analysis, vol. 48. Springer (2012)

  54. Owhadi, H.: Bayesian numerical homogenization. Multiscale Model. Simul. 13, 812–828 (2015)

    MathSciNet  MATH  Google Scholar 

  55. Owhadi, H.: Multigrid with rough coefficients and multiresolution operator decomposition from hierarchical information games. SIAM Rev. 59, 99–149 (2017)

    MathSciNet  MATH  Google Scholar 

  56. Owhadi, H., Zhang, L.: Gamblets for opening the complexity-bottleneck of implicit schemes for hyperbolic and parabolic odes/pdes with rough coefficients. J. Comput. Phys. 347, 99–128 (2017)

    MathSciNet  MATH  Google Scholar 

  57. Peterseim, D.: Variational multiscale stabilization and the exponential decay of fine-scale correctors. In: Building Bridges: Connections and Challenges in Modern Approaches to Numerical Partial Differential Equations, vol. 114 of Lect. Notes Comput. Sci. Eng., pp. 343–369. Springer (2016)

  58. Peterseim, D.: Eliminating the pollution effect in Helmholtz problems by local subscale correction. Math. Comput. 86, 1005–1036 (2017)

    MathSciNet  MATH  Google Scholar 

  59. Peterseim, D., Verfürth, B.: Computational high frequency scattering from high-contrast heterogeneous media. Math. Comput. 89, 2649–2674 (2020)

    MathSciNet  MATH  Google Scholar 

  60. Qian, J., Ying, L.: Fast Gaussian wavepacket transforms and Gaussian beams for the Schrödinger equation. J. Comput. Phys. 229, 7848–7873 (2010)

    MathSciNet  MATH  Google Scholar 

  61. Quach, J.Q., Su, C.-H., Martin, A.M., Greentree, A.D., Hollenberg, L.C.L.: Reconfigurable quantum metamaterials. Opt. Express 19, 11018–11033 (2011)

    Google Scholar 

  62. Scott, L.R., Zhang, S.: Finite element interpolation of nonsmooth functions satisfying boundary conditions. Math. Comput. 54, 483–493 (1990)

    MathSciNet  MATH  Google Scholar 

  63. Tao, T.: Nonlinear Dispersive Equations: Local and Global Analysis. American Mathematical Society (2006)

  64. Thomée, V.: Galerkin Finite Element Methods for Parabolic Problems, vol. 25. Springer (2007)

  65. Wu, Z., Huang, Z.: A Bloch decomposition-based stochastic Galerkin method for quantum dynamics with a random external potential. J. Comput. Phys. 317, 257–275 (2016)

    MathSciNet  MATH  Google Scholar 

  66. Xie, H., Zhang, L., Owhadi, H.: Fast eigenpairs computation with operator adapted wavelets and hierarchical subspace correction. SIAM J. Numer. Anal. 57, 2519–2550 (2019)

    MathSciNet  MATH  Google Scholar 

  67. Yin, D., Zheng, C.: Gaussian beam formulations and interface conditions for the one-dimensional linear Schrödinger equation. Wave Motion 48, 310–324 (2011)

    MathSciNet  MATH  Google Scholar 

  68. Žutić, I., Fabian, J., Sarma, S.D.: Spintronics: Fundamentals and applications. Rev. Mod. Phys. 76, 323–410 (2004)

    Google Scholar 

Download references

Acknowledgements

The research of Z. Zhang is supported by Hong Kong RGC grants (Projects 17300817, 17300318, and 17307921), National Natural Science Foundation of China (Project 12171406), Seed Funding Programme for Basic Research (HKU), and a seed funding from the HKU-TCL Joint Research Center for Artificial Intelligence. The computations were performed using research computing facilities offered by Information Technology Services, the University of Hong Kong.

Funding

The funding was provided by Hong Kong RGC grants (Projects 17300817, 17300318, and 17307921), National Natural Science Foundation of China (Project 12171406), Seed Funding Programme for Basic Research (HKU), and a seed funding from the HKU-TCL Joint Research Center for Artificial Intelligence. The computations were performed using research computing facilities offered by Information Technology Services, the University of Hong Kong.

Author information

Authors and Affiliations

  1. Department of Mathematics, The University of Hong Kong, Pokfulam Road, Hong Kong SAR, People’s Republic of China

    Zhizhang Wu & Zhiwen Zhang

Authors
  1. Zhizhang Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhiwen Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhiwen Zhang.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, Z., Zhang, Z. Convergence Analysis of the Localized Orthogonal Decomposition Method for the Semiclassical Schrödinger Equations with Multiscale Potentials. J Sci Comput 93, 73 (2022). https://doi.org/10.1007/s10915-022-02038-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-02038-9

Keywords

Mathematics Subject Classification

Search

Navigation