Skip to main content

Advertisement

SpringerLink
Log in

An Adaptive Time-Stepping Method for the Binary Fluid-Surfactant Phase Field Model on Evolving Surfaces

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

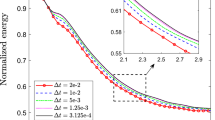
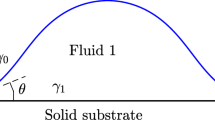
Abstract

In this paper, the binary fluid-surfactant phase field model on evolving surfaces is presented and numerically studied for interfacial sciences. Based on the evolving surface finite element method, the first-order and second-order standard semi-implicit schemes are shown and analyzed to be conditionally stable. The energy dissipation law of the binary fluid-surfactant phase field model does not hold for evolving surfaces with arbitrary surface velocities which can be viewed as an action of external force. Therefore, this paper is dedicated to addressing the difficulties of conditional stability and numerical stiffness caused by the small interfacial parameters instead of energy stability. The first-order and second-order stabilizing approaches are employed to improve the stabilities of semi-implicit schemes. For the efficiency of long time simulation, an adaptive time-stepping technique considering energy evolution and surface velocity is developed. The effectiveness of the proposed stabilizing and adaptive time-stepping approaches is verified by numerical experiments. Various numerical simulations are performed to demonstrate the effectiveness and efficiency of the proposed method, and numerically investigate the behavior of the binary fluid-surfactant on evolving surfaces.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20

Similar content being viewed by others

Data availability

No data was used for the research described in the article. The codes generated during the study are all available on request from the corresponding author Xufeng Xiao.

References

  1. Barreira, R., Elliott, C.M., Madzvamuse, A.: The surface finite element method for pattern formation on evolving biological surfaces. J. Math. Biol. 63(6), 1095–1119 (2011)

    MathSciNet  MATH  Google Scholar 

  2. Dziuk, G., Elliott, C.M.: A fully discrete evolving surface finite element method. SIAM J. Numer. Anal. 50(5), 2677–2694 (2012)

    MathSciNet  MATH  Google Scholar 

  3. Dziuk, G., Elliott, C.M.: Finite element methods for surface PDEs. Acta Numer. 22, 289–396 (2013)

    MathSciNet  MATH  Google Scholar 

  4. Dziuk, G., Elliott, C.M.: Finite elements on evolving surfaces. IMA J. Numer. Anal. 27(2), 262–292 (2007)

    MathSciNet  MATH  Google Scholar 

  5. Dziuk, G., Lubich, C., Mansour, D.: Runge–Kutta time discretization of parabolic differential equations on evolving surfaces. IMA J. Numer. Anal. 32(2), 394–416 (2012)

    MathSciNet  MATH  Google Scholar 

  6. Elliott, C.M., Ranner, T.: Evolving surface finite element method for the Cahn–Hilliard equation. Numer. Math. 129(3), 483–534 (2015)

    MathSciNet  MATH  Google Scholar 

  7. Eggleton, C.D., Tsai, T.M., Stebe, K.J.: Tip streaming from a drop in the presence of surfactants. Phys. Rev. Lett. 87(4), 048302 (2001)

    Google Scholar 

  8. Fonseca, I., Morini, M., Slastikov, V.: Surfactants in foam stability: a phase-field model. Arch. Ration. Mech. Anal. 183(3), 411–456 (2007)

    MathSciNet  MATH  Google Scholar 

  9. Feng, X., Tang, T., Yang, J.: Stabilized Crank–Nicolson/Adams–Bashforth schemes for phase field models. East Asian J. Appl. Math. 3(1), 59–80 (2013)

    MathSciNet  MATH  Google Scholar 

  10. Gompper, G.: Self-assembling amphiphilic systems. Phase Transit Crit Phenomena 16 (1994)

  11. Gu, S., Zhang, H., Zhang, Z.: An energy-stable finite-difference scheme for the binary fluid-surfactant system. J. Comput. Phys. 270, 416–431 (2014)

    MathSciNet  MATH  Google Scholar 

  12. Huang, S., Xiao, X., Feng, X.: An adaptive time-stepping method for the phase-field molecular beam epitaxial growth model on evolving surfaces. Appl. Math. Comput. 439, 127622 (2023)

    MathSciNet  Google Scholar 

  13. Hu, Y.T., Pine, D.J., Leal, L.G.: Drop deformation, breakup, and coalescence with compatibilizer. Phys. Fluids 12(3), 484–489 (2000)

    MATH  Google Scholar 

  14. Hou, D., Qiao, Z.: A linear adaptive BDF2 scheme for phase field crystal equation. ArXiv Preprint arXiv:2206.07625 (2022)

  15. Hansbo, P., Larson, M.G., Zahedi, S.: Characteristic cut finite element methods for convection-diffusion problems on time dependent surfaces. Comput. Methods Appl. Mech. Eng. 293, 431–461 (2015)

    MathSciNet  MATH  Google Scholar 

  16. Hartmann, E.: A marching method for the triangulation of surfaces. Vis. Comput. 14(3), 95–108 (1998)

    MATH  Google Scholar 

  17. He, Y.: A fully discrete stabilized finite-element method for the time-dependent Navier–Stokes problem. IMA J. Numer. Anal. 23(4), 665–691 (2003)

    MathSciNet  MATH  Google Scholar 

  18. Komura, S., Kodama, H.: Two-order-parameter model for an oil-water-surfactant system. Phys. Rev. E 55(2), 1722 (1997)

    Google Scholar 

  19. Liao, H.L., Ji, B., Zhang, L.: An adaptive BDF2 implicit time-stepping method for the phase field crystal model. IMA J. Numer. Anal. 42(1), 649–679 (2022)

    MathSciNet  MATH  Google Scholar 

  20. Liao, H.L., Ji, B., Wang, L., Zhang, Z.: Mesh-robustness of an energy stable BDF2 scheme with variable steps for the Cahn–Hilliard model. J. Sci. Comput. 92(2), 1–26 (2022)

    MathSciNet  MATH  Google Scholar 

  21. Lehrenfeld, C., Olshanskii, M.A., Xu, X.: A stabilized trace finite element method for partial differential equations on evolving surfaces. SIAM J. Numer. Anal. 56(3), 1643–1672 (2018)

    MathSciNet  MATH  Google Scholar 

  22. Lehto, E., Shankar, V., Wright, G.B.: A radial basis function (RBF) compact finite difference (FD) scheme for reaction-diffusion equations on surfaces. SIAM J. Sci. Comput. 39(5), A2129–A2151 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Laradji, M., Guo, H., Grant, M., Zuckermann, M.J.: The effect of surfactants on the dynamics of phase separation. J. Phys. Condens. Matter 4(32), 6715 (1992)

    Google Scholar 

  24. Li, Y., Choi, Y., Kim, J.: Computationally efficient adaptive time step method for the Cahn–Hilliard equation. Comput. Math. Appl. 73(8), 1855–1864 (2017)

    MathSciNet  MATH  Google Scholar 

  25. Macdonald, C.B., Ruuth, S.J.: Level set equations on surfaces via the closest point method. J. Sci. Comput. 35(2), 219–240 (2008)

    MathSciNet  MATH  Google Scholar 

  26. Macdonald, C.B., Ruuth, S.J.: The implicit closest point method for the numerical solution of partial differential equations on surfaces. SIAM J. Sci. Comput. 31(6), 4330–4350 (2010)

    MathSciNet  MATH  Google Scholar 

  27. O’Connor, D.: Phase field models on evolving surfaces. University of Warwick (2016)

  28. Olshanskii, M.A., Reusken, A.: Trace finite element methods for PDEs on surfaces. Geometrically Unfitted Finite Element Methods and Applications, pp. 211–258 (2017)

  29. Olshanskii, M.A., Xu, X.: A trace finite element method for PDEs on evolving surfaces. SIAM J. Sci. Comput. 39(4), A1301–A1319 (2017)

    MathSciNet  MATH  Google Scholar 

  30. Persson, P.O., Strang, G.: A simple mesh generator in MATLAB. SIAM Rev. 46(2), 329–345 (2004)

    MathSciNet  MATH  Google Scholar 

  31. Petras, A., Ling, L., Piret, C., Ruuth, S.J.: A least-squares implicit RBF-FD closest point method and applications to PDEs on moving surfaces. J. Comput. Phys. 381, 146–161 (2019)

    MathSciNet  MATH  Google Scholar 

  32. Petras, A., Ling, L., Ruuth, S.J.: An RBF-FD closest point method for solving PDEs on surfaces. J. Comput. Phys. 370, 43–57 (2018)

    MathSciNet  MATH  Google Scholar 

  33. Petras, A., Ruuth, S.J.: PDEs on moving surfaces via the closest point method and a modified grid based particle method. J. Comput. Phys. 312, 139–156 (2016)

    MathSciNet  MATH  Google Scholar 

  34. Ruuth, S.J., Merriman, B.: A simple embedding method for solving partial differential equations on surfaces. J. Comput. Phys. 227(3), 1943–1961 (2008)

    MathSciNet  MATH  Google Scholar 

  35. Shankar, V., Wright, G.B., Kirby, R.M., Fogelson, A.L.: A radial basis function (RBF)-finite difference (FD) method for diffusion and reaction-diffusion equations on surfaces. J. Sci. Comput. 63(3), 745–768 (2015)

    MathSciNet  MATH  Google Scholar 

  36. Shen, J.: Long time stability and convergence for fully discrete nonlinear Galerkin methods. Appl. Anal. 38(4), 201–229 (1990)

    MathSciNet  MATH  Google Scholar 

  37. Sun, M., Feng, X., Wang, K.: Numerical simulation of binary fluid-surfactant phase field model coupled with geometric curvature on the curved surface. Comput. Methods Appl. Mech. Eng. 367, 113123 (2020)

    MathSciNet  MATH  Google Scholar 

  38. Sun, H., Zhao, X., Cao, H., Yang, R., Zhang, M.: Stability and convergence analysis of adaptive BDF2 scheme for the Swift-Hohenberg equation. Commun. Nonlinear Sci. Numer. Simul. 111, 106412 (2022)

    MathSciNet  MATH  Google Scholar 

  39. Teng, C.H., Chern, I.L., Lai, M.C.: Simulating binary fluid-surfactant dynamics by a phase field model. Discrete Contin. Dyn. Syst.-B 17(4), 1289 (2012)

    MathSciNet  MATH  Google Scholar 

  40. Teramoto, T., Yonezawa, F.: Droplet growth dynamics in a water/oil/surfactant system. J. Colloid Interface Sci. 235, 329–333 (2001)

    Google Scholar 

  41. Van der Sman, R.G.M., Van der Graaf, S.: Diffuse interface model of surfactant adsorption onto flat and droplet interfaces. Rheol. Acta 46, 3–11 (2006)

    Google Scholar 

  42. Yang, X., Ju, L.: Linear and unconditionally energy stable schemes for the binary fluid-surfactant phase field model. Comput. Methods Appl. Mech. Eng. 318, 1005–1029 (2017)

    MathSciNet  MATH  Google Scholar 

  43. Yang, X.: Numerical approximations for the Cahn–Hilliard phase field model of the binary fluid-surfactant system. J. Sci. Comput. 74(3), 1533–1553 (2018)

    MathSciNet  MATH  Google Scholar 

  44. Yang, X.: On a novel fully decoupled, second-order accurate energy stable numerical scheme for a binary fluid-surfactant phase-field model. SIAM J. Sci. Comput. 43(2), B479–B507 (2021)

    MathSciNet  MATH  Google Scholar 

  45. Zhu, G., Kou, J., Sun, S., Yao, J., Li, A.: Decoupled, energy stable schemes for a phase-field surfactant model. Comput. Phys. Commun. 233, 67–77 (2018)

    MathSciNet  Google Scholar 

  46. Zhang, J., Yang, X.: Non-iterative, unconditionally energy stable and large time-stepping method for the Cahn–Hilliard phase-field model with Flory-Huggins-de Gennes free energy. Adv. Comput. Math. 46(3), 1–27 (2020)

    MathSciNet  MATH  Google Scholar 

  47. Zhang, Z., Qiao, Z.: An adaptive time-stepping strategy for the Cahn–Hilliard equation. Commun. Comput. Phys. 11(4), 1261–1278 (2012)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the editors and referees for their valuable comments and suggestions which helped us to improve the results of this paper.

Funding

The authors have not disclosed any funding.

Author information

Authors and Affiliations

  1. College of Mathematics and System Sciences, Xinjiang University, Urumqi, 830046, People’s Republic of China

    Shijie Huang, Xufeng Xiao & Xinlong Feng

Authors
  1. Shijie Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xufeng Xiao
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xinlong Feng
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xufeng Xiao.

Ethics declarations

Conflict of interest

No conflict of interest exists in the submission of this manuscript, and the manuscript is approved by all authors for publication.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This work is supported by the NSF of China (No. 12001466, No. U19A2079 and No. 11671345), the scientific research plan of universities in Xinjiang (No. XJEDU2020Y001 and No. XJEDU2020I001), and the Research Fund from Key Laboratory of Xinjiang Province (No. 2020D04002).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Huang, S., Xiao, X. & Feng, X. An Adaptive Time-Stepping Method for the Binary Fluid-Surfactant Phase Field Model on Evolving Surfaces. J Sci Comput 95, 29 (2023). https://doi.org/10.1007/s10915-023-02150-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-023-02150-4

Keywords

Search

Navigation