Variational Formulation of a General Equilibrium Model with Incomplete Financial Markets and Numeraire Assets: Existence

Abstract

We present a general equilibrium model with incomplete financial markets and numeraire assets. We assume that there are 2 periods of time, say today and tomorrow. In period 0, households exchange goods and assets and then consumption takes place; in period 1, one of S possible states of nature occurs. In each of them, assets pay their returns, which are measured in units of a given physical good, i.e., the numeraire commodity; households exchange goods; finally, consumption takes place. We define a consumption, portfolio holding, commodity and asset price vector as an equilibrium vector associated with a given economy, if at those prices and economies households maximize, and market clears. While the existence proof by Geneakoplos and Polemarchakis (Essays in honor of K.J. Arrow, vol 3, Cambridge University Press, Cambridge, pp 65–95, 1986) uses a fixed point argument, we provide an independent existence result in terms of variational inequalities. That approach allows us to get the desired existence result under some different and more general or realistic assumptions than those usually made in the literature.

Appendices

Appendices

Appendix A: Example

We present an example which describes equilibria in an economy which satisfies all assumptions we made in Sect. 2.²

We consider a simple economy with two households, two states in period 1, one good per spot and one asset with yield 1 in each spot in period 1. Households’ utility functions are of the so-called Cobb–Douglas type, i.e., weighted sum of logarithms. We also consider no-arbitrage good and asset prices, i.e., $p^{0}+q=p^{0}+\nu ^{1}+\nu ^{2}=S+1=3$ with $p^{0},\nu ^{1},\nu ^{2}>0$. Then, household h’s maximization problem is the following one.

$$\begin{aligned} \begin{array}{lll} &{} \max _{\left( x_{h}^{0},x_{h}^{1},x_{h}^{2},b_{h}\right) }\alpha _{h}^{0}\log x_{h}^{0}+\alpha _{h}^{1}\log x_{h}^{1}+\alpha _{h}^{2}\log x_{h}^{2}\\ \text {s.t.} &{} -p^{0}\left( x_{h}^{0}-e_{h}^{0}\right) -qb_{h}\le 0 \\ &{} \left( x_{h}^{1}-e_{h}^{1}\right) +b_{h}\le 0 \\ &{} -\left( x_{h}^{2}-e_{h}^{2}\right) +b_{h}\le 0, \end{array} \end{aligned}$$

where $\left( \alpha _{h}^{0},\alpha _{h}^{1},\alpha _{h}^{2}\right) \in {\mathbb {R}}_{++}^{3}$. Observe that since utility functions are strictly increasing in each good, the solution set to the above problem is equal to the solution set to the problem with equality constraints. Denoted the Lagrange multiplier of household h in state s by $\lambda _{h}^{s}$, for $h\in \left\{ 1,2\right\}$ and $s\in \left\{ 0,1,2\right\}$, we have that the associated Lagrange conditions are

$$\begin{aligned} \left\{ \begin{array}{l} \frac{\alpha _{h}^{0}}{x_{h}^{0}}-\lambda _{h}^{0}p^{0} =0 \\ \frac{\alpha _{h}^{1}}{x_{h}^{1}}-\lambda _{h}^{1} =0 \\ \frac{\alpha _{h}^{2}}{x_{h}^{2}}-\lambda _{h}^{2} =0 \\ -\lambda _{h}^{0}q+\lambda _{h}^{1}+\lambda _{h}^{2} =0 \\ -p^{0}\left( x_{h}^{0}-e_{h}^{0}\right) -qb_{h} =0 \\ -\left( x_{h}^{1}-e_{h}^{1}\right) +b_{h} =0 \\ -\left( x_{h}^{2}-e_{h}^{2}\right) +b_{h} =0 \end{array}\right. \qquad \text {or} \qquad \left\{ \begin{array}{l} x_{h}^{0} =\frac{\alpha _{h}^{0}}{\lambda _{h}^{0}p^{0}}=\frac{ p^{0}e_{h}^{0}-qb_{h}}{p^{0}} \\ x_{h}^{1} =\frac{\alpha _{h}^{1}}{\lambda _{h}^{1}}=e_{h}^{1}+b_{h} \\ x_{h}^{2} =\frac{\alpha _{h}^{2}}{\lambda _{h}^{2}}=e_{h}^{2}+b_{h} \\ -\frac{\alpha _{h}^{0}q}{p^{0}e_{h}^{0}-qb_{h}}+\frac{\alpha _{h}^{1}}{ e_{h}^{1}+b_{h}}+\frac{\alpha _{h}^{2}}{e_{h}^{2}+b_{h}} =0 \\ \lambda _{h}^{0} =\frac{\alpha _{h}^{0}}{p^{0}e_{h}^{0}-qb_{h}} \\ \lambda _{h}^{1} =\frac{\alpha _{h}^{1}}{e_{h}^{1}+b_{h}} \\ \lambda _{h}^{2} =\frac{\alpha _{h}^{2}}{e_{h}^{2}+b_{h}} \end{array}\right. \end{aligned}$$

Observe that equation

$$\begin{aligned} \frac{\alpha _{h}^{0}q}{-p^{0}e_{h}^{0}+qb_{h}}+\frac{\alpha _{h}^{1}}{ e_{h}^{1}+b_{h}}+\frac{\alpha _{h}^{2}}{e_{h}^{2}+b_{h}}=0 \end{aligned}$$

(22)

allows to find $b_{h}$ as a function of q and exogenous variables; recall also that $p^{0}=3-q$.

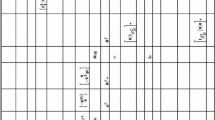
Given to utility weights and to endowments the values listed in the table below, we have that $b_{1}=\frac{1}{4q}\left( -7q+18\right)$ and $b_{2}=\frac{1}{4q }\left( -5q+9\right)$.

Using market clearing for assets ($b_{1}+b_{2}=0$), we then get that the unique equilibrium value of q is $\frac{9 }{4}$ and then equilibrium price in period zero is $p^{0}= \frac{3}{4}$. It also follows that $b_{1}\left( q\right) =\frac{1}{4}=-b_{2}$. Then, the equilibrium allocations are

$$\begin{aligned} x_{1}=\left( \frac{5}{4},\frac{5}{4},\frac{5}{4}\right) \text { and } x_{2}=\left( \frac{7}{4},\frac{7}{4},\frac{7}{4}\right) \text {,} \end{aligned}$$

and the values of the utility functions in equilibrium are

$$\begin{aligned} u_{1}\left( x_{1}\right) =4\log \frac{5}{4}=0.892\,57\text { and } u_{2}\left( x_{2}\right) =4\log \frac{7}{4}=2.\,238\,5\text {.} \end{aligned}$$

Observe also that $q=\nu ^{1}+\nu ^{2}=\frac{3}{2}+\frac{3}{4} =\frac{9}{4}\,$.

The equilibrium in the complete market case.

Markets are complete if the return matrix has full rank, say it is the identity matrix. In that case, household h problem becomes the following one (we normalized to 1 the price of good 2):

$$\begin{aligned} \begin{aligned}&\max _{\left( x_{h}^{0},x_{h}^{1},x_{h}^{2}\right) }\alpha _{h}^{0}\log x_{h}^{0}+\alpha _{h}^{1}\log x_{h}^{1}+\alpha _{h}^{2}\log x_{h}^{2} \\&\text {s.t.}\quad -p^{0}\left( x_{h}^{0}-e_{h}^{0}\right) -p^{1}\left( x_{h}^{1}-e_{h}^{1}\right) -\left( x_{h}^{2}-e_{h}^{2}\right) =0, \end{aligned} \end{aligned}$$

with associated Lagrange conditions

$$\begin{aligned} \begin{aligned}&\frac{\alpha _{h}^{0}}{x_{h}^{0}}-\mu p^{0} =0 \\&\frac{\alpha _{h}^{1}}{x_{h}^{1}}-\mu p^{1} =0 \\&\frac{\alpha _{h}^{2}}{x_{h}^{2}}-\mu =0 \\&-p^{0}\left( x_{h}^{0}-e_{h}^{0}\right) -p^{1}\left( x_{h}^{1}-e_{h}^{1}\right) -\left( x_{h}^{2}-e_{h}^{2}\right) =0 \end{aligned} \end{aligned}$$

We then find that in the complete market case, equilibrium prices are $p^{0}=\frac{12}{17}, p^{1}=\frac{19}{17}, \ p^{2}=1$ and equilibrium allocations and utilities are as described in the table below.

$$\begin{aligned} \begin{array}{ccccccccc} &{} &{} x^{0} &{} &{} x^{1} &{} &{} x^{2} &{} &{} u \\ &{} &{} &{} &{} &{} &{} &{} &{} \\ h=1 &{} &{} \frac{5}{4} &{} &{} \frac{15}{19} &{} &{} \frac{30}{17} &{} &{} 1.\,122\,7 \\ &{} &{} &{} &{} &{} &{} &{} &{} \\ h=2 &{} &{} \frac{7}{4} &{} &{} \frac{42}{19} &{} &{} \frac{21}{17} &{} &{} 2.\,357\,4 \end{array} \end{aligned}$$

The complete market equilibrium allocation is “Pareto superior” to the incomplete market equilibrium allocation, i.e., in the former case both households get a higher value of their utility functions. Indeed, it is a well-known fact that while for any economy, equilibrium allocation in the complete market case is Pareto optimal, or efficient, for almost all economies, they are inefficient in the incomplete market case.

Appendix B: Basic Notions on Variational Inequalities

For the reader’s convenience, we recall here basic definitions of the set-valued analysis and some basic concepts of variational analysis, that are useful in the paper.

Definition B.1

(see, e.g., [23]) Let $F:{\mathbb {R}}^n \rightrightarrows {\mathbb {R}}^m$ be a set-valued map. We define the domain and the graph of F, respectively:

$$\begin{aligned}&Dom\, F:=\{x\in {\mathbb {R}}^n: \,\ F(x)\ne \emptyset \}; \\&Graph(F):=\{(x,y)\in {\mathbb {R}}^n\times {\mathbb {R}}^m: y\in F(x)\}. \end{aligned}$$

Let F be such that $Dom \, F\ne \emptyset$. F is:

• (a) upper semicontinuous at $x\in {\mathbb {R}}^n$, iff for each open set $V\subset {\mathbb {R}}^m$, where $F(x)\subset V$, there exists a neighborhood $U\subset {\mathbb {R}}^n$ of x such that for all $x^{\prime }\in U:\, F(x^{\prime })\subset V$;

• (b) lower semicontinuous at $x\in {\mathbb {R}}^n$, iff for any sequence of elements $\{x_n\}_{n\in {\mathbb {N}}}\subset {\mathbb {R}}^n$, $x_n\rightarrow x$, and for any $y\in F(x)$, there exists a sequence $\{y_n\}_{n\in {\mathbb {N}}}\subset {\mathbb {R}}^m$, with $y_n\in F(x_n)\; \forall n$ and $y_n\rightarrow y$;

• (c) closed, iff for any sequences $\{x_n\}_{n\in {\mathbb {N}} }\subset {\mathbb {R}}^n$, $\{y_n\}_{n\in {\mathbb {N}}}\subset {\mathbb {R}}^m$, if $x_n\rightarrow x$ and $y_n\in F(x_n)$, $y_n\rightarrow y$ then $y\in F(x)$.

Definition B.2

Let $C\subseteq {\mathbb {R}}^n$ be a nonempty, closed and convex set and let $S:C\rightrightarrows {\mathbb {R}}^{n}$ and ${\varPhi }:C\rightrightarrows {\mathbb {R}}^{n}$ be set-valued maps. A generalized quasivariational inequality associated with $C,S,{\varPhi }$, denoted by GQVI, is the following problem:

$$\begin{aligned} \text {``}{} Find {\overline{x}}\in S({\overline{x}})\; s. t. \; \exists \,\ \varphi \in {\varPhi }({\overline{x}}) with \;\; \langle \varphi , x- {\overline{x}}\rangle \ge 0 \;\ \forall x\in S({\overline{x}}).\text {''} \end{aligned}$$

(23)

In particular, when $S(x)=C$ for all $x\in C$, (23) is a Generalized Variational Inequality, GVI; when ${\varPhi }$ is single-valued, (23) reduces to the quasivariational inequality, QVI. When both ${\varPhi }(x)$ is singleton and $S(x)=C$, for all $x\in C$, we have the classical Stampacchia Variational Inequality, VI.

Theorem B.1

(see [24]) Let C be a nonempty, convex and compact subset of ${\mathbb {R}}^n$. Let ${\varPhi }:C \rightrightarrows {\mathbb {R}}^n$ and $S:C \rightrightarrows C$ be two set-valued maps satisfying the following properties:

• (i) S is closed, lower semicontinuous and with nonempty, convex and compact values;

• (ii) ${\varPhi }$ is upper semicontinuous with nonempty, convex and compact values.

Then, the GQVI (23) admits at least a solution.

Now, we deal with the connection between a maximum problem and a suitable variational problem when the objective function is quasiconcave and continuous (see [20, 25, 26]).

Definition B.3

The function $u:{\mathbb {R}}^n\rightarrow {\mathbb {R}}$ is said to be

• (i) quasiconcave, iff for any x, y and $\lambda \in [0,1]$ one has

  $$\begin{aligned} u(\lambda x+(1-\lambda ) y)\ge \min \{u(x), u(y)\}\,; \end{aligned}$$

• (ii) semistrictly quasiconcave, iff for any x, y such that $u(x)\ne u(y)$, one has

  $$\begin{aligned} u(\lambda x+(1-\lambda )y) > \min \{u(x), u(y)\}, \quad \forall \lambda \in ]0,1[\,; \end{aligned}$$

• (iii) strictly quasiconcave, iff for any x, y, with $x\ne y$, one has

  $$\begin{aligned} u(\lambda x+(1-\lambda )y) > \min \{u(x), u(y)\}, \quad \forall \lambda \in ]0,1[. \end{aligned}$$

We can characterize the quasiconcave functions in terms of upper level sets. Let us denote, for any $\alpha \in {\mathbb {R}}$, by $U_{\alpha }(u)$ and $U^>_{\alpha }(u)$ the upper level set and the strict upper level set, respectively, associated with u and $\alpha$:

$$\begin{aligned} U_{\alpha }(u)=\{x\in {\mathbb {R}}^n: u(x)\ge \alpha \},\qquad U^>_{\alpha }(u)=\{x\in {\mathbb {R}}^n: u(x)>\alpha \}. \end{aligned}$$

For a lighter notation, we will use, for any $x\in {\mathbb {R}}^n$, $U_{u(x)}$ and $U^>_{u(x)}$ instead of $U_{u(x)}(u)$ and $U^>_{u(x)}(u)$.

Proposition B.1

(Theorem 2.2.3 in [26]) Let $u:{\mathbb {R}}^n\rightarrow {\mathbb {R}}$ be a function. One has:

u is quasiconcave, if and only if, for any $\alpha \in {\mathbb {R}}$, the upper level set $U_{\alpha }(u)$ is a convex set (the strict upper level set $U^>_{\alpha }(u)$ is a convex set).

When the function u is quasiconcave, let us set N(x) and $N^{>}(x)$ the normal cones, respectively, to the sets $U_{u(x)}$ and $U_{u(x)}^{>}$:

$$\begin{aligned}&N(x):=\{h\in {\mathbb {R}}^n:\;\langle h,z-x\rangle \le 0\quad \forall \;z\in U_{u(x)}\}, \\&N^{>}(x):=\{h\in {\mathbb {R}}^n:\;\langle h,z-x\rangle \le 0\quad \forall \;z\in U_{u(x)}^{>}\}. \end{aligned}$$

It is clear that $N(x)\subseteq N^{>}(x)$ for all $x\in {\mathbb {R}}^n$, and when u is semistrictly quasiconcave one has $N(x)=N^{>}(x)$ for all $x\notin argmax_{{\mathbb {R}}^n}u$.

Let us define ${\mathcal {G}}:{\mathbb {R}}^{n}\rightrightarrows {\mathbb {R}}^{n}$ such that

$$\begin{aligned} {\mathcal {G}}^{\prime }(x):=\left\{ \begin{array}{ll} {\overline{B}}(0,1), &{} if\,\ x\in \displaystyle \mathrm {argmax}_{{\mathbb {R}}^n}u, \\ &{} \\ conv\Big (N^{>}(x)\cap S(0,1)\Big ), &{} if\,\ \displaystyle x\notin \mathrm {argmax}_{{\mathbb {R}}^n}u, \end{array} \right. \end{aligned}$$

(24)

where $\quad {\overline{B}}(0,1)=\{x\in {\mathbb {R}}^{n}:\Vert x\Vert \le 1\}$ is the closed unit ball of ${\mathbb {R}}^{n}$.

Definition B.4

Given a function $u:{\mathbb {R}}^{n}\rightarrow {\mathbb {R}}$, we say that u is

• (i) Locally non-satiated, if $\forall x\in {\mathbb {R}}^n$ and $\forall \varepsilon >0$, $\exists x^{\prime }\in B\left( x,\varepsilon \right)$ such that $u\left( x^{\prime }\right) >u\left( x\right)$;

• (ii) Non-satiated, if $\ \forall x\in {\mathbb {R}}^n$ $\exists x^{\prime }\in {\mathbb {R}}^n$ such that $u\left( x^{\prime }\right) >u\left( x\right)$.

Proposition B.2

If $u:{\mathbb {R}}^{n}\rightarrow {\mathbb {R}}$ is continuous. Then, u is semistrictly quasiconcave and non-satiated if and only if u is quasiconcave and locally non-satiated.

Proposition B.3

Let $u:{\mathbb {R}}^n\rightarrow {\mathbb {R}}$ be continuous, quasiconcave and strictly increasing in the component j. Then, u is semistrictly quasiconcave and non-satiated.

Proof

For any $x\in {\mathbb {R}}^n$ and $\varepsilon >0$, we pose

$$\begin{aligned} (x^{\prime })^i:=\left\{ \begin{array}{lll} x^i, &{} &{} \quad \text {if}\quad i\ne j, \\ x^j+K, &{} &{} \quad \text {if}\quad i=j, \end{array} \right. \end{aligned}$$

with $0<K<\varepsilon$. One has that $x^{\prime }\in B\left( x,\varepsilon \right)$ and, since u is strictly increasing in j, $u(x^{\prime })>u(x)$, that is u is locally non-satiated. Hence, from Proposition B.2 u is semistrictly quasiconcave and non-satiated. $\square$

Theorem B.2

(see [20]) Let $u:{\mathbb {R}}^n\rightarrow {\mathbb {R}}$ be a continuous and semistrictly quasiconcave function. Then, the set-valued map ${\mathcal {G}}^{\prime }$ is with nonempty, convex and compact values, and upper semicontinuous.

Theorem B.3

(see Proposition 4.1 in [22]) Let $u:{\mathbb {R}}^n\rightarrow {\mathbb {R}}^{n}$ be a continuous and semistrictly quasiconcave function and let K be a convex set. Then, ${\widetilde{x}}\in K$ is solution to the maximization problem

$$\begin{aligned} \max _{x\in K}u(x) \end{aligned}$$

if and only if ${\widetilde{x}}$ is solution to GVI

$$\begin{aligned} ``\ \text {Find}\;\;{\widetilde{x}}\in K\;\text {s. t.}\;\exists \,\ g\in {\mathcal {G}}({\widetilde{x}})\;\;\text {with}\;\;\langle g,x-{\widetilde{x}}\rangle \ge 0\quad \forall x\in K.'' \end{aligned}$$

(25)