Abstract
Kremer presented three approaches of comparing fixed-point and revision theories of truth in Kremer (Journal of Philosophical Logic, 38(4), 363–403, 2009). Using these approaches, he established the relationships among ten fixed-point theories suggested by Kripke in (Journal of Philosophy, 72(19), 690–716, 1975) and three revision theories presented by Gupta and Belnap in (1993). This paper continues Kremer’s work. We add five other revision theories to the comparisons, including the theory proposed by Gupta in (Journal of Philosophical Logic, 11(1), 1–60, 1982), the theory proposed by Herzberger in (Journal of Philosophical Logic, 11(1), 61–102, 1982), the theory based on fully-varied revision sequences (and stability) proposed by Gupta and Belnap in (1993), the theory proposed by Yaqūb in (1993), and the theory based on weakly consistent revision sequences (and stability). We show that, the notion of Thomason model defined by Belnap’s limit rule is not equivalent to the one defined by Gupta’s limit rule, and that the theory based on fully-varied revision sequences is ≤2-equivalent to the one based on the greatest intrinsic fixed point of σ.
Similar content being viewed by others
Notes
x≠y is the abbreviation of ¬x = y. x < y is the abbreviation of x ≤ y ∧ x≠y. Similarly, x <Sy is the abbreviation of x ≤Sy ∧ x≠y.
y ≥Sx =dfx ≤Sy. y >Sx =dfx <Sy. y ≥ x =dfx ≤ y. y > x =dfx < y.
For convenience, we use the same notation “≤” for the partial order on the hypotheses, the linear order on the ordinals and the restriction of it to the set 3ω (in the object language \({{\mathscr{L}}}^{+}\) and the metalanguage). We believe that no confusion arises because of this.
When we say An,α is less than or equal to Am,β, we mean that I(≤S) holds between An,α and Am,β. And “An,α is less than Am,β” means that I(≤S) holds between An,α and Am,β, and they are not the same.
In this proof, we use “1” to represent t, and “0” to represent f. For every classical hypothesis h and every α ∈ 3ω, we take the sequence \({\mathscr{S}}=\langle h(A_{n,\alpha }) \mid n\in \omega \rangle \) as a truth value in a broad sense, and we say that h declares line \(\alpha ~{\mathscr{S}}\). Given k ∈{1, 2, 3}, for every n ∈ ω, let the declared truth value of line n of plane k by h be \({\mathscr{S}}_{n}\). Then we also take the sequence \({\mathscr{S}}=\langle {\mathscr{S}}_{n} \mid n\in \omega \rangle \) as a truth value in a broad sense, and we say that h declares plane \(k~{\mathscr{S}}\).
s(x∣An,α) is a function from the set of variables to D, defined as follows: if y = x, then s(x∣An,α)(y) = An,α; otherwise, s(x∣An,α)(y) = s(y).
The underlined place in the table indicates the location of An,α.
Ditto.
The underlined place in the table indicates the location of A0,α. Since α≠ 0,ω, 2ω, α must be greater than or equal to ω × (k − 1) + 1.
The underlined place in the table indicates the location of A0,α.
Ditto.
The underlined place in the table indicates the location of A0,α. Since n is odd, α must be greater than or equal to ω × (k − 1) + 1.
Note that φ6 is a sentence. h⊧φ6 iff h⊧∃xφ6(x).
Given a term t, and a formula with exactly one free variable xφ(x), let φ(t) be the result of replacing all free occurrences of x in φ(x) by t. Note that for every classical hypothesis h, \(\tau _{{\mathscr{M}}}(h)\) is maximally consistent, so \(\tau _{{\mathscr{M}}}(h)\models \varphi _{c}\).
When we say that a classical hypothesis h satisfies φ1(an,α)(n ≥ 1), we mean that there is an n ∈ ω and an α ∈ 3ω such that n ≥ 1 and h satisfies φ1(an,α). When we say that a classical hypothesis h satisfies φ4(a0,α)(α≠ 0,ω, 2ω), we mean that there is an α ∈ 3ω such that α≠ 0,ω, 2ω and h satisfies φ4(a0,α).
It means that there is at most one line that is declared
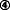 or
or  by h.
by h.It means that for every α, every β ∈ 3ω, if line α is declared
 by h and line β is declared
by h and line β is declared 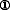 ,
, 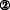 ,
, 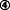 or
or 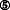 by h, then α > β.
by h, then α > β.When we say some line is
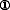 , we mean that this line is declared
, we mean that this line is declared  by h. For every ordinal α < 3ω, if there is an ordinal β such that α = β + 1, then the previous line of line α is line β; otherwise, the previous line of line α does not exist.
by h. For every ordinal α < 3ω, if there is an ordinal β such that α = β + 1, then the previous line of line α is line β; otherwise, the previous line of line α does not exist.When we say there are successive occurrences of
 in some plane, we mean that there are two adjacent lines in this plane that are declared
in some plane, we mean that there are two adjacent lines in this plane that are declared 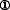 by h. When we say some plane is all-
by h. When we say some plane is all-  , we mean that each line of this plane is declared
, we mean that each line of this plane is declared 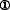 by h.
by h.Let λ be a limit ordinal, and \({\mathscr{S}}\) be a sequence of classical hypotheses whose length is λ. We say that \({\mathscr{S}}\) is a λ-length revision sequence iff for every α < λ, the following conditions hold: (1) if α = β + 1, then \({{\mathscr{S}}}_{\alpha }=\tau _{{\mathscr{M}}}({\mathscr{S}}_{\beta })\); and (2) if α is a limit ordinal, then the sentences that are stably true up to α are declared true by \({{\mathscr{S}}}_{\alpha }\), and the sentences that are stably false up to α are declared false by \({{\mathscr{S}}}_{\alpha }\). Obviously, \({\mathscr{S}}\) is a λ-length revision sequence iff there is a revision sequence \({\mathscr{S}}^{\prime }\) such that \({\mathscr{S}}={\mathscr{S}}^{\prime } \upharpoonright \lambda \).
Since γ is a successor ordinal, \({\mathscr{S}}_{\gamma }\) is maximally consisitent. Hence \({\mathscr{S}}_{\gamma }\) must satisfy φc.
Let \(X\subseteq Sent({{\mathscr{L}}}^{+})\) and \(A\in Sent({{\mathscr{L}}}^{+})\). We say that X declares A true if A ∈ X; otherwise, we say that X declares A false.
For every i ∈ ω, we say that line i of plane k is an even line of plane k if i ∈{0, 2, 4, 6, 8,⋯ }; otherwise, we say that line i of plane k is an odd line of plane k.
References
Belnap, N.D. (1982). Gupta’s rule of revision theory of truth. Journal of Philosophical Logic, 11(1), 103–116.
Chapuis, A. (1996). Alternative revision theories of truth. Journal of Philosophical Logic, 25(4), 399–423.
Cook, R.T. (2013). Paradoxes. Cambridge: Polity Press.
Gupta, A. (1982). Truth and paradox. Journal of Philosophical Logic, 11(1), 1–60.
Gupta, A., & Belnap, N.D. (1993). The revision theory of truth. Cambridge: MIT Press.
Herzberger, H.G. (1982). Notes on naive semantics. Journal of Philosophical Logic, 11(1), 61–102.
Kremer, P. (2009). Comparing fixed-point and revision theories of truth. Journal of Philosophical Logic, 38(4), 363–403.
Kremer, P. (2010). How truth behaves when there’s no vicious reference. Journal of Philosophical Logic, 39(4), 345–367.
Kripke, S.A. (1975). Outline of a theory of truth. Journal of Philosophy, 72(19), 690–716.
Martin, R.L., & Woodruff, P.W. (1975). On representing ‘true-in-l’ in l. Philosophia, 5(3), 213–217.
Tarski, A. (1944). The semantic conception of truth and the foundations of semantics. Philosophy and Phenomenological Research, 4(3), 341–376.
Welch, P.D. (2003). On revision operators. The Journal of Symbolic Logic, 68(2), 689–711.
Yaqūb, A. M. (1993). The liar speaks the truth: a defense of the revision theory of truth. Oxford University Press on Demand.
Acknowledgments
Thanks to the anonymous reviewers for their detailed and helpful comments on earlier manuscripts of this paper. Their comments helped us to make the paper more readable (both in language and in content), and made us realize some errors in the earlier manuscripts. Special thanks to one of the reviewers, who made us realize that the concept of consistent set of sentences defined in Kripke’s paper [9] is different from the one implicit in Kremer’s paper [7].
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This research is supported by NSSFC Grant 14ZDB015.
Appendix: Proof of Lemma 23
Appendix: Proof of Lemma 23
Proof
Besides T and quote names, let the truth language \({{\mathscr{L}}}^{+}\) have only the following nonlogical symbols: countably many nonquote names \(\overline {\alpha }(\alpha \in 3\omega )\), and an,α(n ∈ ω,α ∈ 3ω); three 1-place predicate symbols E, O, and S; four 2-place predicate symbols ≤, ≤S, Ve and Vo; one 3-place predicate symbol P; and three 1-place function symbols F1, F2 and Fω. Define formulas with exactly 1 free variable φ1(x), φ2(x), φ3(x), φ4(x) and φ5(x), and sentences φ6, φc, φothers and An,α(n ∈ ω,α ∈ 3ω) as follows.
φ1(x) is the conjunction of the following four formulas:Footnote 1
-
(1)
Sx;
-
(2)
∀y(Sy ∧ Fωy < Fωx →¬Ty);
-
(3)
\(\forall y(Sy\land y<_{S}x \land F_{\omega } y=F_{\omega } x \to (V_{e} yx\leftrightarrow (\boldsymbol {T} y \leftrightarrow \boldsymbol {T} x)))\);
-
(4)
\(\forall y(Sy\land y\ge _{S}x \to (\boldsymbol {T} y \leftrightarrow EF_{1} y)) \).
φ2(x) is the conjunction of the following five formulas:Footnote 2
-
(1)
Sx;
-
(2)
\(F_{1} x=\overline {0} \land F_{2} x\not = \overline {0}\land F_{2} x\not = \overline {\omega }\land F_{2} x\not = \overline {2\omega }\);
-
(3)
∀y(Sy ∧ Fωy < Fωx →¬Ty);
-
(4)
\(\forall y(Sy\land y<_{S}x \land F_{\omega } y=F_{\omega } x \to (V_{o} yx\leftrightarrow (\boldsymbol {T} y \leftrightarrow \boldsymbol {T} x)))\);
-
(5)
\(\forall y(Sy\land y\ge _{S}x \to (\boldsymbol {T} y \leftrightarrow EF_{1} y)) \).
φ3(x) is the conjunction of the following five formulas:
-
(1)
Sx;
-
(2)
x = a0,0 ∨ x = a0,ω ∨ x = a0,2ω;
-
(3)
∀y(Sy ∧ Fωy < Fωx →¬Ty);
-
(4)
\(\forall y(Sy \land F_{\omega } y=F_{\omega } x \to (\boldsymbol {T} y \leftrightarrow OF_{2} y))\);
-
(5)
\(\forall y(Sy \land F_{\omega } y>F_{\omega } x \to (\boldsymbol {T} y \leftrightarrow EF_{1} y))\).
φ4(x) is the conjunction of the following five formulas:
-
(1)
Sx;
-
(2)
\(F_{1} x=\overline {0}\);
-
(3)
∀y(Sy ∧ Fωy < Fωx →¬Ty);
-
(4)
\(\forall y(Sy \land F_{\omega } y=F_{\omega } x \to (F_{2} y<F_{2} x\to (\boldsymbol {T} y \leftrightarrow \boldsymbol {T} x) )\land (F_{2} y\ge F_{2} x\to (\boldsymbol {T} y \leftrightarrow EF_{2} y)))\);
-
(5)
\(\forall y(Sy \land F_{\omega } y>F_{\omega } x \to (\boldsymbol {T} y \leftrightarrow EF_{1} y)) \).
φ5(x) is the conjunction of the following four formulas:
-
(1)
\(x=\overline {1}\lor x=\overline {2}\lor x=\overline {3}\);
-
(2)
∀y(Sy ∧ Fωy < x →¬Ty);
-
(3)
∀y(Sy ∧ Fωy = x →Ty);
-
(4)
\(\forall y(Sy \land F_{\omega } y> x \to (\boldsymbol {T} y \leftrightarrow EF_{1} y)) \).
-
φ6 = df∀x(Sx →¬Tx).
-
\(\varphi _{c}=_{df}\forall x\forall y\forall z(Pxyz\to ((\boldsymbol {T} x \leftrightarrow \boldsymbol {T} y)\leftrightarrow \boldsymbol {T} z)) \).
-
φothers = df¬(φc ∧ (∃xφ1(x) ∨∃xφ2(x) ∨∃xφ3(x) ∨∃xφ4(x) ∨∃xφ5(x) ∨ φ6)).
For every n ∈ ω and every α ∈ 3ω, An,α is the disjunction of the following eleven formulas:
-
(1)
φc∧∃x(φ1(x)∧an,α ≤Sx∧Fωan,α = Fωx∧((EF1x∧Voan,αx)∨(OF1x∧Vean,αx)));
-
(2)
\(\varphi _{c} \land \exists x(\varphi _{1}(x)\land a_{n,\alpha } >_{S} x \land E\overline {n})\);
-
(3)
φc ∧∃x(φ2(x) ∧ an,α <Sx ∧ Fωan,α = Fωx ∧ Voan,αx);
-
(4)
\(\varphi _{c} \land \exists x(\varphi _{2}(x)\land a_{n,\alpha } >_{S} x \land E\overline {n})\);
-
(5)
\(\varphi _{c} \land \exists x(\varphi _{3}(x)\land F_{\omega } a_{n,\alpha }= F_{\omega } x\land \overline {\alpha }\neq F_{2} x\land E\overline {\alpha })\);
-
(6)
\(\varphi _{c} \land \exists x(\varphi _{3}(x)\land F_{\omega } a_{n,\alpha }> F_{\omega } x\land E\overline {n})\);
-
(7)
\(\varphi _{c} \land \exists x(\varphi _{4}(x) \land F_{\omega } a_{n,\alpha }= F_{\omega } x\land \overline {\alpha }\le F_{2} x\land OF_{2} x)\);
-
(8)
\(\varphi _{c} \land \exists x(\varphi _{4}(x) \land F_{\omega } a_{n,\alpha }= F_{\omega } x\land \overline {\alpha }>F_{2} x\land E\overline {\alpha })\);
-
(9)
\(\varphi _{c} \land \exists x(\varphi _{4}(x) \land F_{\omega } a_{n,\alpha }> F_{\omega } x\land E\overline {n})\);
-
(10)
\(\varphi _{c} \land \exists x(\varphi _{5}(x) \land F_{\omega } a_{n,\alpha }> x\land E\overline {n}\land (\overline {n}=\overline {0}\land x=\overline {1}\to \overline {\alpha } \neq \overline {\omega })\land (\overline {n}=\overline {0}\land x=\overline {2}\to \overline {\alpha } \neq \overline {2\omega }))\);
-
(11)
\(\varphi _{others}\land E\overline {n}\).
Let \({\mathscr{M}}=\langle D,I\rangle \) be the ground model of \({{\mathscr{L}}}^{+}\) with
-
\(D=Sent({{\mathscr{L}}}^{+})\cup 3\omega \);
-
for every α ∈ 3ω, \(I(\overline {\alpha })=\alpha \);
-
for every n ∈ ω and every α ∈ 3ω, I(an,α) = An,α;
-
I(E) = {α ∈ 3ω∣α = ω × m + n,m,n ∈ ω,n is even};
-
I(O) = {α ∈ 3ω∣α = ω × m + n,m,n ∈ ω,n is odd};
-
I(S) = {An,α∣n ∈ ω,α ∈ 3ω};
-
I(≤) = {(α,β)∣α,β ∈ 3ω,α ≤ β};Footnote 3
-
I(≤S) = {(An,α,Am,β)∣m,n ∈ ω,α,β ∈ 3ω,α < β ∨ (α = β ∧ n ≤ m)};
-
I(Ve) = {(An,α,Am,β)∣m,n ∈ ω,α,β ∈ 3ω,∃k ∈ ω(α = β + 2k ∨ β = α + 2k)};
-
I(Vo) = {(An,α,Am,β))∣m,n ∈ ω,α,β ∈ 3ω,∃k ∈ ω(α = β + 2k + 1 ∨ β = α + 2k + 1)};
-
\(I(P)=\{(A_{n,\alpha },A_{m,\beta },A_{n,\alpha }\leftrightarrow A_{m,\beta })\mid m,n \in \omega ,\alpha ,\beta \in 3\omega \}\); and
-
I(F1), I(F2) and I(Fω) are 1-place functions on D f1, f2 and fω respectively.
f1 is defined as follows: for every d ∈ D,
f2 is defined as follows: for every d ∈ D,
fω is defined as follows: for every d ∈ D,
The restriction of I(≤S) to I(S) is a linear order. If n≠m or α≠β, then An,α≠Am,β. Hence, f1, f2 and fω are 1-place functions on D. For every n ∈ ω and every α ∈ 3ω, we say that An,α is in plane k iff fω(An,α) = k (of course, k ∈{1, 2, 3}); say that An,α is in line α; say that An,α is the n th sentence of line α; and say that line α is line t of plane k + 1 if α = ω × k + t and t ∈ ω. Call n the abscissa of An,α and α the ordinate of An,α. The relation I(Ve) holds between An,α and Am,β iff, they are in the same line, or they are in the same plane and there are an odd number of lines between them. The relation I(Vo) holds between An,α and Am,β iff, they are in the same plane, but not in the same line, and there are an even number of lines between them. Note that for every maximally consistent classical hypothesis h, \({\mathscr{M}}+h\models \varphi _{c}\).
We can use the following table to represent the sentences in I(S):

In the table above, a sentence in some plane is less than every sentence in its next plane (if exists); a sentence in some line is less than every sentence in its next line; and for any two different sentences in the same line, the one on the left is less than the one on the right.Footnote 4 We say that An,α is before Am,β, or Am,β is afterAn,α iff, An,α is less than Am,β. Similarly, we say that a sentence is before (after) some line (plane), say that a line is before (after) another line, say that a plane is before (after) another plane, and so on.
Let h be a classical hypothesis that it declares the sentences in line α as follows: h declares the 0th sentence of line α true, h declares the 1th sentence of line α false, h declares the 2th sentence of line α true, h declares the 3th sentence of line α false, and so on. We can use the following table to represent the declared truth value of line α by h:Footnote 5

For every classical hypothesis and every α ∈ 3ω, if the declared truth value of line α by this hypothesis enters a loop from some sentence, then we can use a table of the same kind to represent the declared truth value of line α by this hypothesis. Similarly, for every classical hypothesis and every k ∈{1, 2, 3}, if the declared truth value of each line of plane k by this hypothesis enters a loop from some sentence, and the declared truth value of plane k by this hypothesis enters a loop from some line, then we can use a table to represent the declared truth value of plane k by this hypothesis. For example, let \(h^{\prime }\) be a classical hypothesis that it declares the sentences in plane k (where k ∈{1, 2, 3}) as follows: \(h^{\prime }\) declares the sentences in line 0 of plane k true; \(h^{\prime }\) declares the sentences in line 1 of plane k false; \(h^{\prime }\) declares the sentences in line 2 of plane k with even abscissas true, and others false; and the declared truth value of each of the other lines of plane k by \(h^{\prime }\) is the same as that of line 2 of plane k by \(h^{\prime }\). We can use the following table to represent the declared truth value of plane k by \(h^{\prime }\):

Conventions in this proof: if h is a classical hypothesis and φ is a sentence, then h⊧φ is an abbreviation for \({\mathscr{M}}+h\models \varphi \), and we say that h satisfies φ iff h⊧φ; when we mention a sentence An,α or nonquote name an,α, n ∈ ω and α ∈ 3ω by default; and when we mention plane k, k ∈{1, 2, 3} by default.
Claim 1. Let h be a classical hypothesis, and s be a function from the set of variables to D.
-
(1)
If h⊧∃xφ1(x), then there is a unique element An,α in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{n,\alpha })\models \varphi _{1}(x)\).Footnote 6
-
(2)
If h⊧∃xφ2(x), then there is a unique element A0,α(α≠ 0,ω, 2ω) in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{0,\alpha })\models \varphi _{2}(x)\).
-
(3)
If h⊧∃xφ3(x), then there is a unique element A0,α(α = 0,ω, 2ω) in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{0,\alpha })\models \varphi _{3}(x)\).
-
(4)
If h⊧∃xφ4(x), then there is a unique element A0,α in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{0,\alpha })\models \varphi _{4}(x)\).
-
(5)
If h⊧∃xφ5(x), then there is a unique element k(k = 1, 2, 3) in D such that \({\mathscr{M}}+h,s(x\mid k)\models \varphi _{5}(x)\).
Proof of (1). If h⊧∃xφ1(x), then there is an element d ∈ D such that \({\mathscr{M}}+h,s(x\mid d)\models \varphi _{1}(x)\). According to the first conjunct of φ1(x), d is an element in I(S). Let d be An,α. According to the second conjunct of φ1(x), all sentences in a plane before An,α are declared false by h. According to the fourth conjunct of φ1(x), the sentences after An,α and An,α are declared true by h iff their abscissas are even. In particular, the sentences in a plane after An,α are declared true by h iff their abscissas are even. According to the third conjunct of φ1(x), for each of sentences that is in the same plane with An,α and that is before An,α, its declared truth value by h is the same as that of An,α by h iff, it is in the same line with An,α, or there are an odd number of lines between it and An,α. Suppose that An,α is in plane k. If n is even, then the declared truth value of plane k by h is as follows:Footnote 7

If n is odd, then the declared truth value of plane k by h is as follows:Footnote 8

Obviously, for any d≠An,α, \({\mathscr{M}}+h,s(x\mid d)\not \models \varphi _{1}(x)\). So (1) holds.
Similarly, we can prove (2). By the definition of φ2(x), we can easily prove that there is a unique element A0,α(α≠ 0,ω, 2ω) in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{0,\alpha })\models \varphi _{2}(x)\). Suppose that A0,α is in plane k. All sentences in a plane before plane k are declared false by h. The sentences in a plane after plane k are declared true by h iff their abscissas are even. And the declared truth value of plane k by h is as follows:Footnote 9

Similarly, we can prove (3). By the definition of φ3(x), we can easily prove that there is a unique element A0,α(α = 0,ω, 2ω) in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{0,\alpha })\models \varphi _{3}(x)\). Suppose that A0,α is in plane k. All sentences in a plane before plane k are declared false by h. The sentences in a plane after plane k are declared true by h iff their abscissas are even. And the declared truth value of plane k by h is as follows:Footnote 10
Similarly, we can prove (4). By the definition of φ4(x), we can easily prove that there is a unique element A0,α in I(S) such that \({\mathscr{M}}+h,s(x\mid A_{0,\alpha })\models \varphi _{4}(x)\). Suppose that A0,α is in plane k. All sentences in a plane before plane k are declared false by h. The sentences in a plane after plane k are declared true by h iff their abscissas are even. If α = ω × (k − 1) + n and n is even, then the declared truth value of plane k by h is as follows:Footnote 11

If α = ω × (k − 1) + n and n is odd, then the declared truth value of plane k by h is as follows:Footnote 12
Similarly, we can prove (5). By the definition of φ5(x), we can easily prove that there is a unique element k(k = 1, 2, 3) in D such that \({\mathscr{M}}+h,s(x\mid k)\models \varphi _{5}(x)\). All sentences in a plane before plane k are declared false by h. The sentences in a plane after plane k are declared true by h iff their abscissas are even. And all sentences in plane k are declared true by h.
Claim 2. Let U be the set {φc ∧∃xφ1(x),φc ∧∃xφ2(x),φc ∧∃xφ3(x),φc ∧∃xφ4(x),φc ∧∃xφ5(x),φc ∧ φ6,φothers}. Let h be a classical hypothesis. Then there is a unique sentence φ ∈ U such that h satisfies φ.
The proof of Claim 2 is as follows. Note that if a classical hypothesis satisfies φ6, then this hypothesis declares all sentences in I(S) false. From this and the proof of Claim 1, we can know that if there is an i ∈{1, 2, 3, 4, 5, 6} such that h satisfies ∃xφi(x), then for every j ∈{1, 2, 3, 4, 5, 6}, if j≠i then h does not satisfy ∃xφj(x).Footnote 13 Then by the definition of φothers, we can see that Claim 2 holds.
Claim 3. Suppose that h is a classical hypothesis.
-
(1)
For every n ∈ ω and α ∈ 3ω, if h⊧φc ∧ φ1(an,α), then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{1}(a_{n+1,\alpha })\).Footnote 14
-
(2)
For every α ∈ 3ω such that α≠ 0,ω, 2ω, if h⊧φc ∧ φ2(a0,α), then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{1}(a_{1,\alpha })\).
-
(3)
For α = 0,ω, 2ω, if h⊧φc ∧ φ3(a0,α), then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{4}(a_{0,\alpha +1})\).
-
(4)
For every α ∈ 3ω, if h⊧φc ∧ φ4(a0,α), then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{4}(a_{0,\alpha +1})\).
-
(5)
For k = 1, 2, if \(h\models \varphi _{c} \land \varphi _{5}(\overline {k})\), then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{1}(a_{1,\omega \times k})\). If \(h\models \varphi _{c} \land \varphi _{5}(\overline {3})\), then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{6}\).
-
(6)
If h⊧φc ∧ φ6, then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{6}\).
-
(7)
If h⊧φothers, then \(\tau _{{\mathscr{M}}}(h)\models \varphi _{1}(a_{0,0})\).
Proof of (1). Suppose that h⊧φc ∧ φ1(an,α). By Claim 1, for any Am,β≠An,α, h⊮φ1(am,β). By Claim 2, for any i ∈{2, 3, 4, 5, 6}, h⊮∃xφi(x), and h⊮φothers. Suppose that An,α is in plane k. Obviously, \(\tau _{{\mathscr{M}}}(h)\) satisfies the first conjunct of φ1(an+ 1,α). Let Am,β be any sentence in I(S) with fω(Am,β) < k. By the definition of Am,β, we know that h⊮Am,β. So \(\tau _{{\mathscr{M}}}(h)(A_{m,\beta })={\mathbf {f}}\). Hence \(\tau _{{\mathscr{M}}}(h)\) satisfies the second conjunct of φ1(an+ 1,α). Let Am,β be any sentence in I(S) that is greater than or equal to An+ 1,α. By the definition of Am,β (the second disjunct), we know that h⊧Am,β iff \(h\models E(\overline {m})\). So \(\tau _{{\mathscr{M}}}(h)(A_{m,\beta })={\mathbf {t}}\) iff m is even. Hence \(\tau _{{\mathscr{M}}}(h)\) satisfies the fourth conjunct of φ1(an+ 1,α). In particular, \(\tau _{{\mathscr{M}}}(h)(A_{n+1,\alpha })={\mathbf {t}}\) iff \(h\models E(\overline {n+1})\). By the definition of An,α (the first disjunct), we know that h⊧An,α iff \(h\models O(\overline {n})\). So \(\tau _{{\mathscr{M}}}(h)\) declares An,α and An+ 1,α the same truth value. Let Am,β be any sentence in I(S) that is less than An,α and such that fω(Am,β) = k. By the definition of Am,β (the first disjunct), h⊧Am,β iff, \(h\models E(\overline {n})\land V_{o} a_{m,\beta } a_{n,\alpha }\) or \(h\models O(\overline {n})\land V_{e} a_{m,\beta } a_{n,\alpha }\). Note that h⊧Veam,βan,α iff h⊮Voam,βan,α, and that \(h\models E(\overline {n})\) iff \(h\not \models O(\overline {n})\). So if h⊧Veam,βan,α, then h⊧Am,β iff \(h\models O(\overline {n})\); and if h⊮Veam,βan,α, then h⊧Am,β iff \(h\not \models O(\overline {n})\). Hence, if h⊧Veam,βan,α, then h⊧Am,β iff h⊧An,α; and if h⊮Veam,βan,α, then h⊧Am,β iff h⊮An,α. Therefore, the relation I(Ve) holds between Am,β and An,α iff, \(\tau _{{\mathscr{M}}}(h)\) declares them the same truth value. Note that \(\tau _{{\mathscr{M}}}(h)\) declares An,α and An+ 1,α the same truth value. Hence \(\tau _{{\mathscr{M}}}(h)\) satisfies the third conjunct of φ1(an+ 1,α). Therefore, (1) holds.
Similarly, we can prove (2)–(7).
Claim 4. (1) For every classical hypothesis h, \(\tau _{{\mathscr{M}}}(h)\) satisfies φ1(a0,0), φ1(an,α)(n ≥ 1), φ4(a0,α)(α≠ 0,ω, 2ω) or φ6.Footnote 15
(2) If a classical hypothesis h is a fixed point of \(\tau _{{\mathscr{M}}}\), then h satisfies φ6.
Claim 4 is a corollary of Claim 1, Claim 2 and Claim 3.
Claim 5. For every classical hypothesis h, if there is an i ∈{1, 2, 3, 4, 5, 6} such that h satisfies ∃xφi(x), then h has the following six properties.
(I) h declares each line  ,
,  ,
,  ,
,  or
or  , where sequences
, where sequences  ,
,  ,
,  ,
,  and
and  are defined as follows:
are defined as follows:

-
(II)
The total number of occurrences of
 or
or 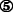 is less than or equal to 1.Footnote 16
is less than or equal to 1.Footnote 16 -
(III)
 only occurs after
only occurs after  ,
,  ,
,  and
and 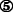 .Footnote 17
.Footnote 17 -
(IV)
 and
and 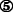 only occur after
only occur after  and
and 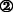 ; if one line is
; if one line is  , then its previous line (if exists) is
, then its previous line (if exists) is  ; and if one line is
; and if one line is 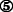 , then its previous line (if exists) is
, then its previous line (if exists) is 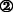 .Footnote 18
.Footnote 18 -
(V)
For every plane k, if there are successive occurrences of
 or successive occurrences of
or successive occurrences of 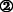 in plane k, then plane k is one of the following four cases:Footnote 19
in plane k, then plane k is one of the following four cases:Footnote 19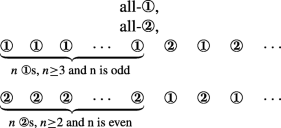
-
(VI)
If some plane is not all-
 , then the previous plane of it (if exists) is all-
, then the previous plane of it (if exists) is all- 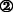 .
.
We can see that Claim 5 holds from Claim 1 and its proof and the definition of φ6.
Claim 6. For every classical hypothesis h, if h has all of the properties (I)–(VI) in Claim 5, then there is an i ∈{1, 2, 3, 4, 5, 6} such that h satisfies ∃xφi(x).
We prove Claim 6 in two cases. (1) Suppose that h declares each plane all-  or all-
or all-  . Since h has the property (III),
. Since h has the property (III),  must occur after
must occur after  . So there are four possibilities: the three planes are all-
. So there are four possibilities: the three planes are all-  ; the first two planes are all-
; the first two planes are all-  , and the third plane is all-
, and the third plane is all-  ; the first plane is all-
; the first plane is all-  , and the last two planes are all-
, and the last two planes are all-  ; and the three planes are all-
; and the three planes are all-  . In these four cases, h satisfies φ6, φ1(a0,2ω), φ1(a0,ω) and φ1(a0,0), respectively.
. In these four cases, h satisfies φ6, φ1(a0,2ω), φ1(a0,ω) and φ1(a0,0), respectively.
(2) Suppose that there is some plane that h declares neither all-  nor all-
nor all-  . Since h has the property (VI), if some plane is neither all-
. Since h has the property (VI), if some plane is neither all-  nor all-
nor all-  , then the planes before it are all-
, then the planes before it are all-  , and the planes after it are all-
, and the planes after it are all-  . So there is at most one such plane. Let plane k be the plane that h declares neither all-
. So there is at most one such plane. Let plane k be the plane that h declares neither all-  nor all-
nor all-  . Next, we prove in two subcases that there is an i ∈{1, 2, 3, 4, 5, 6} such that h satisfies ∃xφi(x).
. Next, we prove in two subcases that there is an i ∈{1, 2, 3, 4, 5, 6} such that h satisfies ∃xφi(x).
(2.1) Suppose that there is no occurrence of  ,
,  or
or  in plane k. Since h has the property (I), only
in plane k. Since h has the property (I), only  and
and  occur in plane k. Since h has the property (III),
occur in plane k. Since h has the property (III),  must occur before
must occur before  . And since h has the property (V), there are no successive occurrences of
. And since h has the property (V), there are no successive occurrences of  in plane k. So the declared truth values of lines in plane k are as follows: line 0 of plane k is
in plane k. So the declared truth values of lines in plane k are as follows: line 0 of plane k is  , and the other lines of plane k are
, and the other lines of plane k are  . Hence h satisfies φ1(a0,ω×(k− 1)+ 1).
. Hence h satisfies φ1(a0,ω×(k− 1)+ 1).
(2.2) Suppose that there is an occurrence of  ,
,  or
or  in plane k. (2.2.1) Suppose that there is an occurrence of
in plane k. (2.2.1) Suppose that there is an occurrence of  in plane k. Since h has the property (II), there is no occurrence of
in plane k. Since h has the property (II), there is no occurrence of  in plane k, and
in plane k, and  occurs only once (in plane k). Suppose that it is line t of plane k that is
occurs only once (in plane k). Suppose that it is line t of plane k that is  . And since h has the properties (IV) and (I), we know that: the lines before line t of plane k must be
. And since h has the properties (IV) and (I), we know that: the lines before line t of plane k must be  or
or  ; the lines after line t of plane k must be
; the lines after line t of plane k must be  ; and if t≠ 0, then line t − 1 of plane k is
; and if t≠ 0, then line t − 1 of plane k is  . Since h has the property (V), if t ≥ 2, then in plane k, there are neither successive occurrences of
. Since h has the property (V), if t ≥ 2, then in plane k, there are neither successive occurrences of  nor successive occurrences of
nor successive occurrences of  before
before  . So, if t ≥ 2, then in plane k,
. So, if t ≥ 2, then in plane k,  and
and  alternately occur before
alternately occur before  . In either case (t ≤ 1 or t ≥ 2), h satisfies ∃xφ1(x). (2.2.2) Suppose that there is an occurrence of
. In either case (t ≤ 1 or t ≥ 2), h satisfies ∃xφ1(x). (2.2.2) Suppose that there is an occurrence of  in plane k. Similar to (2.2.1), we can prove that h satisfies ∃xφ1(x). (2.2.3) Suppose that there is no occurrence of
in plane k. Similar to (2.2.1), we can prove that h satisfies ∃xφ1(x). (2.2.3) Suppose that there is no occurrence of  or
or  in plane k. Then there is an occurrence of
in plane k. Then there is an occurrence of  in plane k. (2.2.3.1) Suppose that there is no occurrence of
in plane k. (2.2.3.1) Suppose that there is no occurrence of  in plane k. Since h has the properties (V) and (I),
in plane k. Since h has the properties (V) and (I),  and
and  alternately occur in plane k, or plane k is one of the other three cases besides all-
alternately occur in plane k, or plane k is one of the other three cases besides all-  shown in the property (V). So h satisfies φ3(a0,ω×(k− 1)), ∃xφ4(x) or \(\varphi _{5}(\overline {k})\). (2.2.3.2) Suppose that there is an occurrence of
shown in the property (V). So h satisfies φ3(a0,ω×(k− 1)), ∃xφ4(x) or \(\varphi _{5}(\overline {k})\). (2.2.3.2) Suppose that there is an occurrence of  in plane k. Since h has the property (III),
in plane k. Since h has the property (III),  must occur after
must occur after  and
and  . Suppose that the earliest occurrence of
. Suppose that the earliest occurrence of  is in line 1 of plane k. Line 0 of plane k can only be
is in line 1 of plane k. Line 0 of plane k can only be  , and so h satisfies φ2(a0,ω×(k− 1)+ 1). Suppose that the earliest occurrence of
, and so h satisfies φ2(a0,ω×(k− 1)+ 1). Suppose that the earliest occurrence of  is after line 1 of plane k. Since h has the properties (V) and (I), in plane k,
is after line 1 of plane k. Since h has the properties (V) and (I), in plane k,  and
and  alternately occur before the earliest occurrence of
alternately occur before the earliest occurrence of  . So h satisfies ∃xφ1(x) or ∃xφ2(x).
. So h satisfies ∃xφ1(x) or ∃xφ2(x).
In conclusion, Claim 6 holds.
Claim 7. For every classical hypothesis h, if h satisfies φc ∧ φothers, then h does not have at least one of the six properties (I)–(VI).
By Claim 6 and the definition of φothers, we can easily see that Claim 7 holds.
Claim 8. Let h0 be the empty hypothesis, i.e., h0(d) = n for every d ∈ D. Suppose that h is a maximally consistent classical hypothesis, and that h⊧φothers. Then \(h\not \ge {\sigma _{2}}_{{\mathscr{M}}}(h_{0})\).
The proof of Claim 8 is as follows. By Claim 7, h does not have at least one of the six properties (I)–(VI). It suffices to prove that no matter which of the six properties h does not have, we can find a sentence that is declared true by h and that is declared false by \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\). We prove this proposition in six cases.
-
(1)
Suppose that h does not have the property (I). Then there is at least one line such that the declared truth value of this line by h is different from
 ,
, 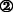 ,
,  ,
,  and
and  . Let line α be such a line. So there is a least natural number n such that the restriction to n of the declared truth value of line α by h is different from
. Let line α be such a line. So there is a least natural number n such that the restriction to n of the declared truth value of line α by h is different from  \( \upharpoonright n\),
\( \upharpoonright n\), 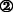 \( \upharpoonright n\),
\( \upharpoonright n\),  \( \upharpoonright n\),
\( \upharpoonright n\),  \( \upharpoonright n\) and
\( \upharpoonright n\) and  \( \upharpoonright n\). Define the sets S1 and S2, and sentence ϕ as follows:
$$ \begin{array}{@{}rcl@{}} &&S_{1}={~}_{df}\{ A_{m,\alpha} \mid m\le n, h (A_{m,\alpha} )=\mathbf{t} \}\\ &&S_{2}={~}_{df}\{ A_{m,\alpha} \mid m\le n,h (A_{m,\alpha} )={\mathbf{f}} \}\\ &&\phi={~}_{df}\bigwedge (S_{1}\cup \{\neg A_{m,\alpha} \mid A_{m,\alpha} \in S_{2}\}) \end{array} $$
\( \upharpoonright n\). Define the sets S1 and S2, and sentence ϕ as follows:
$$ \begin{array}{@{}rcl@{}} &&S_{1}={~}_{df}\{ A_{m,\alpha} \mid m\le n, h (A_{m,\alpha} )=\mathbf{t} \}\\ &&S_{2}={~}_{df}\{ A_{m,\alpha} \mid m\le n,h (A_{m,\alpha} )={\mathbf{f}} \}\\ &&\phi={~}_{df}\bigwedge (S_{1}\cup \{\neg A_{m,\alpha} \mid A_{m,\alpha} \in S_{2}\}) \end{array} $$Since h is maximally consistent, h declares ϕ true. By Claim 4 and the definitions of φ1(x), φ4(x) and φ6, we know that for any classical hypothesis \(h^{\prime }\), the restriction to n of the declared truth value of line α by \(\tau _{{\mathscr{M}}}(h^{\prime })\) is different from the restriction to n of the declared truth value of line α by h. So, it can not be the case that \(\tau _{{\mathscr{M}}}(h^{\prime })\) declares all sentences in S1 true and declares all sentences in S2 false. Therefore, it can not be the case that \(\tau _{{\mathscr{M}}}(h^{\prime })\) declares ϕ true. Hence \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})(\phi )={\mathbf {f}}\).
-
(2)
Suppose that h does not have the property (II). Then the total number of occurrences of
 or
or 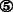 is greater than or equal to 2. (2.1) Suppose that both
is greater than or equal to 2. (2.1) Suppose that both  and
and  occur. Let line α be some line that is declared
occur. Let line α be some line that is declared  by h, and let line β be some line that is declared
by h, and let line β be some line that is declared 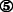 by h. Of course, α≠β. Let n be the least natural number such that the n th sentence of line α (i.e., An,α) is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let m be the least natural number such that the m th sentence of line β (i.e., Am,β) is declared false by h. Let B2 be the set consisting of the first m + 1 sentences of line β. We can represent B1 and B2 as follows:
by h. Of course, α≠β. Let n be the least natural number such that the n th sentence of line α (i.e., An,α) is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let m be the least natural number such that the m th sentence of line β (i.e., Am,β) is declared false by h. Let B2 be the set consisting of the first m + 1 sentences of line β. We can represent B1 and B2 as follows:

Define the sets S1 and S2, and sentence ϕ as follows:
$$ \begin{array}{@{}rcl@{}} &&S_{1}={~}_{df}\{ A_{m,\alpha} \mid A_{m,\alpha} \in B_{1} \cup B_{2}, h (A_{m,\alpha} )=\mathbf{t} \}\\ &&S_{2}={~}_{df}\{ A_{m,\alpha} \mid A_{m,\alpha} \in B_{1} \cup B_{2},h (A_{m,\alpha} )={\mathbf{f}} \}\\ &&\phi={~}_{df}\bigwedge (S_{1}\cup \{\neg A_{m,\alpha} \mid A_{m,\alpha} \in S_{2}\}) \end{array} $$Since h is maximally consistent, h declares ϕ true. By Claim 4 and the definitions of φ1(x), φ4(x) and φ6, we know that for any classical hypothesis \(h^{\prime }\), it can not be the case that \(\tau _{{\mathscr{M}}}(h^{\prime })\) declares all sentences in S1 true and declares all sentences in S2 false. So \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})(\phi )={\mathbf {f}}\). (2.2) Suppose that the number of occurrences of
 is greater than or equal to 2. Choose two lines that are declared
is greater than or equal to 2. Choose two lines that are declared  by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. (2.3) Suppose that the number of occurrences of
by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. (2.3) Suppose that the number of occurrences of 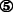 is greater than or equal to 2. Choose two lines that are declared
is greater than or equal to 2. Choose two lines that are declared 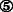 by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared false by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared false by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. -
(3)
Suppose that h does not have the property (III). Then there is a line such that this line is
 and there is a line after this line such that it is
and there is a line after this line such that it is  ,
,  ,
,  or
or 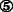 . Let line α be such a line. And let line β be a line after line α that is
. Let line α be such a line. And let line β be a line after line α that is  ,
, 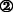 ,
,  or
or 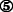 . Of course, α < β. Let B1 be the set consisting of the first two sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
. Of course, α < β. Let B1 be the set consisting of the first two sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. -
(4)
Suppose that h does not have the property (IV). Then there are three possibilities: (4.1) there is a line such that this line is
 or
or 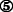 and there is a line after this line such that it is
and there is a line after this line such that it is  or
or  ; (4.2) there is a line that is
; (4.2) there is a line that is  , but its previous line is not
, but its previous line is not  ; (4.3) there is a line that is
; (4.3) there is a line that is 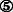 , but its previous line is not
, but its previous line is not  . In case (4.1), let line α be a line that is
. In case (4.1), let line α be a line that is  or
or  and such that there is a line after line α that is
and such that there is a line after line α that is  or
or  . And let line β be a line that is after line α and that is
. And let line β be a line that is after line α and that is  or
or  . α < β. Let n be the greatest natural number such that the first n sentences of line α are declared the same truth value by h. Let B1 be the set consisting of An− 1,α and An,α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. In case (4.2), because of case (2) and case (3), we only need to consider the following case: there is a line that is
. α < β. Let n be the greatest natural number such that the first n sentences of line α are declared the same truth value by h. Let B1 be the set consisting of An− 1,α and An,α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. In case (4.2), because of case (2) and case (3), we only need to consider the following case: there is a line that is  , but its previous line is
, but its previous line is  . Let line α + 1 be such a line. Its previous line, i.e., line α, is
. Let line α + 1 be such a line. Its previous line, i.e., line α, is 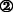 . Define sets B1 and B2 as follows:
. Define sets B1 and B2 as follows:
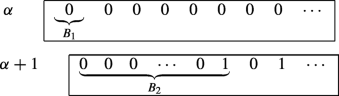
Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false. In case (4.3), because of case (2) and case (3), we only need to consider the following case: there is a line that is
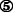 , but its previous line is
, but its previous line is  . Let line α + 1 be such a line. Its previous line, i.e., line α, is
. Let line α + 1 be such a line. Its previous line, i.e., line α, is  . Define sets B1 and B2 as follows:
. Define sets B1 and B2 as follows:
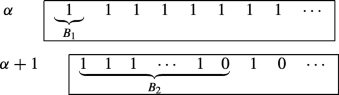
Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
-
(5)
Suppose that h does not have the property (V). Then there is a plane such that there are successive occurrences of
 or successive occurrences of
or successive occurrences of 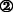 in this plane and this plane is not one of the four cases shown in the property (V). Let plane k be such a plane. (5.1) Suppose that there are successive occurrences of
in this plane and this plane is not one of the four cases shown in the property (V). Let plane k be such a plane. (5.1) Suppose that there are successive occurrences of  in plane k. Obviously, plane k is not one of the following two cases:
in plane k. Obviously, plane k is not one of the following two cases:
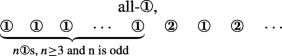
Choose two adjacent lines in plane k that are declared
 by h. Let them be line α and line α + 1 respectively. Then ω × (k − 1) ≤ α < ω × k. We consider in four cases: (5.1.1) in plane k, there is an occurrence of
by h. Let them be line α and line α + 1 respectively. Then ω × (k − 1) ≤ α < ω × k. We consider in four cases: (5.1.1) in plane k, there is an occurrence of  ,
,  or
or  ; (5.1.2) in plane k, there is no occurrence of
; (5.1.2) in plane k, there is no occurrence of  ,
,  or
or  , and there is a line before line α that is
, and there is a line before line α that is 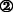 ; (5.1.3) in plane k, there is no occurrence of
; (5.1.3) in plane k, there is no occurrence of  ,
,  or
or 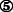 , and all lines before line α are
, and all lines before line α are  , and there are successive occurrences of
, and there are successive occurrences of 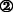 after line α + 1; (5.1.4) in plane k, there is no occurrence of
after line α + 1; (5.1.4) in plane k, there is no occurrence of  ,
,  or
or 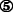 , and all lines before line α are
, and all lines before line α are  , and there are no successive occurrences of
, and there are no successive occurrences of 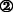 after line α + 1.
after line α + 1.In case (5.1.1), because of case (3) and case (4), we only need to consider the following case: in plane k, there is an occurrence of
 ,
,  or
or 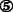 after line α + 1. Let line β be a line in plane k that is
after line α + 1. Let line β be a line in plane k that is  ,
,  or
or  and that is after line α + 1. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of the first two sentences of line α + 1. Choose a sentence in line β that is declared true by h, and another sentence in line β that is declared false by h. Let B3 be the set consisting of these two sentences. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
and that is after line α + 1. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of the first two sentences of line α + 1. Choose a sentence in line β that is declared true by h, and another sentence in line β that is declared false by h. Let B3 be the set consisting of these two sentences. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.In case (5.1.2), let line β be a line in plane k that is
 and that is before line α. Then ω × (k − 1) ≤ β < α. Let B1 be the set consisting of A0,β. Let B2 be the set consisting of A0,α. Let B3 be the set consisting of the first two sentences of line α + 1. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
and that is before line α. Then ω × (k − 1) ≤ β < α. Let B1 be the set consisting of A0,β. Let B2 be the set consisting of A0,α. Let B3 be the set consisting of the first two sentences of line α + 1. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.In case (5.1.3), let line β and line β + 1 be lines in plane k that are declared
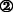 by h. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Let B4 be the set consisting of A0,β+ 1. Similar to (2.1), B1, B2, B3 and B4 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
by h. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Let B4 be the set consisting of A0,β+ 1. Similar to (2.1), B1, B2, B3 and B4 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.In case (5.1.4), there is a unique line in plane k such that, in plane k, all lines before it are
 , and it is
, and it is 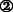 , and in plane k, starting with this line,
, and in plane k, starting with this line,  and
and  alternately occur. Let line β be this line. Then α + 1 < β < ω × k. Since h does not have the property (V), there is not an odd number n greater than or equal to 3 such that β = ω × (k − 1) + n. Then there is an even number n such that β = ω × (k − 1) + n. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
alternately occur. Let line β be this line. Then α + 1 < β < ω × k. Since h does not have the property (V), there is not an odd number n greater than or equal to 3 such that β = ω × (k − 1) + n. Then there is an even number n such that β = ω × (k − 1) + n. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.(5.2) Suppose that there are successive occurrences of
 in plane k. The proof is similar to (5.1).
in plane k. The proof is similar to (5.1). -
(6)
Suppose that h does not have the property (VI). Then there is a plane that is not all-
 and its previous plane is not all-
and its previous plane is not all-  . Let plane k be such a plane. Then k ∈{2, 3}. Since plane k is not all-
. Let plane k be such a plane. Then k ∈{2, 3}. Since plane k is not all-  , then there is a sentence in plane k such that either its abscissa is even and it is declared false by h, or its abscissa is odd and it is declared true by h. Choose such a sentence, and let B1 be the set consisting of it. Since plane k − 1 is not all-
, then there is a sentence in plane k such that either its abscissa is even and it is declared false by h, or its abscissa is odd and it is declared true by h. Choose such a sentence, and let B1 be the set consisting of it. Since plane k − 1 is not all-  , then there is a sentence in plane k − 1 that is declared true by h. Choose such a sentence, and let B2 be the set consisting of it. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
, then there is a sentence in plane k − 1 that is declared true by h. Choose such a sentence, and let B2 be the set consisting of it. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that \({\sigma _{2}}_{{\mathscr{M}}}(h_{0})\) declares false.
In conclusion, Claim 8 holds.
Claim 9. \({\mathbf {T}}^{lfp,\sigma _{2}}\) dictates that truth behaves like a classical concept in \({\mathscr{M}}\).
The proof of Claim 9 is as follows. Let h0 be the empty hypothesis. Let \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})(\alpha \in On)\) be the unique ordinal-length sequence of hypotheses obtained in the construction of the least fixed point of \({\sigma _{2}}_{{\mathscr{M}}}\) according to Kripke’s inductive construction, beginning with h0. To be specific, \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})(\alpha \in On)\) is defined as follows: if α = 0, then \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})=h_{0}\); if α = β + 1, then \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})={\sigma _{2}}_{{\mathscr{M}}}({\sigma _{2}}_{{\mathscr{M}}}^{\beta }(h_{0}))\); and if α is a limit ordinal, then \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})=\bigcup _{\beta < \alpha }{\sigma _{2}}_{{\mathscr{M}}}^{\beta }(h_{0})\). We know that for all ordinals α and β, if β ≤ α, then \({\sigma _{2}}_{{\mathscr{M}}}^{\beta }(h_{0})\le {\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})\); that for every ordinal α, \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})\) is strongly consistent; and that for every ordinal α, there is a maximally consistent classical hypothesis such that it is greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\alpha }(h_{0})\).
By the claims above, the following propositions hold.
-
(1)
By Claim 8, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{1}(h_{0})\) does not satisfy φothers.
-
(2)
By (1), Claim 2 and Claim 1, every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{1}(h_{0})\) satisfies φ1(an,α), φ2(a0,β)(β≠ 0,ω, 2ω), φ3(a0,γ)(γ = 0,ω, 2ω), φ4(a0,λ), \(\varphi _{5}(\overline {k})(k=1,2,3)\) or φ6.
-
(3)
By (2) and Claim 3 (and the definitions of φ1(x), φ4(x) and φ6), for every maximally consistent classical hypothesis \(h\ge {\sigma _{2}}_{{\mathscr{M}}}^{1}(h_{0}) \), \(\tau _{{\mathscr{M}}}(h)(A_{0,0})=\tau _{{\mathscr{M}}}(h)(A_{1,0})\), so \(h\models A_{0,0}\leftrightarrow A_{1,0}\). Hence \({\sigma _{2}}_{{\mathscr{M}}}^{2}(h_{0})(A_{0,0}\leftrightarrow A_{1,0})=\mathbf {t}\). But every maximally consistent classical hypothesis that satisfies φ1(a0,0) declares \(A_{0,0}\leftrightarrow A_{1,0}\) false. Therefore, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{2}(h_{0})\) does not satisfy φ1(a0,0).
-
(4)
By (2), (3) and Claim 3, for every maximally consistent classical hypothesis \(h\ge {\sigma _{2}}_{{\mathscr{M}}}^{2}(h_{0}) \), \(h\models A_{1,0}\leftrightarrow A_{2,0}\). Hence \({\sigma _{2}}_{{\mathscr{M}}}^{3}(h_{0})(A_{1,0}\leftrightarrow A_{2,0})=\mathbf {t}\). Therefore, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{3}(h_{0})\) does not satisfy φ1(a1,0).
-
(5)
Similar to (4), for every natural number n ≥ 2, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{n+2}(h_{0})\) does not satisfy φ1(an,0).
-
(6)
By (2)–(5), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega }(h_{0})\) satisfies φ1(an,α)(α ≥ 1), φ2(a0,β)(β≠ 0,ω, 2ω), φ3(a0,γ)(γ = 0,ω, 2ω), φ4(a0,λ), \(\varphi _{5}(\overline {k})(k=1,2,3)\) or φ6.
-
(7)
By (6) and Claim 3, for every maximally consistent classical hypothesis \(h\ge {\sigma _{2}}_{{\mathscr{M}}}^{\omega }(h_{0}) \), \(h\models A_{0,1}\leftrightarrow A_{1,1}\). So \({\sigma _{2}}_{{\mathscr{M}}}^{\omega +1}(h_{0})(A_{0,1}\leftrightarrow A_{1,1})=\mathbf {t}\). Hence, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega +1}(h_{0})\) satisfies neither φ1(a0,1) nor φ2(a0,1).
-
(8)
Similar to (4)–(5), for every natural number n ≥ 1, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega +n+1}(h_{0})\) does not satisfy φ1(an,1).
-
(9)
By (6)–(8), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{2\omega }(h_{0})\) satisfies φ1(an,α)(α ≥ 2), φ2(a0,β)(β ≥ 2,β≠ω, 2ω), φ3(a0,γ)(γ = 0,ω, 2ω), φ4(a0,λ), \(\varphi _{5}(\overline {k})(k=1,2,3)\) or φ6.
-
(10)
Similar to (7)–(9), for every natural number m ≥ 2 and every natural number i, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega \times m+1}(h_{0})\) does not satisfy φ2(a0,m); any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega \times m+i+1}(h_{0})\) does not satisfy φ1(ai,m); and every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega \times (m+1)}(h_{0})\) satisfies φ1(an,α)(α ≥ m + 1), φ2(a0,β)(β ≥ m + 1,β≠ω, 2ω), φ3(a0,γ)(γ = 0,ω, 2ω), φ4(a0,λ), \(\varphi _{5}(\overline {k})(k=1,2,3)\) or φ6.
-
(11)
By (10), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}}(h_{0})\) satisfies φ1(an,α)(α ≥ ω), φ2(a0,β)(β > ω,β≠ 2ω), φ3(a0,γ)(γ = 0,ω, 2ω), φ4(a0,λ), \(\varphi _{5}(\overline {k})(k=1,2,3)\) or φ6.
-
(12)
By (11) and Claim 3, \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+1}(h_{0})(A_{0,0}\leftrightarrow A_{0,1})=\mathbf {t}\), so any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+1}(h_{0})\) satisfies neither φ3(a0,0) nor φ4(a0,0).
-
(13)
Similar to (12), for every natural number n ≥ 1, \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+n+1}(h_{0})(A_{0,n}\leftrightarrow A_{0,n+1})=\mathbf {t}\), so any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+n+1}(h_{0})\) does not satisfy φ4(a0,n).
-
(14)
By (11)–(13), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+\omega }(h_{0})\) satisfies φ1(an,α)(α ≥ ω), φ2(a0,β)(β > ω,β≠ 2ω), φ3(a0,γ)(γ = ω, 2ω), φ4(a0,λ)(λ ≥ ω), \(\varphi _{5}(\overline {k})(k=1,2,3)\) or φ6.
-
(15)
By (14) and Claim 3, for every maximally consistent classical hypothesis \(h\ge {\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+\omega }(h_{0}) \), \(h\models A_{0,\omega }\leftrightarrow A_{1,\omega }\). So any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+\omega +1}(h_{0})\) satisfies neither \(\varphi _{5}(\overline {1})\) nor φ1(a0,ω).
-
(16)
Similar to (4)–(5), for every natural number n ≥ 1, any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+\omega +n+1}(h_{0})\) does not satisfy φ1(an,ω).
-
(17)
By (14)–(16), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+\omega +\omega }(h_{0})\), i.e., \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+2\omega }(h_{0})\), satisfies φ1(an,α)(α ≥ ω + 1), φ2(a0,β)(β > ω,β≠ 2ω), φ3(a0,γ)(γ = ω, 2ω), φ4(a0,λ)(λ ≥ ω), \(\varphi _{5}(\overline {k})(k=2,3)\) or φ6.
-
(18)
Similar to (7)–(11), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{\omega ^{2}+\omega +\omega ^{2}}(h_{0})\), i.e., \({\sigma _{2}}_{{\mathscr{M}}}^{2\omega ^{2}}(h_{0})\), satisfies φ1(an,α)(α ≥ 2ω), φ2(a0,β)(β > 2ω), φ3(a0,γ)(γ = ω, 2ω), φ4(a0,λ)(λ ≥ ω), \(\varphi _{5}(\overline {k})(k=2,3)\) or φ6.
-
(19)
Similar to (12)–(14), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{2\omega ^{2}+\omega }(h_{0})\) satisfies φ1(an,α)(α ≥ 2ω), φ2(a0,β)(β > 2ω), φ3(a0,2ω), φ4(a0,λ)(λ ≥ 2ω), \(\varphi _{5}(\overline {k})(k=2,3)\) or φ6.
-
(20)
Similar to (15), any maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{2\omega ^{2}+\omega +1}(h_{0})\) satisfies neither \(\varphi _{5}(\overline {2})\) nor φ1(a0,2ω).
-
(21)
Similar to (16)–(18), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{2\omega ^{2}+\omega +\omega ^{2}}(h_{0})\), i.e., \({\sigma _{2}}_{{\mathscr{M}}}^{3\omega ^{2}}(h_{0})\), satisfies φ3(a0,2ω), φ4(a0,λ)(λ ≥ 2ω), \(\varphi _{5}(\overline {3})\) or φ6.
-
(22)
Similar to (19), every maximally consistent classical hypothesis greater than or equal to \({\sigma _{2}}_{{\mathscr{M}}}^{3\omega ^{2}+\omega }(h_{0})\) satisfies \(\varphi _{5}(\overline {3})\) or φ6.
-
(23)
By (22) and Claim 3, for any two sentences An,α and Am,β in I(S), \({\sigma _{2}}_{{\mathscr{M}}}^{3\omega ^{2}+\omega +1}(h_{0})(A_{n,\alpha })={\mathbf {f}}\) and \({\sigma _{2}}_{{\mathscr{M}}}^{3\omega ^{2}+\omega +1}(h_{0})(A_{n,\alpha }\leftrightarrow A_{m,\beta })=\mathbf {t}\).
Hence for any two sentences An,α and Am,β in I(S), \(lfp({\sigma _{2}}_{{\mathscr{M}}})(A_{n,\alpha })={\mathbf {f}}\) and \(lfp({\sigma _{2}}_{{\mathscr{M}}})(A_{n,\alpha }\leftrightarrow A_{m,\beta })=\mathbf {t}\). Let Y be the set \(I(S)\cup \{A_{n,\alpha }\leftrightarrow A_{m,\beta }\mid A_{n,\alpha }, A_{m,\beta }\in I(S) \}\). Then \(Y \subseteq \{ A \in Sent({{\mathscr{L}}}^{+})\mid A\in {\mathbf {V}}_{{\mathscr{M}}}^{lfp,\sigma _{2}}\) or \(\neg A\in {\mathbf {V}}_{{\mathscr{M}}}^{lfp,\sigma _{2}}\}\). \({\mathscr{M}}\) is \((Sent({{\mathscr{L}}}^{+})\setminus Y)\)-neutral. By Theorem 4, we know \({\mathbf {T}}^{lfp,\sigma _{2}} \le _3{\mathbf {T}}^{lfp,\sigma _{2}} \). So \({\mathbf {T}}^{lfp,\sigma _{2}} \) dictates that truth behaves like a classical concept in \({\mathscr{M}}\).
Let \({\mathscr{S}}\) be a sequence of classical hypotheses, and \(lh({\mathscr{S}})\) be a limit ordinal or the class of all ordinals On. We say that line β is stably  in \({\mathscr{S}}\) iff, the sentences in line β with even abscissas are stably true in \({\mathscr{S}}\), and the sentences in line β with odd abscissas are stably false in \({\mathscr{S}}\). We say that line β is unstable in \({\mathscr{S}}\) iff each sentence in line β is unstable in \({\mathscr{S}}\). For every limit ordinal \(\alpha \le lh({\mathscr{S}})\), we say that line β is unstable up to α in \({\mathscr{S}}\) iff each sentence in line β is unstable up to α in \({\mathscr{S}}\). We say that plane k is stably all-
in \({\mathscr{S}}\) iff, the sentences in line β with even abscissas are stably true in \({\mathscr{S}}\), and the sentences in line β with odd abscissas are stably false in \({\mathscr{S}}\). We say that line β is unstable in \({\mathscr{S}}\) iff each sentence in line β is unstable in \({\mathscr{S}}\). For every limit ordinal \(\alpha \le lh({\mathscr{S}})\), we say that line β is unstable up to α in \({\mathscr{S}}\) iff each sentence in line β is unstable up to α in \({\mathscr{S}}\). We say that plane k is stably all-  in \({\mathscr{S}}\) iff, the sentences in plane k with even abscissas are stably true in \({\mathscr{S}}\), and the sentences in plane k with odd abscissas are stably false in \({\mathscr{S}}\). We say that plane k is unstable in \({\mathscr{S}}\) iff each sentence in plane k is unstable in \({\mathscr{S}}\). For every limit ordinal \(\alpha \le lh({\mathscr{S}})\), we say that plane k is unstable up to α in \({\mathscr{S}}\) iff each sentence in plane k is unstable up to α in \({\mathscr{S}}\).
in \({\mathscr{S}}\) iff, the sentences in plane k with even abscissas are stably true in \({\mathscr{S}}\), and the sentences in plane k with odd abscissas are stably false in \({\mathscr{S}}\). We say that plane k is unstable in \({\mathscr{S}}\) iff each sentence in plane k is unstable in \({\mathscr{S}}\). For every limit ordinal \(\alpha \le lh({\mathscr{S}})\), we say that plane k is unstable up to α in \({\mathscr{S}}\) iff each sentence in plane k is unstable up to α in \({\mathscr{S}}\).
Claim 10. Let λ be a limit ordinal, and \({\mathscr{S}}\) be a λ-length revision sequence.Footnote 20 Suppose that there is no hypothesis in \({\mathscr{S}}\) that satisfies φc ∧ φ6. Then if there is a sentence An,α ∈ I(S) such that An,α is stable in \({\mathscr{S}}\) and all planes after An,α are stably all-  in \({\mathscr{S}}\), then α ≥ 1, and line α and all lines after line α are stably
in \({\mathscr{S}}\), then α ≥ 1, and line α and all lines after line α are stably  in \({\mathscr{S}}\).
in \({\mathscr{S}}\).
The proof of Claim 10 is as follows. Let δ be the supremum of the set {β∣An,α or some sentence in a plane after An,α is stable in \({\mathscr{S}}\) from stage β}. Let γ = δ + 1. Note that \({\mathscr{S}}_{\gamma }\) does not satisfy φc ∧ φ6. By Claim 3 and Claim 4, α ≥ 1 and \({\mathscr{S}}_{\gamma }\) satisfies φ1(ap,𝜖)(p ≥ 1,𝜖 < α), φ1(a0,0) or φ4(a0,ε)(ε < ω × (fω(An,α) − 1), ε≠ 0,ω), otherwise the declared truth value of An,α would change after a finite number of applications of \(\tau _{{\mathscr{M}}}\) to \({\mathscr{S}}_{\gamma }\).Footnote 21 Next, we prove by transfinite induction that for every γ ≤ β < λ, \({\mathscr{S}}_{\beta }\) declares line α and all lines after line α  . For β = γ, the proposition holds, because \({\mathscr{S}}_{\gamma }\) satisfies φ1(ap,𝜖)(p ≥ 1,𝜖 < α), φ1(a0,0) or φ4(a0,ε)(ε < ω × (fω(An,α) − 1), ε≠ 0,ω). The argument for the successor case is similar to the argument for the case β = γ. The limit case is trivial.
. For β = γ, the proposition holds, because \({\mathscr{S}}_{\gamma }\) satisfies φ1(ap,𝜖)(p ≥ 1,𝜖 < α), φ1(a0,0) or φ4(a0,ε)(ε < ω × (fω(An,α) − 1), ε≠ 0,ω). The argument for the successor case is similar to the argument for the case β = γ. The limit case is trivial.
Claim 11. Let λ be a limit ordinal, and \({\mathscr{S}}\) be a λ-length revision sequence. Suppose that there is no hypothesis in \({\mathscr{S}}\) that satisfies φc ∧ φ6. Let δ be a limit ordinal such that δ > λ. Let \(X\subseteq Sent({{\mathscr{L}}}^{+})\). Basing on \({\mathscr{S}}\) and using X as the bootstrapper in the interval [λ,δ), we get a unique δ-length revision sequence \({\mathscr{S}}^{\prime }\). Suppose further that X declares plane k neither all-  nor all-
nor all-  ,Footnote 22 and that all planes after plane k are stably all-
,Footnote 22 and that all planes after plane k are stably all-  in \({\mathscr{S}}\). Then in the interval [λ,δ), plane k would be declared neither all-
in \({\mathscr{S}}\). Then in the interval [λ,δ), plane k would be declared neither all-  nor all-
nor all-  , and the declared truth value of each of the planes after plane k would not change. I.e., for every λ ≤ β < δ, \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-
, and the declared truth value of each of the planes after plane k would not change. I.e., for every λ ≤ β < δ, \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-  nor all-
nor all-  , and \({\mathscr{S}}^{\prime }_{\beta }\) declares all planes after plane k all-
, and \({\mathscr{S}}^{\prime }_{\beta }\) declares all planes after plane k all-  .
.
The proof of Claim 11 is as follows. We prove by transfinite induction that for every λ ≤ β < δ, (1) \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-  nor all-
nor all-  ; and (2) \({\mathscr{S}}^{\prime }_{\beta }\) declares all planes after plane k all-
; and (2) \({\mathscr{S}}^{\prime }_{\beta }\) declares all planes after plane k all-  . For β = γ, (2) holds trivially. If there is some sentence An,α in plane k such that An,α is stable in \({\mathscr{S}}\), then by Claim 10, line α is stably
. For β = γ, (2) holds trivially. If there is some sentence An,α in plane k such that An,α is stable in \({\mathscr{S}}\), then by Claim 10, line α is stably  in \({\mathscr{S}}\). Obviously, \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-
in \({\mathscr{S}}\). Obviously, \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-  nor all-
nor all-  . Assume that there is no sentence in plane k that is stable in \({\mathscr{S}}\). Since X declares plane k neither all-
. Assume that there is no sentence in plane k that is stable in \({\mathscr{S}}\). Since X declares plane k neither all-  nor all-
nor all-  , \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-
, \({\mathscr{S}}^{\prime }_{\beta }\) declares plane k neither all-  nor all-
nor all-  , too. So (1) holds for β. Let β be a successor ordinal. By the induction hypothesis and Claim 3, (1) and (2) hold for β. The argument for the limit case is similar to the argument for the case β = γ.
, too. So (1) holds for β. Let β be a successor ordinal. By the induction hypothesis and Claim 3, (1) and (2) hold for β. The argument for the limit case is similar to the argument for the case β = γ.
Claim 12. Let λ be a limit ordinal, and \({\mathscr{S}}\) be a λ-length revision sequence. Let δ be a limit ordinal such that δ > λ. Let \(X\subseteq Sent({{\mathscr{L}}}^{+})\). Basing on \({\mathscr{S}}\) and using X as the bootstrapper in the interval [λ,δ), we get a unique δ-length revision sequence \({\mathscr{S}}^{\prime }\). Suppose further that X declares plane k either all-  or all-
or all-  , where k = 2 or 3, and that plane k and the plane after plane k (if exists) are stably all-
, where k = 2 or 3, and that plane k and the plane after plane k (if exists) are stably all-  in \({\mathscr{S}}\). Then the declared truth value of each of line ω × (k − 1) + 2 and the lines after line ω × (k − 1) + 2 would not change. I.e., for every λ ≤ β < δ, \({\mathscr{S}}^{\prime }_{\beta }\) declares line ω × (k − 1) + 2 and all lines after line ω × (k − 1) + 2
in \({\mathscr{S}}\). Then the declared truth value of each of line ω × (k − 1) + 2 and the lines after line ω × (k − 1) + 2 would not change. I.e., for every λ ≤ β < δ, \({\mathscr{S}}^{\prime }_{\beta }\) declares line ω × (k − 1) + 2 and all lines after line ω × (k − 1) + 2  .
.
The proof of Claim 12 is as follows. We prove by transfinite induction that for every λ ≤ β < δ, (1) it is not the case that \({\mathscr{S}}^{\prime }_{\beta }\) declares one of line ω × (k − 1) and line ω × (k − 1) + 1  , and the other
, and the other  ; and (2) \({\mathscr{S}}^{\prime }_{\beta }\) declares line ω × (k − 1) + 2 and all lines after line ω × (k − 1) + 2
; and (2) \({\mathscr{S}}^{\prime }_{\beta }\) declares line ω × (k − 1) + 2 and all lines after line ω × (k − 1) + 2  . The case β = γ is trivial. Let β = 𝜖 + 1. By the induction hypothesis, \({\mathscr{S}}^{\prime }_{\epsilon }\) satisfies φc ∧ φ1(an,α)(α ≤ ω × (k − 1) + 1), φc ∧ φ2(a0,ε)(ε ≤ ω × (k − 1) + 1,ε≠ 0,ω, 2ω), φc ∧ φ3(a0,ζ)(ζ < ω × (k − 1), ζ = 0,ω), φc ∧ φ4(a0,η)(η < ω × (k − 1)), \(\varphi _{c}\land \varphi _{5}(\overline {i})(i<k,i=1,2)\) or φothers. Then by Claim 3, \({\mathscr{S}}^{\prime }_{\beta }\) satisfies φ1(an+ 1,α), φ1(a1,ε), φ4(a0,ζ+ 1), φ4(a0,η+ 1), φ1(a1,ω×i) or φ1(a0,0). Hence the proposition holds for β. Let β be a limit ordinal. By the induction hypothesis, (2) holds for β. By the induction hypothesis, there is no hypothesis in \({\mathscr{S}}^{\prime } \upharpoonright \beta \) that satisfies φc ∧ φ6. By Claim 10, in \({\mathscr{S}}^{\prime } \upharpoonright \beta \), there are three possibilities: line ω × (k − 1) and line ω × (k − 1) + 1 are unstable; line ω × (k − 1) is unstable and line ω × (k − 1) + 1 is stably
. The case β = γ is trivial. Let β = 𝜖 + 1. By the induction hypothesis, \({\mathscr{S}}^{\prime }_{\epsilon }\) satisfies φc ∧ φ1(an,α)(α ≤ ω × (k − 1) + 1), φc ∧ φ2(a0,ε)(ε ≤ ω × (k − 1) + 1,ε≠ 0,ω, 2ω), φc ∧ φ3(a0,ζ)(ζ < ω × (k − 1), ζ = 0,ω), φc ∧ φ4(a0,η)(η < ω × (k − 1)), \(\varphi _{c}\land \varphi _{5}(\overline {i})(i<k,i=1,2)\) or φothers. Then by Claim 3, \({\mathscr{S}}^{\prime }_{\beta }\) satisfies φ1(an+ 1,α), φ1(a1,ε), φ4(a0,ζ+ 1), φ4(a0,η+ 1), φ1(a1,ω×i) or φ1(a0,0). Hence the proposition holds for β. Let β be a limit ordinal. By the induction hypothesis, (2) holds for β. By the induction hypothesis, there is no hypothesis in \({\mathscr{S}}^{\prime } \upharpoonright \beta \) that satisfies φc ∧ φ6. By Claim 10, in \({\mathscr{S}}^{\prime } \upharpoonright \beta \), there are three possibilities: line ω × (k − 1) and line ω × (k − 1) + 1 are unstable; line ω × (k − 1) is unstable and line ω × (k − 1) + 1 is stably  ; and line ω × (k − 1) and line ω × (k − 1) + 1 are stably
; and line ω × (k − 1) and line ω × (k − 1) + 1 are stably  . Note that X declares plane k either all-
. Note that X declares plane k either all-  or all-
or all-  . So in each case, (1) holds for β.
. So in each case, (1) holds for β.
Claim 13. Let λ be a limit ordinal, and \({\mathscr{S}}\) be a λ-length revision sequence. Suppose that plane 2 and plane 3 are stably all-  in \({\mathscr{S}}\). Let δ be the least cardinal that is larger than \( \arrowvert \mathcal {P}(Sent({{\mathscr{L}}}^{+})) \arrowvert \). Let \(X\subseteq Sent({{\mathscr{L}}}^{+})\). Basing on \({\mathscr{S}}\) and using X as the bootstrapper in the interval [λ,λ + δ), we get a unique (λ + δ)-length revision sequence \({\mathscr{S}}^{\prime }\). Suppose further that X declares plane 1 either all-
in \({\mathscr{S}}\). Let δ be the least cardinal that is larger than \( \arrowvert \mathcal {P}(Sent({{\mathscr{L}}}^{+})) \arrowvert \). Let \(X\subseteq Sent({{\mathscr{L}}}^{+})\). Basing on \({\mathscr{S}}\) and using X as the bootstrapper in the interval [λ,λ + δ), we get a unique (λ + δ)-length revision sequence \({\mathscr{S}}^{\prime }\). Suppose further that X declares plane 1 either all-  or all-
or all-  . Then plane 2 is stably all-
. Then plane 2 is stably all-  in \({\mathscr{S}}^{\prime }\).
in \({\mathscr{S}}^{\prime }\).
The proof of Claim 13 is as follows. First, we prove that there is a hypothesis in the interval [λ,λ + δ) (of \({\mathscr{S}}^{\prime }\), of course) that satisfies φothers. We prove this by reduction to absurdity. Suppose that there is no hypothesis in the interval [λ,λ + δ) that satisfies φothers. In particular, \({\mathscr{S}}^{\prime }_{\lambda }\) does not satisfy φothers. (1) Suppose that there is some sentence in plane 1 that is stable in \({\mathscr{S}}\). Since \({\mathscr{S}}^{\prime }_{\lambda }\) does not satisfy φothers, by Claim 10 and the premise that X declares plane 1 either all-  or all-
or all-  , line 0 of plane 1 is unstable in \({\mathscr{S}}\), and other lines of plane 1 are stably
, line 0 of plane 1 is unstable in \({\mathscr{S}}\), and other lines of plane 1 are stably  in \({\mathscr{S}}\). Then \({\mathscr{S}}^{\prime }_{\lambda }\) satisfies φc ∧ φ1(a0,1) or φc ∧ φ2(a0,1). By Claim 3, \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) satisfies φothers, a contradiction. (2) Suppose that there is no sentence in plane 1 that is stable in \({\mathscr{S}}\). Then \({\mathscr{S}}^{\prime }_{\lambda }\) satisfies either φc ∧ φ1(a0,ω) or \(\varphi _{c}\land \varphi _{5}(\overline {1})\). By Claim 3, line 0 of plane 2 is unstable up to λ + ω in \({\mathscr{S}}^{\prime }\), and all sentences of the form \( A_{n,\omega }\leftrightarrow A_{m,\omega }\), where n,m ∈ ω, are stably true up to λ + ω in \({\mathscr{S}}^{\prime }\). By supposition \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) does not satisfy φothers. Then \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) must satisfy φc. Hence X declares line 0 of plane 2 either
in \({\mathscr{S}}\). Then \({\mathscr{S}}^{\prime }_{\lambda }\) satisfies φc ∧ φ1(a0,1) or φc ∧ φ2(a0,1). By Claim 3, \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) satisfies φothers, a contradiction. (2) Suppose that there is no sentence in plane 1 that is stable in \({\mathscr{S}}\). Then \({\mathscr{S}}^{\prime }_{\lambda }\) satisfies either φc ∧ φ1(a0,ω) or \(\varphi _{c}\land \varphi _{5}(\overline {1})\). By Claim 3, line 0 of plane 2 is unstable up to λ + ω in \({\mathscr{S}}^{\prime }\), and all sentences of the form \( A_{n,\omega }\leftrightarrow A_{m,\omega }\), where n,m ∈ ω, are stably true up to λ + ω in \({\mathscr{S}}^{\prime }\). By supposition \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) does not satisfy φothers. Then \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) must satisfy φc. Hence X declares line 0 of plane 2 either  or
or  , and \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) satisfies φc ∧ φ1(a0,ω+ 1) or φc ∧ φ2(a0,ω+ 1). By Claim 3, we know that: the first two lines of plane 2 are unstable up to λ + 2ω in \({\mathscr{S}}^{\prime }\); all sentences of the form \(A_{n,\alpha }\leftrightarrow A_{m,\alpha }\), where n,m ∈ ω and α = ω or ω + 1, are stably true up to λ + 2ω in \({\mathscr{S}}^{\prime }\); and all sentences of the form \( A_{p,\omega }\leftrightarrow A_{q,\omega +1}\), where p,q ∈ ω, are stably false up to λ + 2ω in \({\mathscr{S}}^{\prime }\). Since \({\mathscr{S}}^{\prime }_{\lambda +2\omega }\) does not satisfy φothers, X declares line 1 of plane 2 either
, and \({\mathscr{S}}^{\prime }_{\lambda +\omega }\) satisfies φc ∧ φ1(a0,ω+ 1) or φc ∧ φ2(a0,ω+ 1). By Claim 3, we know that: the first two lines of plane 2 are unstable up to λ + 2ω in \({\mathscr{S}}^{\prime }\); all sentences of the form \(A_{n,\alpha }\leftrightarrow A_{m,\alpha }\), where n,m ∈ ω and α = ω or ω + 1, are stably true up to λ + 2ω in \({\mathscr{S}}^{\prime }\); and all sentences of the form \( A_{p,\omega }\leftrightarrow A_{q,\omega +1}\), where p,q ∈ ω, are stably false up to λ + 2ω in \({\mathscr{S}}^{\prime }\). Since \({\mathscr{S}}^{\prime }_{\lambda +2\omega }\) does not satisfy φothers, X declares line 1 of plane 2 either  or
or  , and the declared truth value of line 1 of plane 2 by X is different from the declared truth value of line 0 of plane 2 by X, and \({\mathscr{S}}^{\prime }_{\lambda +2\omega }\) satisfies φc ∧ φ1(a0,ω+ 2) or φc ∧ φ2(a0,ω+ 2). Similarly, for every natural number t ≥ 2, X declares line t of plane 2 either
, and the declared truth value of line 1 of plane 2 by X is different from the declared truth value of line 0 of plane 2 by X, and \({\mathscr{S}}^{\prime }_{\lambda +2\omega }\) satisfies φc ∧ φ1(a0,ω+ 2) or φc ∧ φ2(a0,ω+ 2). Similarly, for every natural number t ≥ 2, X declares line t of plane 2 either  or
or  , and the declared truth value of line t of plane 2 by X is different from the declared truth value of line t − 1 of plane 2 by X, and \({\mathscr{S}}^{\prime }_{\lambda +\omega \times (t+1)}\) satisfies φc ∧ φ1(a0,ω+t+ 1) or φc ∧ φ2(a0,ω+t+ 1). So there are two possibilities: X declares all even lines of plane 2
, and the declared truth value of line t of plane 2 by X is different from the declared truth value of line t − 1 of plane 2 by X, and \({\mathscr{S}}^{\prime }_{\lambda +\omega \times (t+1)}\) satisfies φc ∧ φ1(a0,ω+t+ 1) or φc ∧ φ2(a0,ω+t+ 1). So there are two possibilities: X declares all even lines of plane 2  , and all odd lines of plane 2
, and all odd lines of plane 2  ; and X declares all even lines of plane 2
; and X declares all even lines of plane 2  , and all odd lines of plane 2
, and all odd lines of plane 2  .Footnote 23 Plane 2 is unstable up to λ + ω2 in \({\mathscr{S}}^{\prime }\). Since \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}}\) does not satisfy φothers, \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}}\) satisfies either φc ∧ φ3(a0,ω) or φc ∧ φ4(a0,ω). By Claim 3, plane 2 is unstable up to λ + ω2 + ω in \({\mathscr{S}}^{\prime }\); and the sentence \( A_{0,\omega }\leftrightarrow A_{0,\omega +1}\) is stably true up to λ + ω2 + ω in \({\mathscr{S}}^{\prime }\). Hence \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}+\omega }\) must not satisfy φc. Then \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}+\omega }\) satisfies φothers, a contradiction. We conclude that there is a hypothesis in the interval [λ,λ + δ) that satisfies φothers. Let \({\mathscr{S}}^{\prime }_{\gamma }\) be such a hypothesis (where λ ≤ γ < λ + δ).
.Footnote 23 Plane 2 is unstable up to λ + ω2 in \({\mathscr{S}}^{\prime }\). Since \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}}\) does not satisfy φothers, \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}}\) satisfies either φc ∧ φ3(a0,ω) or φc ∧ φ4(a0,ω). By Claim 3, plane 2 is unstable up to λ + ω2 + ω in \({\mathscr{S}}^{\prime }\); and the sentence \( A_{0,\omega }\leftrightarrow A_{0,\omega +1}\) is stably true up to λ + ω2 + ω in \({\mathscr{S}}^{\prime }\). Hence \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}+\omega }\) must not satisfy φc. Then \({\mathscr{S}}^{\prime }_{\lambda +\omega ^{2}+\omega }\) satisfies φothers, a contradiction. We conclude that there is a hypothesis in the interval [λ,λ + δ) that satisfies φothers. Let \({\mathscr{S}}^{\prime }_{\gamma }\) be such a hypothesis (where λ ≤ γ < λ + δ).
Next, we prove by transfinite induction that for every γ + 1 ≤ β < λ + δ, \({\mathscr{S}}^{\prime }_{\beta }\) declares line 2 and all lines after line 2  . Let β = γ + 1. Since \({\mathscr{S}}^{\prime }_{\gamma }\) satisfies φothers, by Claim 3, the proposition holds for β. The limit case is trivial. Let β = 𝜖 + 1. Suppose that 𝜖 is a limit ordinal. By Claim 10, in \({\mathscr{S}}^{\prime }\), there are two possibilities: line 0 and line 1 are unstable up to 𝜖; and line 0 is unstable up to 𝜖 and line 1 is stably
. Let β = γ + 1. Since \({\mathscr{S}}^{\prime }_{\gamma }\) satisfies φothers, by Claim 3, the proposition holds for β. The limit case is trivial. Let β = 𝜖 + 1. Suppose that 𝜖 is a limit ordinal. By Claim 10, in \({\mathscr{S}}^{\prime }\), there are two possibilities: line 0 and line 1 are unstable up to 𝜖; and line 0 is unstable up to 𝜖 and line 1 is stably  up to 𝜖. Hence \({\mathscr{S}}^{\prime }_{\epsilon }\) satisfies φc ∧ φ1(a0,1), φc ∧ φ2(a0,1) or φothers. By Claim 3, \({\mathscr{S}}^{\prime }_{\beta }\) satisfies either φ1(a1,1) or φ1(a0,0). So \({\mathscr{S}}^{\prime }_{\beta }\) declares line 2 and all lines after line 2
up to 𝜖. Hence \({\mathscr{S}}^{\prime }_{\epsilon }\) satisfies φc ∧ φ1(a0,1), φc ∧ φ2(a0,1) or φothers. By Claim 3, \({\mathscr{S}}^{\prime }_{\beta }\) satisfies either φ1(a1,1) or φ1(a0,0). So \({\mathscr{S}}^{\prime }_{\beta }\) declares line 2 and all lines after line 2  . Suppose that 𝜖 is a successor ordinal. By Claim 4 and the induction hypothesis, \({\mathscr{S}}^{\prime }_{\epsilon }\) satisfies either φ1(ap,q)(p ≥ 1,q = 0, 1) or φ1(a0,0). By Claim 3, \({\mathscr{S}}^{\prime }_{\beta }\) satisfies either φ1(ap+ 1,q) or φ1(a1,0). So \({\mathscr{S}}^{\prime }_{\beta }\) declares line 2 and all lines after line 2
. Suppose that 𝜖 is a successor ordinal. By Claim 4 and the induction hypothesis, \({\mathscr{S}}^{\prime }_{\epsilon }\) satisfies either φ1(ap,q)(p ≥ 1,q = 0, 1) or φ1(a0,0). By Claim 3, \({\mathscr{S}}^{\prime }_{\beta }\) satisfies either φ1(ap+ 1,q) or φ1(a1,0). So \({\mathscr{S}}^{\prime }_{\beta }\) declares line 2 and all lines after line 2  . Therefore the proposition holds for β.
. Therefore the proposition holds for β.
Claim 14. Let λ be a limit ordinal, and \({\mathscr{S}}\) be a λ-length revision sequence. Suppose that plane 2 and plane 3 are stably all-  in \({\mathscr{S}}\). Let δ be the least cardinal that is larger than \( \arrowvert \mathcal {P}(Sent({{\mathscr{L}}}^{+})) \arrowvert \). Let C = {An,α ∈ I(S)∣n is even }. Let \(X,Y,Z\subseteq Sent({{\mathscr{L}}}^{+})\). We get a unique (λ + δ × ω × 2)-length revision sequence \({\mathscr{S}}^{\prime }\) basing on \({\mathscr{S}}\) and using X, Y, Z and C as the bootstrappers in the interval [λ,λ + δ × ω × 2) in the following ways: in the intervals of the form [λ + δ × (2m + 1),λ + δ × (2m + 2)), where m ∈ ω, use X as the bootstrapper; in the intervals of the form [λ + δ × (2m + 2),λ + δ × (2m + 3)), where m ∈ ω, use Y as the bootstrapper; in the interval [λ + δ × ω,λ + δ × ω + δ) use Z as the bootstrapper; and use C as the bootstrapper at the other limit stages. Then plane 2 and plane 3 are stably all-
in \({\mathscr{S}}\). Let δ be the least cardinal that is larger than \( \arrowvert \mathcal {P}(Sent({{\mathscr{L}}}^{+})) \arrowvert \). Let C = {An,α ∈ I(S)∣n is even }. Let \(X,Y,Z\subseteq Sent({{\mathscr{L}}}^{+})\). We get a unique (λ + δ × ω × 2)-length revision sequence \({\mathscr{S}}^{\prime }\) basing on \({\mathscr{S}}\) and using X, Y, Z and C as the bootstrappers in the interval [λ,λ + δ × ω × 2) in the following ways: in the intervals of the form [λ + δ × (2m + 1),λ + δ × (2m + 2)), where m ∈ ω, use X as the bootstrapper; in the intervals of the form [λ + δ × (2m + 2),λ + δ × (2m + 3)), where m ∈ ω, use Y as the bootstrapper; in the interval [λ + δ × ω,λ + δ × ω + δ) use Z as the bootstrapper; and use C as the bootstrapper at the other limit stages. Then plane 2 and plane 3 are stably all-  in \({\mathscr{S}}^{\prime }\).
in \({\mathscr{S}}^{\prime }\).
The proof of Claim 14 is as follows. First, we prove that there is no hypothesis in the interval [λ,λ + δ × ω × 2) (of \({\mathscr{S}}^{\prime }\), of course) that declares plane 3 either all-  or all-
or all-  . (1) In the interval [λ,λ + δ), C is used as the bootstrapper. Note that C declares plane 1 neither all-
. (1) In the interval [λ,λ + δ), C is used as the bootstrapper. Note that C declares plane 1 neither all-  nor all-
nor all-  . By Claim 11 (k = 1), in the interval [λ,λ + δ), the declared truth value of each of plane 2 and plane 3 would not change. (2) In the interval [λ + δ,λ + δ × ω), alternately use X and Y as the bootstrappers. Next we prove in four cases that in the interval [λ + δ,λ + δ × ω), the declared truth value of plane 3 would not change, i.e., each hypothesis in the interval [λ + δ,λ + δ × ω) declares plane 3 all-
. By Claim 11 (k = 1), in the interval [λ,λ + δ), the declared truth value of each of plane 2 and plane 3 would not change. (2) In the interval [λ + δ,λ + δ × ω), alternately use X and Y as the bootstrappers. Next we prove in four cases that in the interval [λ + δ,λ + δ × ω), the declared truth value of plane 3 would not change, i.e., each hypothesis in the interval [λ + δ,λ + δ × ω) declares plane 3 all-  . (2.1) Suppose that both X and Y declare plane 1 neither all-
. (2.1) Suppose that both X and Y declare plane 1 neither all-  nor all-
nor all-  . By Claim 11 (k = 1), in the interval [λ + δ,λ + δ × ω), the declared truth value of each of plane 2 and plane 3 would not change. (2.2) Suppose that X declares plane 1 either all-
. By Claim 11 (k = 1), in the interval [λ + δ,λ + δ × ω), the declared truth value of each of plane 2 and plane 3 would not change. (2.2) Suppose that X declares plane 1 either all-  or all-
or all-  , and that Y declares plane 1 neither all-
, and that Y declares plane 1 neither all-  nor all-
nor all-  . By Claim 13, plane 2 is stably all-
. By Claim 13, plane 2 is stably all-  up to λ + 2δ in \({\mathscr{S}}^{\prime }\). By Claim 11 (k = 2) and Claim 12 (k = 2), in the interval [λ + δ,λ + 2δ), the declared truth value of plane 3 would not change. Since Y declares plane 1 neither all-
up to λ + 2δ in \({\mathscr{S}}^{\prime }\). By Claim 11 (k = 2) and Claim 12 (k = 2), in the interval [λ + δ,λ + 2δ), the declared truth value of plane 3 would not change. Since Y declares plane 1 neither all-  nor all-
nor all-  , by Claim 11 (k = 1), in the interval [λ + 2δ,λ + 3δ) the declared truth value of each of plane 2 and plane 3 would not change. In this case, we can see that in the interval [λ + δ,λ + δ × ω), the declared truth value of plane 3 would not change. (2.3) Suppose that X declares plane 1 neither all-
, by Claim 11 (k = 1), in the interval [λ + 2δ,λ + 3δ) the declared truth value of each of plane 2 and plane 3 would not change. In this case, we can see that in the interval [λ + δ,λ + δ × ω), the declared truth value of plane 3 would not change. (2.3) Suppose that X declares plane 1 neither all-  nor all-
nor all-  , and that Y declares plane 1 either all-
, and that Y declares plane 1 either all-  or all-
or all-  . The argument is similar to the argument for (2.2). (2.4) Suppose that both X and Y declare plane 1 either all-
. The argument is similar to the argument for (2.2). (2.4) Suppose that both X and Y declare plane 1 either all-  or all-
or all-  . By Claim 13, plane 2 is stably all-
. By Claim 13, plane 2 is stably all-  up to λ + 2δ in \({\mathscr{S}}^{\prime }\). By Claim 11 (k = 2) and Claim 12 (k = 2), in the interval [λ + δ,λ + 2δ), the declared truth value of plane 3 would not change. Similarly, plane 2 is stably all-
up to λ + 2δ in \({\mathscr{S}}^{\prime }\). By Claim 11 (k = 2) and Claim 12 (k = 2), in the interval [λ + δ,λ + 2δ), the declared truth value of plane 3 would not change. Similarly, plane 2 is stably all-  up to λ + 3δ in \({\mathscr{S}}^{\prime }\), and the declared truth value of plane 3 would not change in the interval [λ + 2δ,λ + 3δ). In this case, we can see that in the interval [λ + δ,λ + δ × ω), the declared truth value of plane 3 would not change. By (2.1)–(2.4), we conclude that the declared truth value of plane 3 would not change in the interval [λ + δ,λ + δ × ω). (3) In the interval [λ + δ × ω,λ + δ × ω + δ), Z is used as the bootstrapper. If Z declares plane 3 neither all-
up to λ + 3δ in \({\mathscr{S}}^{\prime }\), and the declared truth value of plane 3 would not change in the interval [λ + 2δ,λ + 3δ). In this case, we can see that in the interval [λ + δ,λ + δ × ω), the declared truth value of plane 3 would not change. By (2.1)–(2.4), we conclude that the declared truth value of plane 3 would not change in the interval [λ + δ,λ + δ × ω). (3) In the interval [λ + δ × ω,λ + δ × ω + δ), Z is used as the bootstrapper. If Z declares plane 3 neither all-  nor all-
nor all-  , by Claim 11 (k = 3), in the interval [λ + δ × ω,λ + δ × ω + δ), the declared truth value of plane 3 would be neither all-
, by Claim 11 (k = 3), in the interval [λ + δ × ω,λ + δ × ω + δ), the declared truth value of plane 3 would be neither all-  nor all-
nor all-  . If Z declares plane 3 either all-
. If Z declares plane 3 either all-  or all-
or all-  , by Claim 12 (k = 3), each hypothesis in the interval [λ + δ × ω,λ + δ × ω + δ) declares each of line 2ω + 2 and the lines after line 2ω + 2
, by Claim 12 (k = 3), each hypothesis in the interval [λ + δ × ω,λ + δ × ω + δ) declares each of line 2ω + 2 and the lines after line 2ω + 2  . We can see that in the interval [λ + δ × ω,λ + δ × ω + δ), the declared truth value of plane 3 would be neither all-
. We can see that in the interval [λ + δ × ω,λ + δ × ω + δ), the declared truth value of plane 3 would be neither all-  nor all-
nor all-  . (4) In the interval [λ + δ × ω + δ,λ + δ × ω × 2), C is used as the bootstrapper. By Claim 11 (k = 3), in the interval [λ + δ × ω + δ,λ + δ × ω × 2), plane 3 would be declared neither all-
. (4) In the interval [λ + δ × ω + δ,λ + δ × ω × 2), C is used as the bootstrapper. By Claim 11 (k = 3), in the interval [λ + δ × ω + δ,λ + δ × ω × 2), plane 3 would be declared neither all-  nor all-
nor all-  . By (1)–(4), we conclude that there is no hypothesis in the interval [λ,λ + δ × ω × 2) that declares plane 3 either all-
. By (1)–(4), we conclude that there is no hypothesis in the interval [λ,λ + δ × ω × 2) that declares plane 3 either all-  or all-
or all-  .
.
Next, we prove that plane 2 and plane 3 are stably all-  in \({\mathscr{S}}^{\prime }\). Consider the classical hypothesis \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\). \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) declares plane 3 neither all-
in \({\mathscr{S}}^{\prime }\). Consider the classical hypothesis \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\). \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) declares plane 3 neither all-  nor all-
nor all-  . By Claim 4, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ1(An,α)(n ≥ 1), φ1(a0,0) or φ4(a0,β)(β≠ 0,ω, 2ω). If \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ1(An,α)(n ≥ 1), by Claim 3, line α is unstable up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\), and the sentence \( A_{0,\alpha }\leftrightarrow A_{1,\alpha }\) is stably true up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\). Since C is the bootstrapper used at stage λ + δ × ω + δ + ω, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) does not satisfy φc, and hence satisfies φothers. If \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ1(a0,0), similarly, it can be proved that \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) satisfies φothers. If \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ4(a0,β)(β≠ 0,ω, 2ω), by Claim 3, both A0,β and A1,β are unstable up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\), and the sentence \( A_{0,\beta }\leftrightarrow A_{1,\beta }\) is stably true up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\). Since C is the bootstrapper used at stage λ + δ × ω + δ + ω, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) satisfies φothers. In each case, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) satisfies φothers. By Claim 3, plane 2 and plane 3 are stably all-
. By Claim 4, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ1(An,α)(n ≥ 1), φ1(a0,0) or φ4(a0,β)(β≠ 0,ω, 2ω). If \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ1(An,α)(n ≥ 1), by Claim 3, line α is unstable up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\), and the sentence \( A_{0,\alpha }\leftrightarrow A_{1,\alpha }\) is stably true up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\). Since C is the bootstrapper used at stage λ + δ × ω + δ + ω, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) does not satisfy φc, and hence satisfies φothers. If \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ1(a0,0), similarly, it can be proved that \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) satisfies φothers. If \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +1}\) satisfies φ4(a0,β)(β≠ 0,ω, 2ω), by Claim 3, both A0,β and A1,β are unstable up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\), and the sentence \( A_{0,\beta }\leftrightarrow A_{1,\beta }\) is stably true up to λ + δ × ω + δ + ω in \({\mathscr{S}}^{\prime }\). Since C is the bootstrapper used at stage λ + δ × ω + δ + ω, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) satisfies φothers. In each case, \({\mathscr{S}}^{\prime }_{\lambda +\delta \times \omega +\delta +\omega }\) satisfies φothers. By Claim 3, plane 2 and plane 3 are stably all-  up to λ + δ × ω + δ + 2ω in \({\mathscr{S}}^{\prime }\). Note that C declares plane 1 neither all-
up to λ + δ × ω + δ + 2ω in \({\mathscr{S}}^{\prime }\). Note that C declares plane 1 neither all-  nor all-
nor all-  . By Claim 11 (k = 1), in the interval [λ + δ × ω + δ + 2ω,λ + δ × ω × 2) the declared truth value of each of plane 2 and plane 3 would not change. Hence plane 2 and plane 3 are stably all-
. By Claim 11 (k = 1), in the interval [λ + δ × ω + δ + 2ω,λ + δ × ω × 2) the declared truth value of each of plane 2 and plane 3 would not change. Hence plane 2 and plane 3 are stably all-  in \({\mathscr{S}}^{\prime }\).
in \({\mathscr{S}}^{\prime }\).
Claim 15. TY does not dictate that truth behaves like a classical concept in \({\mathscr{M}}\).
The proof of Claim 15 is as follows. Next we construct a Y-sequence, and prove that this revision sequence does not culminate in a fixed point. By Claim 3, a fixed point of \(\tau _{{\mathscr{M}}}\) must satisfy φc ∧ φ6. Let δ be the least cardinal that is larger than \( \arrowvert \mathcal {P}(Sent({{\mathscr{L}}}^{+})) \arrowvert \). Let C = {An,α ∈ I(S)∣n is even }. Let \(U=\{(X,Y,Z)\mid X,Y,Z\subseteq Sent({{\mathscr{L}}}^{+})\}\). \( \arrowvert U \arrowvert =\arrowvert \mathcal {P}(Sent({{\mathscr{L}}}^{+})) \arrowvert <\delta \). So there is a surjection from δ to U. Let f be such a function. For every α ∈ δ, denote the first element of f(α) by Xα, the second element of f(α) by Yα, and the third element of f(α) by Zα. Let h be the classical hypothesis whose extension is C. h does not satisfy φc, and hence satisfies φothers. Define a y-bootstrapping-policy relative to hBr as follows: for every limit ordinal λ,
Let \( {\mathscr{S}}={\mathscr{M}}[h,Br]\). Obviously, \( {\mathscr{S}}\) is a Y-sequence. We prove by transfinite induction that for every ordinal α, plane 2 and plane 3 are stably all-  up to δ × ω × 2 × (α + 1) in \({\mathscr{S}}\). For α = 0, by Claim 3, plane 2 and plane 3 are stably all-
up to δ × ω × 2 × (α + 1) in \({\mathscr{S}}\). For α = 0, by Claim 3, plane 2 and plane 3 are stably all-  up to ω in \({\mathscr{S}}\). By Claim 14, the proposition holds for α. For α = β + 1, by Claim 14 and the induction hypothesis, the proposition holds. Let α be a limit ordinal. By definition, in the interval [δ × ω × 2 × α,δ × ω × 2 × α + δ), C is used as the bootstrapper. Since C declares plane 3 neither all-
up to ω in \({\mathscr{S}}\). By Claim 14, the proposition holds for α. For α = β + 1, by Claim 14 and the induction hypothesis, the proposition holds. Let α be a limit ordinal. By definition, in the interval [δ × ω × 2 × α,δ × ω × 2 × α + δ), C is used as the bootstrapper. Since C declares plane 3 neither all-  nor all-
nor all-  , By Claim 11 (k = 3), in the interval [δ × ω × 2 × α,δ × ω × 2 × α + δ), plane 3 would be declared neither all-
, By Claim 11 (k = 3), in the interval [δ × ω × 2 × α,δ × ω × 2 × α + δ), plane 3 would be declared neither all-  nor all-
nor all-  . By Claim 3, \({\mathscr{S}}_{\delta \times \omega \times 2\times \alpha +1}\) satisfies φ1(an,η)(n ≥ 1), φ1(a0,0) or φ4(a0,ζ)(ζ≠ 0,ω, 2ω). In each case, \({\mathscr{S}}_{\delta \times \omega \times 2\times \alpha +\omega }\) satisfies φothers. By Claim 3, plane 2 and plane 3 are stably all-
. By Claim 3, \({\mathscr{S}}_{\delta \times \omega \times 2\times \alpha +1}\) satisfies φ1(an,η)(n ≥ 1), φ1(a0,0) or φ4(a0,ζ)(ζ≠ 0,ω, 2ω). In each case, \({\mathscr{S}}_{\delta \times \omega \times 2\times \alpha +\omega }\) satisfies φothers. By Claim 3, plane 2 and plane 3 are stably all-  up to δ × ω × 2 × α + 2ω in \({\mathscr{S}}\). By Claim 14, the proposition holds for α. We conclude that for every ordinal α, plane 2 and plane 3 are stably all-
up to δ × ω × 2 × α + 2ω in \({\mathscr{S}}\). By Claim 14, the proposition holds for α. We conclude that for every ordinal α, plane 2 and plane 3 are stably all-  up to δ × ω × 2 × (α + 1) in \({\mathscr{S}}\). Obviously, \({\mathscr{S}}\) does not culminate in a fixed point.
up to δ × ω × 2 × (α + 1) in \({\mathscr{S}}\). Obviously, \({\mathscr{S}}\) does not culminate in a fixed point.
By Claim 9 and Claim 15, \({\mathbf {T}}^{lfp,\sigma _{2}}\not \le _2{\mathbf {T}}^Y\). □
Rights and permissions
About this article
Cite this article
Lin, Q., Liu, H. Comparing More Revision and Fixed-Point Theories of Truth. J Philos Logic 50, 615–671 (2021). https://doi.org/10.1007/s10992-020-09580-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10992-020-09580-7





 or
or  by h.
by h. by h and line β is declared
by h and line β is declared  ,
,  ,
,  or
or  by h, then α > β.
by h, then α > β. , we mean that this line is declared
, we mean that this line is declared  by h. For every ordinal α < 3ω, if there is an ordinal β such that α = β + 1, then the previous line of line α is line β; otherwise, the previous line of line α does not exist.
by h. For every ordinal α < 3ω, if there is an ordinal β such that α = β + 1, then the previous line of line α is line β; otherwise, the previous line of line α does not exist. in some plane, we mean that there are two adjacent lines in this plane that are declared
in some plane, we mean that there are two adjacent lines in this plane that are declared  by h. When we say some plane is all-
by h. When we say some plane is all-  , we mean that each line of this plane is declared
, we mean that each line of this plane is declared  by h.
by h. or
or  is less than or equal to 1.
is less than or equal to 1. only occurs after
only occurs after  ,
,  ,
,  and
and  .
. and
and  only occur after
only occur after  and
and  ; if one line is
; if one line is  , then its previous line (if exists) is
, then its previous line (if exists) is  ; and if one line is
; and if one line is  , then its previous line (if exists) is
, then its previous line (if exists) is  .
. or successive occurrences of
or successive occurrences of 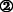 in plane k, then plane k is one of the following four cases:
in plane k, then plane k is one of the following four cases:
 , then the previous plane of it (if exists) is all-
, then the previous plane of it (if exists) is all- 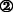 .
. ,
, 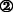 ,
,  ,
,  and
and  . Let line α be such a line. So there is a least natural number n such that the restriction to n of the declared truth value of line α by h is different from
. Let line α be such a line. So there is a least natural number n such that the restriction to n of the declared truth value of line α by h is different from 



 or
or  is greater than or equal to 2. (2.1) Suppose that both
is greater than or equal to 2. (2.1) Suppose that both  and
and  occur. Let line α be some line that is declared
occur. Let line α be some line that is declared  by h, and let line β be some line that is declared
by h, and let line β be some line that is declared  by h. Of course, α≠β. Let n be the least natural number such that the n th sentence of line α (i.e., An,α) is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let m be the least natural number such that the m th sentence of line β (i.e., Am,β) is declared false by h. Let B2 be the set consisting of the first m + 1 sentences of line β. We can represent B1 and B2 as follows:
by h. Of course, α≠β. Let n be the least natural number such that the n th sentence of line α (i.e., An,α) is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let m be the least natural number such that the m th sentence of line β (i.e., Am,β) is declared false by h. Let B2 be the set consisting of the first m + 1 sentences of line β. We can represent B1 and B2 as follows:

 is greater than or equal to 2. Choose two lines that are declared
is greater than or equal to 2. Choose two lines that are declared  by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that
by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared true by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that  is greater than or equal to 2. Choose two lines that are declared
is greater than or equal to 2. Choose two lines that are declared  by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared false by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that
by h. Let the line before the other be line α, and let the other line be line β. Of course, α < β. Let n be the least natural number such that the n th sentence of line α is declared false by h. Let B1 be the set consisting of the first n + 1 sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that  and there is a line after this line such that it is
and there is a line after this line such that it is  ,
,  ,
,  or
or  . Let line α be such a line. And let line β be a line after line α that is
. Let line α be such a line. And let line β be a line after line α that is  ,
,  ,
,  or
or  . Of course, α < β. Let B1 be the set consisting of the first two sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that
. Of course, α < β. Let B1 be the set consisting of the first two sentences of line α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that  or
or  and there is a line after this line such that it is
and there is a line after this line such that it is  or
or  ; (4.2) there is a line that is
; (4.2) there is a line that is  , but its previous line is not
, but its previous line is not  ; (4.3) there is a line that is
; (4.3) there is a line that is  , but its previous line is not
, but its previous line is not  . In case (4.1), let line α be a line that is
. In case (4.1), let line α be a line that is  or
or  and such that there is a line after line α that is
and such that there is a line after line α that is  or
or  . And let line β be a line that is after line α and that is
. And let line β be a line that is after line α and that is  or
or  . α < β. Let n be the greatest natural number such that the first n sentences of line α are declared the same truth value by h. Let B1 be the set consisting of An− 1,α and An,α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that
. α < β. Let n be the greatest natural number such that the first n sentences of line α are declared the same truth value by h. Let B1 be the set consisting of An− 1,α and An,α. Let B2 be the set consisting of the first two sentences of line β. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that  , but its previous line is
, but its previous line is  . Let line α + 1 be such a line. Its previous line, i.e., line α, is
. Let line α + 1 be such a line. Its previous line, i.e., line α, is  . Define sets B1 and B2 as follows:
. Define sets B1 and B2 as follows:

 , but its previous line is
, but its previous line is  . Let line α + 1 be such a line. Its previous line, i.e., line α, is
. Let line α + 1 be such a line. Its previous line, i.e., line α, is  . Define sets B1 and B2 as follows:
. Define sets B1 and B2 as follows:

 or successive occurrences of
or successive occurrences of  in this plane and this plane is not one of the four cases shown in the property (V). Let plane k be such a plane. (5.1) Suppose that there are successive occurrences of
in this plane and this plane is not one of the four cases shown in the property (V). Let plane k be such a plane. (5.1) Suppose that there are successive occurrences of  in plane k. Obviously, plane k is not one of the following two cases:
in plane k. Obviously, plane k is not one of the following two cases:

 by h. Let them be line α and line α + 1 respectively. Then ω × (k − 1) ≤ α < ω × k. We consider in four cases: (5.1.1) in plane k, there is an occurrence of
by h. Let them be line α and line α + 1 respectively. Then ω × (k − 1) ≤ α < ω × k. We consider in four cases: (5.1.1) in plane k, there is an occurrence of  ,
,  or
or  ; (5.1.2) in plane k, there is no occurrence of
; (5.1.2) in plane k, there is no occurrence of  ,
,  or
or  , and there is a line before line α that is
, and there is a line before line α that is  ; (5.1.3) in plane k, there is no occurrence of
; (5.1.3) in plane k, there is no occurrence of  ,
,  or
or  , and all lines before line α are
, and all lines before line α are  , and there are successive occurrences of
, and there are successive occurrences of  after line α + 1; (5.1.4) in plane k, there is no occurrence of
after line α + 1; (5.1.4) in plane k, there is no occurrence of  ,
,  or
or  , and all lines before line α are
, and all lines before line α are  , and there are no successive occurrences of
, and there are no successive occurrences of  after line α + 1.
after line α + 1. ,
,  or
or  after line α + 1. Let line β be a line in plane k that is
after line α + 1. Let line β be a line in plane k that is  ,
,  or
or  and that is after line α + 1. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of the first two sentences of line α + 1. Choose a sentence in line β that is declared true by h, and another sentence in line β that is declared false by h. Let B3 be the set consisting of these two sentences. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that
and that is after line α + 1. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of the first two sentences of line α + 1. Choose a sentence in line β that is declared true by h, and another sentence in line β that is declared false by h. Let B3 be the set consisting of these two sentences. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that  and that is before line α. Then ω × (k − 1) ≤ β < α. Let B1 be the set consisting of A0,β. Let B2 be the set consisting of A0,α. Let B3 be the set consisting of the first two sentences of line α + 1. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that
and that is before line α. Then ω × (k − 1) ≤ β < α. Let B1 be the set consisting of A0,β. Let B2 be the set consisting of A0,α. Let B3 be the set consisting of the first two sentences of line α + 1. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that  by h. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Let B4 be the set consisting of A0,β+ 1. Similar to (2.1), B1, B2, B3 and B4 determine a sentence that h declares true and that
by h. Then α + 1 < β < ω × k. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Let B4 be the set consisting of A0,β+ 1. Similar to (2.1), B1, B2, B3 and B4 determine a sentence that h declares true and that  , and it is
, and it is  , and in plane k, starting with this line,
, and in plane k, starting with this line,  and
and  alternately occur. Let line β be this line. Then α + 1 < β < ω × k. Since h does not have the property (V), there is not an odd number n greater than or equal to 3 such that β = ω × (k − 1) + n. Then there is an even number n such that β = ω × (k − 1) + n. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that
alternately occur. Let line β be this line. Then α + 1 < β < ω × k. Since h does not have the property (V), there is not an odd number n greater than or equal to 3 such that β = ω × (k − 1) + n. Then there is an even number n such that β = ω × (k − 1) + n. Let B1 be the set consisting of A0,α. Let B2 be the set consisting of A0,α+ 1. Let B3 be the set consisting of A0,β. Similar to (2.1), B1, B2 and B3 determine a sentence that h declares true and that  in plane k. The proof is similar to (5.1).
in plane k. The proof is similar to (5.1). and its previous plane is not all-
and its previous plane is not all-  . Let plane k be such a plane. Then k ∈{2, 3}. Since plane k is not all-
. Let plane k be such a plane. Then k ∈{2, 3}. Since plane k is not all-  , then there is a sentence in plane k such that either its abscissa is even and it is declared false by h, or its abscissa is odd and it is declared true by h. Choose such a sentence, and let B1 be the set consisting of it. Since plane k − 1 is not all-
, then there is a sentence in plane k such that either its abscissa is even and it is declared false by h, or its abscissa is odd and it is declared true by h. Choose such a sentence, and let B1 be the set consisting of it. Since plane k − 1 is not all-  , then there is a sentence in plane k − 1 that is declared true by h. Choose such a sentence, and let B2 be the set consisting of it. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that
, then there is a sentence in plane k − 1 that is declared true by h. Choose such a sentence, and let B2 be the set consisting of it. Similar to (2.1), B1 and B2 determine a sentence that h declares true and that