Research on the Energy Allocation Scheme Based on SWIPT Relaying System

Abstract

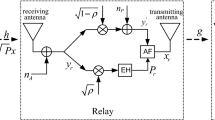
Simultaneous wireless information and power transfer (SWIPT) is a promising communication solution for energy-constrained wireless network. While the interference affects the performance of the system, it also carries energy. In this paper, we investigate an amplify-and-forward (AF) relaying network. In the network, an energy-constrained relay harvests energy from the received RF signal. During the information transmission (IT) period, the relay uses the harvested energy to forward the signal. When the IT is interrupted for some reason, such as strong interference, the relay stores the harvested energy into the energy storage till the interference ends. Particularly, in the IT period, the relay allocates the energy of its storage to the blocks of the IT. Based on the time switching (TS) architecture, the system rates of two energy allocation cases are discussed. It is proved that the scheme based on the energy-allocated-evenly (EAE) is the optimal solution of the system rate maximization, and the mathematical expressions of the system rate based on the EAE scheme is obtained. Then, we discuss the influence of the EAE scheme on the system rate, and the numerical analysis provides practical insights into the effect of two system parameters, namely, interference power and interference factor on the performance of wireless energy harvesting (EH) and IT using AF relay. The results show that the proposed EAE scheme can effectively improve the relaying system rate under an interference channel.

Appendix

Notation: f¹(x)|_x represents the derivative of f(x) with respect to x; f²(x)|_x denotes the derivative of f¹(x)|_x with respect to x.

It is important to point out that α ∈ [0, 1), ${\alpha}_1^{\ast}\in \left[0,1\right)$, ${\alpha}_2^{\ast}\in \left(0,1\right)$, and ${\alpha}_3^{\ast}\in \left[0,1\right)$ hold in the following discussion.

For (21), R^∗ can be regarded as a function of β, R^∗(β). We can observe that R^∗(β → 1⁺)= R^∗(β → 1⁻),i.e., the left limit of R^∗(β) at β = 1 is equal to the right limit, namely, R^∗(β) is continuous at β = 1. Therefore, if we can prove that, when 0 < β < 1, R^∗(β) increases monotonically as β increases; when β ≥ 1, R^∗(β) decreases monotonically as β increases, β = 1 is the optimal solution of R^∗(β). The two cases are shown separately as follows.

• a. Case of 0 < β < 1

When 0 < β < 1, R^∗(β) is expressed as (19). An auxiliary variable λ = ξP_I/β is introduced so that ${R}_{0<\beta <1}^{\ast }$ can be rewritten as

$$ {\displaystyle \begin{array}{l}{R}_{0<\beta <1}^{\ast }=\frac{1}{1+\xi}\\ {}\left(\begin{array}{l}\beta \left(1-{\alpha}_1^{\ast}\right){\log}_2\\ {}\left(1+\frac{hg\eta P\cdot \left(h{\alpha}_1^{\ast }P+\lambda \right)}{g{\eta \sigma}_A^2\cdot \left(h{\alpha}_1^{\ast }P+\lambda \right)+\left(1-{\alpha}_1^{\ast}\right)\left( hP+{\sigma}_A^2\right){\sigma}_R^2}\right)\\ {}+\left(1-\beta \right)\left(1-{\alpha}_2^{\ast}\right){\log}_2\\ {}\left(1+\frac{hg\eta P\cdot h{\alpha}_2^{\ast }P}{g{\eta \sigma}_A^2\cdot h{\alpha}_2^{\ast }P+\left(1-{\alpha}_2^{\ast}\right)\left( hP+{\sigma}_A^2\right){\sigma}_R^2}\right)\end{array}\right)\end{array}} $$

(24)

An auxiliary function r(α, λ) is defined as

$$ r\left(\alpha, \lambda \right)=\left(1-\alpha \right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{a\lambda + b\alpha}{c\lambda + d\alpha +e}\right) $$

(25)

where a = hgηP, b = h²gηP², $c=g{\eta \sigma}_A^2$, $d= hg\eta P{\sigma}_A^2-\left( hP+{\sigma}_A^2\right){\sigma}_R^2$, and $e=\left( hP+{\sigma}_A^2\right){\sigma}_R^2$.

Taking the first and second derivatives of r(α, λ) with respect to λ, we obtain

$$ {\left.{r}^1\left(\alpha, \lambda \right)\right|}_{\lambda}\operatorname{}=\frac{1-\alpha }{\ln \kern.2em 2}\times \frac{\left( ad- bc\right)\alpha + ae}{\left(\left(a+c\right)\lambda +\left(b+d\right)\alpha +e\right)\left( c\lambda + d\alpha +e\right)} $$

(26)

$$ {\displaystyle \begin{array}{l}{r}^2\left(\alpha, \lambda \right)\left|{}_{\lambda}\right.=-\frac{1-\alpha }{\ln\ 2}\cdot \left(\left( ad- bc\right)\alpha + ae\right)\cdot \\ {}\kern2.75em \frac{2c\left(a+c\right)\lambda +\left( ad+2 cd+ bc\right)\alpha +2 ce+ ae}{{\left(\left(\left(a+c\right)\lambda +\left(b+d\right)\alpha +e\right)\left( c\lambda + d\alpha +e\right)\right)}^2}\end{array}} $$

(27)

where,

$$ \left( ad- bc\right)\alpha + ae=\left(1-\alpha \right) hg\eta P\left(\left( hP+{\sigma}_A^2\right){\sigma}_R^2\right) $$

(28)

Obviously, (ad − bc)α + ae is greater than 0, therefore, for any λ > 0, we hold r¹(α, λ)|_λ > 0 and r²(α, λ)|_λ < 0.

According to the auxiliary function, ${R}_{0<\beta <1}^{\ast }$ can be rewritten as

$$ {R}_{0<\beta <1}^{\ast }=\frac{1}{1+\xi}\left(\beta \cdot r\left({\alpha}_1^{\ast },\lambda \right)+\left(1-\beta \right)\cdot r\Big({\alpha}_2^{\ast },0\Big)\right) $$

(29)

Taking the first and second derivatives of ${R}_{0<\beta <1}^{\ast }$ with respect to β, we have

$$ {R}_{0<\beta <1}^{\ast, 1}{\left|{}_{\beta}\operatorname{}=\frac{1}{1+\xi}\left(r\left({\alpha}_1^{\ast },\lambda \right)-\lambda \cdot {r}^1\right({\alpha}_1^{\ast },\lambda \Big)\right|}_{\lambda}\operatorname{}-r\left({\alpha}_2^{\ast },0\right)\Big) $$

(30)

$$ {R}_{0<\beta <1}^{\ast, 2}\left|{}_{\beta}\right.=\frac{\lambda^2}{\beta \cdot \left(1+\xi \right)}\cdot {r}^2\left({\alpha}_1^{\ast },\lambda \right)\left|{}_{\lambda}\right. $$

(31)

Both ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ and ${R}_{0<\beta <1}^{\ast, 2}\left|{}_{\beta}\right.$ are the function of λ. Because of β ∈ (0, 1), we have λ ∈ (ξP_I, +∞). Therefore, we only discuss the change of the function ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ and ${R}_{0<\beta <1}^{\ast, 2}\left|{}_{\beta}\right.$ for λ ∈ (ξP_I, +∞). For any λ > 0, r²(α, λ)|_λ < 0, so ${R}_{0<\beta <1}^{\ast, 2}\left|{}_{\beta}\right.<0$ for λ ∈ (ξP_I, +∞). Consequently, ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ is monotonically decreasing for λ ∈ (ξP_I, +∞). Next, we discuss whether ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ is greater than 0 or less than 0 when λ →  + ∞. From (30), we have

$$ \underset{\lambda \to +\infty }{\lim }{R}_{0<\beta <1}^{\ast, 1}{\left|{}_{\beta}\operatorname{}=\underset{\lambda \to +\infty }{\lim}\left(\frac{1}{1+\xi}\right(r\left({\alpha}_1^{\ast },\lambda \right)-\lambda \cdot {r}^1\Big({\alpha}_1^{\ast },\lambda \Big)\right|}_{\lambda}\operatorname{}-r\left({\alpha}_2^{\ast },0\right)\left)\right) $$

(32)

where, ${\left.\underset{\lambda \to +\infty }{\lim}\lambda \cdot {r}^1\left({\alpha}_1^{\ast },\lambda \right)\right|}_{\lambda}\operatorname{}=0$, and

$$ \underset{\lambda \to +\infty }{\lim}\left(r\left({\alpha}_1^{\ast },\lambda \right)-r\left({\alpha}_2^{\ast },0\right)\right)=\left(1-{\alpha}_1^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{a}{c}\right)-\left(1-{\alpha}_2^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{b{\alpha}_2^{\ast }}{d{\alpha}_2^{\ast }+e}\right) $$

(33)

According to the discussion above, we already know ${\alpha}_1^{\ast }<{\alpha}_2^{\ast }$, so we have

$$ {\displaystyle \begin{array}{l}\left(1-{\alpha}_1^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{a}{c}\right)-\left(1-{\alpha}_2^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{b{\alpha}_2^{\ast }}{d{\alpha}_2^{\ast }+e}\right)>\\ {}\left(1-{\alpha}_1^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{a}{c}\right)-\left(1-{\alpha}_1^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(1+\frac{b{\alpha}_2^{\ast }}{d{\alpha}_2^{\ast }+e}\right)=\\ {}\left(1-{\alpha}_1^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(\frac{\left(c+a\right)d{\alpha}_2^{\ast }+e\left(c+a\right)}{c\left(b+d\right){\alpha}_2^{\ast }+ ce}\right)\end{array}} $$

(34)

Besides, we can obtain

$$ {\displaystyle \begin{array}{l}\left(c+a\right)d{\alpha}_2^{\ast }+e\left(c+a\right)-\left(c\left(b+d\right){\alpha}_2^{\ast }+ ce\right)\\ {}=\left( ad- bc\right){\alpha}_2^{\ast }+ ae\\ {}=\left(1-{\alpha}_2^{\ast}\right) hg\eta P\left(\left( hP+{\sigma}_A^2\right){\sigma}_R^2\right)>0\end{array}} $$

(35)

Accordingly, we obtain

$$ \left(c+a\right)d{\alpha}_2^{\ast }+e\left(c+a\right)>c\left(b+d\right){\alpha}_2^{\ast }+ ce $$

(36)

and then

$$ \frac{\left(c+a\right)d{\alpha}_2^{\ast }+e\left(c+a\right)}{c\left(b+d\right){\alpha}_2^{\ast }+ ce}>1 $$

(37)

Further, we have

$$ \left(1-{\alpha}_1^{\ast}\right)\mathrm{lo}{\mathrm{g}}_2\left(\frac{\left(c+a\right)d{\alpha}_2^{\ast }+e\left(c+a\right)}{c\left(b+d\right){\alpha}_2^{\ast }+ ce}\right)>0 $$

(38)

and then lim _λ→+∞$\left(r\left({\alpha}_1^{\ast },\lambda \right)-r\left({\alpha}_2^{\ast },0\right)\right)>0$. Therefore, ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ is greater than 0 when λ →  + ∞. For λ ∈ (ξP_I, +∞), because ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ is monotonically decreasing, we obtain ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ > 0, i.e., ${R}_{0<\beta <1}^{\ast, 1}\left|{}_{\beta}\right.$ > 0 for β ∈ (0, 1). Therefore, ${R}_{0<\beta <1}^{\ast }$ is monotonically increasing for any β ∈ (0, 1).

• b. Case of β ≥ 1

When β ≥ 1, R^∗(β) is expressed as (20). Similarly, we can obtain

$$ {R}_{\beta \ge 1}^{\ast }=\frac{r\left({\alpha}_3^{\ast },\lambda \right)}{1+\xi } $$

(39)

Taking the first derivative of ${R}_{\beta \ge 1}^{\ast }$ with respect to β, we have

$$ {R}_{\beta \ge 1}^{\ast, 1}\left|{}_{\beta}\right.=-\frac{\lambda }{\beta \left(1+\xi \right)}{r}^1\left({\alpha}_3^{\ast },\lambda \right)\left|{}_{\lambda}\right. $$

(40)

For any λ > 0, ${r}^1\left({\alpha}_3^{\ast },\lambda \right)\left|{}_{\lambda}\right.>0$, so we have R_β ≥ 1^{∗, 1}|_β < 0. As a result, ${R}_{\beta \ge 1}^{\ast }$ decreases monotonically for any β ≥ 1.

To sum up, R^∗(β) is monotonically increasing for any β ∈ (0, 1), R^∗(β) decreases monotonically for any β ≥ 1, and R^∗(β) is continuous at the point β = 1, so β = 1 is the optimal solution of R^∗(β).