A Physical Layer Network Coding Based Modify-and-Forward with Opportunistic Secure Cooperative Transmission Protocol

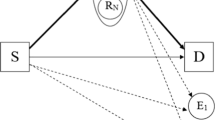
Abstract

This paper investigates a new secure relaying scheme, namely physical layer network coding based modify-and-forward (PMF), in which a relay node linearly combines the decoded data sent by a source node with an encrypted key before conveying the mixed data to a destination node. We first derive the general expression for the generalized secrecy outage probability (GSOP) of the PMF scheme and then use it to analyse the GSOP performance of various relaying and direct transmission strategies. The GSOP performance comparison indicates that these transmission strategies offer different advantages depending on the channel conditions and target secrecy rates, and relaying is not always desirable in terms of secrecy. Subsequently, we develop an opportunistic secure transmission protocol for cooperative wireless relay networks and formulate an optimisation problem to determine secrecy rate thresholds (SRTs) to dynamically select the optimal transmission strategy for achieving the lowest GSOP. The conditions for the existence of the SRTs are derived for various channel scenarios.

Appendices

Appendix A: Proof of Lemma 1

Given $Y=\frac {a^{2}X}{b^{2}X + 1}$ and X = c|Z|² where c is a positive constant, it can be deduced that a² ≥ b²Y. The cdf of Y can be computed by [41]

$$ F_{Y}(y)=\Pr\{Y\leqslant y\}=\Pr\left\{X\leqslant\frac{y}{a^{2}-b^{2}y}\right\}. $$

(42)

Note that the pdf and cdf of X are given by

$$ f_{X}(x)=\frac{1}{c}\exp\left( -\frac{x}{c}\right), $$

(43)

$$ F_{X}(x) = Pr\{X\leqslant x\}={{\int}_{0}^{x}}f_{X}(t)dt= 1-\exp\left( -\frac{x}{c}\right), $$

(44)

respectively. Substituting Eq. 44 into Eq. 42, we have

$$ F_{Y}(y)=F_{X}\left( \frac{y}{a^{2}-b^{2}y}\right)= 1-\exp\left( -\frac{y}{c(a^{2}-b^{2}y)}\right) $$

(45)

The pdf of Y can be therefore obtained by

$$ f_{Y}(y)=\frac{dF_{Y}(y)}{dy}=\frac{a^{2}}{c(a^{2}-b^{2}y)^{2}}\exp\left[-{\frac{y}{c(a^{2}-b^{2}y)}}\right]. $$

(46)

Appendix B: Proof of Theorem 1

For brevity, let $X=\gamma _{\mathcal {SR}}$, $Y=\gamma _{\mathcal {SD}}$, $Z=\gamma _{\mathcal {RD}}$, $U=\gamma _{\mathcal {SE}}$ and $V=\gamma _{\mathcal {RE}}$. We can rewrite Eq. 14 as

$$ P^{(PMF)}_{out} = \Pr\left\{\left[\log_{2}\left( \frac{1+\min\{X,Y + Z\}}{1+U+V}\right)\right]^{+}\!<2 \theta R_{s}\right\}. $$

(47)

Note that the secure communication is possible with a positive secrecy capacity if the legitimate links, including the direct and/or relaying links, have higher channel gains over the eavesdropper links. In order to prevent the secrecy outage from always happening, it is assumed that min{X, Y + Z} > U + V. From Eq. 47, we have

$$\begin{array}{@{}rcl@{}} P_{out}^{(PMF)}&=&\Pr\!\left\{\log_{2}\left( \frac{1+\min\{X,Y + Z\}}{1+U+V}\right)<2 \theta R_{s}\right\}\\ & =&\Pr\!\left\{2^{-2R_{s}}(1 + \min\{X,Y + Z\}) - 1\!<\!U+V \right\},\\ \end{array} $$

(48)

Considering two scenarios of X ≤ Y + Z and X > Y + Z, Eq. 48 can be rewritten by Eq. 49 (see the top of next page).

$$\begin{array}{@{}rcl@{}} P_{out}^{(PMF)}\!\!&=&\!\underbrace{\Pr\{2^{-2 \theta R_{s}}(1 + X) - 1\!<\!U + V\!<\!X\}\Pr\{X\!\leqslant\! Y + Z\}}_{\displaystyle \triangleq P_{1}} \\ &&\!+\underbrace{\Pr\{2^{-2 \theta R_{s}}(1 + Y + Z) - 1\!<\!U + V\!<\!Y + Z\}\Pr\{X\!>\! Y + Z\}}_{\displaystyle \triangleq P_{2}}.\\ \end{array} $$

(49)

Deriving P₁ and P₂ in Eq. 49, it can be observed that, if $X\leqslant \min \{Y,2^{2 \theta R_{s}}-1\}$, then $\Pr \{2^{-2 \theta R_{s}}(1+X)-1<U+V<X\}= 1$ and Pr{X ≤ Y + Z} = 1 since $U+V\geqslant 0$ and $Z\geqslant 0$. This means P₁ = 1 and P₂ = 0, i. e. $P_{out}^{(PMF)}= 1$ (outage occurs). Similarly, if $Y+Z\leqslant \min \{X,2^{2 \theta R_{s}}-1\}$, then $\Pr \{2^{-2 \theta R_{s}}(1+Y+Z)-1<U+V<Y+Z\}= 1$ and Pr{X > Y + Z} = 1 since $U+V\geqslant 0$, and thus outage happens. Therefore, in order to avoid the outage, by considering all these above conditions, we can arrive at Eqs. 50 and 51 (see the top of next page).

$$\begin{array}{@{}rcl@{}} P_{1} &=& {\int}_{2^{2 \theta R_{s}}-1}^{\infty}f_{Y}(y){\int}_{y}^{\infty}f_{X}(x){\int}_{x-y}^{\infty}f_{Z}(z){{\int}_{0}^{x}}f_{U}(u){\int}_{2^{-2 \theta R_{s}}(1+x)-1-u}^{x-u}f_{V}(v) dv du dz dx dy \\ &&+ {\int}_{2^{2 \theta R_{s}}-1}^{\infty}f_{X}(x){\int}_{x}^{\infty}f_{Y}(y){{\int}_{0}^{x}}f_{U}(u){\int}_{2^{-2 \theta R_{s}}(1+x)-1-u}^{x-u}f_{V}(v) dv du dy dx \\ &&+ {\int}_{0}^{2^{2 \theta R_{s}}-1}f_{Y}(y){\int}_{y}^{2^{2 \theta R_{s}}-1}f_{X}(x){\int}_{x-y}^{\infty}f_{Z}(z){{\int}_{0}^{x}}f_{U}(u){\int}_{0}^{x-u}f_{V}(v) dv du dz dx dy \\ &&+ {\int}_{0}^{2^{2 \theta R_{s}}-1}f_{Y}(y){\int}_{2^{2 \theta R_{s}}-1}^{\infty}f_{X}(x){\int}_{x-y}^{\infty}f_{Z}(z){{\int}_{0}^{x}}f_{U}(u){\int}_{2^{-2 \theta R_{s}}(1+x)-1-u}^{x-u}f_{V}(v) dv du dz dx dy, \end{array} $$

(50)

$$ P_{2}={\int}_{2^{2 \theta R_{s}}-1}^{\infty}f_{X}(x) {\int}_{2^{2 \theta R_{s}}-1}^{x}f_{Y}(y) {\int}_{2^{2 \theta R_{s}}-1-y}^{x-y}f_{Z}(z) {\int}_{0}^{y+z}f_{U}(u){\int}_{2^{-2 \theta R_{s}}(1+y+z)-1-u}^{y+z-u}f_{V}(v) dv du dz dy dx. $$

(51)

For simplicity, let us define

$$ I_{1}(x)\triangleq {{\int}_{0}^{x}}f_{U}(u){\int}_{2^{-2 \theta R_{s}}(1+x)-1-u}^{x-u}f_{V}(v) dv du, $$

(52)

$$ I_{2}(x)\triangleq {{\int}_{0}^{x}}f_{U}(u){\int}_{0}^{x-u}f_{V}(v) dv du. $$

(53)

Substituting Eqs. 50 and 51 into Eq. 49 with I₁(x) and I₂(x), the theorem is proved.

Appendix C: Proof of Proposition 1

From $\frac {d f(x)}{d x}>\frac {d g(x)}{d x}>0$, there exist x₁ > 0 and x₂ > 0 such that

$$ \frac{f(x_{1})-f(0)}{x_{1}}>\frac{g(x_{2})-g(0)}{x_{2}}. $$

(54)

If f(0) < g(0), then there exists a crossing point x^′ = x₁ = x₂ > 0 such that f(x^′) = g(x^′) and thus (f(x^′) − f(0))/x^′ > (g(x^′) − g(0))/x^′ satisfying Eq. 54. Conversely, if there exists a crossing point x^′ = x₁ = x₂ > 0 satisfying Eq. 54, then we can easily deduce that f(0) < g(0).

Proof of uniqueness: Let us assume that there exists 0 < x^″ ≠ x^′ satisfying f(x^″) = g(x^″) and f(x^′) = g(x^′). We have

$$ \frac{f(x^{\prime\prime})-f(x^{\prime})}{x^{\prime\prime}-x^{\prime}}=\frac{g(x^{\prime\prime})-g(x^{\prime})}{x^{\prime\prime}-x^{\prime}}, $$

(55)

which contradicts the fact that $\frac {d f(x)}{d x}>\frac {d g(x)}{d x}$.

Therefore, we can conclude that ∃!x^′ > 0 : f(x^′) = g(x^′) if and only if f(0) < g(0).

Appendix D: Proof of Theorem 2

In the DT protocol, as R_s → 0, it can be observed from Eq. 26 that

$$ P^{(DT)}_{out}\to \frac{\bar{\gamma}_{\mathcal{SE}}}{\bar{\gamma}_{\mathcal{SD}}+\bar{\gamma}_{\mathcal{SE}}}\triangleq P^{(DT)}_{0}. $$

(56)

In the RT protocol, as R_s → 0, Φ(x) in Eq. 37 approaches

$$\begin{array}{@{}rcl@{}} {\Phi}(x) \!&\to&\! \left( \!1 + \frac{x}{\bar{\gamma}_{\mathcal{SR}}}\right) \!\left( \frac{1}{\bar{\gamma}^{-1}_{\mathcal{SR}} + x^{-1}} - \frac{1}{\bar{\gamma}^{-1}_{\mathcal{SE}} + \bar{\gamma}^{-1}_{\mathcal{SR}} + x^{-1}}\right)\\ &&=\frac{x^{2}\bar{\gamma}_{\mathcal{SR}}}{x\bar{\gamma}_{\mathcal{SR}} + x\bar{\gamma}_{\mathcal{SE}} + \bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}}}. \end{array} $$

(57)

Substituting Eq. 57 into Eq. 36, the limit of $P^{(RT)}_{out}$ can be obtained by Eq. 58.

$$\begin{array}{@{}rcl@{}} P^{(RT)}_{out}&\to& 1-\frac{\frac{\bar{\gamma}_{\mathcal{RD}}^{2}\bar{\gamma}_{\mathcal{SR}}}{\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SR}}+\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}}}-\frac{\bar{\gamma}_{\mathcal{SD}}^{2}\bar{\gamma}_{\mathcal{SR}}}{\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SR}}+\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}}}}{\bar{\gamma}_{\mathcal{RD}}-\bar{\gamma}_{\mathcal{SD}}} \\ &=& \frac{\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SE}}(\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SR}}+\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}})+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}^{2}_{\mathcal{SE}}(\bar{\gamma}_{\mathcal{SD}}+\bar{\gamma}_{\mathcal{SR}})}{(\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SR}}+\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}})(\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SR}}+\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}})} \\ &\triangleq& P^{(RT)}_{0}. \end{array} $$

(58)

Denote ${\Delta }=P^{(RT)}_{0}-P^{(DT)}_{0}$. Solving Δ < 0, after some mathematical manipulations, we obtain the condition of the channel quality of various links as in Eq. 59.

$$\begin{array}{@{}rcl@{}} {\Delta} <0 &\Leftrightarrow& \bar{\gamma}_{\mathcal{SR}}\bar{\gamma}^{2}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{RD}}+\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}^{2}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}^{2}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SE}}<\bar{\gamma}^{2}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{RD}}+\bar{\gamma}^{2}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SE}} \\&\Leftrightarrow&\bar{\gamma}_{\mathcal{SR}}(\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}^{2}_{\mathcal{SD}}-\bar{\gamma}_{\mathcal{RD}}\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}}+\bar{\gamma}^{2}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}})+\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{RD}}(\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}}-\bar{\gamma}^{2}_{\mathcal{SR}})<0 \\&\Leftrightarrow& \bar{\gamma}_{\mathcal{SR}}[\bar{\gamma}_{\mathcal{RD}}(\bar{\gamma}^{2}_{\mathcal{SD}}-\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{SE}}/2)+\bar{\gamma}_{\mathcal{SE}} (\bar{\gamma}^{2}_{\mathcal{SD}}-\bar{\gamma}_{\mathcal{SR}}\bar{\gamma}_{\mathcal{RD}}/2)]+\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{RD}}(\bar{\gamma}_{\mathcal{SD}}\bar{\gamma}_{\mathcal{SE}}-\bar{\gamma}^{2}_{\mathcal{SR}})<0. \end{array} $$

(59)

It can be seen that, if $\bar {\gamma }_{\mathcal {SD}}\bar {\gamma }_{\mathcal {SE}}<\bar {\gamma }^{2}_{\mathcal {SR}}$, $\bar {\gamma }_{\mathcal {SD}}<\sqrt {\bar {\gamma }_{\mathcal {SR}}\bar {\gamma }_{\mathcal {SE}}/2}$ and $\bar {\gamma }_{\mathcal {SD}}<\sqrt {\bar {\gamma }_{\mathcal {SR}}\bar {\gamma }_{\mathcal {RD}}/2}$, then Δ < 0, i.e. $P^{(RT)}_{0}<P^{(DT)}_{0}$. Additionally, as in the conventional relaying scheme, the gradient of the GSOP performance of the RT scheme is higher than that of the DT scheme and the GSOP of both schemes increases as a function of the target secrecy rate, i.e. $\frac {dP^{(RT)}_{out}}{dR_{s}}>\frac {dP^{(DT)}_{out}}{dR_{s}}>0$. Therefore, from Proposition 1, we can conclude that $\exists ! R^{\prime }_{s}>0: P^{(DT)}_{out}(R^{\prime }_{s})=P^{(RT)}_{out}(R^{\prime }_{s})$.