Skip to main content
SpringerLink
Log in

Fractional collocation boundary value methods for the second kind Volterra equations with weakly singular kernels

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

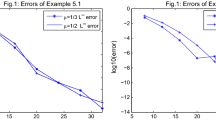
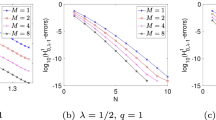
Abstract

We discuss the numerical solution to a class of weakly singular Volterra integral equations in this paper. Firstly, the fractional Lagrange interpolation is applied to deal with the singularity of the solution, and efficient fractional collocation boundary value methods are developed. Secondly, local convergence estimates are derived from examining the asymptotic property of the solution and the interpolation remainder. We find that the second kind Volterra integral equation with a weakly singular kernel can be efficiently solved on a uniform grid. Finally, several numerical examples are given to illustrate the performance of fractional collocation boundary value methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. In the remaining part, we will denote the various constant to be B for simplicity.

References

  1. Roberts, C.A., Lasseigne, D.G., Olmstead, W.E.: Volterra equations which model explosion in a diffusive medium. J. Integr. Eq. Appl. 5(4), 531–546 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  2. Olmstead, W.E., Roberts, C.A.: Explosion in a diffusive strip due to a source with local and nonlocal features. Methods Appl. Anal. 3(3), 345–357 (1996)

    MathSciNet  MATH  Google Scholar 

  3. Brunner, H.: Volterra Integral Equations: An Introduction to Theory and Applications. Cambridge University Press, Cambridge (2017)

    Book  MATH  Google Scholar 

  4. Diethelm, K., Ford, N.J.: Analysis of fractional differential equations. J. Math. Anal. Appl. 265(2), 229–248 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  5. Baumann, G., Stenger, F.: Fractional calculus and Sinc methods. Fract. Calc. Appl. Anal. 14(4), 568–622 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  6. Diethelm, K., Ford, N.J.: Volterra integral equations and fractional calculus: do neighboring solutions intersect? J. Integr. Eq. Appl. 24(1), 25–37 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  7. Esmaeili, S., Shamsi, M., Dehghan, M.: Numerical solution of fractional differential equations via a Volterra integral equation approach. Central Eur. J. Phys. 11(10), 1470–1481 (2013)

    Google Scholar 

  8. Brunner, H.: Collocation Methods for Volterra Integral and Related Functional Equations. Cambridge University Press, Cambridge (2004)

    Book  MATH  Google Scholar 

  9. Diogo, T.: Collocation and iterated collocation methods for a class of weakly singular Volterra integral equations. J. Comput. Appl. Math. 229(2), 363–372 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  10. Zhao, J., Xiao, J., Ford, N.J.: Collocation methods for fractional integro-differential equations with weakly singular kernels. Numer. Algorithm. 65(4), 723–743 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  11. Shen, J., Tang, T., Wang, L.: Spectral Methods: Algorithms, Analysis and Applications. Springer, Berlin (2011)

    Book  MATH  Google Scholar 

  12. Xie, Z., Li, X., Tang, T.: Convergence analysis of spectral Galerkin methods for Volterra type integral equations. J. Sci. Comput. 53(2), 414–434 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  13. Huang, C., Stynes, M.: A spectral collocation method for a weakly singular Volterra integral equation of the second kind. Adv. Comput. Math. 42(5), 1015–1030 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  14. Brunner, H., Linz, P.: Analytical and numerical methods for Volterra equations. Math. Comput. 48(178), 841 (1987)

    Article  Google Scholar 

  15. Berrut, J.P., Hosseini, S.A., Klein, G.: The linear barycentric rational quadrature method for Volterra integral equations. SIAM J. Sci. Comput. 36(1), A105–A123 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  16. Li, M., Huang, C.: The linear barycentric rational quadrature method for auto-convolution Volterra integral equations. J. Sci. Comput. 78(1), 549–564 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  17. Lubich, C. h.: Runge-Kutta theory for Volterra and Abel integral equations of the second kind. Math. Comput. 41(163), 87–102 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  18. Crisci, M.R., Jackiewicz, Z., Russo, E., Vecchio, A.: Global stability analysis of the Runge-Kutta methods for Volterra integral and integro-differential equations with degenerate kernels. Computing 45(4), 291–300 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  19. Garrappa, R.: Order conditions for Volterra RungeC̈Kutta methods. Appl. Numer. Math. 60(5), 561–573 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  20. van der Houwen, P.J., Riele, H.J.J.: Linear multistep methods for Volterra integral equations of the second kind. Queueing Systems (1982)

  21. Lubich, C. h.: Fractional linear multistep methods for Abel-Volterra integral equations of the second kind. Math. Comput. 45(172), 463–469 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  22. Garrappa, R.: On some explicit Adams multistep methods for fractional differential equations. J. Comput. Appl. Math. 229(2), 392–399 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  23. Brunner, H.: The numerical solution of weakly singular Volterra integral equations by collocation on graded meshes. Math. Comput. 45(172), 417–437 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  24. Diogo, T., McKee, S., Tang, T.: Collocation methods for second-kind Volterra integral equations with weakly singular kernels. Proc. R. Soc. Edinb. 124, 199–210 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  25. Brunner, H.: Nonpolynomial spline collocation for Volterra equations with weakly singular kernels. SIAM J. Numer. Anal. 20(6), 1106–1119 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  26. Cao, Y., Herdman, T., Xu, Y.: A hybrid collocation method for Volterra integral equations with weakly singular kernels. SIAM J. Numer. Anal. 41(1), 364–381 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  27. Rebelo, M., Diogo, T.: A hybrid collocation method for a nonlinear Volterra integral equation with weakly singular kernel. J. Comput. Appl. Math. 234(9), 2859–2869 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  28. Ford, N.J., Morgado, M.L., Rebelo, M.: Nonpolynomial collocation approximation of solutions to fractional differential equations. Fract. Calc. Appl. Anal. 16(4), 874–891 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  29. Ford, N.J., Morgado, M.L., Rebelo, M.: A nonpolynomial collocation method for fractional terminal value problems. J. Comput. Appl. Math. 275(1), 392–402 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  30. Pedas, A., Vainikko, G.: Smoothing transformation and piecewise polynomial collocation for weakly singular Volterra integral equations. Computing 73(3), 271–293 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  31. Diogo, T., Lima, P.M., Pedas, A., Vainikko, G.: Smoothing transformation and spline collocation for weakly singular Volterra integro-differential equations. Appl. Numer. Math. 114, 63–76 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  32. Hou, D., Xu, C.: A fractional spectral method with applications to some singular problems. Adv. Comput. Math. 43(5), 911–944 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  33. Pedas, A., Tamme, E., Vikerpuur, M.: Smoothing transformation and spline collocation for nonlinear fractional initial and boundary value problems. J. Comput. Appl. Math. 317, 1–16 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Cai, H., Chen, Y.: A fractional order collocation method for second kind Volterra integral equations with weakly singular kernels. J. Sci. Comput. 75(2), 970–992 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  35. Brugnano, L., Trigiante, D.: Solving differential problems by multistep initial and boundary value methods. Cordon and Breach Science Publishers (1998)

  36. Lopez, L., Trigiante, D.: Boundary value methods and BV-stability in the solution of initial value problems. Appl. Numer. Math. 11(1), 225–239 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  37. Chen, H., Zhang, C.: Boundary value methods for Volterra integral and integro-differential equations. Appl. Math. Comput. 218(6), 2619–2630 (2011)

    MathSciNet  MATH  Google Scholar 

  38. Ma, J., Xiang, S.: A collocation boundary value method for linear Volterra integral equations. J. Sci. Comput. 71(1), 1–20 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  39. Zayernouri, M., Karniadakis, G.E.: Fractional spectral collocation method. SIAM J. Sci. Comput. 36(1), A40–A62 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  40. Olver, F., Lozier, D., Boisvert, R., Clark, C.: NIST Handbook of Mathematical Functions. Cambridge University Press, Cambridge (2010)

    MATH  Google Scholar 

Download references

Funding

This work is supported by NSF of China (No. 11761020), Scientific Research Foundation for Young Talents of Department of Education of Guizhou Province (No. 2016125), Major Scientific and Technological Special Project of Guizhou Province (No. 20183001), and Science and Technology Foundation of Guizhou Province (No. QKH[2017]5788).

Author information

Authors and Affiliations

  1. Guizhou Provincial Key Laboratory of Public Big Data, Guizhou University, Guiyang, 550025, Guizhou, People’s Republic of China

    Junjie Ma & Huilan Liu

  2. School of Mathematics and Statistics, Guizhou University, Guiyang, 550025, Guizhou, People’s Republic of China

    Junjie Ma & Huilan Liu

Authors
  1. Junjie Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Huilan Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Huilan Liu.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ma, J., Liu, H. Fractional collocation boundary value methods for the second kind Volterra equations with weakly singular kernels. Numer Algor 84, 743–760 (2020). https://doi.org/10.1007/s11075-019-00777-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-019-00777-9

Keywords

Search

Navigation