Perfect quantum multiple-unicast network coding protocol

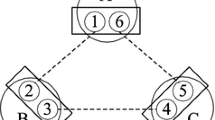
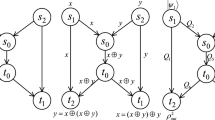
Abstract

In order to realize long-distance and large-scale quantum communication, it is natural to utilize quantum repeater. For a general quantum multiple-unicast network, it is still puzzling how to complete communication tasks perfectly with less resources such as registers. In this paper, we solve this problem. By applying quantum repeaters to multiple-unicast communication problem, we give encoding–decoding schemes for source nodes, internal ones and target ones, respectively. Source-target nodes share EPR pairs by using our encoding–decoding schemes over quantum multiple-unicast network. Furthermore, quantum communication can be accomplished perfectly via teleportation. Compared with existed schemes, our schemes can reduce resource consumption and realize long-distance transmission of quantum information.

Appendix

1.1 The proof of Theorem 1

Proof

Based on the procedure of $\text {Con}^{A_{1}}_{A_{2}\rightarrow A_{2}^{'},\ldots ,A_{m}\rightarrow A_{m}^{'}}$, we give the result step by step.

Step 1, u applies $\text {CNOT}(A_{1},A_{2})\otimes ...\otimes \text {CNOT}(A_{1},A_{m})$ to the state $|{\varPhi }_\mathrm{init}\rangle$, then

$$\begin{aligned} |{\varPsi }_{1}\rangle \triangleq&\,\text {CNOT}(A_{1},A_{2})\otimes ...\otimes \text {CNOT}(A_{1},A_{m})|{\varPsi }_\mathrm{init}\rangle \nonumber \\ =&\, |00\rangle _{A_{1}A^{'}_{1}}\otimes |{\varPsi }^{+}\rangle _{A_{2}A^{'}_{2}}\otimes ...\otimes |{\varPsi }^{+}\rangle _{A_{m}A^{'}_{m}}\otimes |{\varPhi }\rangle \nonumber \\&+ |11\rangle _{A_{1}A^{'}_{1}}\otimes |{\varPhi }^{+}\rangle _{A_{2}A^{'}_{2}}\otimes ...\otimes |{\varPhi }^{+}\rangle _{A_{m}A^{'}_{m}}\otimes |{\varPhi }\rangle . \end{aligned}$$

(8)

Step 2, u measures $A_{2},\ldots ,A_{m}$ in the basis $\{|0\rangle ,|1\rangle \}$, let measurement result be $\alpha =(a_{2},a_{3},\ldots ,a_{m})$, $a_{i}\in \{0,1\}$, then

$$\begin{aligned} \begin{aligned} |{\varPsi }_{2}\rangle \triangleq&I^{\otimes ^{2}}\otimes (M \otimes I)^{\otimes ^{m-1}}\otimes I|{\varPsi }_{1}\rangle \\ =&|00\rangle _{A_{1}A^{'}_{1}}\otimes |a_{2}a_{3}...a_{m}\rangle _{A^{'}_{2}...A^{'}_{m}}\otimes |{\varPhi }\rangle +\, |11\rangle _{A_{1}A^{'}_{1}}\otimes |\overline{a_{2}} \overline{a_{3}}...\overline{a_{m}}\rangle _{A^{'}_{2}...A^{'}_{m}}\otimes |{\varPhi }\rangle . \end{aligned} \end{aligned}$$

where $A_{2},\ldots ,A_{m}$ are disregarded since they are not entangled anymore.

Step 3, u applies $I^{\otimes ^{2}}\otimes ^{m}_{i=2}X^{a_{i}}\otimes I$ to $|{\varPsi }_{2}\rangle$, then

$$\begin{aligned} \begin{aligned} |{\varPsi }_{3}\rangle \triangleq&\,I^{\otimes ^{2}}\otimes ^{m}_{i=2}X^{a_{i}}\otimes I|{\varPsi }_{2}\rangle \\ =&\, |000...000\rangle _{A_{1} A^{'}_{1} A^{'}_{2}...A^{'}_{m}}\otimes |{\varPhi }\rangle +\,|111...111\rangle _{A_{1} A^{'}_{1} A^{'}_{2},\ldots ,A^{'}_{m}}\otimes |{\varPhi }\rangle . \end{aligned} \end{aligned}$$

$\square$

1.2 The proof of Theorem 2

Proof

Based on procedure of $\text {Con}^{B^{'}_{1},\ldots ,B^{'}_{m}}_{C_{1}\rightarrow C^{'}_{1},\ldots ,C_{n}\rightarrow C^{'}_{n}}$ over the states of the internal nodes, we give the result step by step.

Step 1, u applies $\text {CNOT}(B^{'}_{1}\oplus ...\oplus B^{'}_{m}, C_{1}) ,\ldots ,\text {CNOT}(B^{'}_{1}\oplus ...\oplus B^{'}_{m}, C_{n})$ to $|{\varPsi }_\mathrm{init}\rangle$; then

$$\begin{aligned} \begin{aligned} |{\varPsi }_{1}\rangle \triangleq&\text {CNOT}(B^{'}_{1}\oplus ...\oplus B^{'}_{m}, C_{1})\otimes \cdots \otimes \text {CNOT}(B^{'}_{1}\oplus ...\oplus B^{'}_{m}, C_{n})|{\varPsi }_\mathrm{init}\rangle \\ =&\left( \sum _{b_{1}+\cdots +b_{m}=0\pmod {2}}|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}} \otimes |{\varPsi }^{+}\rangle _{C_{1}C^{'}_{1}}\otimes ...\otimes |{\varPsi }^{+}\rangle _{C_{n}C^{'}_{n}}\right) \otimes |{\varPhi }\rangle \\&\quad +\left( \sum _{b_{1}+\cdots +b_{m}=1\pmod {2}}|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}} \otimes |{\varPhi }^{+}\rangle _{C_{1}C^{'}_{1}}\otimes ...\otimes |{\varPhi }^{+}\rangle _{C_{n}C^{'}_{n}}\right) \otimes |{\varPhi }. \end{aligned} \end{aligned}$$

(9)

Step 2, u measures $C_{1},\ldots ,C_{n}$ in the basis ${\{|0\rangle ,|1\rangle \}}$, if measurement result $\beta =(\beta _{1},\ldots ,\beta _{n}),\beta _{i}\in \{0,1\}$, the state becomes

$$\begin{aligned} \begin{aligned} |{\varPsi }_{2}\rangle \triangleq&I^{\otimes ^{m}}\otimes (M \otimes I)^{\otimes ^{n}}\otimes I|{\varPsi }_{1}\rangle \\ =&\left( \sum _{b_{1}+\cdots +b_{m}=0\pmod {2}}|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}} \otimes |\beta _{1}\beta _{2}...\beta _{n}\rangle _{C^{'}_{1},..,C^{'}_{n}}\right) \otimes |{\varPhi }\rangle \\&\quad +\left( \sum _{b_{1}+\cdots +b_{m}=1\pmod {2}}|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}} \otimes |\overline{\beta _{1}},\overline{\beta _{2}},\ldots ,\overline{\beta _{n}}\rangle _{C^{'}_{1},..,C^{'}_{n}}\right) \otimes |{\varPhi }\rangle .\\ \end{aligned} \end{aligned}$$

(10)

Step 3, u applies $I^{\otimes m}\otimes ^{n}_{i=2}X^{\beta _{i}}\otimes I$ to $|{\varPsi }_{2}\rangle$, then

$$\begin{aligned} \begin{aligned} |{\varPsi }_{3}\rangle =&I^{\otimes m}\otimes ^{n}_{i=2}\sigma ^{\beta _{i}}_{X}\otimes I|{\varPsi }_{2}\rangle \\ =&\left( \sum _{b_{1}+\cdots +b_{m}=0\pmod {2}}|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}} \otimes |00...0\rangle _{C^{'}_{1},..,C^{'}_{n}}\right) \otimes |{\varPhi }\rangle \\&\quad +\,\left( \sum _{b_{1}+\cdots +b_{m}=1\pmod {2}}|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}} \otimes |11...1\rangle _{C^{'}_{1},..,C^{'}_{n}}\right) \otimes |{\varPhi }\rangle . \end{aligned} \end{aligned}$$

(11)

$\square$

1.3 The proof of Theorem 4

Proof

Based on procedure of $\text {Delete} C^{'}_{i}\rightarrow {B^{'}_{1},\ldots ,B^{'}_{m}}$, we give the conclusion step by step.

Step 1, u applies Hadamard operator to $C^{'}_{i}$ ; then,

$$\begin{aligned} \begin{aligned} |{\varPsi }_{1}\rangle \triangleq&I^{\otimes ^{m}}\otimes H |{\varPsi }_\mathrm{init}\rangle \\ =&|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|+\rangle _{C^{'}_{i}} +|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|-\rangle _{C^{'}_{i}}. \end{aligned} \end{aligned}$$

(12)

Step 2, u measures $C^{'}_{i}$ in the basis ${\{|0\rangle ,|1\rangle \}}$,

when $a=0$, the result is

$$\begin{aligned} \begin{aligned} |{\varPsi }_{2_{0}}\rangle =&|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|0\rangle _{C^{'}_{i}} +|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|0\rangle _{C^{'}_{i}}, \end{aligned} \end{aligned}$$

(13)

when $a=1$, the result is

$$\begin{aligned} \begin{aligned} |{\varPsi }_{2_{1}}\rangle =&|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|0\rangle _{C^{'}_{i}} -|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|0\rangle _{C^{'}_{i}}. \end{aligned} \end{aligned}$$

(14)

Step 3, if $a=1$, then u applies $Z^{\otimes m}\otimes I$ to $|{\varPsi }_{2_{1}}\rangle$, we get

$$\begin{aligned} \begin{aligned} |{\varPsi }_{3}\rangle \triangleq&\sigma _{Z}^{\otimes m}\otimes I|{\varPsi }_{2_{1}}\rangle \\ =&|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|0\rangle _{C^{'}_{i}} +|b_{1}...b_{m}\rangle _{B^{'}_{1},\ldots ,B^{'}_{m}}|0\rangle _{C^{'}_{i}}. \end{aligned} \end{aligned}$$

(15)

$C^{'}_{i}$ can be disregarded because it is not entangled anymore. $\square$