Performance analysis of the satellite-to-ground continuous-variable quantum key distribution with orthogonal frequency division multiplexed modulation

Abstract

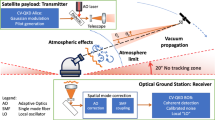
Continuous-variable quantum key distribution (CVQKD) is aiming at widespread application and adoption in different scenarios, and thus, the application of satellite-to-ground link may play a vital role in establishing the global secure quantum communications. In this paper, we propose an improved tunable CVQKD scheme for the satellite-to-ground free space optical (FSO) link in an orthogonal frequency division multiplexing (OFDM) system. The OFDM-based CVQKD can effectively suppress the random fading effect that resulted from the atmospheric channel since it divides the initial transmission channel into multi-subcarriers working in parallel so as to compensate the weakness of single-channel transmission of the signals. Moreover, the influence of the intensity scintillation, atmospheric transmittance and phase noise caused by atmospheric turbulence is involved in security analysis. The results reveal that satellite-to-ground CVQKD system can reduce the atmosphere influence in an OFDM system. Compared with the single-channel CVQKD in the FSO link, the secret key rate is improved with the OFDM technique within a certain range of subcarrier numbers. Nevertheless, the reduction of symbols per channel cannot make the asymptotic assumption due to the limitation of technology and devices.

Appendices

A The scintillation index analysis

In this appendix, we illustrate the Rytov variance ${\delta }^2_{R}$ in Sect. 2.1. Rytov variance, which is used to estimate the optical fluctuation intensity, can be defined as

$$\begin{aligned} {\delta }^2_{R}=2.25k^{7/6}sec^{11/6}(\zeta ) \int _{h_0}^{H} C_n^2 (h)(h-h_0)^{5/6}\mathrm {d}h, \end{aligned}$$

(26)

where $k=2 \pi / \lambda$ is the optical wave number, $H=h_0+Lcos \zeta$ is the satellite altitude, $h_0$ is the height above ground level, L is the propagation distance, $\zeta$ is the zenith angle, and $C_n^2(h)$ is the refraction index structure constant parameter. When ${\delta }^2_{R} < 1$, it indicates the weak turbulence, when ${\delta }^2_{R} \cong 1$, it indicates the medium turbulence, and when ${\delta }^2_{R} > 1$, it indicates the strong turbulence. As for $C_n^2(h)$, one of the most widely used models is the Hufnagel–Valley (H–V) model described by [42]

$$\begin{aligned} \begin{aligned} C_n^2(h) = 0.00594(v/27)^2(10^{-5}h)^{10} e^{-\frac{h}{1000}} +2.7 \times 10^{-16} e^{-\frac{h}{1500}}+Ae^{-\frac{h}{100}}, \end{aligned} \end{aligned}$$

(27)

where v is the pseudowind in meters per second (m/s) and A is the nominal value of $C_n^2(0)$ at the ground in $m^{-2/3}$.

Figure 10 shows $C_n^2$ in the satellite altitude of 0 km, 5 km, 15 km and 20 km based on the varying wind speed v and constant $C_n^2(0)$. In the satellite-to-ground scenario, $C_n^2(0)$ expresses the atmospheric refraction structure above the ocean near-surface. From the weather research and forecasting (WRF) model outputs [23], the near-surface refractive index structure constant is mainly varying in the range of $10^{-15}{-} 10^{-13}\,\mathrm {m}^{-2/3}$. The wind speed v can be extracted from the ECMWF Re-Analysis (ERA-Interim) data set [43], which ranges roughly from 0 to 25 m/s. We can see clearly that the ocean near-surface turbulence level has little effect above 5 km and the wind speed governs the profile behavior primarily in the vicinity of 10 km. Accordingly, the atmospheric turbulence could be negligible when the altitude is higher than 10 km.

Fig. 10

The refraction structure $C_n^2$ at altitudes 0 km, 5 km, 10 km and 20 km

B The atmospheric transmittance analysis

In this appendix, we illustrate the expressions of the above parameters in Sect. 2.2. The transmittance $T_0$ can be estimated by [12]

$$\begin{aligned} T_0= & {} 1 -I_0 \left( a^2 \left[ \frac{1}{W_1^2}-\frac{1}{w_2^2} \right] \right) {\mathrm {exp}} \left[ -a^2 \left( \frac{1}{W_1^2} + \frac{1}{W_2^2} \right) \right] -2 \left\{ 1-{\mathrm {exp}} \left[ -\frac{a^2}{2} \left( \frac{1}{W_1} - \frac{1}{W_2} \right) ^2 \right] \right\} \nonumber \\&\times {\mathrm {exp}} \left\{ - \left[ \frac{ \frac{(W_1+W_2)^2}{|W_1^2-W_2^2|}}{R\left( \frac{1}{W_1}-\frac{1}{W_2}\right) } \right] ^{ \lambda \left( \frac{1}{W_1}-\frac{1}{W_2}\right) } \right\} . \end{aligned}$$

(28)

$R(\cdot )$ and $\lambda (\cdot )$, which are scale and shape functions, respectively,

$$\begin{aligned} R(\xi )= & {} \left[ {\mathrm {ln}} \left( 2 \frac{1-{\mathrm {exp}}[-\frac{1}{2}a^2\xi ^2]}{1-{\mathrm {exp}}[-a^2\xi ^2 ]I_0 (a^2\xi ^2) } \right) \right] ^{ -\frac{1}{\lambda (\xi )}} , \end{aligned}$$

(29)

$$\begin{aligned} \lambda (\xi )= & {} 2a^2 \xi ^2 \frac{{\mathrm {exp}}(-a^2\xi ^2) I_1(a^2\xi ^2)}{1-{\mathrm {exp}}(-a^2\xi ^2)I_0(a^2\xi ^2)} \times \left[ {\mathrm {ln}} \left( 2 \frac{1-{\mathrm {exp}}[-\frac{1}{2}a^2\xi ^2]}{1-{\mathrm {exp}}[-a^2\xi ^2 ]I_0 (a^2\xi ^2) } \right) \right] ^{-1}\!,\nonumber \\ \end{aligned}$$

(30)

where $I_i(\cdot )$ is the modified Bessel function of i-th order [44]. For the given angle $\chi =\phi -\varphi _0$, the effective spot radius $W_{\mathrm {eff}}(\cdot )$ can be approximated by

$$\begin{aligned} \begin{aligned} W_{\mathrm {eff}}^2(\chi ) = 4a^2 \left\{ {\mathscr {W}} \left( \frac{4a^2}{W_1W_2} {\mathrm {exp}}\left[ \frac{a^2}{W_1^2} (1+2{\mathrm {cos}}^2\chi ) \right] \times {\mathrm {exp}} \left[ \frac{a^2}{W_2^2}(1+2\mathrm {sin}^2 \chi ) \right] \right) \right\} , \end{aligned} \end{aligned}$$

(31)

Here, ${\mathscr {W}}(\cdot )$ is the Lambert W function [45].

Based on Eqs. (6), (28)–(31), the probability distribution of the atmospheric transmittance can be evaluated using the Monte Carlo method [46]. In the above model, there are five parameters $(x_0,y_0,W_1,W_2,\phi )$. As shown in Fig. 11, parameter $\phi$ is uniformly distributed and independent of others. Besides, parameters $(W_1,W_2)$ can be obtained by multiplying large number of small random contributions, which gives a good argument for assuming that $(W_1,W_2)$ is log-normally distributed. Therefore, random parameters $\varTheta _1={\mathrm {ln}} \frac{W_1}{W_0}$ and $\varTheta _2={\mathrm {ln}} \frac{W_2}{W_0}$ are introduced to calculate the probability distribution of the atmospheric transmittance, where $W_0$ is the transmitter beam. The correlation of $(x_0,y_0,\varTheta _1,\varTheta _2)$ could be defined by its covariance matrix, which reads [12, 26]

(32)

Combing the results of the scintillation index in Eqs. (26)–(27) with the turbulent regimes, the satellite-to-ground link can be regarded to be affected by weak-to-moderate turbulence ($\delta _R^2 < 1$). The elements of $\varXi$ is expressed by [26]

$$\begin{aligned} \begin{aligned} \langle x_0^2\rangle&= \langle y_0^2\rangle =0.33W_0^2 \delta _R^2 \varOmega ^{-\frac{7}{6}} , \\ \langle \varTheta _1^2 \rangle&= \langle \varTheta _2^2 \rangle ={\mathrm {ln}} \left[ 1+\frac{1.2\delta _R^2 \varOmega ^{\frac{5}{6}}}{(1+2.96\delta _R^2 \varOmega ^{\frac{5}{6}} )^2} \right] ,\\ \langle \varTheta _1 \varTheta _2 \rangle&= \langle \varTheta _2^2 \rangle ={\mathrm {ln}} \left[ 1-\frac{0.8\delta _R^2 \varOmega ^{\frac{5}{6}}}{(1+2.96\delta _R^2 \varOmega ^{\frac{5}{6}} )^2} \right] , \end{aligned} \end{aligned}$$

(33)

where $\varOmega =\frac{kW_0^2}{2L}$ is the Fresnel parameter of the beam and their expectations read

$$\begin{aligned} \begin{aligned} \langle x_0\rangle&= \langle y_0\rangle =0 ,\\ \langle \varTheta _1 \rangle&= \langle \varTheta _2 \rangle = {\mathrm {ln}} \left[ \frac{ \left( 1+2.96\delta _R^2 \varOmega ^{\frac{5}{6}} \right) ^2 }{ \varOmega ^2 \sqrt{ \left( 1+2.96\delta _R^2 \varOmega ^{\frac{5}{6}} \right) ^2 +1.2\delta _R^2 \varOmega ^{\frac{5}{6}} } } \right] . \end{aligned} \end{aligned}$$

(34)

Fig. 11

The aperture of radius a and the elliptical beam profile with the half axis $W_1$ rotated on the angle $\phi$ relative to the x-axis and on the angle $\chi$ relative to the $r_0$-associated axis are shown

C Crosstalk noise on the subchannels

In this appendix, we illustrate crosstalk noise in Sect. 4. The effect of interchannel crosstalk in the modulation is depicted in Fig. 12. The crosstalk information $\gamma _i (N_i)$ on the i-th subchannel $N_i$ is expressed as [34]

$$\begin{aligned} \gamma _i (N_i) = \sum _{j\ne i} x_j \chi (A_j:B_j) , \end{aligned}$$

(35)

where $0 \le x_j \le 1$, $\chi (A_j:B_j)=H(B_j)-H(B_j|A_j)$ is the Holevo information of Alice and Bob conveyed by the neighboring subchannel $N_j$. Since the crosstalk noise $\gamma _i (N_i)$ on the subchannel $N_i$ acts for Bob as Gaussian noise, it can be modeled by $\gamma _i (N_i) \in \mathscr {C} {\mathbb {N}} (0,\sigma ^2_{\gamma _i})$ with variance $\sigma ^2_{\gamma _i} {\mathbb {E}} [|\gamma _i|^2]$. This additional noise does not change the rate formulas of the system, since this additional noise is already contained in the subchannel’s noise variance $\sigma _{N_i}^2$ (see Ref. [41], Theorem 2). In other words, the mutual information between Alice and Bob is completely characterized for a given $N_i$ by the noise variance $\sigma _{N_i}^2$, which can be decomposed as

$$\begin{aligned} \sigma _{N_i}^2 = \sigma _{E_i}^2 + \sigma _{\gamma _i}^2. \end{aligned}$$

(36)

where $\sigma _{E_i}^2$ is the noise variance of Eve’s optimal Gaussian collective attack. Accordingly, the $\chi (B:E)$ Holevo information of Eve changes is as follows [34]

$$\begin{aligned} \chi (B:E)_{\gamma _i} = \chi (B:E) +\sum _{n} \sum _{j\ne i} |T_{E,i}|^2 x_j \chi (A_j:B_j) . \end{aligned}$$

(37)

Furthermore, crosstalk noise has no effect on the security of the modulation, since it allows no more leaking of information to an eavesdropper than single-carrier CVQKD protocols (see Ref. [34], Theorem 4). Moreover, the influence of excess noise has been analyzed in Ref. [40].

Fig. 12

The effect of crosstalk noise between subchannels. Due to the imperfections of practical devices, some information from neighboring subchannels can be leaked to a given subchannel, which acts as noise to the receiver