Proof-of-principle demonstration of decoy-state quantum key distribution with biased basis choices

Abstract

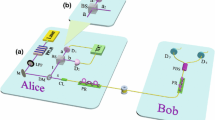
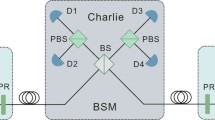
Decoy-state method has been widely employed in the quantum key distribution (QKD), since it can not only solve photon-number-splitting attacks but also substantially improve the QKD performance. In conventional three-intensity decoy-state proposal, one not only needs to randomly modulate light sources to different intensities, but also has to prepare them into different bases, which may cost a lot of random numbers in practical applications. Here, we propose a simple decoy-state scheme with biased basis choices where the decoy pulses are only prepared in X basis. Through this way, it can save cost of random numbers and further simplify the electronic control system. Moreover, we carry out corresponding proof-of-principle demonstration. By incorporating with lower-loss asymmetric Mach–Zehnder interferometers and superconducting single-photon detectors, we can obtain a secret key rate of 1.65 kbps at 201 km and 19.5 bps at 280 km coiled optical fibers, respectively, showing very promising applications in future quantum communications.

Appendix

In this appendix, we will give the derivation process of Eqs. (1) and (2).

For the WCS, the probability of finding an i-photon state with intensity $\xi$$(\xi \in \{ \mu , \nu \})$ is given by: $P_{\xi }(i) = \frac{\xi ^{i}}{i!}e^{-\xi }$. Then, we have

$$\begin{aligned} \frac{P_{\mu }(i)}{P_{\nu }(i)} -\frac{P_{\mu }(i-1)}{P_{\nu }(i-1)} =&\frac{\frac{\mu ^i}{i!} e^{-\mu }}{\frac{\nu ^i}{i!} e^{-\nu }} - \frac{\frac{\mu ^{i-1}}{(i-1)!} e^{-\mu }}{\frac{\nu ^{i-1}}{(i-1)!} e^{-\nu }}\nonumber \\ =&\left( \frac{\mu }{\nu }\right) ^{i-1}e^{-(\mu -\nu )}\left( \frac{\mu }{\nu }-1\right) . \end{aligned}$$

(A1)

For any $i \ge 2$, when $\mu>\nu >0$, $\frac{\mu }{\nu }>1$, therefore, the following inequalities hold

$$\begin{aligned} \dfrac{P_{\mu }(i)}{P_{\nu }(i)} \ge \dfrac{P_{\mu }(2)}{P_{\nu }(2)} \ge \dfrac{P_{\mu }(1)}{P_{\nu }(1)}. \end{aligned}$$

(A2)

The Proof for Eq. (1) of the main text is done.

The gains of signal and decoy states can be written as:

$$\begin{aligned} Q_{\mu }^{X}= & {} \sum _{i=0}^\infty Y_{i}^{X}P_{\mu }(i) = P_{\mu }(0)Y_{0}+ P_{\mu }(1)Y_{1}^{X}+ \sum _{i=2}^\infty P_{\mu }(i)Y_{i}^{X}, \end{aligned}$$

(A3)

$$\begin{aligned} Q_{\nu }^{X}= & {} \sum _{i=0}^\infty Y_{i}^{X}P_{\nu }(i) = P_{\nu }(0)Y_{0}+ P_{\nu }(1)Y_{1}^{X}+ \sum _{i=2}^\infty P_{\nu }(i)Y_{i}^{X}. \end{aligned}$$

(A4)

With Eqs. (A3) and (A4), it is easy to get

$$\begin{aligned} Q_{\nu }^{X}P_{\mu }(2)-Q_{\mu }^{X}P_{\nu }(2)&=[P_{\nu }(0)P_{\mu }(2)-P_{\mu }(0)P_{\nu }(2)]Y_{0}+[P_{\nu }(1)P_{\mu }(2)\nonumber \\&\quad -P_{\mu }(1)P_{\nu }(2)]Y_{1}^{X} + \sum _{i=2}^\infty [P_{\nu }(i)P_{\mu }(2)-P_{\mu }(i)P_{\nu }(2)]Y_{i}^{X}. \end{aligned}$$

(A5)

Considering the conditions in Eq. (A2), we can get the following inequality:

$$\begin{aligned}&Q_{\nu }^{X}P_{\mu }(2)-Q_{\mu }^{X}P_{\nu }(2)\le [p_{\nu }(0)p_{\mu }(2)-p_{\mu }(0)p_{\nu }(2)]Y_{0}+[p_{\nu }(1)p_{\mu }(2)\nonumber \\&\quad -p_{\mu }(1)p_{\nu }(2)]Y_{1}^{X}. \end{aligned}$$

(A6)

It is easy to reach

$$\begin{aligned} Y_{1}^{X}\ge \frac{Q_{\nu }^{X}P_{\mu }(2)-Q_{\mu }^{X}P_{\nu }(2)-[P_{\nu }(0)P_{\mu }(2)-P_{\mu }(0)P_{\nu }(2)]Y_{0}}{P_{\nu }(1)P_{\mu }(2)-P_{\mu }(1)P_{\nu }(2)}. \end{aligned}$$

(A7)

The proof for Eq. (2) of the main text is finished.