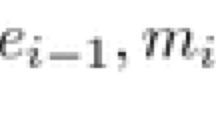Epistemic inconsistency and categorical coherence: a study of probabilistic measures of coherence

Abstract

Is logical consistency required for a set of beliefs or propositions to be categorically coherent? An affirmative answer is often assumed by mainstream epistemologists, and yet it is unclear why. Cases like the lottery and the preface call into question the assumption that beliefs must be consistent in order to be epistemically rational. And thus it is natural to wonder why all inconsistent sets of propositions are incoherent. On the other hand, Easwaran and Fitelson (2015) have shown that particular kinds of inconsistency entail the epistemically ‘irrationality’ of holding certain sets of beliefs. In cases of the latter kind of inconsistency, it seems more reasonable to insist that such sets of beliefs or propositions are categorically incoherent. What the precise relationship is between coherence and consistency depends on the nature of the coherence relation. We shall examine recent attempts to explicate the coherence relation in terms of probabilistic measures of confirmation or agreement to see what they can teach us about the relationship between coherence and consistency. We shall show that some probabilistic measures of coherence allow for inconsistent sets to be categorically coherent, while satisfying plausible epistemic rationality constraints. Other probabilistic measures of coherence impose very strong logical consistency requirements, and some measures are tolerant of most forms of inconsistency. As we try to understand what distinguishes coherence measures in this respect, we will also draw some important lessons about Bayesian confirmation measures and differences in the way that they treat contradictory propositions.

Appendices

Appendices

Appendix 1: Proof of Lemmas needed for later proofs

Lemma 1

For any $\varphi '$ and $\psi '$ where $\psi '\ne \bot$, if $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$ then $m(\varphi ',\,\psi ')\le Pr(\varphi '|\psi ')$ for all Pr.

Proof

The claim follows by definition in the cases where $m\in \{d_{1}^{*},\ d_{2}^{*}\}$. Thus, we just need to give a demonstration for when $m\in \{\lambda ^{*},\ z^{*}\}$. We begin by first proving the conditional holds for $m=z^{*}$. We prove this by deriving the inequality in the consequent from the following theorem of the probability calculus:

$$\begin{aligned} \begin{array}{c} \begin{array}{cc} 1\ge Pr(\varphi '|\psi ') &{} \ \ (Th\end{array}1)\\ \Updownarrow \\ -Pr(\varphi ')\le -Pr(\varphi ')\cdot Pr(\varphi '|\psi ')\\ \Updownarrow \\ Pr(\varphi '|\psi ')-Pr(\varphi ')\le Pr(\varphi '|\psi ')-Pr(\varphi ')\cdot Pr(\varphi '|\psi ')\\ \Updownarrow \\ \dfrac{Pr(\varphi '|\psi ')-Pr(\varphi ')}{[1-Pr(\varphi ')]}\le Pr(\varphi '|\psi ')\\ \Updownarrow \\ \dfrac{Pr(\varphi '|\psi ')-Pr(\varphi ')}{Pr(\lnot \varphi ')}\le Pr(\varphi '|\psi ') \end{array} \end{aligned}$$

So whenever $\psi '$ provides some confirmation for $\varphi '$, $z^{*}(\varphi ',\ \psi ')\le Pr(\varphi '|\psi ')$. And whenever $\psi '$ fails to provide confirmation for $\varphi '$, $z(\varphi ',\ \psi ')\le 0\le Pr(\varphi '|\psi ')$. Thus, the Lemma holds in the case where $m=z^{*}$. We follow a similar strategy for our proof in the case where $m=\lambda ^{*}$. $\square$

Proof

$$\begin{aligned} \begin{array}{c} \begin{array}{cc} 1\ge Pr(\varphi '|\psi ') &{} \ \ (Th\end{array}1)\\ \Updownarrow \\ -Pr(\varphi '|\lnot \psi ')\le -Pr(\varphi '|\lnot \psi ')\cdot Pr(\varphi '|\psi ')\\ \Updownarrow \\ Pr(\varphi '|\psi ')-Pr(\varphi '|\lnot \psi ')\le Pr(\varphi '|\psi ')-Pr(\varphi '|\psi ')\cdot Pr(\varphi '|\lnot \psi ')\\ \Updownarrow \\ Pr(\varphi '|\psi ')-Pr(\varphi '|\lnot \psi ')\le Pr(\varphi '|\psi ')\cdot [1-Pr(\varphi '|\lnot \psi ')]\\ \Updownarrow \\ \dfrac{Pr(\varphi '|\psi ')-Pr(\varphi '|\lnot \psi ')}{1-Pr(\varphi '|\lnot \psi ')}\le Pr(\varphi '|\psi ') \end{array} \end{aligned}$$

Thus, whenever $\psi '$ provides some confirmation for $\varphi '$, $\lambda (\varphi ',\ \psi ')\le Pr(\varphi '|\psi ')$. And whenever $\psi '$ fails to provide confirmation for $\varphi '$, $\lambda (\varphi ',\ \psi ')\le 0\le Pr(\varphi '|\psi ')$. Thus, the Lemma holds in the case where $m=\lambda$. $\square$

Lemma 2

For any $\varphi '$ and $\psi '$, if $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$, then $m(\varphi ',\,\psi ')\le a(\varphi ',\ \psi ')$.

Proof

If $Pr(\varphi '|\psi ')>0$ then it follows that $\psi '\ne \bot$, so Lemma 1 entails that $m(\varphi ',\,\psi ')\le Pr(\varphi '|\psi ')=a(\varphi ',\ \psi ')$ for $m\in \{z,\ \lambda \}$. If $Pr(\varphi '|\psi ')=0$ or $\psi '=\bot$, then $m(\varphi ',\,\psi ')\le 0=a(\varphi ',\ \psi ')$ for all $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$.

Appendix 2: Proof of Theorem 1

Theorem 1

If an agent believes all of the members of $\varDelta$ and the resulting set of judgments satisfies (R), then $\varDelta$ does not contain any subsets that are logically mostly false.

Since Easwaran and Fitelson prove a slightly more general claim, we needn’t give the full proof. Instead, we aim to explain why the above follows from their more general theorem. The first step is to recall their notion of a witnessing$_{2}$ set.

Definition 8

S is a witnessing $_{2}$ set iff at every world w, at least half of the judgments in S are inaccurate. (Easwaran and Fitelson 2015, p. 86)

Easwaran and Fitelson (2015, p. 93) prove that any set of judgments that satisfies (R) does not contain a witnessing $_{2}$ set. Thus, all that remains to be shown is that if one were to believe all members of a set of propositions that contains a subset that is logically mostly false, then the resulting judgment set would contain a witnessing$_{2}$ set.

Let us suppose an agent believes each member of a set of propositions $\varDelta$ and that $\varDelta$ contains a subset $\varGamma$ that is logically mostly false. The set of judgments, call it $S_{\varGamma }$, such that each of the members of $\varGamma$ is true will then be a witnessing$_{2}$ set. To prove this, for the sake of a reductio, let us suppose $S_{\varGamma }$ is not a witnessing$_{2}$ set. Then there is a world w where more than half of the members of $S_{\varGamma }$ are accurate, which holds if and only if more than half of the members of $\varGamma$ are true at w. Let $\varGamma _{w}=\{\varphi :\ w\vDash \varphi \ \& \ \varphi \in \varGamma \}$. By hypothesis, $|\varGamma _{w}|>\dfrac{|\varGamma |}{2}$ and $\varGamma _{w}$ is satisfiable. Thus, we would then derive that $\varGamma$ is not logically mostly false contrary to our assumption for the reductio.

What we have thus shown is that if an agent believes all members of a set that contains a subset that is logically mostly false, it follows that the resulting set of judgments contains a witnessing$_{2}$ set, and, from Easwaran and Fitelson’s proof (2015, p. 93), it follows that the resulting judgment set violates (R). Thus, Theorem 1 follows from their proof.

Appendix 3: Proof of Theorem 2

Theorem 2

If .

The proof proceeds via a few simple observations. First, assuming $\varDelta$ is inconsistent, any pair, $\langle \varGamma ,\,\varGamma '\rangle$, that partitions $\varDelta$ will be such that $a(\langle \varGamma ,\,\varGamma '\rangle )=0$. Thus, we divide the pairs in $[\varDelta ]$ into those that partition $\varDelta$ and those that don’t.

Definition 9

$[\varDelta ]^{P}=_{def}\{\langle \varGamma ,\,\varGamma '\rangle |\,\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]$ and $\varGamma \cup \varGamma =\varDelta \}$.

Definition 10

$[\varDelta ]^{NP}=_{def}\{\langle \varGamma ,\,\varGamma '\rangle |\,\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]$ and $\varGamma \cup \varGamma \ne \varDelta \}$.

Now, $C_{a}$ is the average of the values assigned to all of the subset pairs in $[\varDelta ]$. Since those that parition $\varDelta$ are all assigned 0, we have it that:

$$\begin{aligned} C_{a}(\varDelta )=\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{NP}}a(\langle \varGamma ,\,\varGamma '\rangle )}{|[\varDelta ]|} \end{aligned}$$

Next, we define a bijection from $[\varDelta ]^{NP}$ to $[\varDelta ]^{NP}$ as follows.

Definition 11

$f:[\varDelta ]^{NP}\rightarrow [\varDelta ]^{NP}$ such that $f(\langle \varGamma ,\,\varGamma '\rangle )=\langle \varDelta -\varGamma \cup \varGamma ',\,\varGamma '\rangle$.

The key thing to note is that $\varDelta -\varGamma \cup \varGamma '$ is incompatible with $\varGamma$ on the assumption that $\varGamma '$ is satisfied. Thus, f takes each element in $[\varDelta ]^{{ NP}}$ to a unique element in $[\varDelta ]^{NP}$ such that the sum of the values assigned to both by $a(\cdot )$ is less than or equal to 1. In other words, we chose f so that the following holds:

$$\begin{aligned} a(\langle \varGamma ,\,\varGamma '\rangle )\ +\ a(f(\langle \varGamma ,\,\varGamma '\rangle )\le 1 \end{aligned}$$

Since f is a bijection on $[\varDelta ]^{NP}$, it follows that

$$\begin{aligned} C_{a}(S)=\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{NP}}a(\langle \varGamma ,\,\varGamma '\rangle )}{|[\varDelta ]|}=\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{NP}}a(f(\langle \varGamma ,\,\varGamma '\rangle ))}{|[\varDelta ]|} \end{aligned}$$

And, hence, we have it that

$$\begin{aligned} =\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{NP}}a(\langle \varGamma ,\,\varGamma '\rangle )+a(f(\langle \varGamma ,\,\varGamma '\rangle ))}{2\cdot |[\varDelta ]|} \end{aligned}$$

From the fact that $a(\langle \varGamma ,\,\varGamma '\rangle )\ +\ a(f(\langle \varGamma ,\,\varGamma '\rangle )\le 1$, it follows that

Appendix 4: Proof of Theorem 3

Theorem 3

Let $m\in \{r_{1}^{*},\ r_{2}^{*}\}$. Then for any $r>0$, there exists an inconsistent set $\varDelta$ and probability function Pr s.t. $\mathcal {C}_{m,\, Pr}(\varDelta )>r$.

Proof

The proof is actually quite simple. Consider the probability distribution in Figure 1. Suppose $m\in \{r_{1}^{*},\ r_{2}^{*}\}$ and $\varDelta =\{h_{1},\ h_{2},\ e\}$. Then we have it that

$$\begin{aligned} \mathcal {C}_{m}(\varDelta )=\dfrac{\sum \limits _{\langle \varGamma ,\ \varGamma '\rangle }m(\bigwedge \varGamma ,\ \bigwedge \varGamma ')}{12}\ge \dfrac{m(h_{1},\ e)-11}{12} \end{aligned}$$

Since $m(h_{1},\ e)$ converges to $\infty$ as x tends to 0 and y tends to 1 on both confirmation measures, the above value converges to $\infty$. $\square$

Appendix 5: Proof of Theorems 4 and 6

Theorem 4

For any $\epsilon >0$, there exists an inconsistent set $\varDelta$ and probability function Pr s.t. $C_{F,\, Pr}(\varDelta )>1-\epsilon$.

Theorem 6

Let $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$. Then for any $\epsilon >0$, there exists an inconsistent set $\varDelta$ and probability function Pr s.t. .

In our proof of Theorems 4 and 6, we are going to consider a particular sort of inconsistent set of propositions. The probability function over the propositions will be structured similarly to that of a standard lottery paradox, except that we shall assume that either all of the propositions are false, or else exactly one of the propositions is false. The probability space will collapse into the probability space of a standard lottery paradox on the assumption that at least one of the lottery propositions is true (One could form each proposition explicitly by conjoining each lottery proposition from an n ticket lottery to some low probability proposition, i.e., we could let $g_{i} \text{ be } l_{i}\wedge p$ where $l_{i}$ is a standard lottery proposition and p is some proposition with a very low probability). And, we allow the probability that all of the lottery propositions are false be $1-r$ (in the explicit formulation, that would be to set $Pr(g_i)=r\cdot \dfrac{n-1}{n}$). The important assumptions are that we let $\varDelta =\{\varphi _{1},...,\ \varphi _{n}\}$ be a minimally inconsistent set of propositions where

(A1) $Pr(\varphi _{i})<r$,

(A2) For all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]$ where $|\varGamma |=j$ and $|\varGamma '|=k$,

$$\begin{aligned} Pr(\bigwedge \varGamma |\bigwedge \varGamma ')=\dfrac{n-(j+k)}{n-k} \end{aligned}$$

(On the assumption that exactly one proposition in $\varDelta$ is false, something that holds if $\bigwedge \varGamma '$ is true, $\bigwedge \varGamma$ is true just in case $\varGamma$ doesn’t contain the one false proposition, and there are $n-(j+k)$ tickets not in $\varGamma$ that could be the false proposition out of the $n-k$ propositions not in $\varGamma '$).

The next step is to note that given our assumptions above, it immediately follows that

(A3) For all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$, $Pr(\bigwedge \varGamma |\lnot \bigwedge \varGamma ')=\dfrac{Pr(\bigwedge \varGamma \wedge \lnot \bigwedge \varGamma ')}{Pr(\lnot \bigwedge \varGamma ')}\le \dfrac{r}{1-r}\ge r$

And from (A3) and the definition of our confirmation measures, we get that

(F1) For all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$, $F^{*}(\varGamma ,\ \varGamma ')=\dfrac{Pr(\bigwedge \varGamma |\bigwedge \varGamma ')-Pr(\bigwedge \varGamma |\lnot \bigwedge \varGamma ')}{Pr(\bigwedge \varGamma |\bigwedge \varGamma ')+Pr(\bigwedge \varGamma |\lnot \bigwedge \varGamma ')}\ge \dfrac{\dfrac{1}{n}-\dfrac{r}{1-r}}{\dfrac{1}{n}+\dfrac{r}{1-r}}$

(F2) Let $m\in \{\lambda ^{*},\ d_{2}^{*}\}$. For all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$,

$$\begin{aligned} m(\varGamma ,\ \varGamma ')\ge Pr(\bigwedge \varGamma |\bigwedge \varGamma ')-Pr(\bigwedge \varGamma |\lnot \bigwedge \varGamma ')\ge Pr(\bigwedge \varGamma |\bigwedge \varGamma ')-\dfrac{r}{1-r} \end{aligned}$$

Next, we must note that $Pr(\bigwedge \varGamma )\le r\le \dfrac{r}{1-r}$ , and so

(F3) Let $m\in \{z^{*},\ d_{1}^{*}\}$. Then for all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$, $m(\varGamma ,\ \varGamma ')\ge Pr(\bigwedge \varGamma |\bigwedge \varGamma ')-Pr(\bigwedge \varGamma )\ge Pr(\bigwedge \varGamma |\bigwedge \varGamma ')-\dfrac{r}{1-r}$

In all three cases, given that the conditional probabilities are independent of r, the strength of the confirmation that subset pairs in $[\varDelta ]^{NP}$ provide to one another increases as r approaches 0. In fact, in the case of F, each subset pair in $[\varDelta ]^{NP}$ provides near maximal confirmation for one another as r approaches 0. And a nearby result holds for the other two confirmation measures. Consider again our bijection on $[\varDelta ]^{NP}$ that we defined in proof of Theorem 2 above. Given that the conditional probabilities are those of a standard lottery on the assumption that not all of the propositions in $[\varDelta ]$ are false, we get from (A2) that

(F4) For all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$,

$$\begin{aligned} Pr(\bigwedge \varGamma |\bigwedge \varGamma ')+Pr(\bigwedge \left( \varDelta -(\varGamma \cup \varGamma '\right) |\varGamma ')=1. \end{aligned}$$

The reason for this is that, assuming $\bigwedge \varGamma '$ is true, there is exactly one false proposition in $\varDelta$. It cannot be in both $\varGamma$ and $\varDelta -(\varGamma \cup \varGamma ')$, since they are disjoint, but it must be in one of them since $\varGamma \cup \left( \varDelta -(\varGamma \cup \varGamma ')\right) =\varDelta -\varGamma '$.

Relying on the bijection, f, on $[\varDelta ]^{NP}$ defined in our proof of Theorem 2 above, (F2–F4) , gives us that

(F5) For all $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$ and $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$, $m(\langle \varGamma ,\ \varGamma '\rangle )+m\left( f\left( \langle \varGamma ,\ \varGamma '\rangle \right) \right) \ge 1-2\cdot \dfrac{r}{1-r}$.

These are all of the facts that we need about the degree of confirmation provided by the subset pairs of $[\varDelta ]$ to complete the proof. The final steps in the proof relate to the cardinality of the subset pairs in $[\varDelta ]^{NP}$ in relation to $[\varDelta ]$ and to what happens as r approaches 0 and n approaches $\infty$. So, next notice, given that $\varDelta$ is inconsistent, that for $m\in \{F^{*},\ \lambda ^*,\ z^*,\ d^{*}_{1},\ d^{*}_{2}\}$,

$$\begin{aligned} C_{m}(\varDelta )=\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{NP}}m(\langle \varGamma ,\,\varGamma '\rangle )}{|[\varDelta ]|}-\dfrac{|[\varDelta ]^{P}|}{|[\varDelta ]|} \end{aligned}$$

By the fact that f is a bijection (similar to the Proof of Theorem 2), this means that for $m\in \{F^{*},\ \lambda ^*,\ z^*,\ d^{*}_{1},\ d^{*}_{2}\}$

Thus,

$$\begin{aligned} \underset{r\rightarrow 0}{lim}C_{m}(\varDelta )\ge =\dfrac{|[\varDelta ]^{NP}|-|[\varDelta ]^{P}|}{2\cdot |[\varDelta ]|} \end{aligned}$$

Anagolously, we have it that

$$\begin{aligned} C_{F^{*}}(\varDelta )\ge \dfrac{|[\varDelta ]^{NP}|\cdot \left( \dfrac{\dfrac{1}{n}-\dfrac{r}{1-r}}{\dfrac{1}{n}+\dfrac{r}{1-r}}\right) }{|[\varDelta ]|}-\dfrac{|[\varDelta ]^{P}|}{|[\varDelta ]|} \end{aligned}$$

Since ,

$$\begin{aligned} \underset{r\rightarrow 0}{lim}C_{F^{*}}(\varDelta )\ge \dfrac{|[\varDelta ]^{NP}|-|[\varDelta ]^{P}|}{|[\varDelta ]|}. \end{aligned}$$

Roche (2013) notes that $|[\varDelta ]|=3^{n}-2^{n+1}+1$, and it is easy to verify that $|[\varDelta ]^{P}|=2^{n}-2$ (we can choose each member $\langle \varGamma ,\ \varGamma '\rangle$ of $[\varDelta ]^{P}$ by selecting any non-empty proper subset of $\varDelta$ to be $\varGamma$, and then letting $\varGamma '=\varDelta -\varGamma$). And thus, $|[\varDelta ]^{NP}|=3^{n}-3\cdot 2^{n}+3$. Thus, it follows that

$$\begin{aligned} \dfrac{|[\varDelta ]^{NP}|-|[\varDelta ]^{P}|}{|[\varDelta ]|}=\dfrac{3^{n}-2^{n+2}+3}{3^{n}-2^{n+1}+1} \end{aligned}$$

Finally, since

$$\begin{aligned} \underset{n\rightarrow \infty }{lim}\dfrac{3^{n}-2^{n+2}+3}{3^{n}-2^{n+1}+1}=\underset{n\rightarrow \infty }{lim}\dfrac{\left( 3^{n}-2^{n+2}\right) }{3^{n}-2^{n+1}}=\underset{n\rightarrow \infty }{lim}\left( 1-\dfrac{2}{\left( \dfrac{3}{2}\right) ^{n}-2}\right) =1 \end{aligned}$$

It follows that for $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$

$$\begin{aligned} \underset{(r,\ n)\rightarrow (0,\ \infty )}{lim}C_{m}(\varDelta )\ge \dfrac{1}{2} \end{aligned}$$

And

$$\begin{aligned} \underset{(r,\ n)\rightarrow (0,\ \infty )}{lim}C_{F^{*}}(\varDelta )\ge 1 \end{aligned}$$

Appendix 6: Proof of Theorem 5

Theorem 5

If $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$ and $\varDelta$ is an inconsistent set of propositions, then .

This theorem follows directly from Lemma 2 and Theorem 2. That is to say, we have it that for all $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$

$$\begin{aligned} C_{m,\, Pr}(\varDelta )\le C_{a,\, Pr}(\varDelta ) \end{aligned}$$

And Theorem 2 says when $\varDelta$ is inconsistent.

Appendix 7: Proof of Theorem 7

Theorem 7

If $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$ and $\varphi _{1}$ and $\varphi _{2}$ are inconsistent, then for any evidential proposition $\psi$, $m(\varphi _{1},\,\psi )+m(\varphi _{2},\,\psi )\le 1$.

From Lemma 1, we have it that if $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$ and $\varphi _{1}$ and $\varphi _{2}$ are inconsistent and $\psi \ne \bot$,

$$\begin{aligned} m(\varphi _{1},\,\psi )+m(\varphi _{2},\,\psi )\le Pr(\varphi _{1}|\psi )+Pr(\varphi _{2}|\psi )\le 1 \end{aligned}$$

The only case that we haven’t covered is when $\psi =\bot$. In that case, $m(\varphi _{1},\,\psi )+m(\varphi _{2},\,\psi )=-2$ by our method for extending the confirmation measures, and thus the theorem is true.

Appendix 8: Proof of Theorem 8

Theorem 8

If $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$ and $\varDelta$ is an evidentially inconsistent set of propositions, then $C_{m,\, Pr}(\varDelta )<0$ for all probability functions Pr.

Let $\varDelta =\{\varphi _{1},\ \varphi _{2},...,\ \varphi _{n}\}$ be an evidentially inconsistent set of propositions where $\varDelta _{w}\subseteq \varDelta$ is a witnessing$_2$ set and $m\in \{\lambda ^{*},\ z^{*},\, d_{1}^{*},\ d_{2}^{*}\}$. Then the following propositions are true by defintion of m:

Proposition 1

For any $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]$, if $|(\varGamma \cup \varGamma ')\cap \varDelta _{w}|>\dfrac{|\varDelta _{w}|}{2}$, then $m(\langle \varGamma ,\ \varGamma '\rangle )=-1$.

Definition 12

Again, we define a bijection on $[\varDelta ]^{NP}$ that will allow us to make use of Lemma 1.

$f:[\varDelta ]^{NP}\rightarrow [\varDelta ]^{NP}$ such that $f(\langle \varGamma ,\,\varGamma '\rangle )=\langle \varDelta -\varGamma \cup \varGamma ',\,\varGamma '\rangle$.

Proposition 2

For any $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$,

$$\begin{aligned} if\, |\varGamma \cap \varDelta _{w}|\ne \dfrac{|\varDelta _{w}|}{2}, then m(\langle \varGamma ,\ \varGamma '\rangle ) + m(f(\langle \varGamma ,\ \varGamma '\rangle ))\le 0. \end{aligned}$$

If the antecent is true, then either $\langle \varGamma ,\,\varGamma '\rangle$ or $f(\langle \varGamma ,\,\varGamma '\rangle )$ contains more than half of $\varDelta _{w}$ (this is easy to verify, so I leave the proof to the interested reader) and by Proposition 1, this means either $m(\langle \varGamma ,\ \varGamma '\rangle )$ or $m(f(\langle \varGamma ,\ \varGamma '\rangle ))$ is $-1$.

Proposition 3

For any $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}$, if $\varGamma '\cap \varDelta _{w}\ne \emptyset$, then $m(\langle \varGamma ,\ \varGamma '\rangle )$ + $m(f(\langle \varGamma ,\ \varGamma '\rangle ))\le 0$.

Again, either $\langle \varGamma ,\,\varGamma '\rangle$ or $f(\langle \varGamma ,\,\varGamma '\rangle )$ contains more than half of $\varDelta _{w}$ if the antecedent is true. From Propositions 1–3, it follows that if $m(\langle \varGamma ,\ \varGamma '\rangle )+m(f(\langle \varGamma ,\ \varGamma '\rangle ))>0$, then $\langle \varGamma ,\ \varGamma '\rangle$ and $f(\langle \varGamma ,\ \varGamma '\rangle )$ are both members of the following set:

Definition 13

$[\varDelta ]^{1}=_{def}\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}:\ |\varGamma \cap \varDelta _{w}|=\dfrac{|\varDelta _{w}|}{2}\ \text{ and } \varGamma '\cap \varDelta _{w}=\emptyset$.

To avoid having to do any combinatorics, we divide $[\varDelta ]^{NP}$ up further. First, we note that the following Proposition holds.

Proposition 4

For any $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]$, if $|\varGamma '\cap \varDelta _{w}|=\dfrac{|\varDelta _{w}|}{2}+1$ and $\varGamma \cap \varDelta _{w}=\emptyset$, then $m(\langle \varGamma ,\ \varGamma '\rangle )$ + $m(f(\langle \varGamma ,\ \varGamma '\rangle ))=-2$.

Thus, there is a corresponding set:

Definition 14

$[\varDelta ]^{2}=_{def}\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{NP}:\ |\varGamma '\cap \varDelta _{w}|=\dfrac{|\varDelta _{w}|}{2}+1\ \text{ and } \varGamma \cap \varDelta _{w}\ne \emptyset \}$.

And, last we define the remainder of $[\varDelta ]^{NP}$ as follows.

Definition 15

$[\varDelta ]^{3}=_{def}[\varDelta ]^{NP}-\left( [\varDelta ]^{1}\cup [\varDelta ]^{2}\right)$

From Proposition 1 above, it follows that

$$\begin{aligned} C_{m}(\varDelta )=\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{NP}}m(\langle \varGamma ,\,\varGamma '\rangle )}{|[\varDelta ]|}-\dfrac{|[\varDelta ]^{P}|}{|[\varDelta ]|} \end{aligned}$$

And thus, by Propositions 1–4,

$$\begin{aligned} C_{m}(\varDelta )<\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{1}}m(\langle \varGamma ,\,\varGamma '\rangle )}{|[\varDelta ]|}-\dfrac{\underset{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{2}}{\sum }2}{|[\varDelta ]|} \end{aligned}$$

Applying Lemma 1 and the fact that f is a bijection on $[\varDelta ]^{1},$ we get that

$$\begin{aligned} C_{m}(\varDelta )<\dfrac{\sum \limits _{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{1}}1}{|[\varDelta ]|}-\dfrac{\underset{\langle \varGamma ,\,\varGamma '\rangle \in [\varDelta ]^{2}}{\sum }2}{|[\varDelta ]|} \end{aligned}$$

And, this simplifies to the claim that

$$\begin{aligned} C_{m}(\varDelta )<\dfrac{|[\varDelta ]^{1}|-2\cdot |[\varDelta ]^{2}|}{|[\varDelta ]|} \end{aligned}$$

Thus, we just need to show that $|[\varDelta ]^{1}|\le 2\cdot |[\varDelta ]^{2}|$. Let $|\varDelta _{w}|=k$. We shall break up the cases where k is even and odd. In the case where k is odd, $[\varDelta ]^{1}=\emptyset$, and the theorem must hold. Thus, let us assume that k is even. It is a straightforward exercise to show that the following biconditional holds (Just treat the construction of subset pairs as a two step process, where we first choose the relevant subsets of $[\varDelta _{w}]$, and then use those subsets to match up with subset pairs from $[\varDelta -\varDelta _{w}]$ or subsets of $\varDelta$).

$$\begin{aligned} \begin{array}{ccc} |[\varDelta ]^{1}|\le 2\cdot |[\varDelta ]^{2}|&\text{ if } \text{ and } \text{ only } \text{ if }&\left. \genfrac(){0.0pt}0{k}{\dfrac{k}{2}}\le \left. 2\cdot \genfrac(){0.0pt}0{k}{\dfrac{k}{2}+1}\right. \right. \end{array} \end{aligned}$$

To complete the proof, we prove the right hand side of the above biconditional. Assuming that k is even, the biconditional is easily simplified as follows:

$$\begin{aligned} \begin{array}{c} \genfrac(){0.0pt}0{k}{\dfrac{k}{2}}\le \left. 2\cdot \genfrac(){0.0pt}0{k}{\dfrac{k}{2}+1}\right. \\ \text{ iff }\\ \dfrac{(k+2)}{k}\le \left. 2\right. \end{array} \end{aligned}$$

And, of course, $\dfrac{(k+2)}{k}\le \left. 2\right.$ for all $k\ge 2$. Given that we have already handled the cases where k is odd and hence where $k=1$, we have thus shown that

$$\begin{aligned} C_{m}(\varDelta )<\dfrac{|[\varDelta ]^{1}|-2\cdot |[\varDelta ]^{2}|}{|[\varDelta ]|}\le 0 \end{aligned}$$

Appendix 9: Proof of Theorem 9

Theorem 9

$m\in \{F^{*},\ r_{1}^{*},\ r_{2}^{*}\}$. Then there exists a set of propositions $\varDelta$ and a probability function Pr s.t. $C_{m,\, Pr}(\varDelta )>0$.

The cases where $m\in \{r_{1}^{*},\ r_{2}^{*}\}$ are already covered by our proof of Theorem 3. The probability space we considered included a contradictory pair of propositions, and thus a logically mostly false set of propositions. Thus, we just need to consider the case where $m=F^{*}$.

Let the probabilities on $h_{1}$, $h_{2}$ and e be like those given in Figure 1 (though we shall vary the value of x and y). Any set of propositions containing all three of these propositions will be evidentially inconsistent. But, now, let us consider the set of propositions: $\{e_{1},\ e_{2},\ e_{3}\}$ s.t. for all $1\le i\le 3$, $e_{i}$ is logically equivalent to e. Let us consider the coherence value on $\mathcal {C}_{F^{*}}$ that the set $\varDelta =\{e,\ e_{1},\ e_{2},\ e_{3},\ h_{1},\ h_{2}\}$ converges to when x approaches 0 and y approaches 1. First, because of the nature of logical relations between the members of the set $\varDelta$, any subset pair, $\langle \varGamma ,\ \varGamma '\rangle$, falls into one of four cases:

$\begin{array}{cclcl} (i) &{} \ &{} \{h_{1},\ h_{2}\}\subseteq \varGamma \cup \varGamma ' &{} \ &{} F^{*}(\varGamma ,\ \varGamma ')=-1\\ (ii) &{} &{} \{h_{1},\ h_{2}\}\not \subseteq \varGamma \cup \varGamma \text{ and } (h_{1}\in \varGamma '\ \text{ or } h_{2}\in \varGamma ') &{} &{} F^{*}(\varGamma ,\ \varGamma ')=1\\ (iii) &{} &{} \{h_{1},\ h_{2}\}\not \subseteq \varGamma \cup \varGamma \text{ and } (h_{1}\in \varGamma \ \text{ or } h_{2}\in \varGamma ) &{} &{} F^{*}(\varGamma ,\ \varGamma ')\thickapprox 1\\ (iv) &{} &{} \{h_{1},\ h_{2}\}\cap (\varGamma \cup \varGamma ')=\emptyset &{} &{} F^{*}(\varGamma ,\ \varGamma ')=1 \end{array}$

As the above shows, the only case that varies is (iii) depending on the values of x and y. As x approaches 0 and y approaches 1, the pairs that fall into case (iii) are a assigned a value by $F^{*}$ that converges on 1. So, then estimating $C_{F^{*}}(\varDelta )$ is simply a matter of adding up all of the subset pairs falling into cases $(ii)-(iv)$ and substracting the number of subset pairs that fall into case (i) and then dividing by $|[\varDelta ]|$. To simplify the calculations, we can define:

Definition 16

$[\varDelta ]^{(i)}=\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]: \{h_{1},\ h_{2}\}\subseteq \varGamma \cup \varGamma '\}$

Then the estimate comes to the following

$$\begin{aligned} C_{F^{*}}(\varDelta )\thickapprox \dfrac{|[\varDelta ]|-2\cdot |[\varDelta ]^{(i)}|}{|[\varDelta ]|} \end{aligned}$$

Roche (2013) observes that if we let n be the cardinality of $\varDelta$, then $|[\varDelta ]|=3^{n}-2^{n+1}+1$. So, we have it that

$$\begin{aligned} C_{F^{*}}(\varDelta )\thickapprox \dfrac{3^{n}-2^{n+1}+1-2\cdot |[\varDelta ]^{(i)}|}{3^{n}+2^{n+1}-1} \end{aligned}$$

Thus, we just need to calculate $|[\varDelta ]^{(i)}|$. To simplify our calculation further, note that we can divide $[\varDelta ]^{(i)}$ into four distinct sets

Definition 17

$[\varDelta ]_{1}^{(i)}=\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{(i)}:\ \{h_{1},\ h_{2}\}\subseteq \varGamma \}$

Definition 18

$[\varDelta ]_{2}^{(i)}=\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{(i)}:\ \{h_{1},\ h_{2}\}\subseteq \varGamma '\}$

Definition 19

$[\varDelta ]_{3}^{(i)}=\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{(i)}:\ h_{1}\in \varGamma ' \text{ and } h_{2}\in \varGamma \}$

Definition 20

$[\varDelta ]_{4}^{(i)}=\{\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]^{(i)}:\ h_{1}\in \varGamma \text{ and } h_{2}\in \varGamma '\}$

The next step is to calculate $|\varDelta ]_{1}^{(i)}|$. A subset pair $\langle \varGamma ,\ \varGamma '\rangle \in [\varDelta ]_{1}^{(i)}$ if $\varGamma '$ is a non-empty subset of $\{e,\ e_{1},\ e_{2},\ e_{3}\}$ and $\varGamma =\{h_{1},\ h_{2}\}$, or else $\langle \varGamma -\{h_{1},\ h_{2}\},\ \varGamma '\rangle \in [\{e,\ e_{1},\ e_{2},\ e_{3}\}]$. Thus,

$$\begin{aligned} |[\varDelta ]_{1}^{(i)}|=(3^{n-2}-2^{n-1}+1)+(2^{n-2}-1) \end{aligned}$$

And symmetry considerations give us that

$$\begin{aligned} |[\varDelta ]_{1}^{(i)}|=|[\varDelta ]_{2}^{(i)}|=3^{n-2}-2^{n-2} \end{aligned}$$

Calculating $[\varDelta ]_{3}^{(i)}$ and $[\varDelta ]_{4}^{(i)}$ is even more straightforward. There are $3^{n-2}$ empty or non-empty subset pairs of $\{e,\ e_{1},\ e_{2},\ e_{3}\}$ that can be combined with $\langle \{h_{2}\},\ \{h_{1}\}\rangle$ to form subset pairs in $[\varDelta ]_{3}^{(i)}$ . So, again by symmetry considerations we get that

$$\begin{aligned} |[\varDelta ]_{3}^{(i)}|=|[\varDelta ]_{4}^{(i)}|=3^{n-2} \end{aligned}$$

Putting this altogether, we get that