Jointing Adaptive Modulation Relay Selection Protocols for Two-Way Opportunistic Relaying Systems with Amplify-and-Forward Policy

Abstract

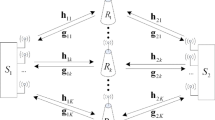
Two novel best-relay selection protocols, the jointing adaptive modulation max–min (AM-MM) protocol and the jointing adaptive modulation maximum harmonic mean (AM-MHM) protocol, are proposed for two-way opportunistic relaying systems with amplify-and-forward policy (TWOR-AF). By integrating the adaptive modulation with the conventional max–min (NonAM-MM) and maximum harmonic mean protocols, the effect of the modulation schemes used at both sources is exploited perfectly in the proposed AM-MM and AM-MHM protocols. The analytical expressions to the approximate upper bounds of overall average symbol error probability (SEP) for TWOR-AF systems with these different relay selection protocols are obtained through theoretic analysis. The numerical results demonstrate that the average SEP of TWOR-AF systems can be improved greatly when the proposed jointing adaptive modulation relay selection protocols are performed. Furthermore, in certain channel realizations, the adaptive modulation non-selection protocol outperforms the conventional NonAM-MM protocol in which the adaptive modulation is not integrated.

Appendix

1.1 Appendix 1

Proof of Theorem 1

From expression (4), $\gamma _{1k} =\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}$, $\gamma _{2k} =\frac{\rho ^{2}H_{1k} H_{2k} }{\rho H_{1k} +2\rho H_{2k} +1}$, where $H_{1k} =\left| {h_{1k} } \right|^{2}$ and $H_{2k} =\left| {h_{2k} } \right|^{2}$ are exponentially distributed variables with distributed parameters $\lambda _k$ and $\mu _k$ respectively, we get $H_{1k} \ge 0$,$H_{2k} \ge 0$,$\rho \ge 0$, and $\gamma _{1k} \ge 0$, $\gamma _{2k} \ge 0$. To analyze the PDF of $\gamma _{1k}$ and $\gamma _{2k}$, we should get the CDF of $\gamma _{1k}$ and $\gamma _{2k}$ at first. For $\gamma >0$, we have

$$\begin{aligned} \begin{aligned} F_{\gamma _{1k} } (\gamma )&=P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}\le \gamma \right) \\&=1-P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}>\gamma \right) \\ \end{aligned} \end{aligned}$$

(36)

By dividing $P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}>\gamma \right)$ into three cases, we can get

$$\begin{aligned} \begin{array}{l} P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}>\gamma \right) \\ \quad =P(H_{2k} >\frac{2\gamma }{\rho },H_{1k} >\frac{\rho H_{2k} \gamma +\gamma }{\rho ^{2}H_{2k} -2\rho \gamma })+P\left(H_{2k} <\frac{2\gamma }{\rho },H_{1k} <\frac{\rho H_{2k} \gamma +\gamma }{\rho ^{2}H_{2k} -2\rho \gamma }\right) \\ \quad +P\left(H_{2k} =\frac{2\gamma }{\rho },\frac{2\rho \gamma H_{1k} }{2\rho H_{1k} +2\gamma +1}>\gamma \right) \\ \end{array} \end{aligned}$$

(37)

When $H_{2k} <\frac{2\gamma }{\rho }$, we can get $\frac{\rho H_{2k} \gamma +\gamma }{\rho ^{2}H_{2k} -2\rho \gamma }<0$. However we know that $H_{1k} \ge 0$ from analysis mentioned before, so $P(H_{2k} <\frac{2\gamma }{\rho },H_{1k} <\frac{\rho H_{2k} \gamma +\gamma }{\rho ^{2}H_{2k} -2\rho \gamma })=0$. We can also obtain that $P(H_{2k} =\frac{2\gamma }{\rho },\frac{2\rho \gamma H_{1k} }{2\rho H_{1k} +2\gamma +1}>\gamma )=0$ due to $\rho \ge 0$,$H_{1k} \ge 0$,$\gamma >0$. Thus we can get

$$\begin{aligned}&P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}>\gamma \right) \nonumber \\&\quad =P\left(H_{2k} >\frac{2\gamma }{\rho },H_{1k} >\frac{\rho H_{2k} \gamma +\gamma }{\rho ^{2}H_{2k} -2\rho \gamma }\right) \nonumber \\&\quad =\int \limits _{\frac{2\gamma }{\rho }}^\infty {\int \limits _{\frac{\rho y\gamma +\gamma }{\rho ^{2}y-2\rho \gamma }}^\infty {\lambda _k } } e^{-\lambda _k x}\mu _k e^{-\mu _k y}dxdy \nonumber \\&\quad =\mu _k \int \limits _{\frac{2\gamma }{\rho }}^\infty {e^{-\lambda _k \frac{\rho y\gamma +\gamma }{\rho ^{2}y-2\rho \gamma }}} e^{-\mu _k y}dy \nonumber \\&\quad =\frac{\mu _k }{\rho }e^{-\frac{\lambda _k +2\mu _k }{\rho }\gamma }\int \limits _0^\infty {\exp \left(-\frac{\lambda _k \gamma }{\rho }\cdot \frac{2\gamma +1}{t}-\frac{\mu _k t}{\rho }\right)} dt \end{aligned}$$

(38)

Using the Equation (3.471.9) in [23], the integral in (38) can be obtained easily, leading to

$$\begin{aligned}&P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}>\gamma \right) \nonumber \\&\quad =\frac{\mu _k }{\rho }e^{-\frac{\lambda _k +2\mu _k }{\rho }\gamma }\cdot 2\sqrt{\frac{\lambda _k \gamma (2\gamma +1)}{\mu _k }}K_1 \left(2\sqrt{\frac{\lambda _k \mu _k \gamma (2\gamma +1)}{\rho ^{2}}}\right) \end{aligned}$$

(39)

where $K_1 (\cdot )$ is the first order modified Bessel function of the second kind defined by (8.432.6) in [23]. As stated in [18], in high SNR, the modified Bessel function can be approximated as $K_1 (z)\approx 1/z$. Thus, with high SNR approximation we have

$$\begin{aligned} P\left(\frac{\rho ^{2}H_{1k} H_{2k} }{2\rho H_{1k} +\rho H_{2k} +1}>\gamma \right)\approx e^{-\frac{\lambda _k +2\mu _k }{\rho }\gamma } \end{aligned}$$

(40)

For $\gamma >0$, combining (40) and (36), we have

$$\begin{aligned} F_{\gamma _{1k} } (\gamma )\approx 1-e^{-\frac{\lambda _k +2\mu _k }{\rho }\gamma } \end{aligned}$$

(41)

Taking the derivative of $F_{\gamma _{1k} } (\gamma )$ with respect to $\gamma$, we can get the approximately expression for the PDF of $\gamma _{1k}$ at high SNR,

$$\begin{aligned} f_{\gamma _{1k} } (\gamma )\approx \left\{ {\begin{array}{ll} \frac{\lambda _k +2\mu _k }{\rho }e^{-\frac{\lambda _k +2\mu _k }{\rho }\gamma },&\quad for\;\gamma >0 \\ 0,&\quad for\;\gamma \le 0 \\ \end{array}} \right. \end{aligned}$$

(42)

Similar to the analysis of $f_{\gamma _{1k} } (\gamma )$, we can obtain the approximately expression for the PDF of $\gamma _{2k}$ at high SNR, that is

$$\begin{aligned} f_{\gamma _{2k} } (\gamma )\approx \left\{ {\begin{array}{ll} \frac{2\lambda _k +\mu _k }{\rho }e^{-\frac{2\lambda _k +\mu _k }{\rho }\gamma },&\quad for\;\gamma >0 \\ 0,&\quad for\;\gamma \le 0 \\ \end{array}} \right. \end{aligned}$$

(43)

$\square$

1.2 Appendix 2

Proof of Theorem 3

Using the definition $w_k =\min (w_{1k} ,w_{2k} )$, the CDF of the $w_k$ can be given by

$$\begin{aligned} F_{w_k } (w)=P(w_k \le w)=1-P(w_{1k} >w,w_{2k} >w) \end{aligned}$$

(44)

Like analysis of expression (37), we can obtain

$$\begin{aligned} \begin{array}{l} P(w_{1k} >w,w_{2k} >w) =P\left(\left(H_{1k} >\frac{w}{\rho {}\Delta M_2 },H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right)\right.\\ \quad \left.\cap \left(H_{1k} >\frac{2w}{\rho {}\Delta M_1 },H_{2k} >\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right)\right) \\ \end{array} \end{aligned}$$

(45)

where $A\cap B$ means intersection of set $A$ and set $B$. According to the solving process of equations $\frac{w}{\rho {}\Delta M_2 }=\frac{2w}{\rho {}\Delta M_1 }$and $\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}=\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}$, expression (45) can be divided into three cases.

• Case 1: for $\Delta M_1 \le \frac{1}{2}\Delta M_2$ When $\Delta M_1 \le \frac{1}{2}\Delta M_2$, we can get $\frac{2w}{\rho {}\Delta M_1 }>\frac{w}{\rho {}\Delta M_2 }$and $\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}>\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}$, so

  $$\begin{aligned} P(w_{1k} >w,w_{2k} >w)=P\left(H_{1k} >\frac{2w}{\rho {}\Delta M_1 },H_{2k} >\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right) \end{aligned}$$

  (46)

• Case 2: for $\frac{1}{2}\Delta M_2 <\Delta M_1 <\Delta M_2 +3w$ This case can be further divided into two situations: Situation 2(a), when $w\le \frac{\Delta M_2 }{3}$, $\frac{1}{2}\Delta M_2 <\Delta M_1 <\Delta M_2 +3w$ or when $w>\frac{\Delta M_2 }{3}$, $\frac{1}{2}\Delta M_2 <\Delta M_1 <2\Delta M_2$, we have

  $$\begin{aligned} \begin{array}{l} P(w_{1k} >w,w_{2k} >w)=P\left(\frac{2w}{\rho {}\Delta M_1 }<H_{1k} <N(w),H_{2k} >\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right) \\ \quad +P\left(H_{1k} \ge N(w),H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right) \\ \end{array} \end{aligned}$$

  (47)

  where $N(w)=\frac{\Delta M_2 -\Delta M_1 +3w+\sqrt{(\Delta M_2 -\Delta M_1 +3w)^{2}-4w(\Delta M_2 -2\Delta M_1 )}}{2\rho (2\Delta M_1 -\Delta M_2 )}$ is the positive solution of equation $\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}=\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}$ with respect to $H_{1k}$. Situation 2(b), when $w>\frac{\Delta M_2 }{3}$,$2\Delta M_2 \le \Delta M_1 <\Delta M_2 +3w$, we have

  $$\begin{aligned} P(w_{1k} >w,w_{2k} >w)=P\left(H_{1k} >\frac{w}{\rho {}\Delta M_2 },H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right) \end{aligned}$$

  (48)

• Case 3: for $\Delta M_1 \ge \Delta M_2 +3w$

This case can also be further divided into two situations:

Situation 3(a), when$w\le \frac{\Delta M_2 }{3}$,$\Delta M_2 +3w\le \Delta M_1 <2\Delta M_2$, we have

$$\begin{aligned} \begin{array}{l} P(w_{1k} >w,w_{2k} >w)=P\left(\frac{2w}{\rho {}\Delta M_1 }<H_{1k} <N(w),H_{2k} >\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right) \\ \quad +P\left(H_{1k} \ge N(w),H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right) \\ \end{array} \end{aligned}$$

(49)

Situation 3(b), when$w>\frac{\Delta M_2 }{3}$,$\Delta M_1 \ge \Delta M_2 +3w$ or when $w\le \frac{\Delta M_2 }{3}$,$\Delta M_1 \ge 2\Delta M_2$, we have

$$\begin{aligned} P(w_{1k} >w,w_{2k} >w)=P\left(H_{1k} >\frac{w}{\rho {}\Delta M_2 },H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right) \end{aligned}$$

(50)

Note that expressions (47) and (49) are the same, expressions (48) and (50) are the same, so we can incorporate situation 2(a) with situation 3(a), while incorporating situation 2(b) with situation 3(b),

$$\begin{aligned} \begin{array}{l} P(w_{1k} >w,w_{2k} >w)= \\ \left\{ {\begin{array}{ll} P\left(H_{1k} >\frac{2w}{\rho {}\Delta M_1 },H_{2k} > \frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right),&\quad for \Delta M_1 \le \frac{1}{2}\Delta M_2 \\ P\left(\frac{2w}{\rho {}\Delta M_1 }<H_{1k} <N(w),H_{2k} > \frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right) \\ \quad +\,P\left(H_{1k} \ge N(w),H_{2k} >\frac{2w\rho H_{1k} +w}{ \rho ^{2}H_{1k} \Delta M_2 -\rho w}\right),&\quad for\frac{1}{2}\Delta M_2 <\Delta M_1 <2\Delta M_2 \\ P\left(H_{1k} >\frac{w}{\rho {}\Delta M_2 },H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right),&\quad for\Delta M_1 \ge 2\Delta M_2 \\ \end{array}} \right. \\ \end{array} \end{aligned}$$

(51)

Using the similar analysis method as Appendix 1, we have the approximately expression of $F_{w_k } (w)$ at high SNR for $\Delta M_1 \le \frac{1}{2}\Delta M_2$ and $\Delta M_1 \ge 2\Delta M_2$,

$$\begin{aligned} F_{w_k } (w)&= 1-P(w_{1k} >w,w_{2k} >w)\approx 1-e^{-\frac{(\mu _k +2\lambda _k )w}{\rho \Delta M_1 }},\Delta M_1 \le \frac{1}{2}\Delta M_2\end{aligned}$$

(52)

$$\begin{aligned}&F_{w_k } (w)\approx 1-e^{-\frac{(\lambda _k +2\mu _k )w}{\rho \Delta M_2 }},\;\Delta M_1 \ge 2\Delta M_2 \end{aligned}$$

(53)

For $\frac{1}{2}\Delta M_2 <\Delta M_1 <2\Delta M_2$, we get

$$\begin{aligned}&P\left(\frac{2w}{\rho {}\Delta M_1 }<H_{1k} <N(w),H_{2k} >\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right) \nonumber \\&\quad +P\left(H_{1k} \ge N(w),H_{2k} >\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right) \nonumber \\&\quad =P\left(H_{1k} >\frac{2w}{\rho {}\Delta M_1 },H_{2k} >\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}\right) \nonumber \\&\quad -P\left(H_{1k} \ge N(w),\frac{w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_1 -2\rho w}<H_{2k} <\frac{2w\rho H_{1k} +w}{\rho ^{2}H_{1k} \Delta M_2 -\rho w}\right) \nonumber \\&\quad =e^{-\frac{(\mu _k +2\lambda _k )w}{\rho \Delta M_1 }}-\int \limits _{N(w)}^\infty \lambda _k e^{-\lambda _k x}\left(e^{-\mu _k \frac{w\rho x+w}{\rho ^{2}x\Delta M_1 -2\rho w}}- e^{-\mu _k \frac{2w\rho x+w}{\rho ^{2}x\Delta M_2 -\rho w}}\right)dx \nonumber \\&\quad =e^{-\frac{(\mu _k +2\lambda _k )w}{\rho \Delta M_1 }}+\int \limits _0^{N(w)} {\lambda _k e^{-\lambda _k x}e^{-\mu _k \frac{w\rho x+w}{\rho ^{2}x\Delta M_1 -2\rho w}}} dx-\int \limits _0^{N(w)} {\lambda _k e^{-\lambda _k x}e^{-\mu _k \frac{2w\rho x+w}{\rho ^{2}x\Delta M_2 -\rho w}}dx} \nonumber \\ \end{aligned}$$

(54)

The second term on the right-hand side of the last Equation in (54) can be written as

$$\begin{aligned}&\int \limits _0^{N(w)} {\lambda _k e^{-\lambda _k x}e^{-\mu _k \frac{w\rho x+w}{\rho ^{2}x\Delta M_1 -2\rho w}}} dx \nonumber \\&\quad =\frac{\lambda _k }{\rho \Delta M_1 }e^{-\frac{2\lambda _k +\mu _k }{\rho \Delta M_1 }w}\int \limits _{-2w}^{\rho N(w)\Delta M_1 -2w} {e^{-\frac{\mu _k w}{\rho \Delta M_1 }\cdot \frac{2w+\Delta M_1 }{t}}e^{-\frac{\lambda _k t}{\rho \Delta M_1 }}} dt \nonumber \\&\quad \approx \frac{\lambda _k }{\rho \Delta M_1 }e^{-\frac{2\lambda _k +\mu _k }{\rho \Delta M_1 }w}\cdot \rho N(w)\Delta M_1 e^{-2\sqrt{\frac{\lambda _k \mu _k w(2w+\Delta M_1 )}{\rho ^{2}\Delta M_1^2 }}} \nonumber \\&\quad \approx \lambda _k N(w) e^{-\frac{w}{\rho \Delta M_1 }\left(\sqrt{\mu { }_k}+\sqrt{2\lambda _k }\right)^{2}} \end{aligned}$$

(55)

The first approximation in (55) uses $x+y\approx 2\sqrt{xy}$, the second approximation is ignoring $\Delta M_1$ due to high SNR. Using the same analytical method as (55), we have the third term on the right-hand side of the last Equation in (54),

$$\begin{aligned} \int \limits _0^{N(w)} {\lambda _k e^{-\lambda _k x}e^{-\mu _k \frac{2w\rho x+w}{\rho ^{2}x\Delta M_2 -\rho w}}dx} \approx \lambda _k N(w) e^{-\frac{w}{\rho \Delta M_2 }\left(\sqrt{2\mu { }_k}+\sqrt{\lambda _k }\right)^{2}} \end{aligned}$$

(56)

Substituting (55, 56) into (54), we get

$$\begin{aligned}&F_{w_k } (w)\approx 1-e^{-\frac{(\mu _k +2\lambda _k )w}{\rho \Delta M_1 }}-\lambda _k N(w) e^{-\frac{w}{\rho \Delta M_1 }\left(\sqrt{\mu { }_k}+\sqrt{2\lambda _k }\right)^{2}} \nonumber \\&\quad +\lambda _k N(w) e^{-\frac{w}{\rho \Delta M_2 }\left(\sqrt{2\mu { }_k}+\sqrt{\lambda _k }\right)^{2}},\;\frac{1}{2}\Delta M_2 <\Delta M_1 <2\Delta M_2 \end{aligned}$$

(57)

Combining (52, 53) and (57), we get Theorem 3.$\square$

1.3 Appendix 3

The CDF of $z_k$ can be shown as

$$\begin{aligned} F_{z_k } (w)=P(z_k \le w)=1-P(z_k >w) \end{aligned}$$

(58)

According to the definition of $z_k$ in expression (25) and using the similar analytical method as expression (37), we can divide $P(z_k >w)$ into three parts, and only one part is not equal to zero.

$$\begin{aligned} P(z_k >w)&= P\left(H_{2k} >\frac{\Delta M_2 w+2\Delta M_1 w}{\rho \Delta M_1 \Delta M_2 }, \right. \nonumber \\&\left. H_{1k} >\frac{(2\rho \Delta M_2 +\rho \Delta M_1 )H_{2k} w+\Delta M_2 w+\Delta M_1 w}{\rho ^{2}H_{2k} \Delta M_1 \Delta M_2 -\rho \Delta M_2 w-2\rho \Delta M_1 w}\right) \end{aligned}$$

(59)

Due to $H_{1k}$ and $H_{2k}$ are exponentially distributed variables with distributed parameters $\lambda _k$ and $\mu _k$ respectively,

$$\begin{aligned} P(z_k >w)&= \int \limits _{\frac{\Delta M_2 w+2\Delta M_1 w}{\rho \Delta M_1 \Delta M_2 }}^\infty {\mu _k e^{-\mu _k y}e^{-\lambda _k \frac{(2\rho \Delta M_2 +\rho \Delta M_1 )yw+\Delta M_2 w+\Delta M_1 w}{\rho ^{2}y\Delta M_1 \Delta M_2 -\rho \Delta M_2 w-2\rho \Delta M_1 w}}} dy \nonumber \\&= \frac{\mu _k e^{-\lambda _k \frac{(2\Delta M_2 +\Delta M_1 )w}{\rho \Delta M_1 \Delta M_2 }}e^{-\mu _k \frac{(\Delta M_2 +2\Delta M_1 )w}{\rho \Delta M_1 \Delta M_2 }}}{\rho \Delta M_1 \Delta M_2 (2\Delta M_2 +\Delta M_1 )w}\int \limits _0^\infty {e^{-\frac{\beta }{t}-\alpha t}} dt \end{aligned}$$

(60)

where$\beta =\lambda _k \frac{(2\Delta M_2 +\Delta M_1 )w}{\rho \Delta M_1 \Delta M_2 }[\Delta M_1 \Delta M_2 (\Delta M_1 +\Delta M_2 )w+(\Delta M_2 +2\Delta M_1 )(2\Delta M_2 +\Delta M_1 )w^{2}]$, $\alpha =\frac{\mu _k }{\rho \Delta M_1 \Delta M_2 (2\Delta M_2 +\Delta M_1 )w}$. Using the equation (3.471.9) in [23] and the modified Bessel function approximation $K_1 (z)\approx 1/z$ for high SNR [18], we have

$$\begin{aligned} P(z_k >w)\approx e^{-\frac{\lambda _k (2\Delta M_2 +\Delta M_1 )+\mu _k (\Delta M_2 +2\Delta M_1 )}{\rho \Delta M_1 \Delta M_2 }w} \end{aligned}$$

(61)

Combining (58) and (61), the CDF of $z_k$ can be approximately written as

$$\begin{aligned} F_{z_k } (w)=1-P(z_k >w)\approx 1-e^{-\frac{\lambda _k (2\Delta M_2 +\Delta M_1 )+\mu _k (\Delta M_2 +2\Delta M_1 )}{\rho \Delta M_1 \Delta M_2 }w},\quad w>0 \end{aligned}$$

(62)

Taking the derivative of $F_{z_k } (w)$ with respect to $w$, we can get the approximately expression for the PDF of $z_k$ at high SNR,

$$\begin{aligned} f_{z_k } (w)&\approx \frac{\lambda _k (2\Delta M_2 +\Delta M_1 )+\mu _k (\Delta M_2 +2\Delta M_1 )}{\rho \Delta M_1 \Delta M_2 } \nonumber \\&\times e^{-\frac{\lambda _k (2\Delta M_2 +\Delta M_1 )+\mu _k (\Delta M_2 +2\Delta M_1 )}{\rho \Delta M_1 \Delta M_2 }w},\quad w>0 \end{aligned}$$

(63)