Abstract
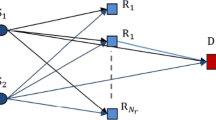
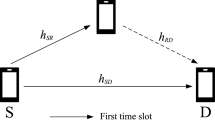
In this paper, we investigate the capacity gain obtained by putting a multiple-antenna relay in an original single-hop multiple-input multiple-output system using an orthogonal space-time block code over uncorrelated Rayleigh fading channels. As relaying techniques, we consider decode-and-forward relaying as well as decouple-and-forward relaying in which no decoding is performed at the relay. Focusing on the low signal-to-noise ratio regime, closed-form expressions of the probability of the capacity gain are provided for the respective relaying schemes. The probability results in an explicit function of an average power ratio of the per-hop channel in dual-hop relaying to the single-hop channel. Numerical examples show the impact of the power ratio, the relaying strategy, the number of antennas and the relay location on the capacity gain, respectively.




Similar content being viewed by others
Notes
It is noted that since the outage performance in this paper is expressed as a function of received SNR, we do not need to explicitly decode the MIMO signal in the simulation but just compute the SNR with a Monte Carlo method. Consequently, we do not use a specific channel estimation method but assume perfect knowledge of channel information at the respective receivers as described in Sect. 2.1.
References
Lee, I.-H., & Kim, D. (2008). Probability of SNR gain by dual-hop relaying over single-hop transmission in SISO Rayleigh fading channels. IEEE Communications Letters, 12(10), 734–736.
Tarokh, V., Jafarkhani, H., & Calderbank, A. R. (1999). Space-time block codes from orthogonal designs. IEEE Transactions on Information Theory, 45, 1456–1467.
Lee, I.-H., & Kim, D. (2008). End-to-end BER analysis for dual-hop OSTBC transmissions over Rayleigh fading channels. IEEE Transactions on Communications, 56(3), 347–351.
Chen, S., Wang, W., Zhang, X., & Sun, Z. (2010). Performance analysis of OSTBC transmission in amplify-and-forward cooperative relay networks. IEEE Transactions on Vehicular Technology, 59(1), 105–113.
Chalise, B. K., & Vandendorpe, L. (2008). Outage probability analysis of a MIMO relay channel with orthogonal space-time block codes. IEEE Communications Letters, 12(4), 280–282.
Yang, L., & Zhang, Q. T. (2010). Performance analysis of MIMO relay wireless networks with orthogonal STBC. IEEE Transactions on Vehicular Technology, 59(7), 3668–3674.
Yang, Q., Kwak, K. S., & Fu, F. (2010). Closed-form symbol error rate expression of decode-and-forward relaying using orthogonalspace-time block coding. IET Communications, 4(3), 368–375.
Yang, S., & Belfiore, J.-C. (2007). Diversity of MIMO multihop relay channels. IEEE Transactions on Information Theory (submitted). http://arxiv.org/pdf/0708.0386.pdf.
Lee, I.-H., & Kim, D. (2010). Achieving maximum spatial diversity with decouple-and-forward relaying in dual-hop OSTBC transmissions. IEEE Transactions on Wireless Communications, 9(3), 921–925.
Lee, I.-H., Park, J.-H., & Kim, D. (2010). Outage performance of multi-hop decouple-and-forward relaying in spatially correlated MIMO channels. IEICE Transactions on Communications, E93-B(5), 1298–1301.
IEEE Standard 802.16e-2005. (2006). IEEE standard for local and metropolitan area networks–Part 16: Air interface for fixed and mobile broadband wireless access systems. http://ieee802.org/16/pubs/80216e.html.
3GPP TS 36.211 v8.5.0. (2008). Evolved universal terrestrial radio access(E-UTRA); physical channels and modulation. http://www.3gpp.org/ftp/Specs/html-info/36211.htm.
IEEE 802.16j-06/015. (2006). Harmonized contribution on 802.16j (mobile multihop relay) usage models. http://www.ieee802.org/16/relay/docs/80216j-06_015.pdf.
3GPP TS 36.216 V10.3.1. (2011). Evolved universal terrestrial radio access (E-UTRA); physical layer for relaying operation. http://www.3gpp.org/ftp/Specs/html-info/36216.htm.
Li, X., Luo, T., Yue, G., & Yin, C. (2001). A squaring method to simplify the decoding of orthogonal space-time block codes. IEEE Transactions on Communications, 49(10), 1700–1703.
Haykin, S., & Moher, M. (2004). Modern wireless communications. New Jersey: Prentice Hall.
Larsson, E. G., & Stoica, P. (2003). Space-time block coding for wireless communications. Cambridge: Cambridge University Press.
Laneman, J. N., Tse, D. N. C., & Wornell, G. W. (2004). Cooperative diversity in wireless networks: Efficient protocols and outage behavior. IEEE Transactions on Information Theory, 50(12), 3062–3080.
Proakis, J. G. (2001). Digital communications (4th ed.). New York: McGraw-Hill.
Louie, R. H. Y., Li, Y., & Vucetic, B. (May 2008). Performance analysis of beamforming in two hop amplify and forward relay networks. In Proceedings of IEEE international conference on communications (ICC 2008), Beijing, China (pp. 4311–4315).
Gradshteyn, I. S., & Ryzhik, I. M. (2007). Table of integrals, series, and products (7th ed.). MA: Academic.
National Research Council. (1997). The evolution of untethered communications. Washington: National Academy Press.
Baum, D. S., El-Sallabi, H., et al. (2005). Final report on link level and system level channel models. WINNER project deliverable D5.4, v. 1.4. http://projects.celtic-initiative.org/winner+.
Itu-R, M. 2135-1. (2009). Guidelines for evaluation of radio interface technologies for IMT-advanced. http://www.itu.int/pub/R-REP-M.2135-1-2009.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A: Derivation of \(\mathcal P ^{DCF}_{A}(\lambda )\)
Inserting (9) and (10) into (7), \(\mathcal P ^{DCF}_{A}(\lambda )\) for \(\lambda > 1/R_c\) is obtained by
Using [21, eq. (6.621.3)], the integral in (18) is solved as follows:
where
and \(\xi =m_0+m_2\). Inserting (19) into (18) and using \(L_1 = G_1/G_0\) and \(L_2 = G_2/G_0\), (18) can be expressed as (11).
Appendix B: Derivation of \(\mathcal P ^{DF}_A(\lambda )\)
Let \(I_i(\gamma ) = \sum ^{m_i-1}_{k=0} \frac{1}{k!} \left( \frac{z_i \gamma }{G_i} \right) ^k e^{-z_i \gamma / G_i}\) for \(i=1,2\), where \(z_1=z^S\) and \(z_2=z^R\). Inserting (9) and (14) into (8), \(\mathcal P ^{DF}_A(\lambda )\) for \(\lambda > 1/R_c\) is then obtained by
Using [21, eq. (3.381.4)], (22) is obtained by
Using \(L_1 = G_1/G_0\) and \(L_2 = G_2/G_0\), (23) can be expressed as (15).
Substituting (9) and (14) into (8), \(\mathcal P ^{DF}_A(\lambda )\) for \(\lambda \le 1/R_c\) is obtained by
Using [21, eq. (3.381.4)], (24) is then obtained by
Using \(L_1 = G_1/G_0\) and \(L_2 = G_2/G_0\), (25) can be expressed as (16).
Rights and permissions
About this article
Cite this article
Lee, IH., Kim, D. Probability Analysis of Capacity Gain by Cooperative Relaying Over Single-Hop OSTBC Transmission in Low SNR Regime. Wireless Pers Commun 75, 293–307 (2014). https://doi.org/10.1007/s11277-013-1363-x
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11277-013-1363-x