Secure Cognitive Relay Network: Joint the Impact of Imperfect Spectrum Sensing and Outdated Feedback

Abstract

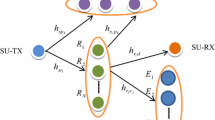
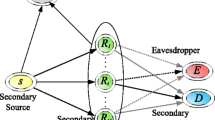
In this paper, we consider cognitive relay networks with Nth best relay and beamforming schemes under the joint impact of imperfect spectrum sensing and outdated channel feedback. Specifically, we examine the secrecy performance by deriving the exact and asymptotic analytical expressions for the secrecy outage probability. Based on the analysis, we observe that these two types of channel impairments, i.e., imperfect spectrum sensing and outdated channel feedback, greatly affect the system performance. Particularly, the outdated channel feedback (i.e., the outdated coefficient $\rho\,<\,1$) reduces the secrecy outage diversity gain of the two schemes, i.e., Nth best relay and beamforming, to one regardless of the number of relays. Our proposed analysis provides a helpful guideline for security system designers to cope with the unpleasant channel impairments.

Appendices

Appendix 1: Proof of Theorem 1

With assumption $K=|{\mathbb {D}}_{{K_c}}|$, based on (2), (12) and (13), $P_{SO1}$ in (20) is expressed as

$$\begin{aligned} P_{SO1}^{N}& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr (\gamma _{{\mathsf {SR}}_k}>\gamma _{th1}) \prod _{m \in {\mathbb {\overline{D}}_{K_c}}}\Pr (\gamma _{{\mathsf {SR}}_m}<\gamma _{th1}) \\&\quad \times\,\Pr \left( \left[ C_{{\mathsf {R}}_{N}{\mathsf {D}}}-C_{{\mathsf {R}}_{N}{\mathsf {E}}} \right] ^{+}<R_{s}|\alpha =0\right) \\&=\phi _{K}(\gamma _{th1}) \underbrace{\Pr \left( \left[ \frac{1+\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}{1 +\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}}\right] ^{+}<\gamma _{th2}\right) }_{P_{SOA}^{N}}. \end{aligned}$$

(48)

According to (30), $\phi _{K}(\gamma _{th1})$ is obtained as follows.

$$\begin{aligned} \phi _{K}(\gamma _{th1})& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr (\gamma _{{\mathsf {SR}}_k}>\gamma _{th1}) \prod _{m \in {\mathbb {\overline{D}}_{K_c}}}\Pr (\gamma _{{\mathsf {SR}}_m}<\gamma _{th1}) \\&=\exp \left( -\frac{K\gamma _{th1}}{\overline{\gamma }_{{\mathsf {SR}}}}\right) \left[ 1-\exp \left( -\frac{\gamma _{th1}}{\overline{\gamma }_{{\mathsf {SR}}}}\right) \right] ^{M-K}. \end{aligned}$$

(49)

$P_{SOA}^{N}=1$ in (48) when $K<N$. Otherwise, $P_{SOA}^{N}$ is derived as

$$\begin{aligned} {P_{SOA}^{N}}& = \Pr \left( \frac{1+\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}{1+\gamma _{{\mathsf {R}}_{N} {\mathsf {E}}}}<\gamma _{th2}, \gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}>\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}\right) \\&\quad +\,\Pr \left( \frac{1+\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}{1+\gamma _{{\mathsf {R}}_{N} {\mathsf {E}}}}<\gamma _{th2}, \gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}<\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}\right) \\&=\sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \left\{ 1- \exp \left[ -\frac{B_{t}(\gamma _{th2}-1)}{A_{t}\overline{\gamma }_{\mathsf {RD}}}\right] \frac{{A_{t}\overline{\gamma }_{\mathsf {RD}}}}{{B_{t}{\overline{\gamma }_{\mathsf {RE}}} \gamma _{th2}}+{A_{t}\overline{\gamma }_{\mathsf {RD}}}} \right\} . \end{aligned}$$

(50)

By substituting (50) into (48), $P_{SO1}^{N}$ is obtained as

$$\begin{aligned} P_{SO1}^{N}&=\phi _{K}(\gamma _{th1}) \left\{ U(N-K) + U(K-N)\sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \right. \\&\quad \times\,\left. \left\{ 1- \exp \left[ -\frac{B_{t}(\gamma _{th2}-1)}{A_{t}\overline{\gamma }_{\mathsf {RD}}}\right] \frac{{A_{t}\overline{\gamma }_{\mathsf {RD}}}}{{B_{t}{\overline{\gamma }_{\mathsf {RE}}} \gamma _{th2}}+{A_{t}\overline{\gamma }_{\mathsf {RD}}}} \right\} \right\} . \end{aligned}$$

(51)

$P_{SO2}$ in (20) is different from $P_{SO1}$ for the reason that the failure of spectrum sensing will introduce the interference from active PUs to the secondary network, i.e., $\alpha =1$, so $P_{SO2}$ is expressed as

$$\begin{aligned} P_{SO2}^{N}& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_k}}{1 +\gamma _{{\mathsf {PR}}_{k}}}>\gamma _{th1}\right) \prod _{m \in {\mathbb {\overline{D}}_{K_c}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_m}}{1 +\gamma _{{\mathsf {PR}}_{m}}}<\gamma _{th1}\right) \\&\quad \times\,\underbrace{\Pr \left( \left[ \frac{1+Z_N}{1+Y_N}\right] ^{+}<\gamma _{th2}\right) }_{P_{SOB}^{N}}, \end{aligned}$$

(52)

where $Z_N=\frac{\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}{1+\gamma _{{\mathsf {P}}{\mathsf {D}}}}$, $Y_N=\frac{\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}}{1+\gamma _{{\mathsf {P}}{\mathsf {E}}}}$,

$\Pr \left( \frac{\gamma _{{\mathsf {SR}}_k}}{1+\gamma _{{\mathsf {PR}}_{k}}}>\gamma _{th1}\right)$ in (52) is derived as

$$\begin{aligned}&\Pr \left( \frac{\gamma _{{\mathsf {SR}}_k}}{1+\gamma _{{\mathsf {PR}}_{k}}}>\gamma _{th1}\right) =\exp \left( -\frac{\gamma _{th1}}{\overline{\gamma }_{{\mathsf {SR}}}}\right) \frac{\overline{\gamma }_{{\mathsf {SR}}}}{\gamma _{th1}\overline{\gamma }_{{\mathsf {PR}}} +\overline{\gamma }_{{\mathsf {SR}}}}. \end{aligned}$$

(53)

With the similar method as (53), $\Pr \left( \frac{\gamma _{{\mathsf {SR}}_m}}{1+\gamma _{{\mathsf {PR}}_{m}}}<\gamma _{th1}\right)$ in (52) is derived as

$$\begin{aligned} \Pr \left( \frac{\gamma _{{\mathsf {SR}}_m}}{1+\gamma _{{\mathsf {PR}}_{m}}}<\gamma _{th1}\right)& = 1-\exp \left( -\frac{\gamma _{th1}}{\overline{\gamma }_{{\mathsf {SR}}}}\right) \frac{\overline{\gamma }_{{\mathsf {SR}}}}{\gamma _{th1}\overline{\gamma }_{{\mathsf {PR}}} +\overline{\gamma }_{{\mathsf {SR}}}}. \end{aligned}$$

(54)

When considering ${P_{SOB}^{N}},\,{P_{SOB}^{N}}=1$ for $K<N$, otherwise, ${P_{SOB}^{N}}$ is derived as

$$\begin{aligned} {P_{SOB}^{N}} = \int _{0}^{\infty } F_{{Z_N}}\left( {\gamma _{th2}(1+y)-1}\right)\,f_{{Y_N}}\left( {y}\right) dy. \end{aligned}$$

(55)

With the aid of (29), $F_{{Z_N}}\left( {z}\right)$ and $F_{{Y_N}}\left( {y}\right)$ are derived as follows

$$\begin{aligned} F_{{Z_N}}\left( {z}\right)& = \int _{0}^{\infty }F_{{\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}}\left( {(1+x)z}\right)\,f_{{\gamma _{{\mathsf {P}}{\mathsf {D}}}}}\left( {x}\right) dx \\& = \sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \left[ 1- \frac{\exp \left( -\xi _{2a} z\right) }{z \xi _{2a} \overline{\gamma }_{{\mathsf {P}}{\mathsf {D}}}+1} \right] , \end{aligned}$$

(56)

$$\begin{aligned} F_{{Y_N}}\left( {y}\right)& = \int _{0}^{\infty }F_{{\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}}}\left( {(1+x)y}\right)\,f_{{\gamma _{{\mathsf {P}}{\mathsf {E}}}}}\left( {x}\right) dy \\& = 1- \exp \left( -\frac{y}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \frac{1}{\xi _{2b} y+1}. \end{aligned}$$

(57)

By differentiating (57), the $f_{{Y_N}}\left( {y}\right)$ is obtained as

$$\begin{aligned}&f_{{Y_N}}\left( {y}\right) = \exp \left( -\frac{y}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \left( \frac{1}{{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}(\xi _{2b} y+1)} +\frac{\xi _{2b}}{(\xi _{2b} y+1)^{2}} \right) . \end{aligned}$$

(58)

By substituting (56) and (58) into (55), ${P_{SOB}^{N}}$ is derived as

$$\begin{aligned}{P_{SOB}^{N}} &= \int _{0}^{\infty } \sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \left[ 1-\frac{\exp \left( -\xi _{2a}(\gamma _{th2}(1+y)-1)\right) }{(\gamma _{th2}(1+y)-1) \xi _{2a}\overline{\gamma }_{{\mathsf {P}}{\mathsf {D}}}+1} \right] \\&\quad \times\,\left( \frac{1}{{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}(\xi _{2b} y+1)} +\frac{\xi _{2b}}{(\xi _{2b} y+1)^{2}} \right) \exp \left( -\frac{y}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) dy \\ &= \sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \left\{ 1- \exp \left[ -\xi _{2a}(\gamma _{th2}-1)\right] \frac{\xi _{2c}}{\xi _{2a}\gamma _{th2}\overline{\gamma }_{{\mathsf {P}}{\mathsf {D}}}} \right. \\&\quad \times\,\left\{ \frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \varPhi _{2}\left( 1,1,1;2;\xi _{2b},\xi _{2c},\xi _{2a}\gamma _{th2} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}\right) \right. \\&\quad +\,\left. \left. \xi _{2b} \varPhi _{2}\left( 1,2,1;2;\xi _{2b},\xi _{2c},\xi _{2a}\gamma _{th2}+ \frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}\right) \right\} \right\} . \end{aligned}$$

(59)

By substituting (53), (54) and (59) into (52), $P_{SO2}^{N}$ is obtained as

$$\begin{aligned}P_{SO2}^{N} &= \eta _{K}(\gamma _{th1}) \left\{ U(N-K) + U(K-N) \right. \\&\quad \times\,\sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \left\{ 1-\exp \left[ -\xi _{2a}(\gamma _{th2}-1)\right] \frac{\xi _{2c}}{\xi _{2a}\gamma _{th2}\overline{\gamma }_{{\mathsf {P}}{\mathsf {D}}}} \right. \\&\quad \times\,\left\{ \frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \varPhi _{2}\left( 1,1,1;2;\xi _{2b},\xi _{2c},\xi _{2a}\gamma _{th2} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}\right) \right. \\&\quad +\,\left. \left. \left. \xi _{2b} \varPhi _{2}\left( 1,2,1;2;\xi _{2b},\xi _{2c},\xi _{2a}\gamma _{th2} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}\right) \right\} \right\} \right\} . \end{aligned}$$

(60)

Finally, substituting (23), (24), (51), (60) into (20), the outage probability $P_{sout}^{N}$ is obtained as (31).

Appendix 2: Proof of Lemma 1

Based on Taylor expansion, $\phi _K$, $\eta _K$ and the CDF $F_{{\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}}\left( {z}\right)$ are expressed as follows

$$\begin{aligned} \phi _{K}^{\infty }(\gamma _{th1})& = \left( \frac{\gamma _{th1}}{\overline{\gamma }_{{\mathsf {SR}}}}\right) ^{M-K}, \end{aligned}$$

(61)

$$\begin{aligned} \eta _{K}^{\infty }(\gamma _{th1})& = \left[ \frac{(1+{\overline{\gamma }_{{\mathsf {PR}}}})\gamma _{th1}}{\overline{\gamma }_{{\mathsf {SR}}}}\right] ^{M-K}, \end{aligned}$$

(62)

$$\begin{aligned} F_{{\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}}^{\infty }\left( {z}\right)& = \left\{ \begin{array}{ll} \sum \nolimits _{t=0}^{K_N} \frac{\theta _{t}}{A_{t}\overline{\gamma }_{\mathsf {RD}}}z,& \rho\,<\,1,\,\,K\,\,\ge\,\,{N}\\ \theta _{0}\frac{z^{K_{N-1}}}{\overline{\gamma }_{\mathsf {RD}}^{K_{N-1}}}, & \rho =1,\,\,K\ge {N}\\ 1, & K\,<\,N.\\ \end{array} \right. \end{aligned}$$

(63)

Then substituting (63) into (50), the asymptotic $P_{SOA}^{N}$ is derived

$$\begin{aligned} P_{SOA}^{N\infty }& = \left\{ \begin{array}{ll} \frac{\lambda _{N1a}^{\infty }(\gamma _{th2})}{\overline{\gamma }_{\mathsf {RD}}} , &{} \rho\,<\,1\\ \frac{\lambda _{N1b}^{\infty }(\gamma _{th2})}{\overline{\gamma }_{\mathsf {RD}}^{K_{N-1}}} , &{} \rho =1. \end{array} \right. \end{aligned}$$

(64)

Similarly, substituting (63) into (56), the asymptotic $F_{{Z_N}}\left( {z}\right)$ for $K>N$ is derived,

$$\begin{aligned} F_{{\gamma _{Z_{N}}}}^{\infty }\left( {z}\right)& = \left\{ \begin{array}{ll} \sum \limits _{t=0}^{K_N} \frac{\theta _{t}(1+\overline{\gamma }_{\mathsf {PD}})}{A_{t} \overline{\gamma }_{\mathsf {RD}}}z,&{} \rho\,<\,1\\ \theta _{0}\sum \limits _{u=0}^{K_{N-1}}\sum \nolimits _{v=0}^{u} \frac{\kappa _{uv} z^{K_{N-1}-v}}{\overline{\gamma }_{\mathsf {RD}}^{K_{N-1}}}, &{} \rho =1.\\ \end{array} \right. \end{aligned}$$

(65)

Then inserting $F_{{Z_N}}^{\infty }\left( {z}\right)$ into (55), we obtain

$$\begin{aligned}&P_{SOB}^{N\infty } = \left\{ \begin{array}{ll} \frac{\lambda _{N2a}^{\infty }(\gamma _{th2})}{\overline{\gamma }_{\mathsf {RD}}} , &{} \rho\,<\,1\\ \frac{\lambda _{N2b}^{\infty }(\gamma _{th2})}{\overline{\gamma }_{\mathsf {RD}}^{K_{N-1}}} , &{} \rho =1. \end{array} \right. \end{aligned}$$

(66)

Finally, substituting (61) and (64) into (48), the asymptotic $P_{SO1}^{N}$ can be obtained. Similarly, substituting (62) and (66) into (52), the asymptotic $P_{SO2}^{N}$ can be obtained. Then inserting the asymptotic $P_{SO1}^{N}$ and $P_{SO2}^{N}$ into (20), the asymptotic outage probability is derived as (32).

Appendix 3: Proof of Theorem 2

When considering $P_{SO1}^{BF}$, the SUs are not affected by the interference from PUs, i.e., $\alpha =0$. Based on (2), (17), (18), so $P_{SO1}^{BF}$ for the decoding set $|{\mathbb {D}}_{{K_c}}|$ is expressed as

$$\begin{aligned} P_{SO1}^{BF}& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr (\gamma _{{\mathsf {SR}}_k}>\gamma _{th1}) \prod _{m \in {\mathbb {\overline{D}}_{K_c}}}\Pr (\gamma _{{\mathsf {SR}}_m}<\gamma _{th1}) \\&\quad \times\,\Pr \left( \left[ C_{{\mathsf {R}}{\mathsf {D}}}-C_{{\mathsf {R}} {\mathsf {E}}}\right] ^{+}<R_{s}|\alpha =0\right) \\&=\phi _{K}(\gamma _{th1}) \underbrace{\Pr \left( \left[ \frac{1+\gamma _{{\mathsf {R}} {\mathsf {D}}}}{1+\gamma _{{\mathsf {R}}{\mathsf {E}}}}\right] ^{+}<\gamma _{th2}\right) }_{P_{SOA}^{BF}}. \end{aligned}$$

(67)

$P_{SOA}^{BF}=1$ in (67) when $K<N$. Otherwise, with the aid of (33) and (34), $P_{SOA}^{BF}$ is derived as

$$\begin{aligned} {P_{SOA}^{BF}}& = \Pr \left( \frac{1+\gamma _{{\mathsf {R}}{\mathsf {D}}}}{1+\gamma _{{\mathsf {R}} {\mathsf {E}}}}<\gamma _{th2}, \gamma _{{\mathsf {R}}{\mathsf {D}}}>\gamma _{{\mathsf {R}}{\mathsf {E}}}\right) + \Pr \left( \frac{1+\gamma _{{\mathsf {R}}{\mathsf {D}}}}{1+\gamma _{{\mathsf {R}} {\mathsf {E}}}}<\gamma _{th2}, \gamma _{{\mathsf {R}}{\mathsf {D}}}<\gamma _{{\mathsf {R}}{\mathsf {E}}}\right) \\& = \int _{0}^{\infty } \int _{0}^{\gamma _{th2}(1+y)-1} f_{{\gamma _{{\mathsf {R}}{\mathsf {D}}}}}\left( {x}\right) f_{{\gamma _{{\mathsf {R}}{\mathsf {E}}}}}\left( {y}\right) dxdy \\&=1- \sum _{k=0}^{K-1}\sum _{u=0}^{K-1-k} \sum _{v=0}^{u} \frac{\beta _{k}}{u!} {{u}\atopwithdelims (){v}} \frac{\left( \gamma _{th2}-1 \right) ^{u-v}\gamma _{th2}^{v}}{\overline{\gamma }_{RD}^{u} \overline{\gamma }_{\mathsf {RE}}} \exp \left( -\frac{\gamma _{th2}-1}{\overline{\gamma }_{\mathsf {RD}}}\right) \\&\quad \times\,\varGamma (v+1) \left( \frac{\gamma _{th2}}{\overline{\gamma }_{\mathsf {RD}}} +\frac{1}{\overline{\gamma }_{\mathsf {RE}}} \right) ^{-(v+1)}. \end{aligned}$$

(68)

By substituting (49) and (68) into (67), $P_{SO1}^{BF}$ is obtained as

$$\begin{aligned}P_{SO1}^{BF} &=\phi _{K}(\gamma _{th1}) \left\{ \delta (K) + U(K-1) \left[ 1- \sum _{k=0}^{K-1}\sum _{u=0}^{K-1-k} \sum _{v=0}^{u} \frac{\beta _{k}}{u!} {{u}\atopwithdelims (){v}} \gamma _{th2}^{v} \right. \right. \\&\quad \times\,\left. \left. \frac{\left( \gamma _{th2}-1 \right) ^{u-v}}{\overline{\gamma }_{RD}^{u}\overline{\gamma }_{\mathsf {RE}}} \exp \left( -\frac{\gamma _{th2}-1}{\overline{\gamma }_{\mathsf {RD}}}\right) \varGamma (v+1) \left( \frac{\gamma _{th2}}{\overline{\gamma }_{\mathsf {RD}}} +\frac{1}{\overline{\gamma }_{\mathsf {RE}}} \right) ^{-(v+1)} \right] \right\} . \end{aligned}$$

(69)

$P_{SO2}^{BF}$ in (20) should consider the interference from active PUs to the secondary network, i.e., $\alpha =1$, so $P_{SO2}^{BF}$ is expressed as

$$\begin{aligned} P_{SO2}^{BF}& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_k}}{1 +\gamma _{{\mathsf {PR}}_{k}}}>\gamma _{th1}\right) \prod _{m \in {\mathbb {\overline{D}}_{K_c}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_m}}{1 +\gamma _{{\mathsf {PR}}_{m}}}<\gamma _{th1}\right) \\&\quad \times\,\underbrace{\Pr \left( \left[ \frac{1+Z_{B}}{1+Y_{B}}\right] ^{+}<\gamma _{th2}\right) }_{P_{SOB}^{BF}}, \end{aligned}$$

(70)

where $Z_{B}=\frac{\gamma _{{\mathsf {R}}{\mathsf {D}}}}{1+\gamma _{{\mathsf {P}}{\mathsf {D}}}}$, $Y_{B}=\frac{\gamma _{{\mathsf {R}}{\mathsf {E}}}}{1+\gamma _{{\mathsf {P}}{\mathsf {E}}}}$.

When considering ${P_{SOB}^{BF}}$, ${P_{SOB}^{BF}}=1$ for $K<N$, otherwise, ${P_{SOB}^{BF}}$ is derived as

$$\begin{aligned} {P_{SOB}^{BF}} = \int _{0}^{\infty } F_{{Z_B}}\left( {\gamma _{th2}(1+y)-1}\right)\,f_{{Y_B}}\left( {y}\right) dy. \end{aligned}$$

(71)

With the aid of (33) and (34), $F_{{Z_B}}\left( {z}\right)$ and $F_{{Y_B}}\left( {y}\right)$ are derived as follows:

$$\begin{aligned} F_{{Z_B}}\left( {z}\right)& = 1- \sum _{k=0}^{K-1}\sum _{u=0}^{K-1-k} \sum _{v=0}^{u} \frac{\beta _{k}\varGamma (v+1)\overline{\gamma }_{{{\mathsf {P}} {\mathsf {D}}}}^{v}z^{u}}{u!\overline{\gamma }_{RD}^{u}} {u\atopwithdelims (){v}} \\& \quad \times\,\exp \left( -\frac{z}{\overline{\gamma }_{\mathsf {RD}}} \right) \left( \xi _{4a} z +1\right) ^{-(v+1)}, \end{aligned}$$

(72)

$$\begin{aligned} F_{{Y_B}}\left( {y}\right)& = \int _{0}^{\infty }F_{{\gamma _{{\mathsf {R}}{\mathsf {E}}}}}\left( {(1+x)y}\right)\,f_{{\gamma _{{\mathsf {P}}{\mathsf {E}}}}}\left( {x}\right) dx \\& = 1- \exp \left( -\frac{y}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \frac{1}{\xi _{2b} y+1}. \end{aligned}$$

(73)

By differentiating (73), the $f_{{Y_B}}\left( {y}\right)$ is obtained as

$$\begin{aligned}&f_{{Y_B}}\left( {y}\right) = \exp \left( -\frac{y}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \left( \frac{1}{{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}(\xi _{2b} y+1)} +\frac{\xi _{2b}}{(\xi _{2b} y+1)^{2}} \right) . \end{aligned}$$

(74)

By substituting (72) and (74) into (71), ${P_{SOB}^{BF}}$ is derived as

$$\begin{aligned}{P_{SOB}^{BF}} &= 1- \int _{0}^{\infty } \sum _{k=0}^{K-1}\sum _{u=0}^{K-1-k} \sum _{v=0}^{u} \frac{\beta _{k}\varGamma (v+1)\overline{\gamma }_{{{\mathsf {P}}{\mathsf {D}}}}^{v}}{u! \overline{\gamma }_{RD}^{u}} {u\atopwithdelims (){v}} [\gamma _{th2}(1 +y)-1]^{u} \\&\quad \times \,\left[ \xi _{4a}(\gamma _{th2}(1 + y)-1) +1\right] ^{-(v+1)} \exp \left( -\frac{\gamma _{th2}(1 + y)-1}{\overline{\gamma }_{\mathsf {RD}}} -\frac{y}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \\&\quad \times \, \left( \frac{1}{{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}(\xi _{2b} y+1)} +\frac{\xi _{2b}}{(\xi _{2b} y+1)^{2}} \right) dy \\&= 1- \sum _{k=0}^{K-1}\sum _{u=0}^{K-1-k} \sum _{v=0}^{u} \sum _{t=0}^{u} \frac{\beta _{k}\varGamma (v+1)\overline{\gamma }_{{{\mathsf {P}}{\mathsf {D}}}}^{v}}{u! \overline{\gamma }_{RD}^{u}} {u\atopwithdelims (){v}} {u\atopwithdelims (){t}} \gamma _{th2}^{t} [\gamma _{th2}-1]^{u-t} \varGamma (t+1) \\&\quad \times \, \left( \frac{\xi _{4c}}{\xi _{4a}\gamma _{th2}}\right) ^{v+1} \left[ \frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \varPhi _{2}\left( t+1,1,v+1;t+2;\xi _{2b},\xi _{4c},\frac{\gamma _{th2}}{ \overline{\gamma }_{\mathsf {RD}}} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \right. \\&\quad +\,\,\left. \xi _{2b} \varPhi _{2}\left( t+1,2,v+1;t+2;\xi _{2b},\xi _{4c}, \frac{\gamma _{th2}}{\overline{\gamma }_{\mathsf {RD}}} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \right] \exp \left( -\frac{\gamma _{th2}-1}{\overline{\gamma }_{\mathsf {RD}}} \right) . \end{aligned}$$

(75)

By substituting (53), (54), (75) into (70), $P_{SO2}^{BF}$ is obtained

$$\begin{aligned}P_{SO2}^{BF} &= \eta _{K}(\gamma _{th1}) \left\{ \delta (K) + U(K-1) \right. \\&\quad \times \left\{ 1- \sum _{k=0}^{K-1}\sum _{u=0}^{K-1-k} \sum _{v=0}^{u} \sum _{t=0}^{u} \frac{\beta _{k}\varGamma (v+1)\overline{\gamma }_{{{\mathsf {P}} {\mathsf {D}}}}^{v}}{u!\overline{\gamma }_{RD}^{u}} {u\atopwithdelims (){v}} {u\atopwithdelims (){t}} \gamma _{th2}^{t} \right. \\&\quad \times (\gamma _{th2}-1)^{u-t} \left( \frac{\xi _{4c}}{\xi _{4a}\gamma _{th2}}\right) ^{v+1} \exp \left( -\frac{\gamma _{th2}-1}{\overline{\gamma }_{\mathsf {RD}}} \right) \varGamma (t+1) \\&\quad \times \left[ \frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \varPhi _{2}\left( t+1,1,v+1;t+2;\xi _{2b},\xi _{4c},\frac{\gamma _{th2}}{\overline{\gamma }_{\mathsf {RD}}} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \right. \\&\quad +\left. \left. \left. \xi _{2b} \varPhi _{2}\left( t+1,2,v+1;t+2;\xi _{2b},\xi _{4c},\frac{\gamma _{th2}}{\overline{\gamma }_{\mathsf {RD}}} +\frac{1}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \right] \right\} \right\} . \end{aligned}$$

(76)

Finally, substituting (23), (24), (69), (76) into (20), the secrecy outage probability $P_{sout}^{BF}$ is obtained as (35).

Appendix 4: Proof of Theorem 3

We denote $P_{O1}$ and $P_{O2}$ in (38) as $P_{NO1}$ and $P_{NO2}$ respectively.

Furtherly, based on (2), (29) and (49), $P_{NO1}$ for $|{\mathbb {D}}_{{K_c}}|$ is expressed as

$$\begin{aligned}P_{NO1} &= \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr (\gamma _{{\mathsf {SR}}_k}>\gamma _{th1}) \prod _{m \in {\overline{\mathbb {D}}_{K_c}}}\Pr (\gamma _{{\mathsf {SR}}_m}<\gamma _{th1}) \Pr (\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}<\gamma _{th1}) \\& =\phi _{K}(\gamma _{th1}) \left\{ U(N-K) + \left\{ \sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} \left[ 1-\exp \left( -\frac{B_{t}x}{A_{t}\overline{\gamma }_{\mathsf {RD}}}\right) \right] \right\} U(K-N) \right\} . \end{aligned}$$

(77)

$P_{NO2}$ is different from $P_{NO1}$, and the interference from active PUs to the secondary network is considered, i.e., $\alpha =1$. Based on (54) and (56), $P_{NO2}$ is expressed as

$$\begin{aligned} P_{NO2}& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_k}}{1 +\gamma _{{\mathsf {PR}}_{k}}}>\gamma _{th1}\right) \prod _{m \in {\overline{\mathbb {D}}_{K_c}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_m}}{1 +\gamma _{{\mathsf {PR}}_{m}}}<\gamma _{th1}\right) \\&\quad \times \,\Pr \left( \frac{\gamma _{{\mathsf {R}}_{N}{\mathsf {D}}}}{1 +\gamma _{{\mathsf {P}}{\mathsf {D}}}}<\gamma _{th1}\right) \\&= \eta _{K}(\gamma _{th1}) \left\{ U(N-K) + \sum _{t=0}^{K-N} \frac{\theta _{t}}{B_{t}} U(K-N) \right. \\&\quad \times \,\left[ 1- \frac{A_{t}\overline{\gamma }_{{\mathsf {R}}{\mathsf {D}}}}{\gamma _{th1}B_{t} \overline{\gamma }_{{\mathsf {P}}{\mathsf {D}}}+A_{t}\overline{\gamma }_{{\mathsf {R}}{\mathsf {D}}}} \left. \exp \left( -\frac{B_{t}\gamma _{th1}}{A_{t}\overline{\gamma }_{\mathsf {RD}}}\right) \right] \right\} . \end{aligned}$$

(78)

Finally, substituting (23), (24), (77), (78), into (38), the connection outage probability $P_{out}^{N}$ is obtained as (44).

Appendix 5: Proof of Theorem 4

According to (41), we denote $P_{I1}$ and $P_{I2}$ as $P_{NI1}$ and $P_{NI2}$ respectively.

Based on (30) and (49), $P_{NI1}$ can be obtained as

$$\begin{aligned}P_{NI1} &= \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr (\gamma _{{\mathsf {SR}}_k}>\gamma _{th1}) \prod _{m \in {\overline{\mathbb {D}}_{{K_c}}}}\Pr (\gamma _{{\mathsf {SR}}_m}<\gamma _{th1}) \Pr (\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}>\gamma _{th1}) \\&=\exp \left( -\frac{Kx}{\overline{\gamma }_{{\mathsf {SR}}}}\right) \left[ 1-\exp \left( -\frac{x}{\overline{\gamma }_{{\mathsf {SR}}}}\right) \right] ^{M-K} \left[ \exp \left( -\frac{\gamma _{th1}}{\overline{\gamma }_{{\mathsf {R}}_{N}{\mathsf {E}}}} \right) \right] U(K-N), \end{aligned}$$

(79)

where $\Pr \left( \gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}>\gamma _{th1}\right) =0$ when $K<N$.

$P_{NI2}$ will also consider the interference from PUs to the secondary network, i.e., $\alpha =1$. Based on (53), (54) and (57), $P_{NI2}$ is obtained as

$$\begin{aligned} P_{NI2}& = \prod _{k \in {{\mathbb {D}}_{{K_c}}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_k}}{1 +\gamma _{{\mathsf {PR}}_{k}}}>\gamma _{th1}\right) \prod _{m \in {\overline{\mathbb {D}}_{K_c}}}\Pr \left( \frac{\gamma _{{\mathsf {SR}}_m}}{1 +\gamma _{{\mathsf {PR}}_{m}}}<\gamma _{th1}\right) \\&\quad \times \,\Pr \left( \frac{\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}}{1+\gamma _{{\mathsf {P}} {\mathsf {E}}}}>\gamma _{th1}\right) \\&=\eta _{K}(\gamma _{th1}) \exp \left( -\frac{\gamma _{th1}}{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}} \right) \frac{\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}U(K-N)}{\overline{\gamma }_{{{\mathsf {P}}{\mathsf {E}}}}\gamma _{th1} +\overline{\gamma }_{{{\mathsf {R}}{\mathsf {E}}}}}, \end{aligned}$$

(80)

where $\Pr \left( \frac{\gamma _{{\mathsf {R}}_{N}{\mathsf {E}}}}{1+\gamma _{{\mathsf {P}}{\mathsf {E}}}}>\gamma _{th1}\right) =0$ when $K<N$.

Finally, substituting (23), (24), (79), (80) into (41), the probability $P_{int}^{N}$ is obtained as (45).