Modeling the macro-structure of the heart: healthy and diseased

Abstract

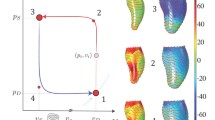
In a common heart pathology, dilated cardiomyopathy, the ventricular chamber undergoes a complex remodeling that changes the shape of the ventricle and the architecture of the fibers in the myocardium. In order to effectively surgically reconstruct the heart it is necessary to understand the relative impact of these changes on heart function. Here we present a series of simulations that model different pathological changes separately. We use the sensitive relationship between twist of the ventricle and its ejection fraction to judge the functional impact of the different damage types. We show that the anomalies found in the relation between torsion and ejection fraction for dilated cardiomyopathy are predominantly caused by the damage to the architecture of the myofibrils of the heart, rather than gross geometry.

Appendices

Appendix 1: Geometrical parameters in formulating the double helical heart—healthy and diseased

In this model we assume that the double helical band to approximate the myocardium fiber architecture. While detailed description of how these equations are formulated will be published elsewhere, we give a brief overview here. As a first step we describe the changing orientation of the fibers by specifying the long-axis coordinate, z _cent, as a function of polar coordinate θ for the centerline of the band:

$$ z_{\rm {cent}} = C_1-C_2{\frac{\theta^{A_1}} {A_2}}{{\exp}}\left[-{\frac{\theta} {B_2}}\right] $$

(3)

where C ₁, C ₂, A ₁, A ₂, B ₂ are constants. The polar coordinate θ varies $\theta_{{\text {max}}} \geq \theta \geq 0,$ where θ _max corresponds to the start of the band at the pulmonary aorta, and θ = 0 corresponds to the end of the ascending segment.

The r-coordinate of the centerline should now be constructed in such a way that the centerline correctly skirts the ventricular chamber surfaces. So for a parabolic chamber the centerline r _cent:

$$ \begin{aligned}{}&r_{{\text {cent}}} = \sqrt{{\frac{1} {A_3}}z_{{\text {cent}}}+{\text {ZA}}*C_3}[\hbox{Factor}\,1] [\hbox{Factor}\,2],\\&\hbox{Factor}\,1 = 1+C_4\cos{\left({\frac{\theta-\theta_{{\text {crossing}}}} {2}}\right)} ,\\ &\hbox{Factor}\,2 = 1+C_5{\text {exp}}{\left(-(\theta-\theta_{{\text {right}}})^2\right)},\end{aligned} $$

(4)

where, A ₃, ZA, C ₃, C ₄ and C ₅ are constants. We introduce Factor 1 to avoid self-overlap of the band at $\theta_{\rm crossing} = \pi \left( -1 + \cot {\frac{\pi} {A_1 B_2}}\right)$ and θ _crossing + 2π. The factor bends the centerline away from self-crossing. We also introduce Factor 2, which is only significant in the region of anterior surface and describes the fact that this surface bends significantly outwards from the septum. We choose the middle of anterior surface to correspond to θ _right = 3.8 π. The position of the apex of the left ventricular chamber is taken to be at z = C ₃. The constants of Eqs. 3 and 4 are not independent when related to the geometry of the heart, for example a combined change in A ₁ and ZA controls the fiber orientation in the region of the apex. The shape of the chamber can be controlled by changes in the constant A ₃. The centerline can also be written in a similar way for a spherical chamber:

$$ r_{\text{cent}} = \sqrt{R_0^2-[z_{\text{cent}}+\text{ZA}*C_3-R_0]^2}[\text{Factor}\ 1] [\text{Factor} \ 2] $$

(5)

where R ₀ characterizes the spherical shape of the ventricle, while all the other constants are the same as for Eq. 4.

To make the mathematical description of the 3D shape of the band simpler, we approximate the direction of the width to be along the z axis. The parameterized three dimensional structure is then described in cartesian coordinate, (x, y, z), by:

$$ \begin{aligned} z &= z_{{\text {cent}}} + \xi \quad r = r_{{\text {cent}}}(z) + \zeta \\ x &= r \cos{\theta} \quad y = r \sin{\theta} \\ \end{aligned} $$

(6)

where −w/2 < ξ < w/2 and 0 < ζ < t, with w and t are the width and the thickness of the band, respectively.

The parameters that remain constant for all models have values of: C ₁ = 4.9, C ₂ = 7, A ₂ = 0.5, C ₃ = −0.77, C ₄ = 1/6 and C ₅ =  1. The following is the list of the models used in this paper and the parameter values for each one (unless otherwise specified Eq. 4 was used):

• Normal: A ₁ = 1.3, ZA = 1.1, A ₃ = 1.

• Weakly Oblique: A ₁ = 1.1, ZA = 1.4, A ₃ = 1.

• Moderately Oblique: A ₁ = 1.0, ZA = 1.6, A ₃ = 1.

• Strongly Oblique: A ₁ = 0.9, ZA = 1.7, A ₃ = 1.

• Moderately Dilated: A ₁ = 1.3, ZA = 1.1, A ₃ = 1/4.

• Strongly Dilated: in this case equation (5) was used with A ₁ =  1.3, ZA = 1.1, A ₃ = 1, R ₀ = 3.8.

• Other Damage: A ₁ = 1.3, ZA = 1.1, A ₃ = 1.

Appendix 2: Calculating twist of the left ventricle

In order to measure twist we attach a strip of soft material to the left ventricle. This strip of material (twist indicator) is made of a material that is two orders of magnitude softer than the non-active material (i.e. the Young’s modulus of the twist indicator material is one hundredth of the Young’s modulus of the non-active material). As a result the twist indicator does not influence the movement of the band. In its initial configuration the strip is on a vertical plane containing the long axis of the left ventricle and at θ = 2.96π. During the deformation of the band the strip can deform in plane or out of the plane. When the long axis of the left ventricle shortens during contraction the indicator strip deforms in plane. In the shortening of the long-axis the top and bottom portions of the left ventricle band come closer together, and to accommodate this deformation the strip buckles outwards. However, unless the top and bottom portions of the band rotate with respect to each other, the buckling will occur in the same vertical plane. Conversely, in twisting of the left ventricle the bottom and top portions of the band move with respect to each other in the horizontal plane. As a result, the strip deforms out of plane. The greater the twist of the left ventricle, the greater the out of plane deformation. To measure the out of plane deformation we keep track of the position of the nodes on the strip indicator. The way we calculate the out of plane distance is akin to the least squares method in 3D, we imagine two parallel planes sandwiching these nodes and minimize the distance between the planes. Because the two planes are always parallel (i.e. the normals are the same) there are two possible arrangements: In the first configuration, one plane is defined by a triplet of points, while the other is defined by a single point and the the normal of the first plane. In the second possible configuration, each plane contains a pair of points. Once the minimum distance, the minimal sandwich thickness, is calculated we can translate that measure directly into twist or torsion. Twist of the left ventricle is normally defined as the slope of the fit line to the relationship between the rotational angle of a marker and its long-axial position. Torsion of the left ventricle is defined as the average of the rotations of markers placed at different positions of the long-axis. We could use the nodes of the twist indicator as markers and calculate the rotation of each one and then average to calculate torsion or twist, but this would only introduce unnecessary errors. Instead, we directly relate the out of plane deformation of the twist indicator to the values of twist and torsion. Assuming that the twist changes linearly with the z−coordinate of the long axis, with no twist at the basal plane and maximal twist at the apex, the maximum out of plane movement will be at ${\frac{1} {3}}$ LA from the apex in the case of a parabolic and $(1-1/\sqrt{2})R_0$ in the case of a spherical chamber, where LA and R ₀ is the long axis and the sphere radius, respectively. As a result twist is calculated from out of plane deformation (d) of the twist indicator:

$$ \hbox{twist}_{\rm parabolic}=-{\frac{3\sqrt{3}} {2}}\,{\frac{d} {(\hbox{LA})(\hbox{r}_{\rm base})}} $$

(7)

$$ \hbox{twist}_{\rm sphere}=-{\frac{2d} {R_0^2}} $$

(8)

where, LA and r _base are the long axis length and radius at the base (top), respectively. Once twist is known torsion can be calculated from the relationship between twist and torsion torsion =(LA) twist/2:

$$ \hbox{torsion}_{\rm parabolic}={\frac{3\sqrt{3}} {4}}\,{\frac{d} {r_{{\text {base}}}}} $$

(9)

$$ \hbox{torsion}_{\rm sphere}={\frac{d} {R_0}} $$

(10)