Simulation models for mobile-to-mobile channels with isotropic and nonisotropic scattering

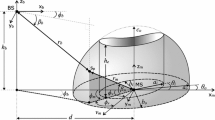
Abstract

Recent radio channel measurements in peer-to-peer (P2P) and mobile-to-mobile network indicate that, depending on the mobility of the terminals and scattering properties of the environment, the predominate fading mechanism contains a combination of Rayleigh and double Rayleigh fading with or without line-of-sight (LoS) component. In this paper, we present the statistical and deterministic models by the sum-of-complex-sinusoids to simulate the channels in isotropic and non-isotropic scattering. The auto- and cross correlations of the in-phase, quadrature components, and squared envelopes of the channels are derived. Extensive Monte Carlo simulations are performed to validate the statistical properties of the proposed models. The time-average statistical properties and the corresponding variances are also investigated to justify that the models achieve satisfactory convergence performance.

Appendices

Appendix A: Proof of Eqs. 13 and 16, auto- and cross correlations for model ST-ISO-LoS

The real and imaginary parts of h_ST(t) can be expressed, respectively, as

$$ \begin{array}{@{}rcl@{}} &h_{c} (t)=\mathfrak{Re}\left[h_{\text{ST}}(t)\right)=\frac{1}{\sqrt{1+K}}\left( g_{c}(t)+\sqrt{K} \cos L(t) \right], \end{array} $$

(48)

$$ \begin{array}{@{}rcl@{}} &h_{s} (t)=\mathfrak{Im}\left[ h_{\text{ST}}(t)\right)=\frac{1}{\sqrt{1+K}}\left( g_{s}(t)+\sqrt{K}\sin L(t) \right] \end{array} $$

(49)

where g_c(t) and g_s(t) are the real and imaginary parts of g_ST(t), and $L(t)=2\pi f_{3} t \cos \limits \phi _{3}+\phi _{0}$. The ACF for h_ST(t) is calculated as

$$ \begin{array}{@{}rcl@{}} &&\mathbb{E}\left[h_{c}(t) h_{c}(\varrho)\right]=\frac{1}{1+K}\\ &&\mathbb{E}\left[\left( g_{c}(t)+\sqrt{K} \cos L(t) \right) \left( g_{c}(\varrho) + \sqrt{K} \cos L(\varrho) \right) \right] \end{array} $$

(50)

The CCF for h_ST(t) is obtained as

$$ \begin{array}{@{}rcl@{}} &&\mathbb{E}\left[h_{c}(t) h_{s}(t + \tau)\right] = \frac{1}{1+K}\\ &&\mathbb{E}\left[\left( g_{c}(t)+\sqrt{K} \cos L(t) \right) \left( g_{s}(t)+\sqrt{K}\sin L(t) \right) \right]. \end{array} $$

(51)

Note that phase ϕ₀ in L(t) and L(ϱ) is independent of other random variables in g_c(t) and g_s(t). By taking the expectation with respect to ϕ₀, one can obtain the autocorrelation and cross-correlation as specified in Eq. 13 and Eq. 15 using the results below:

$$ \begin{array}{@{}rcl@{}} R_{g_{c}g_{c}}(\tau)&=&\mathbb{E}\left[g_{c}(t) g_{c}(t+\tau)\right]=J_{0}(2\pi f_{1} \tau)J_{0}(2\pi f_{2}\tau) \end{array} $$

(52)

$$ \begin{array}{@{}rcl@{}} R_{g_{s}g_{s}}(\tau)&=&\mathbb{E}\left[g_{s}(t) g_{s}(t+\tau) \right]=R_{g_{c}g_{c}}(\tau) \end{array} $$

(53)

$$ \begin{array}{@{}rcl@{}} R_{g_{c}g_{s}}(\tau)&=&\mathbb{E}\left[g_{c}(t)g_{s}(t+\tau) \right]=0, R_{g_{s}g_{c}}(\tau)\\ &=&\mathbb{E}\left[g_{s}(t)g_{c}(t+\tau) \right]=0 \end{array} $$

(54)

1.1 Proof for Eqs. 52 and 54

From Eq. 9, the real and imaginary parts of g_ST(t) are given, respectively, by

$$ \begin{array}{@{}rcl@{}} g_{c} (t)&=&\mathfrak{Re}\left[g_{\text{ST}}(t)\right]=\sqrt{\frac{2}{NM}} \sum\limits_{n,m=1}^{N,M} \left( A_{n}(t)B_{m}(t)-C_{n}(t)D_{m}(t)\right) \end{array} $$

(55)

$$ \begin{array}{@{}rcl@{}} g_{s} (t)&=&\mathfrak{Im}\left[ g_{\text{ST}}(t)\right]=\sqrt{\frac{2}{NM}} \sum\limits_{n,m=1}^{N,M} \left( A_{n}(t)D_{m}(t)+C_{n}(t)B_{m}(t)\right) \end{array} $$

(56)

For brevity of notations, we replace t + τ with ϱ in the proceeding appendices. The autocorrelation can be obtained by

$$ \begin{array}{@{}rcl@{}} \mathbb{E}\left[g_{c}(t) g_{c}(\varrho)\right]&=&\frac{2}{NM}\mathbb{E}\left[ \sum\limits_{n,m=1}^{N,M} \left( A_{n}(t)B_{m}(t)-C_{n}(t)D_{m}(t)\right) \sum\limits_{k,j=1}^{N,M} \left( A_{k}(\varrho) B_{j}(\varrho)- C_{k}(\varrho) D_{j}(\varrho)\right) \right]\\ &=& \frac{2}{NM}\mathbb{E}\left[\sum\limits_{n,k=1}^{N,N} A_{n}(t)A_{k}(\varrho)\sum\limits_{m,j=1}^{M,M} B_{m}(t)B_{j}(\varrho)+ \sum\limits_{n,k=1}^{N,N} C_{n}(t)C_{k}(\varrho)\sum\limits_{m,j=1}^{M,M} D_{m}(t)D_{j}(\varrho)\right.\\ &&\left.-\sum\limits_{n,k=1}^{N,N} A_{n}(t)C_{k}(\varrho)\sum\limits_{m,j=1}^{M,M} B_{m}(t)D_{j}(\varrho)-\sum\limits_{n,k=1}^{N,N} C_{n}(t)A_{k}(\varrho)\sum\limits_{m,j=1}^{M,M} D_{m}(t)B_{j}(\varrho) \right] \end{array} $$

(57)

The cross-correlation is evaluated as

$$ \begin{array}{@{}rcl@{}} \mathbb{E}\left[g_{c}(t) g_{s}(\varrho)\right]=\mathbb{E}\left[ \frac{2}{NM} \sum\limits_{n,m=1}^{N,M} \left( A_{n}(t)B_{m}(t)-C_{n}(t)D_{m}(t)\right) \sum\limits_{j,k=1}^{N,M} \left( A_{j}(\varrho)D_{k}(\varrho)+C_{j}(\varrho)B_{k}(\varrho)\right) \right] \end{array} $$

(58)

Since 𝜃_n,Θ_n,Φ_m,Ψ_m ∈ [−π,π) are statistically independent and uniformly distributed for all n and m, we have $\mathbb {E} [{\sum }_{n,k=1, n\neq k}^{N,N} A_{n}(t)A_{k}(\varrho )] = \mathbb {E} [{\sum }_{n,k=1, n\neq k}^{N,N} C_{n}(t)C_{k}(\varrho )]=0$, and $\mathbb {E} [{\sum }_{m,j=1, m\neq j}^{M,M} B_{m}(t)B_{j}(\varrho )]= \mathbb {E} [{\sum }_{m,j=1, m\neq j}^{M,M} D_{m}(t)D_{j}(\varrho )]=0$. Using the following identities which are easily obtained from [51, (p. 420-421)], one can justify $\mathbb {E}\left [g_{c}(t) g_{c}(\varrho )\right ]=J_{0}(2\pi f_{1} \tau ) J_{0}(2\pi f_{2} \tau )$ and $\mathbb {E}\left [g_{c}(t) g_{s}(\varrho )\right ]=0$.

$$ \begin{array}{@{}rcl@{}} &&\mathbb{E}\left [\sum\limits_{n,k=1}^{N,N} A_{n}(t)A_{k}(\varrho)\right]= \mathbb{E} \left[{\sum\limits_{n}^{N}} A_{n}(t)A_{n}(\varrho)\right]= \frac{1}{2} \mathbb{E} \left[ \sum\limits_{n=1}^{N} \tilde{A}_{n}(\tau)\right]= \frac{N}{2} J_{0}(2\pi f_{1} \tau) \end{array} $$

(59)

$$ \begin{array}{@{}rcl@{}} &&\mathbb{E} \left[\sum\limits_{n,k=1}^{N,N} C_{n}(t)C_{k}(\varrho)\right]= \mathbb{E} \left[{\sum\limits_{n}^{N}} C_{n}(t)C_{n}(\varrho)\right]= \frac{1}{2} \mathbb{E} \left[\sum\limits_{n=1}^{N} \tilde{C}_{n}(\tau)\right]= \frac{N}{2} J_{0}(2\pi f_{1} \tau) \end{array} $$

(60)

$$ \begin{array}{@{}rcl@{}} && \sum\limits_{m=1,j}^{M,M} \mathbb{E}[ B_{m}(t)B_{j}(\varrho)] = \sum\limits_{m=1}^{M} \mathbb{E}[ B_{m}(t)B_{m}(\varrho)] = \frac{1}{2} \mathbb{E} \left[ \sum\limits_{m=1}^{M} \tilde{B}_{m}(\tau)\right] = \frac{M}{2} J_{0}(2\pi f_{2} \tau ) \end{array} $$

(61)

$$ \begin{array}{@{}rcl@{}} && \sum\limits_{m=1,j}^{M,M} \mathbb{E}[ D_{m}(t)D_{j}(\varrho)] = \sum\limits_{m=1}^{M} \mathbb{E}[ D_{m}(t)D_{m}(\varrho)] = \frac{1}{2} \sum\limits_{m=1}^{M} \mathbb{E} [\tilde{D}_{m}(\tau) ]= \frac{M}{2} J_{0}(2\pi f_{2} \tau ) \end{array} $$

(62)

$$ \begin{array}{@{}rcl@{}} &&\sum\limits_{n,k=1}^{N,N}\mathbb{E}[A_{n}(t)C_{k}(\varrho)]= \sum\limits_{m,j=1}^{M,M}\mathbb{E}[B_{m}(t)D_{j}(\varrho)]=0. \end{array} $$

(63)

Appendix B: Proof of Eq. 18: Squared envelope correlation for Model ST-ISO-LoS

The squared envelope correlation for h_ST(t) contains four terms as indicated in Eq. 17. The first term is evaluated as

$$ \begin{array}{@{}rcl@{}} \mathbb{E}\left[h_{c}^{2}(t) {h_{c}^{2}}(\varrho)\right]&=&\frac{1}{(1+K)^{2}}\mathbb{E}\left[\left( g_{c}(t)+\sqrt{K} \cos L(t) \right)^{2}\left( g_{c}(t)+\sqrt{K} \cos L(t) \right)^{2}\right] \end{array} $$

(64)

$$ \begin{array}{@{}rcl@{}} &=&\frac{1}{(1+K)^{2}}\mathbb{E}\left[\left( {g^{2}_{c}}(t)+ 2\sqrt{K} g_{c}(t)\cos L(t) + K \cos^{2} L(t)\right) \right.\\ &&\left.\times \left( {g^{2}_{c}}(\varrho)+ 2\sqrt{K} g_{c}(\varrho)\cos L(\varrho) + K \cos^{2} L(\varrho) \right)\right] \end{array} $$

(65)

Note that $\mathbb {E}[g_{c}(t)]=\mathbb {E}[g_{c}(\varrho )]=0$, $\mathbb {E}[\cos \limits \left (\ell L(t)\right )]=\mathbb {E}[\cos \limits \left (\ell L(\varrho )\right )]=0,\ell =1,2$, and $\mathbb {E}[{g^{2}_{c}}(t)] =\mathbb {E}[{g^{2}_{c}}(\varrho )]=R_{g_{c}g_{c}}(0)=1$. We have

$$ \begin{array}{@{}rcl@{}} \mathbb{E}\left[h_{c}^{2}(t) {h_{c}^{2}}(\varrho)\right]&=&\frac{1}{(1+K)^{2}}\left( \mathbb{E}\left[g_{c}^{2}(t) {g_{c}^{2}}(\varrho)\right] + {4K}R_{g_{c} g_{c}}(\tau)\mathbb{E}\left[ \cos L(t) \cos L(\varrho)\right] \right. \end{array} $$

(66)

$$ \begin{array}{@{}rcl@{}} &&\left.+K \mathbb{E}\left[ \cos^{2} L(t) +\cos^{2} L(\varrho) \right] +K^{2} \mathbb{E}\left[ \cos^{2} L(t) \cos^{2} L(\varrho) \right] \right) \end{array} $$

(67)

The autocorrelation of the squared quadrature component $\mathbb {E}\left [h_{s}^{2}(t) {h_{s}^{2}}(\varrho )\right ]$ can be evaluated following the similar steps. Using identities $\mathbb {E}\left [ {\cos \limits } L(t) {\cos \limits } L(\varrho )\right ]= \frac {1}{2}\cos \limits \left (2\pi f_{3} \tau \cos \limits \phi _{3}\right ), \mathbb {E}\left [ \cos \limits ^{2} L(t)\right ]=\mathbb {E}\left [\cos \limits ^{2} L(\varrho ) \right ]=\frac {1}{2}$, and $\mathbb {E}\left [ \cos \limits ^{2} L(t) \cos \limits ^{2} L(\varrho ) \right ]= \frac {1}{4}+ \frac {1}{8}\cos \limits \left (4\pi f_{3} \tau \cos \limits \phi _{3}\right )$, we summarize the auto- and cross-correlations, respectively, as

$$ \begin{array}{@{}rcl@{}} &&\mathbb{E}\left[h_{x}^{2}(t) {h_{x}^{2}}(\varrho)\right] = \frac{8\mathbb{E}\left[g_{x}^{2}(t) {g_{x}^{2}}(\varrho)\right] + 16K R_{g_{x} g_{x}}(\tau) \cos\left( 2\pi f_{3} \tau \cos\phi_{3}\right) + {8K + 2K^{2} + K^{2} \cos\left( 4\pi f_{3} \tau \cos\phi_{3}\right)}}{8(1+K)^{2}} \end{array} $$

(68)

$$ \begin{array}{@{}rcl@{}} &&\mathbb{E}\left[h_{c}^{2}(t) {h_{s}^{2}}(\varrho)\right]= \mathbb{E}\left[h_{s}^{2}(t) {h_{c}^{2}}(\varrho)\right]=\frac{8\mathbb{E}\left[g_{c}^{2}(t) {g_{s}^{2}}(\varrho)\right]+ {8K + 2K^{2} - K^{2} \cos\left( 4\pi f_{3} \tau \cos\phi_{3}\right)}}{8(1+K)^{2}} \end{array} $$

(69)

where x = {c,s}. Note $R_{|g|^{2}|g|^{2}}(\tau ) =\mathbb {E}\left [g_{c}^{2}(t) {g_{c}^{2}}(\varrho )\right ]+\mathbb {E}\left [g_{c}^{2}(t) {g_{s}^{2}}(\varrho )\right ]+\mathbb {E}\left [g_{s}^{2}(t) {g_{c}^{2}}(\varrho )\right ]+\mathbb {E}\left [g_{s}^{2}(t) {g_{s}^{2}}(\varrho )\right ]$. Inserting Eq. 68 and Eq. 69 into Eq. 17 yields Eq. 18.

1.1 Proof for $R_{|g|^{2}|g|^{2}}(\tau )$ in Eq. 20

The first term in the squared envelope correlation $R_{|g|^{2}|g|^{2}}(\tau )$ is expressed as

$$ \begin{array}{@{}rcl@{}} \mathbb{E}\left[g_{c}^{2}(t) {g_{c}^{2}}(\varrho)\right]&=&\frac{4}{N^{2}M^{2}} \mathbb{E}\left[\sum\limits_{n,m=1}^{N,M} \left( A_{n}(t)B_{m}(t) - C_{n}(t)D_{m}(t)\right) \sum\limits_{u,p=1}^{N,M} \left( A_{u}(t)B_{p}(t)- C_{u}(t)D_{p}(t)\right) \right.\\ &&\left.\times \sum\limits_{q,r=1}^{N,M}\left( A_{q}(\varrho)B_{r}(\varrho) -C_{q}(\varrho) D_{r}(\varrho)\right) \sum\limits_{s,j=1}^{N,M} \left( A_{s}(\varrho) B_{j}(\varrho) - C_{s}(\varrho)D_{j}(\varrho)\right)\right]. \end{array} $$

(70)

Expanding Eq. 70 and taking expectation with respect to the phases, we obtain $\mathbb {E}\left [g_{c}^{2}(t) {g_{c}^{2}}(\varrho )\right ]=\frac {4}{N^{2}M^{2}}({\Upsilon }_{A} {\Upsilon }_{B}+{\Upsilon }_{C} {\Upsilon }_{D}+ \frac {M^{2}N^{2}}{8}+ 4 \kappa )$, where ${\Upsilon }_{X}= \mathbb {E}\left [{\sum }_{n}^{N} X_{n}(t) {{\sum }_{u}^{N}} X_{u}(t) {{\sum }_{q}^{N}} X_{q}(\varrho ) {{\sum }_{s}^{N}} X_{s}(\varrho )\right ]$, where X = {A,B,C,D}, and

$$ \begin{array}{@{}rcl@{}} \kappa=\mathbb{E}\left[ \sum\limits_{n,s}^{N,N} A_{n}(t) A_{s}(\varrho) \sum\limits_{u,q}^{N,N} C_{u}(t) C_{q}(\varrho) \sum\limits_{m,j}^{M,M} B_{m}(t)B_{j}(\varrho) \sum\limits_{p,r=1}^{M,M}D_{p}(t) D_{r}(\varrho)\right] \end{array} $$

(71)

Similarly, we have $\mathbb {E}\left [g_{c}^{2}(t) {g_{s}^{2}}(\varrho )\right ]=\mathbb {E}\left [g_{s}^{2}(t) {g_{c}^{2}}(\varrho )\right ] = \frac {4}{N^{2}M^{2}}\left (\frac {M^{2}}{4}({\Upsilon }_{A}+{\Upsilon }_{C})+\frac {N^{2}}{4}({\Upsilon }_{B}+{\Upsilon }_{D})-4 \kappa \right )$, and $\mathbb {E}\left [g_{s}^{2}(t) {g_{s}^{2}}(\varrho )\right ]=\frac {4}{N^{2}M^{2}}({\Upsilon }_{A} {\Upsilon }_{D}+{\Upsilon }_{C} {\Upsilon }_{B}+ \frac {M^{2}N^{2}}{8}+ 4 \kappa )$. The term Υ_A is evaluated as

$$ \begin{array}{@{}rcl@{}} {\Upsilon}_{A} &=& \mathbb{E}\left[ {\sum\limits_{p}^{N}} {A^{2}_{p}}(t){\sum\limits_{j}^{N}} {A^{2}_{j}}(\varrho)\right]\\ &&+\mathbb{E}\left[ \sum\limits_{n,u, n \neq u}^{N,N} A_{n}(t)A_{u}(t)\sum\limits_{q,s, q \neq s}^{N,N} A_{q}(\varrho)A_{s}(\varrho) \right] \end{array} $$

(72)

The first term in Eq. 72 is obtained as

$$ \begin{array}{@{}rcl@{}} \mathbb{E}\left[ {\sum\limits_{p}^{N}} {A^{2}_{p}}(t){\sum\limits_{j}^{N}} {A^{2}_{j}}(\varrho)\right] =\frac{2N^{2}+NJ_{0}(4 \pi f_{1} \tau)}{8}. \end{array} $$

(73)

The second term in Eq. 72 contains the following seven cases:

• Case 1. n≠u,q≠s,n = q,u≠s;

• Case 2. n≠u,q≠s,n = s,q≠u;

• Case 3. n≠u,q≠s,u = q,n≠s;

• Case 4. n≠u,q≠s,u = s,n≠q;

• Case 5. n≠u,q≠s,n≠q,u≠s;

• Case 6. n≠u,q≠s,n = q,u = s;

• Case 7. n≠u,q≠s,n = s,u = q.

The value of $\mathbb {E}\left [ {{\sum }_{n}^{N}} A_{n}(t)A_{n}(\varrho ){\sum }_{s, s\neq n}^{N} A_{s}(t)A_{s}(\varrho )\right ]$ is zero for Cases 1 to 5 and identical for Cases 6 and 7, and $\mathbb {E}\left [ {{\sum }_{n}^{N}} A_{n}(t)A_{n}(\varrho ){\sum }_{s, s\neq n}^{N} A_{s}(t)A_{s}(\varrho )\right ]_{\text {case 6 or 7}} =\frac {N^{2}{J^{2}_{0}}(2 \pi f_{1} \tau ) - {{\sum }_{n}^{N}} \left (\mathbb {E}[\tilde {A}_{n}(\tau )]\right )^{2}}{4}$. Therefore, we have ${\Upsilon }_{A}= \frac {1}{8}\left (2N^{2}+NJ_{0}(4 \pi f_{1} \tau )+ 4N^{2}{J^{2}_{0}}(2 \pi f_{1} \tau )\right )- \frac {1}{2} {{\sum }_{n}^{N}} \left (\mathbb {E}[\tilde {A}_{n}(\tau ) ]\right )^{2}$. In fact, the value of Υ_A can also be obtained following the steps in [31, Appendix I Eq.(48)].

Using the same procedure on Υ_C, we have ${\Upsilon }_{C}= \frac {1}{8} \left (2N^{2}+NJ_{0}(4 \pi f_{1} \tau )+ 4N^{2}{J^{2}_{0}}(2 \pi f_{1} \tau )\right ) -\frac {1}{2} {{\sum }_{n}^{N}} \left (\mathbb {E}[\tilde {C}_{n}(\tau ) ]\right )^{2}$. It is straightforward to justify $\mathbb {E}[\tilde {C}_{n}(\tau )]=\mathbb {E}[\tilde {C}_{N-n}(\tau )], n=1, \cdots , N$ by the following steps:

$$ \begin{array}{@{}rcl@{}} \mathbb{E}[\tilde {C}_{n}(\tau)]&=& {\int}_{-\pi}^{\pi}\cos \left( 2 \pi f_{1} \tau \cos (\frac{2n\pi - \pi +\psi}{4N})\right)\frac{1}{2\pi} d\psi \\ &=& {\int}_{\frac{(n-1)\pi}{2N}}^{\frac{n \pi}{2N}}\cos \left( 2 \pi f_{1} \tau \cos \theta \right) \frac{4N}{2\pi} d\psi\\ & =& {\int}_{\frac{(N-n-1)\pi}{2N}}^{\frac{(N-n) \pi}{2N}}\cos \left( 2 \pi f_{1} \tau \sin \varphi \right) \frac{4N}{2\pi} d\varphi = \mathbb{E}[\tilde {C}_{N-n}(\tau)]\\ \end{array} $$

(74)

Denote $\xi (f_{1},\tau )= {{\sum }_{n}^{N}} \left (\mathbb {E}\left [\tilde {A}_{n}(\tau ) \right ]\right )^{2}={{\sum }_{n}^{N}} \left (\mathbb {E}\left [\tilde {C}_{n}(\tau ) \right ]\right )^{2}$. Then, we have

$$ \begin{array}{@{}rcl@{}} {\Upsilon}_{A}&= {\Upsilon}_{C}= \frac{2N^{2}+NJ_{0}(4 \pi f_{1} \tau)+ 4N^{2}{J^{2}_{0}}(2 \pi f_{1} \tau)}{8} -\frac{\xi(f_{1},\tau)}{2} \end{array} $$

(75)

$$ \begin{array}{@{}rcl@{}} {\Upsilon}_{B}&= {\Upsilon}_{D}= \frac{2M^{2}+MJ_{0}(4 \pi f_{2} \tau)+4 M^{2}{J^{2}_{0}}(2 \pi f_{2} \tau)}{8}-\frac{ \xi(f_{2},\tau)}{2}, \end{array} $$

(76)

where $\xi (f_{2},\tau )={{\sum }_{m}^{M}} \left (\mathbb {E}\left [\tilde {B}_{m}(\tau ) \right ]\right )^{2}={{\sum }_{m}^{M}} \left (\mathbb {E}\left [\tilde {D}_{n}(\tau ) \right ]\right )^{2}$. Inserting the results for $\mathbb {E}\left [g_{c}^{2}(t) {g_{c}^{2}}(\varrho )\right ], \mathbb {E}\left [g_{c}^{2}(t) {g_{s}^{2}}(\varrho )\right ], \mathbb {E}\left [g_{s}^{2}(t) {g_{c}^{2}}(\varrho )\right ]$, and $\mathbb {E}\left [g_{s}^{2}(t) {g_{s}^{2}}(\varrho )\right ]$ into $R_{|g|^{2}|g|^{2}}(\tau )$, we obtain

$$ \begin{array}{@{}rcl@{}} R_{|g|^{2}|g|^{2}}(\tau) &=& \frac{1}{N^{2}M^{2}}\left( 2{\Upsilon}_{A} +2{\Upsilon}_{C}+ {N^{2}}\right)\\ &&\left( 2{\Upsilon}_{B}+ 2{\Upsilon}_{D}+{M^{2}}\right) \end{array} $$

(77)

$$ \begin{array}{@{}rcl@{}} &=& \frac{1}{N^{2}M^{2}}\left( 4{\Upsilon}_{A} + {N^{2}}\right)\left( 4{\Upsilon}_{B}+{M^{2}}\right). \end{array} $$

(78)

Inserting the results in Eq. 75 and Eq. 76 into Eq. 78 yields Eq. 20.

Appendix C: Proof of Eq. 27: Variance of time-average correlations for Model ST-ISO-LoS

We present the derivation of the variance of the time-average autocorrelation for the in-phase component in Eq. 27. The same steps can be applied to obtain that for the quadrature component. Recall $\text {Var}\left [\tilde {R}_{{h_{c}}h_{c}}(\tau ) \right ] =\mathbb {E}[\tilde {R}_{{h_{c}}h_{c}}^{2}(\tau )]- \left ({R}_{{h_{c}}h_{c}}(\tau ) \right )^{2}$. We have

$$ \begin{array}{@{}rcl@{}} \mathbb{E}[\tilde{R}_{{h_{c}}h_{c}}^{2}(\tau)]&=&\mathbb{E}\left[\left( \frac{2\tilde{R}_{{g_{c}}g_{c}}(\tau) +K \cos\left( 2\pi f_{3} \tau \cos\phi_{3} \right)}{2(1+K)} \right)^{2}\right] \end{array} $$

(79)

$$ \begin{array}{@{}rcl@{}} &=&\frac{1}{4(1+K)^{2}} \left( 4 \mathbb{E}\left[ \tilde{R}_{{g_{c}}g_{c}}^{2}(\tau)\right]+ 4K\cos\left( 2 \pi f_{3} \tau \cos\phi_{3} \right)\mathbb{E}\left[ \tilde{R}_{{g_{c}}g_{c}}(\tau)\right]+\left( K\cos(2\pi f_{3}\tau \cos\phi_{3}) \right)^{2}\right) \end{array} $$

(80)

Note $\mathbb {E}[\tilde {R}_{g_{c} g_{c}} (\tau )] = J_{0} (2\pi f_{1} \tau )J_{0} (2\pi f_{2} \tau )$ and $\text {Var}[\tilde {R}_{g_{c} g_{c}}(\tau ) ] =\mathbb {E}[ \tilde {R}_{g_{c} g_{c}}^{2}(\tau )]-({R}_{g_{c} g_{c}}(\tau ))^{2}$. Using the results obtained for ${R}_{{h_{c}}h_{c}}(\tau )$ in Appendix A, one can achieve Eq. 27. Steps to obtain $\text {Var}[\tilde {R}_{g_{c} g_{c}}(\tau ) ]$ in Eq. 34 is presented below in Appendix C-A.

1.1 Proof for $\text {Var}[\tilde {R}_{g_{c} g_{c}}(\tau ) ]$ in Eq. 34

The variance of the time-average autocorrelation of the real part for g_ST(t) is $\text {Var}[\tilde {R}_{g_{c} g_{c}}(\tau ) ] =\mathbb {E}[ \tilde {R}_{g_{c} g_{c}}^{2}(\tau )]-({R}_{g_{c} g_{c}}(\tau ))^{2}$. The first term is evaluated as

$$ \begin{array}{@{}rcl@{}} \mathbb{E}[ \tilde{R}_{g_{c} g_{c}}^{2}(\tau)] &=& \frac{1}{4M^{2}N^{2}} \mathbb{E}\left[\sum\limits_{n,m=1}^{N,M} \left( \tilde{A}_{n}(\tau) \tilde{B}_{m}(\tau) + \tilde{C}_{n}(\tau) \tilde{D}_{m}(\tau)\right) \sum\limits_{p,q=1}^{N,M} \left( \tilde{A}_{p}(\tau) \tilde{B}_{q}(\tau) + \tilde{C}_{p}(\tau) \tilde{D}_{q}(\tau)\right)\right] \end{array} $$

(81)

$$ \begin{array}{@{}rcl@{}} &=& \frac{1}{4N^{2}M^{2}} \left( \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N} \tilde{A}_{n}(\tau)\tilde{A}_{q}(\tau) \sum\limits_{m,p=1}^{M,M} \tilde{B}_{m}(\tau)\tilde{B}_{p}(\tau) \right] +2 \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N} \tilde{A}_{n}(\tau)\tilde{C}_{q}(\tau) \sum\limits_{m,p=1}^{M,M} \tilde{B}_{m}(\tau)\tilde{D}_{p}(\tau) \right]\right.\\ &&\left. +\mathbb{E}\left[\sum\limits_{n,q=1}^{N,N}\tilde{C}_{n}(\tau)\tilde{C}_{q}(\tau)\sum\limits_{m,p=1}^{M,M}\tilde{D}_{m}(\tau)\tilde{D}_{p}(\tau \right] \right) \end{array} $$

(82)

We have the following identities:

$$ \begin{array}{@{}rcl@{}} \mathbb{E}[\sum\limits_{n,q=1}^{N,N}\tilde{A}_{n}(\tau)\tilde{A}_{q}(\tau)] &=&N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau)+ \mathbb{E}\left[\sum\limits_{n=1}^{N} \tilde{A}_{n}(\tau) \tilde{A}_{n}(\tau)\right ]- \sum\limits_{n=1}^{N} \mathbb{E}[ \tilde{A}_{n}(\tau) ]\mathbb{E}[ \tilde{A}_{n}(\tau)] \end{array} $$

(83)

$$ \begin{array}{@{}rcl@{}} &=& N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau)+ \frac{N}{2}+\frac{N J_{0}(4 \pi f_{1} \tau)}{2}- {\xi(f_{1},\tau)} \end{array} $$

(84)

$$ \begin{array}{@{}rcl@{}} && \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N}\tilde{C}_{n}(\tau)\tilde{C}_{q}(\tau)\right] = \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N}\tilde{A}_{n}(\tau)\tilde{A}_{q}(\tau)\right] \end{array} $$

(85)

$$ \begin{array}{@{}rcl@{}} && \mathbb{E}\left[\sum\limits_{m,p=1}^{M,M}\tilde{B}_{m}(\tau)\tilde{B}_{p}(\tau) \right] = \mathbb{E}\left[\sum\limits_{m,p=1}^{M,M}\tilde{D}_{m}(\tau)\tilde{D}_{p}(\tau) \right] = M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau) + \frac{M}{2} + \frac{M J_{0}(4 \pi f_{2} \tau)}{2}- {\xi(f_{2},\tau)} \end{array} $$

(86)

$$ \begin{array}{@{}rcl@{}} && \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N}\tilde{A}_{n}(\tau)\tilde{C}_{q}(\tau) \right] = N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau)+V_{\tilde{A}\tilde{C}} \end{array} $$

(87)

$$ \begin{array}{@{}rcl@{}} && \mathbb{E}\left[\sum\limits_{m,p=1}^{M,M}\tilde{B}_{m}(\tau)\tilde{D}_{p}(\tau) \right] = M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau)+V_{\tilde{B}\tilde{D}} \end{array} $$

(88)

where $V_{\tilde {A}\tilde {C}} = {\sum }_{n=1}^{N} (\mathbb {E}[ \tilde {A}_{n}(\tau ) \tilde {C}_{n}(\tau )]-\mathbb {E}[ \tilde {A}_{n}(\tau )]\mathbb {E}[ \tilde {C}_{n}(\tau )] )$, $V_{\tilde {B}\tilde {D}}= {\sum }_{m=1}^{M} \left (\mathbb {E}[ \tilde {B}_{m}(\tau ) \tilde {D}_{m}(\tau )]-\mathbb {E}[ \tilde {B}_{m}(\tau )]\mathbb {E}[ \tilde {D}_{m}(\tau )] \right )$. Inserting Eq. 84 to Eq. 88 into Eq. 82, we obtain

$$ \begin{array}{@{}rcl@{}} \mathbb{E}[ \tilde{R}_{g_{c} g_{c}}^{2}(\tau)]&=& \frac{1}{4M^{2}N^{2} } \left( 2 \left( N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau)+ \frac{N}{2}+\frac{N J_{0}(4 \pi f_{1} \tau)}{2}- {\xi(f_{1},\tau)}\right) \right. \end{array} $$

(89)

$$ \begin{array}{@{}rcl@{}} &&\left. \times \left( M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau) + \frac{M}{2} + \frac{M J_{0}(4 \pi f_{2} \tau)}{2} - {\xi(f_{2},\tau)}\right) + 2 \left( N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau) + V_{\tilde{A}\tilde{C}}\right)\left( M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau) + V_{\tilde{B}\tilde{D}}\right) \right) \\ &=& \frac{1}{8M^{2}N^{2} } \left( \left( 2N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau) + N + {N J_{0}(4 \pi f_{1} \tau)} - 2~ {\xi(f_{1},\tau)}\right) \right.\\ &&\left. \times \left( 2M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau) + M + {M J_{0}(4 \pi f_{2} \tau)} - 2~{\xi(f_{2},\tau)}\right) + 4 \left( N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau) + V_{\tilde{A}\tilde{C}}\right)\left( M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau) + V_{\tilde{B}\tilde{D}}\right)\right)\\ \end{array} $$

(90)

Recall $\left (\tilde {R}_{g_{c} g_{c}} (\tau )\right )^{2}={J^{2}_{0}} (2\pi f_{1} \tau ){J^{2}_{0}} (2\pi f_{2} \tau )$. We have

$$ \begin{array}{@{}rcl@{}} \text{Var}[\tilde{R}_{g_{c} g_{c}}(\tau) ] &=& \frac{1}{8M^{2}N^{2} } \left( 2 M^{2} {J^{2}_{0}}(2 \pi f_{2} \tau) \left( N+{N J_{0}(4 \pi f_{1} \tau)}- 2~ {\xi(f_{1},\tau)}+2V_{\tilde{A}\tilde{C}} \right) \right. \end{array} $$

$$ \begin{array}{@{}rcl@{}} &&+2 N^{2} {J^{2}_{0}}(2 \pi f_{1} \tau)\left( M+{M J_{0}(4 \pi f_{2} \tau)}-2~ \xi(f_{2},\tau) +2V_{\tilde{B}\tilde{D}}\right)\\ &&\left.+\left( N + {N J_{0}(4 \pi f_{1} \tau)}-2~ \xi(f_{1},\tau) \right) \left( M + {M J_{0}(4 \pi f_{2} \tau)}-2~\xi(f_{2},\tau)\right) + 4V_{\tilde{A}\tilde{C}}V_{\tilde{B}\tilde{D}}\right) \end{array} $$

(91)

Reorganizing Eq. 91, one can obtain Eq. 34.

The variance of time-average autocorrelation of the imaginary part of g(t) is $\text {Var}[\tilde {R}_{g_{s} g_{s}}(\tau ) ] =\mathbb {E}[ \tilde {R}_{g_{s} g_{s}}^{2}(\tau )]-({R}_{g_{s} g_{s}}(\tau ))^{2}$. The first term is evaluated as

$$ \begin{array}{@{}rcl@{}} \mathbb{E}[ \tilde{R}_{g_{s} g_{s}}^{2}(\tau)]&=& \frac{1}{4M^{2}N^{2} } \mathbb{E}\left[\sum\limits_{n,m=1}^{N,M} \left( \tilde{A}_{n}(\tau) \tilde{D}_{m}(\tau) + \tilde{C}_{n}(\tau) \tilde{B}_{m}(\tau)\right) \sum\limits_{p,q=1}^{N,M} \left( \tilde{A}_{p}(\tau) \tilde{D}_{q}(\tau) + \tilde{C}_{p}(\tau) \tilde{B}_{q}(\tau)\right)\right]\\ &&= \frac{1}{4N^{2}M^{2}} \left( \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N} \tilde{A}_{n}(\tau)\tilde{A}_{q}(\tau) \sum\limits_{m,p=1}^{M,M} \tilde{D}_{m}(\tau)\tilde{D}_{p}(\tau) \right] +2 \mathbb{E}\left[\sum\limits_{n,q=1}^{N,N} \tilde{A}_{n}(\tau)\tilde{C}_{q}(\tau) \sum\limits_{m,p=1}^{M,M} \tilde{B}_{m}(\tau)\tilde{D}_{p}(\tau) \right]\right.\\ &&\left. +\mathbb{E}\left[\sum\limits_{n,q=1}^{N,N}\tilde{C}_{n}(\tau)\tilde{C}_{q}(\tau)\sum\limits_{m,p=1}^{M,M}\tilde{B}_{m}(\tau)\tilde{B}_{p}(\tau) \right]\right) \end{array} $$

(92)

We have $\text {Var}[\tilde {R}_{g_{s} g_{s}}(\tau ) ] =\text {Var}[\tilde {R}_{g_{c} g_{c}}(\tau ) ]$.