Generalized diversity combining of energy harvesting multiple antenna relay networks: outage and throughput performance analysis

Abstract

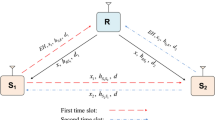
In this paper, the generalized diversity combining of an energy constrained multiple antenna decode-and-forward relay network is considered. Using power splitting and time switching architectures in consort with diversity combining at the relay, six protocols are proposed, i.e., power splitting with selection combining (PSSC), power splitting with maximum ratio combining (PSMRC), power splitting with generalized selection combining (PSGSC), time switching with selection combining (TSSC), time switching with maximum ratio combining (TSMRC), and time switching with generalized selection combining (TSGSC). The outage probability and throughput performance of each protocol is analyzed by first developing the closed form analytical expressions and then verifying these through the Monte Carlo simulation method. Simulation results show that system performance improves both with increasing the number of antennas and decreasing the distance between the source and relay. The TSSC/TSMRC/TSGSC protocols yield better outage performance whereas the PSSC/PSMRC/PSGSC protocols achieve relatively higher throughput performance. Finally, the effects of power splitting ratio, energy harvesting time ratio, energy conversion efficiency, sample down conversion noise, and the target signal-to-noise ratio on system performance are analyzed and presented.

Appendices

Appendix A: Proof of equation (12.3)

The first probability in (12.2), $\Pr \left [ {\upsilon \psi {g_{\mathrm {X}}} < {\psi _{t}}} \right ]$, is expressed under the CDF of RV g _X as

$$ \Pr \left[ {\upsilon \psi {g_{\mathrm{X}}} < {\psi_{t}}} \right] = {F_{{g_{\mathrm{X}}}}}\left( \varphi \right) $$

(A1)

where $\varphi \triangleq \frac {{{\psi _{t}}}}{{\upsilon \psi }}$.

The second probability in (12.2), $\Pr \left [ {\upsilon \psi {g_{\mathrm {X}}} \ge {\psi _{t}},\omega \psi {g_{\mathrm {X}}}{g_{2}} < {\psi _{t}}} \right ]$, is given by

$$ \begin{array}{l} \Pr \left[ {\upsilon \psi {g_{\mathrm{X}}} \ge {\psi_{t}},\omega \psi {g_{\mathrm{X}}}{g_{2}} < {\psi_{t}}} \right]\\ \overset{\left( {\text{{A2}}.1} \right)}{=} \int_{\varphi}^{\infty} {{f_{{g_{\mathrm{X}}}}}(x){F_{{g_{2}}}}\left( {\frac{{{\psi_{t}}}}{{\omega \psi x}}} \right)dx} \\ \overset{\left( {\text{{A2}}.2} \right)}{=} \int_{\varphi}^{\infty} {{f_{{g_{\mathrm{X}}}}}(x)\left( {1 - {e^{ - \frac{{{\lambda_{2}}{\psi_{t}}}}{{\omega \psi x}}}}} \right)dx} \\ \overset{\left( {\text{{A2}}.3} \right)}{=} 1 - {F_{{g_{\mathrm{X}}}}}\left( \varphi \right) - \int_{\varphi}^{\infty} {{f_{{g_{\mathrm{X}}}}}(x){e^{ - {\lambda_{2}}\theta /x}}dx} \end{array} $$

(A2)

where $\theta \triangleq \frac {{{\psi _{t}}}}{{\omega \psi }}$, ${f_{{g_{\mathrm {X}}}}}\left (x \right )$ is the PDF of RV g _X; (A2.2) is obtained by substituting the CDF ${F_{{g_{2}}}}\left ({\frac {{{\psi _{t}}}}{{\omega \psi x}}} \right ) = 1 - {e^{ - \frac {{{\lambda _{2}}{\psi _{t}}}}{{\omega \psi x}}}}$ into (A2.1); (A2.3) is obtained by substituting $\int _{\varphi }^{\infty } {{f_{{g_{\mathrm {X}}}}}(x)dx} = 1 - \int _{0}^{\varphi } {{f_{{g_{\mathrm {X}}}}}(x)dx} = 1 - {F_{{g_{\mathrm {X}}}}}\left (\varphi \right )$ into (A2.2).

Combining Eqs. A1 and A2, we finish the proof for equation (12.2).

Appendix B: Proof of $P_{out}^{PSSC}$

Firstly, the CDF of g _SC is given by

$$\begin{array}{@{}rcl@{}} {F_{{g_{SC}}}}(x) &=& \Pr \left[ {{g_{SC}} < x} \right] = \Pr \left[ {\max\limits_{i = 1,...,N} {g_{1i}} < x} \right]\notag\\ &=& \Pr \left[ {{g_{11}} < x,{g_{12}} < x,...,{g_{1N}} < x} \right]\notag\\ &=& {F_{{g_{11}}}}(x){F_{{g_{12}}}}(x)...{F_{{g_{1N}}}}(x)\notag\\ &=& {\left( {1 - {e^{ - {\lambda_{1}}x}}} \right)^{N}} \end{array} $$

(B1)

The PDF of g _SC can be obtained by differentiating (B1)

$$ {f_{{g_{SC}}}}(x) = \frac{{\partial {F_{{g_{SC}}}}(x)}}{{\partial x}} = N{\lambda_{1}}{e^{ - {\lambda_{1}}x}}{\left( {1 - {e^{ - {\lambda_{1}}x}}} \right)^{N - 1}} $$

(B2)

Secondly, from the binomial theorem ${\left ({u + v} \right )^{n}} = \sum \limits _{k = 0}^{n} {{C_{n}^{k}}{u^{n - k}}{v^{k}}}$ [27, pp. 10], where ${C_{n}^{k}} = \frac {{n!}}{{k!\left ({n - k} \right )!}}$. We obtain the following result:

$$ {\left( {1 - {e^{ - {\lambda_{1}}x}}} \right)^{N - 1}} = \sum\limits_{k = 0}^{N - 1} {C_{N - 1}^{k}{{\left( { - 1} \right)}^{k}}{e^{ - {\lambda_{1}}kx}}} $$

(B3)

Then, $P_{out}^{PSSC}$ can be rewritten by

$$\begin{array}{@{}rcl@{}} P_{out}^{PSSC} &=& 1 - {\lambda_{1}}N\sum\limits_{k = 0}^{N - 1} {C_{N - 1}^{k}{{\left( { - 1} \right)}^{k}}} \int_{\varphi}^{\infty} {{e^{ - {\lambda_{1}}\left( {k + 1} \right)x - {\lambda_{2}}\theta /x}}dx} \notag\\ &=& 1 - {\lambda_{1}}N\sum\limits_{k = 0}^{N - 1} {C_{N - 1}^{k}{{\left( { - 1} \right)}^{k}}}\notag\\ &&\times\left( \int_{0}^{\infty} {{e^{ - {\lambda_{1}}\left( {k + 1} \right)x - {\lambda_{2}}\theta /x}}dx} \right.\notag\\&&\qquad\left.- \int_{0}^{\varphi} {{e^{ - {\lambda_{1}}\left( {k + 1} \right)x - {\lambda_{2}}\theta /x}}dx} \right) \end{array} $$

(B4)

We note that $\theta = \frac {{{\lambda _{2}}{\psi _{t}}}}{{\omega \psi }} \approx 0$ and $\varphi = \frac {{{\psi _{t}}}}{{\upsilon \psi }} \approx 0$ when ψ is high. Thus, the integral $\int _{0}^{\varphi } {{e^{ - {\lambda _{1}}\left ({k + 1} \right )x - {\lambda _{2}}\theta /x}}dx}$ is insignificant due to $\frac {{{\psi _{t}}}}{{\upsilon \psi }} \approx 0$, and $\int _{0}^{\varphi } {{e^{ - {\lambda _{1}}\left ({k + 1} \right )x - {\lambda _{2}}\theta /x}}dx}$ can be approximated to $\int _{0}^{\varphi } {{e^{ - {\lambda _{1}}\left ({k + 1} \right )x}}dx}$. We obtain:

$$ \int_{0}^{\varphi} {{e^{ - {\lambda_{1}}\left( {k + 1} \right)x - {\lambda_{2}}\theta /x}}dx} \approx \int_{0}^{\varphi} {{e^{ - {\lambda_{1}}\left( {k + 1} \right)x}}dx} = \frac{{1 - {e^{ - {\lambda_{1}}\left( {k + 1} \right)\varphi }}}}{{{\lambda_{1}}\left( {k + 1} \right)}} $$

(B5)

Finally, $P_{out}^{PSSC}$ can be expressed by using the formula $\int _{0}^{\infty } {{e^{ - \frac {\beta }{{4x}} - \gamma x}}dx = \sqrt {\frac {\beta }{\gamma }} } {K_{1}}\left ({\sqrt {\beta \gamma } } \right )$ [26, Eq. 3.324.1], where ${K_{1}}\left (. \right )$ is the first-order modified Bessel function of the second kind

$$ \begin{array}{l} P_{out}^{PSSC} \approx 1 - {\lambda_{1}}N\sum\limits_{k = 0}^{N - 1} {C_{N - 1}^{k}{{\left( { - 1} \right)}^{k}}} \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\times \left[ {2\sqrt {\frac{\theta }{{{\lambda_{1}}\left( {k + 1} \right)}}} {K_{1}}\left( {2\sqrt {{\lambda_{1}}{\lambda_{2}}\theta \left( {k + 1} \right)} } \right) - \frac{{1 - {e^{ - {\lambda_{1}}\left( {k + 1} \right)\varphi }}}}{{{\lambda_{1}}\left( {k + 1} \right)}}} \right] \end{array} $$

(B6)

This finishes the proof of $P_{out}^{PSSC}$.

Appendix C: Proof of $P_{out}^{PSM\!RC}$

Firstly, because g _MRC is summation of i.i.d. exponential RVs, its PDF is given from [28, Eq. 12] as

$$ {f_{{g_{MRC}}}}(x) = \frac{{{\lambda_{1}^{N}}}}{{\left( {N - 1} \right)!}}{x^{N - 1}}{e^{ - {\lambda_{1}}x}} $$

(C1)

Then , we rewrite $P_{out}^{PSMRC}$ as

$$ \begin{array}{l} P_{out}^{PSMRC} = 1 - \frac{{{\lambda_{1}^{N}}}}{{\left( {N - 1} \right)!}}\int_{\varphi}^{\infty} {{x^{N - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} \\ = 1 - \frac{{{\lambda_{1}^{N}}}}{{\left( {N - 1} \right)!}}\left[ {\int_{0}^{\infty} {{x^{N - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} - \int_{0}^{\varphi} {{x^{N - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} } \right]\\ \approx 1 - \frac{{{\lambda_{1}^{N}}}}{{\left( {N - 1} \right)!}}\left[ {\int_{0}^{\infty} {{x^{N - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} - \int_{0}^{\varphi} {{x^{N - 1}}{e^{ - {\lambda_{1}}x}}dx} } \right] \end{array} $$

(C2)

Using the formula ${\int _{0}^{u}} {{x^{v - 1}}{e^{ - \mu x}}dx} = {\mu ^{ - v}}\gamma \left ({v,\mu u} \right )$ [26, Eq. 3.381.1] and $\int _{0}^{\infty } {{x^{v - 1}}{e^{ - \frac {\beta }{x} - \gamma x}}dx} = 2{\left ({\frac {\beta }{\gamma }} \right )^{v/2}}{K_{v}}\left ({2\sqrt {\beta \gamma } } \right )$ [26, Eq. 3.471.9], where K _v (.) is a modified Bessel function of the second kind, $\gamma \left ({v,\mu u} \right )$ is the incomplete gamma function and it is defined by $\gamma \left ({v,\mu u} \right ) \triangleq \int _{0}^{\mu u} {{e^{ - t}}{t^{v - 1}}dt} = \int _{0}^{\infty } {{e^{ - t}}{t^{v - 1}}dt} - \int _{\mu u}^{\infty } {{e^{ - t}}{t^{v - 1}}dt} = \Gamma \left (v \right ) - \Gamma \left ({v,\mu u} \right )$ [26, Eq. 8.350.1], with $\Gamma \left (v \right ) \triangleq \int _{0}^{\infty } {{e^{ - t}}{t^{v - 1}}dt}$ [26, Eq. 8.310.1], $\Gamma \left ({v,\mu u} \right ) \triangleq \int _{\mu u}^{\infty } {{e^{ - t}}{t^{v - 1}}dt}$ [26, Eq. 8.350.2]. We obtain the following results

$$ \int_{0}^{\varphi} {{x^{N - 1}}{e^{ - {\lambda_{1}}x}}dx} = {\left( {{\lambda_{1}}} \right)^{ - N}}\gamma \left( {N,{\lambda_{1}}\varphi } \right) $$

(C3a)

$$ \int_{0}^{\infty} {{x^{N - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} = 2{\left( {{\lambda_{2}}\theta /{\lambda_{1}}} \right)^{N/2}}{K_{N}}\left( {2\sqrt {{\lambda_{1}}{\lambda_{2}}\theta } } \right) $$

(C3b)

Substituting Eqs. C3a and C3b into Eq. C2, $P_{out}^{PSMRC}$ is given by

$$ P_{out}^{PSM\!RC} = 1 \!-\! \frac{{{\lambda_{1}^{N}}}}{{\left( {N\! - \!1} \right)!}}\left[ {2{{\left( {{\lambda_{2}}\theta /{\lambda_{1}}} \right)}^{N/2}}{K_{N}}\left( {2\sqrt {{\lambda_{1}}{\lambda_{2}}\theta } } \right)\! -\! {{\left( {{\lambda_{1}}} \right)}^{ - N}}\gamma \left( {N,{\lambda_{1}}\varphi } \right)} \right] $$

(C4)

This finishes the proof of $P_{out}^{PSMRC}$.

Appendix D: Proof of $P_{out}^{PSGSC}$

Firstly, the PDF of g _GSC is given from [29, Eq. 8] as

$$ {f_{GSC}}\left( x \right) = {C_{N}^{L}}\left\{ \begin{array}{l} \frac{{{\lambda_{1}}^{L}{x^{L - 1}}{e^{ - {\lambda_{1}}x}}}}{{\left( {L - 1} \right)!}} + {\lambda_{1}}\sum\limits_{l = 1}^{N - L} {{{\left( { - 1} \right)}^{L + l - 1}}C_{N - L}^{l}} \\ \times {\left( {\frac{L}{l}} \right)^{L - 1}}{e^{ - {\lambda_{1}}x}}\left[ {{e^{ - \frac{{l{\lambda_{1}}x}}{L}}} - \sum\limits_{m = 0}^{L - 2} {\frac{1}{{m!}}{{\left( { - \frac{{l{\lambda_{1}}x}}{L}} \right)}^{m}}} } \right] \end{array} \right\} $$

(D1)

Then, $P_{out}^{PSGSC}$ can be rewritten by

$$ \begin{array}{l} \!\!\!\!P_{out}^{PSMRC} \\ \!\!\!= 1 \!-\! {C_{N}^{L}}\int_{\frac{{{\psi_{t}}}}{{\upsilon \psi }}}^{\infty} {\left\{ \begin{array}{l} \frac{{{\lambda_{1}}^{L}{x^{L - 1}}{e^{ - {\lambda_{1}}x}}}}{{\left( {L - 1} \right)!}} + {\lambda_{1}}\sum\limits_{l = 1}^{N - L} {{{\left( { - 1} \right)}^{L + l - 1}}C_{N - L}^{l}} \\ \times {\left( {\frac{L}{l}} \right)^{L - 1}}{e^{ - {\lambda_{1}}x}}\left[ {{e^{ - \frac{{l{\lambda_{1}}x}}{L}}}\! -\! \sum\limits_{m = 0}^{L - 2} {\frac{1}{{m!}}{{\left( { - \frac{{l{\lambda_{1}}x}}{L}} \right)}^{m}}} } \right] \end{array} \right\}{e^{ - {\lambda_{2}}\theta /x}}dx} \\ \!\!\!= 1 \!-\! {C_{N}^{L}}\left\{ \begin{array}{l} \frac{{{\lambda_{1}}^{L}}}{{\left( {L - 1} \right)!}}{I_{1}}\\ + {\lambda_{1}}\sum\limits_{l = 1}^{N \!-\! L} {{{\left( { - 1} \right)}^{L + l - 1}}C_{N - L}^{l}{{\left( {\frac{L}{l}} \right)}^{L - 1}}\left[ {{I_{2}}\! - \!\sum\limits_{m = 0}^{L - 2} {\frac{1}{{m!}}{{\left( { - \frac{{l{\lambda_{1}}}}{L}} \right)}^{m}}{I_{3}}} } \right]} \end{array} \right\} \end{array} $$

(D2)

where ${I_{1}} \triangleq \int _{\varphi }^{\infty } {{x^{L - 1}}{e^{ - {\lambda _{1}}x - {\lambda _{2}}\theta /x}}dx}$, ${I_{2}} \triangleq \int _{\varphi }^{\infty } {{e^{ - \left ({{\lambda _{1}} + \frac {{l{\lambda _{1}}}}{L}} \right )x - {\lambda _{2}}\theta /x}}dx}$, and ${I_{3}} \triangleq \int _{\varphi }^{\infty } {{x^{m}}{e^{ - {\lambda _{1}}x - {\lambda _{2}}\theta /x}}dx}$.

The expression of integrals I ₁, I ₂, and I ₃ can be given by

$$ \begin{array}{l} {I_{1}}\overset{\left( {\text{{D3a}}\text{{.1}}} \right)}{=} \int_{\varphi}^{\infty} {{x^{L - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} \\ \overset{\left( {\text{{D3a}}\text{{.2}}} \right)}{\approx} \int_{0}^{\infty} {{x^{L - 1}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} - \int_{0}^{\varphi} {{x^{L - 1}}{e^{ - {\lambda_{1}}x}}dx} \\ \overset{\left( {\text{{D3a}}\text{{.3}}} \right)}{=} 2{\left( {{\lambda_{2}}\theta /{\lambda_{1}}} \right)^{L/2}}{K_{L}}\left( {2\sqrt {{\lambda_{1}}{\lambda_{2}}\theta } } \right) - {\left( {{\lambda_{1}}} \right)^{ - L}}\gamma \left( {L,{\lambda_{1}}\varphi } \right) \end{array} $$

(D3a)

$$ \begin{array}{l} {I_{2}}\overset{\left( {\text{{D3b}}\text{{.1}}} \right)}{=} \int_{\varphi}^{\infty} {{e^{ - \left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)x - {\lambda_{2}}\theta /x}}dx} \\ \overset{\left( {\text{{D3b}}\text{{.2}}} \right)}{\approx} \int_{0}^{\infty} {{e^{ - \left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)x - {\lambda_{2}}\theta /x}}dx} - \int_{0}^{\varphi} {{e^{ - \left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)x}}dx} \\ \overset{\left( {\text{{D3b}}\text{{.3}}} \right)}{=} 2\sqrt {{\lambda_{2}}\theta /\left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)} {K_{1}}\left( {2\sqrt {{\lambda_{2}}\theta \left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)} } \right) \\- \frac{{1 - {e^{ - \left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)\varphi }}}}{{\left( {{\lambda_{1}} + \frac{{l{\lambda_{1}}}}{L}} \right)}} \end{array} $$

(D3b)

$$ \begin{array}{l} {I_{3}}\overset{\left( {\text{{D3c}}\text{{.1}}} \right)}{=} \int_{\varphi}^{\infty} {{x^{m}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} \\ \overset{\left( {\text{{D3c}}\text{{.2}}} \right)}{\approx} \int_{0}^{\infty} {{x^{m}}{e^{ - {\lambda_{1}}x - {\lambda_{2}}\theta /x}}dx} - \int_{0}^{\varphi} {{x^{m}}{e^{ - {\lambda_{1}}x}}dx} \\ \overset{\left( {\text{{D3c}}\text{{.3}}} \right)}{=} 2{\left( {{\lambda_{2}}\theta /{\lambda_{1}}} \right)^{(m + 1)/2}}{K_{m + 1}}\left( {2\sqrt {{\lambda_{1}}{\lambda_{2}}\theta } } \right) \\- {\left( {{\lambda_{1}}} \right)^{ - (m + 1)}}\gamma \left( {m + 1,{\lambda_{1}}\varphi } \right) \end{array} $$

(D3c)

where (D3a.2), (D3b.2) and (D3c.2) are obtained by approximating $\int _{0}^{\varphi } {{x^{L - 1}}{e^{ - {\lambda _{1}}x - {\lambda _{2}}\theta /x}}dx} \approx \int _{0}^{\varphi } {{x^{L - 1}}{e^{ - {\lambda _{1}}x}}dx}$, $\int _{0}^{\varphi } {{e^{ - \left ({{\lambda _{1}} + \frac {{l{\lambda _{1}}}}{L}} \right )x - {\lambda _{2}}\theta /x}}dx} \approx \int _{0}^{\varphi } {{e^{ - \left ({{\lambda _{1}} + \frac {{l{\lambda _{1}}}}{L}} \right )x}}dx}$ and $\int _{0}^{\varphi } {{x^{m}}{e^{ - {\lambda _{1}}x - {\lambda _{2}}\theta /x}}dx} \approx \int _{0}^{\varphi } {{x^{m}}{e^{ - {\lambda _{1}}x}}dx}$, respectively. (D3a.3), (D3b.3) and (D3c.3) are attained by using ${\int _{0}^{u}} {{x^{v - 1}}{e^{ - \mu x}}dx} = {\mu ^{ - v}}\gamma \left ({v,\mu u} \right )$ and $\int _{0}^{\infty } {{x^{v - 1}}{e^{ - \frac {\beta }{x} - \gamma x}}dx} = 2{\left ({\frac {\beta }{\gamma }} \right )^{v/2}}{K_{v}}\left ({2\sqrt {\beta \gamma } } \right )$. Substituting Eqs. D3a, D3b, and D3c into Eq. D2, the proof is concluded.