Analyzing a manufacturer-retailer sustainable supply chain under cap-and-trade policy and revenue sharing contract

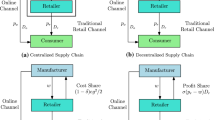
Abstract

Due to growing public awareness about environment-friendly (green) products, green improvement has become an important factor in supply chain management. This paper deals with a two-echelon sustainable supply chain where both the manufacturer and the retailer are environmentally conscious. Market demand is assumed to be dependent on the selling price and green activities of both the channel members, while the carbon emissions are affected by the greening level of the product. In a make-to-order setting, this paper develops four models, viz. centralized, decentralized, retailer-led revenue sharing and bargaining revenue sharing under the cap-and-trade policy, and compares the optimal outcomes analytically. Numerical examples are taken to investigate the influence of some key model-parameters on optimal decisions. Our results demonstrate that besides improving the greening level of the product, the retailer-led revenue sharing can achieve a win-win situation for both the manufacturer and the retailer. Although the bargaining revenue sharing results in lower profit for the retailer, through promoting the greening level of the product effectively and diminishing the selling price it appears favorable for consumers, the manufacturer and the entire supply chain. Sensitivity analysis illustrates that a higher value of carbon trading cost encourages the manufacturer in improving the greening level and so, reducing the carbon emissions.

Appendix

The profit function for the manufacturer is

$$\begin{aligned} \Pi _m(w,\theta _m)= & {} (w - c) D - c_e [(a - b \theta _m)D - E] - \frac{1}{2}\lambda _1 \theta _m^2. \end{aligned}$$

The profit function for the retailer is

$$\begin{aligned} \Pi _r(p,\theta _r)= & {} (p - w) D - \frac{1}{2}\lambda _2 \theta _r^2. \end{aligned}$$

The profit function of the entire supply chain is

$$\begin{aligned} \Pi (p,\theta _m,\theta _r)= & {} (p - c) D - c_e [(a - b \theta _m)D - E] - \frac{1}{2}\lambda _1 \theta _m^2 - \frac{1}{2}\lambda _2 \theta _r^2. \end{aligned}$$

Proof of Proposition 1

Now,

$$\frac{\partial \Pi ^{C}}{\partial p} = D_0 + \alpha (c + a c_e) - 2 \alpha p + (\beta - \alpha c_e b) \theta _m + \gamma \theta _r$$

,

$$\frac{\partial ^2\Pi ^{C}}{\partial p^2} = - 2 \alpha < 0$$

,

$$\frac{\partial \Pi ^{C}}{\partial \theta _m} = D_0 c_e b - \beta (c + a c_e) (\beta - \alpha c_e b)p + (2 \beta c_e b - \lambda _1) \theta _m + \gamma c_e b \theta _r$$

,

$$\frac{{\partial ^{2} \Pi ^{C} }}{{\partial \theta _{m}^{2} }} = - \lambda _{1} + 2\beta c_{e} b{{ < }}0,\;{\text{as}}\;\lambda _{1}{ > }\frac{{(\beta + \alpha c_{e} b)^{2} }}{{2\alpha }}{ > }2\beta c_{e} b$$

,

$$\frac{\partial \Pi ^{C}}{\partial \theta _r} =\gamma p + \gamma c_e b \theta _m - \lambda _2 \theta _r - \gamma (c + a c_e)$$

,

$$\frac{\partial ^2\Pi ^{C}}{\partial \theta _r^2} = - \lambda _2 < 0$$

,

$$\frac{\partial ^2\Pi ^{C}}{\partial p \partial \theta _m} = \beta - \alpha c_e b,\, \frac{\partial ^2\Pi ^{C}}{\partial p \partial \theta _r} = \gamma ,\, \frac{\partial ^2\Pi ^{C}}{\partial \theta _m\partial \theta _r} = \gamma c_e b$$

.

The corresponding Hessian matrix is given by

$$H=\left( \begin{array}{ccc} \frac{\partial ^2\Pi ^{C}}{\partial p^2} &{} \frac{\partial ^2\Pi ^{C}}{\partial p \partial \theta _m} &{} \frac{\partial ^2\Pi ^{C}}{\partial p \partial \theta _r}\\ \frac{\partial ^2\Pi ^{C}}{\partial \theta _m\partial p} &{} \frac{\partial ^2\Pi ^{C}}{\partial \theta ^2_m} &{} \frac{\partial ^2\Pi ^{C}}{\partial \theta _m\partial \theta _r}\\ \frac{\partial ^2\Pi ^{C}}{\partial \theta _r\partial p} &{} \frac{\partial ^2\Pi ^{C}}{\partial \theta _r\partial \theta _m} &{} \frac{\partial ^2\Pi ^{C}}{\partial \theta _r^2} \end{array} \right)$$

=

$$\left( \begin{array}{ccccc} - 2 \alpha &{} \beta - \alpha c_e b &{} \gamma \\ \beta - \alpha c_e b &{} - \lambda _1 + 2 \beta c_e b &{} \gamma c_e b\\ \gamma &{} \gamma c_e b &{} - \lambda _2 \end{array} \right)$$

Now, the leading principle minors are $M_1 = - 2 \alpha < 0$, $M_2 = 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2 > 0$, and

$$|H| = - [\lambda _1 (2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2] < 0$$

. Thus the Hessian matrix is negative definite. Using the first order conditions for optimality i.e.

$$\frac{\partial \Pi ^{C}}{\partial p}=0, \frac{\partial \Pi ^{C}}{\partial \theta _m}=0,$$

and $\frac{\partial \Pi ^{C}}{\partial \theta _r}=0$, the optimal decision variables can be obtained as given in Proposition 1. $\square$

Proof of Proposition 2

$\frac{\partial \Pi _r^D}{\partial p} = D_0 + \alpha w - 2 \alpha p + \beta \theta _m + \gamma \theta _r$,

$\frac{\partial ^2\Pi _r^{D}}{\partial p^2} = - 2 \alpha < 0$,

$\frac{\partial \Pi _r^{D}}{\partial \theta _r} = \gamma p - \lambda _2 \theta _r - \gamma w$,

$\frac{\partial ^2\Pi _r^{D}}{\partial \theta _r^2} = - \lambda _2 < 0$,   $\frac{\partial ^2\Pi _r^{D}}{\partial p \partial \theta _r} = \gamma$,

The corresponding Hessian matrix is given by

$H=\left( \begin{array}{ccc} \frac{\partial ^2\Pi _r^{D}}{\partial p^2} &{} \frac{\partial ^2\Pi _r^{D}}{\partial p \partial \theta _r}\\ \frac{\partial ^2\Pi _r^{D}}{\partial \theta _r\partial p} &{} \frac{\partial ^2\Pi _r^{D}}{\partial \theta ^2_r} \end{array} \right)$=$\left( \begin{array}{ccccc} - 2 \alpha &{} \gamma \\ \gamma &{} - \lambda _2 \end{array} \right)$

$|H| = 2 \alpha \lambda _2 - \gamma ^2 > 0$. It is clear that the Hessian matrix corresponding to the retailer’s profit function is negative definite, and so $\Pi _r^D$ is jointly concave in p and $\theta _r$. Solving equations $\frac{\partial \Pi _r^D}{\partial p}=0$, and $\frac{\partial \Pi _r^D}{\partial \theta _r}=0$ simultaneously, we get

$$\begin{aligned} p= & {} \frac{\lambda _2 (D_0 + \alpha w + \beta \theta _m) - \gamma ^2 w}{2 \alpha \lambda _2 - \gamma ^2},\,\, \theta _r = \frac{\gamma (D_0 - \alpha w + \beta \theta _m)}{2 \alpha \lambda _2 - \gamma ^2} \end{aligned}$$

Substituting these values in the manufacturer’s profit function, we get the profit function of the manufacturer as follows:

$$\begin{aligned} \Pi _m^D(w,\theta _m)= & {} \frac{\alpha \lambda _2 (D_0 - \alpha w + \beta \theta _m)[w - c - c_e (a - b \theta _m)]}{2 \alpha \lambda _2 - \gamma ^2}+ c_e E - \frac{1}{2}\lambda _1 \theta _m^2. \end{aligned}$$

Now,

$\frac{\partial \Pi _m^{D}}{\partial w} = \frac{\alpha \lambda _2 [D_0 + \alpha (c + a c_e) - 2 \alpha w + (\beta - \alpha c_e b) \theta _m]}{2 \alpha \lambda _2 - \gamma ^2}$,

$\frac{\partial ^2\Pi _m^{D}}{\partial w^2} = - \frac{2 \alpha ^2 \lambda _2}{2 \alpha \lambda _2 - \gamma ^2} < 0$,

$\frac{\partial \Pi _m^{D}}{\partial \theta _m} = \frac{\alpha \lambda _2 [D_0 b c_e - \beta (c + a c_e) + w (\beta - \alpha c_e b)] - \lambda _1 (2 \lambda _2 - \gamma ^2) \theta _m}{2 \alpha \lambda _2 - \gamma ^2}$,

$\frac{\partial ^2\Pi _m^{D}}{\partial \theta _m^2} = - \lambda _1 + \frac{2 \alpha \beta \lambda _2 c_e b}{2 \alpha \lambda _2 - \gamma ^2} < 0$,   $\frac{\partial ^2\Pi _m^{D}}{\partial w\partial \theta _m} = \frac{\alpha \lambda _2 (\beta - \alpha c_e b)}{2 \alpha \lambda _2 - \gamma ^2}$.

The Hessian matrix corresponding to the manufacturer’s profit function is given by

$H=\left( \begin{array}{ccc} \frac{\partial ^2\Pi _m^{D}}{\partial w^2} &{} \frac{\partial ^2\Pi _m^{D}}{\partial w\partial \theta _m}\\ \frac{\partial ^2\Pi _m^{D}}{\partial \theta _m\partial w} &{} \frac{\partial ^2\Pi _m^{D}}{\partial \theta _m^2} \end{array} \right)$=$\left( \begin{array}{ccccc} - \frac{2 \alpha ^2 \lambda _2}{2 \alpha \lambda _2 - \gamma ^2} &{} \frac{\alpha \lambda _2 (\beta - \alpha c_e b)}{2 \alpha \lambda _2 - \gamma ^2}\\ \frac{\alpha \lambda _2 (\beta - \alpha c_e b)}{2 \alpha \lambda _2 - \gamma ^2} &{} - \lambda _1 + \frac{2 \alpha \beta \lambda _2 c_e b}{2 \alpha \lambda _2 - \gamma ^2} \end{array} \right)$

Now, $|H| = \frac{\alpha ^2 \lambda _2 [2 \lambda _1 (2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]}{(2 \alpha \lambda _2 - \gamma ^2)^2} > 0$, since $2 \lambda _1 (2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2 = [\lambda _1 (\alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2] + [\lambda _1 (\alpha \lambda _2 - \gamma ^2)] + 2 \alpha \lambda _1 \lambda _2 > 0$. Therefore, the Hessian matrix corresponding to the manufacturer’s profit function is jointly concave in w and $\theta _m$. Using the first order conditions for optimality i.e. $\frac{\partial \Pi _m^{D}}{\partial w}=0,$ and $\frac{\partial \Pi _m^{D}}{\partial \theta _m}=0$, we get the optimal decisions of the manufacturer, and substituting these values, we get the optimal decisions of the retailer as given in Proposition 2. $\square$

Proof of Proposition 3

$$p^{D} - p^{C} = \frac{{\lambda _{1} (2\alpha \lambda _{2} - \gamma ^{2} )[D_{0} - \alpha (c + ac_{e} )][\lambda _{1} (\alpha \lambda _{2} - \gamma ^{2} ) - \lambda _{2} \beta (\beta + \alpha c_{e} b)]}}{{[\lambda _{1} (2\alpha \lambda _{2} - \gamma ^{2} ) - \lambda _{2} (\beta + \alpha c_{e} b)^{2} ][2\lambda _{1} (2\alpha \lambda _{2} - \gamma ^{2} ) - \lambda _{2} (\beta + \alpha c_{e} b)^{2} ]}}{{ > }}0\;{\text{as}},\;(\beta + \alpha c_{e} b)^{2} {{ > }}\beta (\beta + \alpha c_{e} b)$$

.

$\theta _m^C - \theta _m^D = \frac{\lambda _1 \lambda _2 (2 \alpha \lambda _2 - \gamma ^2)[D_0 - \alpha (c + a c_e)]}{[\lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]} > 0$

$\theta _r^C - \theta _r^D = \frac{\gamma \lambda _1^2 (2 \alpha \lambda _2 - \gamma ^2)[D_0 - \alpha (c + a c_e)]}{[\lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]} > 0$

$D^C - D^D = \frac{\alpha \lambda _1^2 \lambda _2 (2 \alpha \lambda _2 - \gamma ^2)[D_0 - \alpha (c + a c_e)]}{[\lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]} > 0$.

$\Pi ^C - \Pi ^D = \frac{\lambda _1^3 \lambda _2 (2 \alpha \lambda _2 - \gamma ^2)^2[D_0 - \alpha (c + a c_e)]^2}{2 [\lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]^2} > 0$. $\square$

Proof of Proposition 4

First part of Proposition 4 is similar to that of Proposition 2. First, we solve the first order conditions for optimality of the retailer’s profit function (4) to obtain the values of p and $\theta _r$ in terms of $w,\, \theta _m$ and $\phi$, and then putting these values in the manufacturer’s profit function (3) and solving the first order conditions for optimality of this profit function, we get the values of w and $\theta _m$ in terms of $\phi$. Substituting all these values in the retailer’s profit function, we get

$\Pi _r^R = \frac{\lambda _1^2 \lambda _2 \phi [D_0 - \alpha (c + a c_e)]^2 (2 \alpha \lambda _2 - \gamma ^2 \phi )}{2 [\lambda _2\left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) + 2 \lambda _1 (\alpha \lambda _2 - \gamma ^2)\phi ]^2}.$

Now,

$\frac{\partial \Pi _r^{R}}{\partial \phi } = \frac{\lambda _1^2 \lambda _2^2 [D_0 + \alpha (c + a c_e)]^2 [\phi \left( 2 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2 (\beta + \alpha c_e b)^2\right) - \alpha \lambda _2 \left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) ]}{2 [\lambda _2\left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) + 2 \lambda _1 (\alpha \lambda _2 - \gamma ^2)\phi ]^3}$.

So, from $\frac{\partial \Pi _r^{R}}{\partial \phi } = 0$, we get

$\phi ^R = \phi = \frac{\alpha \lambda _2 [2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2]}{2 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2 (\beta + \alpha c_e b)^2}$. [$\frac{\partial ^2\Pi _r^{R}}{\partial \phi ^2}]_{\phi = \phi ^R} = - \frac{\lambda _1^2 [D_0 - \alpha (C + a c_e)]^2 [2 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2 (\beta + \alpha c_e b)^2]^4}{\lambda _2[2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2]^3 [4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2 \left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]^3}$. Therefore, $\phi ^R$ maximizes the retailer’s profit. Using this value of $\phi ^R$, we can get the optimal decisions of the manufacturer and the retailer as given in Proposition 4. $\square$

Proof of Proposition 5

$\theta _m^C - \theta _m^R = \frac{(\beta + \alpha c_e b)[D_0 - \alpha (c + a c_e)][(\beta + \alpha c_e b)^2\gamma ^4 + 2 \alpha \lambda _2 \gamma ^2\left( \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) + 2 \alpha ^2 \lambda _2^2 \left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) ]}{[\lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$\theta _m^R - \theta _m^D = \frac{2 \lambda _1 (\alpha \lambda _2 - \gamma ^2)^2(\beta + \alpha c_e b)^3[D_0 - \alpha (c + a c_e)]}{[2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$\theta _r^D - \theta _r^R = \frac{\gamma \lambda _1 (\alpha \lambda _2 - \gamma ^2)(\beta + \alpha c_e b)^2[D_0 - \alpha (c + a c_e)]}{[2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$p^D - p^R = \frac{\lambda _1 (\alpha \lambda _2 - \gamma ^2)(\beta + \alpha c_e b)^2[D_0 - \alpha (c + a c_e)][2 \alpha \lambda _2 \left( \alpha \lambda _1 - \beta (\beta + \alpha c_e b)\right) - \gamma ^2(\beta ^2 - \alpha ^2 c_e^2 b^2)]}{\alpha [2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$p^R - p^C = \frac{\lambda _1[D_0 - \alpha (c + a c_e)]}{[2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [ \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} \times \left[ 2 \alpha \lambda _2 \left( \alpha \lambda _1 - \beta (\beta + \alpha c_e b)\right) \left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) + \gamma ^4\left( 2 \alpha \lambda _1^2 - c_e b (\beta + \alpha c_e b)^3\right) - \gamma ^2 \lambda _2 \left( 6 \alpha ^2 \lambda _1^2 - 2 \alpha \lambda _1 (\beta + \alpha c_e b)(2 \beta + \alpha c_e b) + (\beta + \alpha c_e b)^2 (\beta ^2 - \alpha ^2 c_e^2 b^2)\right) \right] > 0$

$D^C - D^R = \frac{\alpha \lambda _1^2 [D_0 - \alpha (c + a c_e)][2 \alpha ^2 \lambda _2^2 \left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) - 2 \alpha \lambda _2 \gamma ^2 \left( \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) - \gamma ^4(\beta + \alpha c_e b)^2]}{[2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [ \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$D^R - D^D = \frac{2 \alpha \lambda _1^2 (\alpha \lambda _2 - \gamma ^2)^2 (\beta + \alpha c_e b)^2 [D_0 - \alpha (c + a c_e)]}{[2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$ $\square$

Proof of Proposition 6

$\Pi _m^R - \Pi _m^D = \frac{ \lambda _1^2 (\alpha \lambda _2 - \gamma ^2)^2 (\beta + \alpha c_e b)^2 [D_0 - \alpha (c + a c_e)]^2}{[2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2][4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$\Pi _r^R - \Pi _r^D = \frac{ \lambda _1^2 \lambda _2 (\alpha \lambda _2 - \gamma ^2)^2 (\beta + \alpha c_e b)^4 [D_0 - \alpha (c + a c_e)]^2}{2 [2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]^2 [4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$\Pi ^R - \Pi ^D = \frac{ \lambda _1^2 (\alpha \lambda _2 - \gamma ^2)^2 (\beta + \alpha c_e b)^2 [D_0 - \alpha (c + a c_e)]^2[4 \lambda _1 (2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]}{2 [2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [2 \lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2]^2 [4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$

$\Pi ^C - \Pi ^R = \frac{ \lambda _1^2 [D_0 - \alpha (c + a c_e)]^2[\alpha ^2 \lambda _2^2 \left( 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2\right) - \alpha \lambda _2 \gamma ^2 \left( \alpha \lambda _1 - 2 (\beta + \alpha c_e b)^2\right) - \gamma ^4 (\beta + \alpha c_e b)^2]}{2 [2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2] [\lambda _1(2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2] [4 \alpha ^2 \lambda _1 \lambda _2 - \gamma ^2\left( 2 \alpha \lambda _1 + (\beta + \alpha c_e b)^2\right) ]} > 0$ $\square$

Proof for the thresholds in Assumption 4

If we look at the proof of Proposition 1, we can observe that the negative definiteness of the Hessian matrix needs $(i)\,\, 2 \alpha \lambda _1 - (\beta + \alpha c_e b)^2 > 0$, and $(ii)\,\,\lambda _1 (2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2 > 0$. If we choose $\lambda _1$ in such a way that $\lambda _1 > \frac{(\beta + \alpha c_e b)^2}{\alpha }$, then condition (i) will be easily satisfied. In this regard, it can be mentioned that $\lambda _1 > \frac{(\beta + \alpha c_e b)^2}{2 \alpha }$ is sufficient for condition (i). But a lower threshold of $\lambda _1$ may give higher greening level of the product ($\theta _m$) and this may cause a negative unit emission ($a - b \theta _m$) which is absurd. That’s why, we consider a higher threshold for $\lambda _1$. As $\lambda _1 (2 \alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2 = \lambda _2 [\alpha \lambda _1 - (\beta + \alpha c_e b)^2] + \lambda _1 [\alpha \lambda _2 - \gamma ^2]$, for the existence of condition (ii) we choose $\lambda _2 > \frac{\gamma ^2}{\alpha }$. Also, the condition $0< \phi ^R < 1$ demands $\alpha \lambda _2 > \gamma ^2.$

Again, positivity of the optimal selling price of the centralized model demands ($i)\,\, \lambda _1 - c_e b (\beta + \alpha c_e b) > 0$ and ($ii)\,\,\lambda _1 (\alpha \lambda _2 - \gamma ^2) - \lambda _2 \beta (\beta + \alpha c_e b) > 0$. As $(\beta + \alpha c_e b)^2 = (\beta + \alpha c_e b)(\beta + \alpha c_e b) > \alpha c_e b(\beta + \alpha c_e b)$, here condition (i) will be easily satisfied for the previously chosen threshold value of $\lambda _1$. Now, as $(\beta + \alpha c_e b)^2 = (\beta + \alpha c_e b)(\beta + \alpha c_e b) > \beta (\beta + \alpha c_e b)$, if we choose $\lambda _1 (\alpha \lambda _2 - \gamma ^2) - \lambda _2 (\beta + \alpha c_e b)^2 > 0$, then condition (ii) will also be satisfied. $\square$