Hostname: page-component-76fb5796d-skm99 Total loading time: 0 Render date: 2024-04-28T03:35:48.934Z Has data issue: false hasContentIssue false
  • English
  • Français

CONSERVATIVITY OF ULTRAFILTERS OVER SUBSYSTEMS OF SECOND ORDER ARITHMETIC

Published online by Cambridge University Press:  01 August 2018

ANTONIO MONTALBÁN  and
RICHARD A. SHORE
ANTONIO MONTALBÁN
Affiliation:
DEPARTMENT OF MATHEMATICS UNIVERSITY OF CALIFORNIA, BERKELEY BERKELEY, CA 94720, USAE-mail: antonio@math.berkeley.edu
RICHARD A. SHORE
Affiliation:
DEPARTMENT OF MATHEMATICS CORNELL UNIVERSITY ITHACA, NY 14853, USAE-mail:shore@math.cornell.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We extend the usual language of second order arithmetic to one in which we can discuss an ultrafilter over of the sets of a given model. The semantics are based on fixing a subclass of the sets in a structure for the basic language that corresponds to the intended ultrafilter. In this language we state axioms that express the notion that the subclass is an ultrafilter and additional ones that say it is idempotent or Ramsey. The axioms for idempotent ultrafilters prove, for example, Hindman’s theorem and its generalizations such as the Galvin--Glazer theorem and iterated versions of these theorems (IHT and IGG). 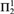We prove that adding these axioms to IHT produce conservative extensions of ACA0 +IHT, ${\rm{ACA}}_{\rm{0}}^ +$, ATR0, ${\rm{\Pi }}_2^1$-CA0, and 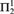${\rm{\Pi }}_2^1$-CA0 for all sentences of second order arithmetic and for full Z2 for the class of 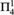${\rm{\Pi }}_4^1$ sentences. We also generalize and strengthen a metamathematical result of Wang (1984) to show, for example, that any 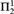${\rm{\Pi }}_2^1$ theorem ∀X∃YΘ(X,Y) provable in ACA0 or 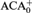${\rm{ACA}}_{\rm{0}}^ +$ there are e,k ∈ ℕ such that ACA0 or 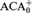${\rm{ACA}}_{\rm{0}}^ +$ proves that ∀X(Θ(X, Φe(J(k)(X))) where Φe is the eth Turing reduction and J(k) is the kth iterate of the Turing or Arithmetic jump, respectively. 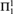(A similar result is derived for ${\rm{\Pi }}_3^1$ theorems of ${\rm{\Pi }}_1^1$-CA0 and the hyperjump.)

Keywords

03B3003D8003F2503F35reverse mathematicsHindman’s Theoremidempotent ultrafiltersconservation theorems
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 2 , June 2018 , pp. 740 - 765
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Avigad, J., An effective proof that open sets are Ramsey. Archive for Mathematical Logic, vol. 37 (1998), pp. 235–240.CrossRefGoogle Scholar
Blass, A., Hirst, J. L., and Simpson, S. G., Logical analysis of some theorems of combinatorics and topological dynamics, Logic and Combinatorics (Arcata, California, 1985) (Simpson, S. G., editor), Contemporary Mathematics, vol. 65, American Mathematical Society, Providence, RI, 1987, pp. 125–156.CrossRefGoogle Scholar
Bergelson, V. and Hindman, N., Additive and multiplicative Ramsey theorems in N–some elementary results. Combinatorics, Probability and Computing, vol. 2 (1993), pp. 221–241.CrossRefGoogle Scholar
Bergelson, V. and Hindman, N., Ultrafilters and multidimensional Ramsey theorems. Combinatorica, vol. 9 (1989), pp. 1–7.CrossRefGoogle Scholar
Enayat, A., From bounded arithmetic to second order arithmetic via automorphisms, Logic in Tehran (Enayat, A., Kalantari, I., and Moniri, M., editors), Lecture Notes in Logic, vol. 26, Association for Symbolic Logic, La Jolla, CA, 2006, 87–113.Google Scholar
Hindman, N., The existence of certain ultrafilters on ℕ and a conjecture of Graham and Rothschild. Proceedings of the American Mathematical Society, vol. 36 (1972), pp. 341–346.Google Scholar
Hindman, N., Algebra in the Stone-Čech compactification and its applications to Ramsey theory. Scientiae Mathematicae Japonicae, vol. 62 (2005), pp. 321–329.Google Scholar
Hindman, N. and Strauss, D., Algebra in the Stone-Čech Compactification, Theory and Applications, second ed., De Gruyter, Berlin, Boston, 2012.Google Scholar
Hirschfeldt, D., Slicing the Truth: On the Computable and Reverse Mathematics of Combinatorial Principles (Chong, C., Feng, Q., Slaman, T. A., Woodin, W. H., and Yang, Y., editors), World Scientific, Singapore, 2014.CrossRefGoogle Scholar
Hirst, J. L., Hindman’s theorem, ultrafilters, and reverse mathematics, this Journal, vol. 69 (2004), pp. 65–72.Google Scholar
Kirby, L. A. S., Ultrafilters and types on models of arithmetic. Annals of Pure and Applied Mathematics, vol. 27 (1984), pp. 215–252.Google Scholar
Kreuzer, A., Non-principal ultrafilters, program extractions and higher order reverse mathematics. Journal of Mathematical Logic, vol. 12 (2012), 1250002 (16 pp.).CrossRefGoogle Scholar
Kreuzer, A., On idempotent ultrafilters in higher-order reverse mathematics, this Journal, vol. 80 (2015a), pp. 179–193.Google Scholar
Kreuzer, A., Minimal idempotent ultrafilters and the Auslander-Ellis theorem, preprint, 2015b, arXiv:1305.6530.Google Scholar
McAloon, K., Paris-Harrington incompleteness and progressions of theories, Recursion Theory (Ithaca, N.Y., 1982) (Nerode, A. and Shore, R. A., editors), Proceedings of Symposia in Pure Mathematics, vol. 42, American Mathematical Society, Providence, RI, 1985, pp. 447–460.CrossRefGoogle Scholar
Simpson, S. G., Subsystems of Second Order Arithmetic, second ed., Perspectives in Logic, Association for Symbolic Logic and Cambridge University Press, New York, 2009.CrossRefGoogle Scholar
Todorcevic, S., Introduction to Ramsey Spaces, Annals of Mathematical Studies, vol. 174, Princeton University Press, Princeton, NJ, 2010.Google Scholar
Towsner, H., A combinatorial proof of the dense Hindman’s theorem. Discrete Mathematics vol. 311 (2011a), pp. 1380–1384.CrossRefGoogle Scholar
Towsner, H., Hindman’s theorem: An ultrafilter argument in second order arithmetic, this Journal, vol. 76 (2011b), pp. 353–360.Google Scholar
Towsner, H., Ultrafilters in reverse mathematics. Journal of Mathematical Logic, vol. 14 (2014), 145001 (11 pp.).CrossRefGoogle Scholar
Wang, H., Popular Lectures on Mathematical Logic, Revised reprint of the 1981 second ed., Dover Publications, Inc., New York, 1993.Google Scholar