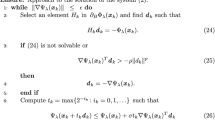Abstract
We investigate the theoretical and numerical properties of two global optimization techniques for the solution of mixed complementarity problems. More precisely, using a standard semismooth Newton-type method as a basic solver for complementarity problems, we describe how the performance of this method can be improved by incorporating two well-known global optimization algorithms, namely a tunneling and a filled function method. These methods are tested and compared with each other on a couple of very difficult test examples.
Similar content being viewed by others
References
Billups, S.C. (1995), Algorithms for complementarity problems and generalized equations. Ph.D. Thesis, Computer Sciences Department, University of Wisconsin-Madison, Madison, WI.
Billups, S.C. (1995), Improving the robustness of complementarity solvers using proximal perturbations. Technical Report, Department of Mathematics, University of Colorado, Denver, CO.
Billups, S.C. (1998), A homotopy based algorithm for mixed complementarity problems. Technical Report, Department of Mathematics, University of Colorado, Denver, CO.
Billups, S.C. and Ferris, M.C. (1997), QPCOMP: A quadratic program based solver for mixed complementarity problems. Mathematical Programming 76, 533–562.
Brooke, A., Kendrick, D. and Meeraus, A. (1998), GAMS: A User's Guide. The Scientific Press, South San Francisco, CA.
Chen, B., Chen, X. and Kanzow, C. (1997), A penalized Fischer-Burmeister NCP-function: Theoretical investigation and numerical results. Preprint 126, Institute of Applied Mathematics, University of Hamburg, Hamburg, Germany.
De Luca, T., Facchinei, F. and Kanzow, C. (1996), A semismooth equation approach to the solution of nonlinear complementarity problems. Mathematical Programming 75, 407–439.
Dirkse, S.P. and Ferris, M.C. (1995), MCPLIB: A collection of nonlinear mixed complementarity problems. Optimization Methods and Software 5, 319–345.
Facchinei, F. and Soares, J. (1997), A new merit function for nonlinear complementarity problems and a related algorithm. SIAM Journal on Optimization 7, 225–247.
Ferris, M.C., Kanzow, C. and Munson, T.S. Feasible descent algorithms for mixed complementarity problems. Mathematical Programming, to appear.
Ferris, M.C. and Munson, T.S. (1999), Interfaces to PATH 3.0: Design, implementation and usage. Computational Optimization and Applications 12, 207–227.
Ferris, M.C. and Pang, J.-S. (1997), Engineering and economic applications of complementarity problems. SIAM Review 39, 669–713.
Fischer, A. A special Newton-type optimization method. Optimization 24, 269–284.
Fletcher, R. (1987), Practical Methods of Optimization. John Wiley & Sons, New York, NY.
Ge, R. (1990), A filled function method for finding a global minimizer of a function of several variables. Mathematical Programming 46, 191–204.
Geiger, C. and Kanzow, C. (1996), On the resolution of monotone complementarity problems. Computational Optimization and Applications 5, 155–173.
Gómez, S. and Barrón, C. (1991), The exponential tunneling method. Technical Report, Instituto de Investigaciones en Matematicas Aplicadas y en Sistemas, Universidad Nacional Autonoma de Mexico, Mexico.
Horst, R. and Pardalos, P.M. (eds.) (1995), Handbook of Global Optimization. Kluwer Academic Publishers, 1995.
Kostreva, M.M. and Zheng, Q. (1994), Integral global optimization method for solution of nonlinear complementarity problems. Journal of Global Optimization 5, 181–193.
Levy, A.V. and Gómez, S. (1984), The Tunneling Method Applied to Global Optimization. In: P.T. Boggs, R.H. Byrd and R.B. Schnabel (eds.), Numerical Optimization. SIAM, Philadelphia, PA.
Levy, A.V. and Montalvo, A. (1985), The tunneling algorithm for the global minimization of functions. SIAM Journal on Scientific and Statistical Computation 6, 15–29.
Mangasarian, O.L. and Solodov, M.V. (1993), Nonlinear complementarity as unconstrained and constrained minimization. Mathematical Programming 62, 277–297.
Qi, L. (1993), Convergence analysis of some algorithms for solving nonsmooth equations. Mathematics of Operations Research 18, 227–244.
Robinson, S.M. (1980), Strongly regular generalized equations. Mathematics of Operations Research 5, 43–62.
Sellami, H. and Robinson, S.M. (1996), Homotopies Based on Nonsmooth Equations for Solving Nonlinear Variational Inequalities. In: G. Di Pillo and F. Giannessi (eds.), Nonlinear Optimization and Applications. Plenum Press, New York, NY.
Sellami, H. and Robinson, S.M. (1997), Implementation of a continuation method for normal maps. Mathematical Programming 76, 563–578.
Sun, D. A regularization Newton method for solving nonlinear complementarity problems. Applied Mathematics and Optimization, to appear.
Sun, D. and Womersley, R.S. A new unconstrained differentiable merit function for box constrained variational inequality problems and a damped Gauss-Newton method. SIAM Journal on Optimization, to appear.
Watson, L.T. (1979), Solving the nonlinear complementarity problem by a homotopy method. SIAM Journal on Control and Optimization 17, 36–46.
Yamashita, N. and Fukushima, M. (1995), On stationary points of the implicit Lagrangian for nonlinear complementarity problems. Journal of Optimization Theory and Applications 84, 653–663.
Zhou, G., Sun, D. and Qi, L. (1998), Numerical Experiments for a Class of Squared Smoothing Newton Methods for Box Constrained Variational Inequality Problems. in M. Fukushima and L. Qi (eds.), Reformulation: Nonsmooth, Piecewise Smooth, Semismooth and Smoothing Methods. Kluwer Academic Publishers, Norwell, MD, 421–441.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Kanzow, C. Global Optimization Techniques for Mixed Complementarity Problems. Journal of Global Optimization 16, 1–21 (2000). https://doi.org/10.1023/A:1008331803982
Issue Date:
DOI: https://doi.org/10.1023/A:1008331803982