Hostname: page-component-8448b6f56d-c47g7 Total loading time: 0 Render date: 2024-04-19T13:15:29.701Z Has data issue: false hasContentIssue false
  • English
  • Français

Truth definitions without exponentiation and the Σ1 collection scheme

Published online by Cambridge University Press:  12 March 2014

Zofia Adamowicz ,
Leszek Aleksander Kołodziejczyk  and
J. Paris
Zofia Adamowicz
Affiliation:
Mathematical Institute of the Polish Academy of Sciences, Śniadeckich 8, 00-956 Warszawa, Poland, E-mail: zosiaa@impan.pl
Leszek Aleksander Kołodziejczyk
Affiliation:
Institute of Mathematics, University of Warsaw, Banacha 2, 02-097 Warszawa, Poland, E-mail: lak@mimuw.edu.pl
J. Paris
Affiliation:
School of Mathematics, University of Manchester, Manchester M13 9PL, UK, E-mail: jeff.paris@manchester.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that:

  1. • if there is a model of IΔ0 + ¬exp with cofinal Σ1-definable elements and a Σ1 truth definition for Σ1 sentences, then IΔ0 + ¬exp + ¬BΣ1 is consistent,

  2. • there is a model of IΔ0 + Ω1 + ¬exp with cofinal Σ1-definable elements, both a Σ2 and a Π2 truth definition for Σ1 sentences, and for each n ≥ 2, a Σn truth definition for Σn sentences.

The latter result is obtained by constructing a model with a recursive truth-preserving translation of Σ1 sentences into boolean combinations of 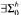sentences.

We also present an old but previously unpublished proof of the consistency of IΔ0 + ¬exp + ¬BΣ1 under the assumption that the size parameter in Lessan's Δ0 universal formula is optimal. We then discuss a possible reason why proving the consistency of IΔ0 + ¬exp + ¬BΣ1 unconditionally has turned out to be so difficult.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 77 , Issue 2 , June 2012 , pp. 649 - 655
Copyright
Copyright © Association for Symbolic Logic 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[AKZ03] Adamowicz, Z., Kołodziejczyk, L. A., and Zbierski, P., An application of a reflection principle, Fundamenta Mathematicae, vol. 180 (2003), pp. 139–159.Google Scholar
[Bus95] Buss, S. R., Relating the bounded arithmetic and polynomial time hierarchies, Annals of Pure and Applied Logic, vol. 75 (1995), pp. 67–77.CrossRefGoogle Scholar
[HP93] Hájek, P. and Pudlák, P., Metamathematics of first-order arithmetic, Springer-Verlag, 1993.Google Scholar
[KP78] Kirby, L. A. S. and Paris, J. B., Σn collection schemas in arithmetic. Logic colloquium 77 (Macintyre, A.. Pacholski, L., and Paris, J., editors), Studies in Logic and the Foundations of Mathematics, vol. 96, North-Holland, 1978, pp. 199–209.Google Scholar
[Les78] Lessan, H., Models of arithmetic, Ph.D. thesis, University of Manchester, 1978.Google Scholar
[WP89] Wilkie, A. J. and Paris, J. B., On the existence of end-extensions of models of bounded induction, Logic, methodology, and philosophy of science VIII (Moscow 1987) (Fenstad, J. E., Frolov, I. T., and Hilpinen, R., editors), North-Holland, 1989, pp. 143–161.Google Scholar
[Zam96] Zambella, D., Notes on polynomially bounded arithmetic, this Journal, vol. 61 (1996), pp. 942–966.Google Scholar
[Zam97] Zambella, D., End extensions of models of polynomially bounded arithmetic, Annals of Pure and Applied Logic, vol. 88 (1997), pp. 263–277.Google Scholar