Hostname: page-component-8448b6f56d-gtxcr Total loading time: 0 Render date: 2024-04-23T14:40:56.179Z Has data issue: false hasContentIssue false
  • English
  • Français

Relative recursive enumerability of generic degrees

Published online by Cambridge University Press:  12 March 2014

Masahiro Kumabe
Masahiro Kumabe*
Affiliation:
Department of Mathematics, University of Minnesota, Minneapolis, Minnesota 55455
Get access Rights & Permissions [Opens in a new window]

Extract

Let ω be the set of natural numbers, i.e. {0, 1, 2, 3, …}. A string is a mapping from an initial segment of ω into {0, 1}. We identify a set A ≤ ω with its characteristic function. A set A ≤ ω is called n-generic if it is Cohen-generic for n-quantifier arithmetic. This is equivalent to saying that for every 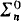set of strings S, there is a σ < A such that σ ∈ S or (∀ν ≥ σ)(ν ∉ S). By degree we mean Turing degree (of unsolvability). We call a degree n-generic if it has an n-generic representative. For a degree a, D(≤ a) denotes the set of degrees recursive in a.

The relation between generic degrees and minimal degrees has been widely studied. Spector [9] proved the existence of minimal degrees. Shoenfield [8] simplified the proof by using trees. In the construction of a minimal degree, given σ we extend σ to ν so that ν is in the (splitting or nonsplitting) subtree of a given tree. But in the construction of a generic set, given σ we extend σ to ν to meet the given dense set. So these two constructions are quite different. Jockusch [5] showed that any 2-generic degree bounds no minimal degree. Chong and Jockusch [3] showed that any 1-generic degree below 0′ bounds no minimal degree.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 56 , Issue 3 , September 1991 , pp. 1075 - 1084
Copyright
Copyright © Association for Symbolic Logic 1991

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Chong, C. T., Minimal degrees recursive in I-generic degrees (to appear).Google Scholar
[2] Chong, C. T. and Downey, R. G., On degrees bounding minimal degrees (to appear).Google Scholar
[3] Chong, C. T. and Jockusch, C. G., Minimal degrees and 1-generic degrees below 0′, Computation and proof theory (logic colloquium '83, part ii), Lecture Notes in Mathematics, vol. 1104, Springer-Verlag, Berlin, 1984, pp. 63–77.CrossRefGoogle Scholar
[4] Haught, C., The degrees below a 1-generic degree < 0′, this Journal, vol. 51 (1986), pp. 770–777.Google Scholar
[5] Jockusch, C. G., Degrees of generic sets, Recursion theory, its generalizations and applications (proceedings, Leeds, 1979), London Mathematical Society Lecture Note Series, vol. 45, Cambridge University Press, Cambridge, 1980, pp. 110–139.CrossRefGoogle Scholar
[6] Kumabe, M., A 1-generic degree which bounds a minimal degree, this Journal, vol. 55 (1990), pp. 733–743.Google Scholar
[7] Lerman, M., Degrees of unsohability, Springer-Verlag, Berlin, 1983.CrossRefGoogle Scholar
[8] Shoenfield, J. R., A theorem on minimal degrees, this Journal, vol. 31 (1966), pp. 539–544.Google Scholar
[9] Spector, C., On degrees of recursive unsohability, Annals of Mathematics, ser. 2, vol. 64 (1956), pp. 581–592.CrossRefGoogle Scholar