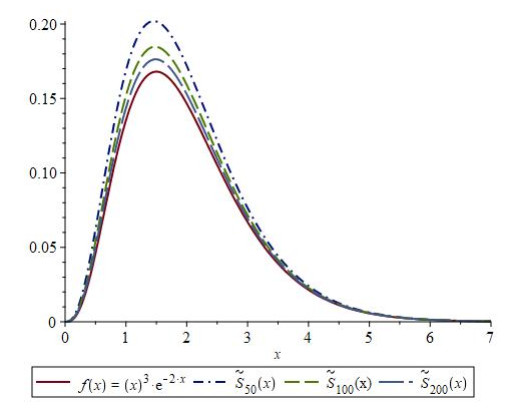
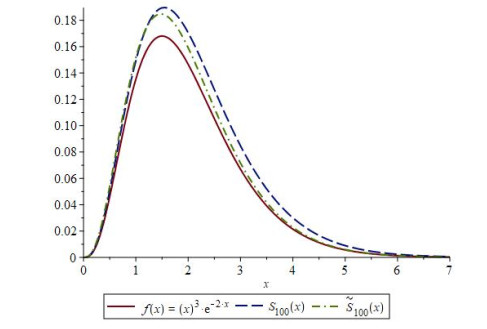
|
[1]
|
U. Abel and V. Gupta, Rate of convergence of exponential type operators related to p(x)=2x3/2 for functions of bounded variation, Revista de la Real Academia de Ciencias Exactas Físicas y Naturales. Serie A. Matemáticas (RACSAM), 114 (2020), Paper No. 188, 8 pp.
doi: 10.1007/s13398-020-00919-y.


|
|
[2]
|
U. Abel, V. Gupta and V. Kushnirevych, Asymptotic expansions for certain exponential-type operators connected with 2x3/2, Mathematical Sciences, 15 (2021), 311-315.
doi: 10.1007/s40096-021-00382-9.


|
|
[3]
|
U. Abel, V. Gupta and M. Sisodia, Some new semi-exponential operators, Revista de la Real Academia de Ciencias Exactas Físicas y Naturales. Serie A. Matemáticas (RACSAM), 116 (2022), Paper No. 87, 12 pp.
doi: 10.1007/s13398-022-01228-2.


|
|
[4]
|
T. Acar, A. Aral, D. Cárdenas-Morales and P. Garrancho, Szasz-Mirakyan type operators which fix exponentials, Results in Mathematics, 72 (2017), 1393-1404.
doi: 10.1007/s00025-017-0665-9.


|
|
[5]
|
T. Acar, A. Aral and H. H. Gonska, On Szász-Mirakyan operators preserving e2ax,a>0, Mediterranian J. Math., 14 (2017), Paper No. 6, 14 pp.
doi: 10.1007/s00009-016-0804-7.


|
|
[6]
|
T. Acar, A. Aral and I. Raşa, Positive Linear Operators Preserving τ and τ2, Constructive Mathematical Analysis, 2 (2019), 98-102.


|
|
[7]
|
T. Acar, M. Cappelletti Montano, P. Garrancho and V. Leonessa, On Bernstein-Chlodovsky operators preserving e−2x, Bulletin of the Belgian Mathematical Society-Simon Stevin, 26 (2019), 681-698.
doi: 10.36045/bbms/1579402817.


|
|
[8]
|
T. Acar, M. Cappelletti Montano, P. Garrancho and V. Leonessa, Voronovskaya type results for Bernstein-Chlodovsky operators preserving e−2x, J. Math. Anal. Appl., 491 (2020), 124307, 14 pp.
doi: 10.1016/j.jmaa.2020.124307.


|
|
[9]
|
R. A. DeVore and G. G. Lorentz, Constructive Approximation, Springer, Berlin, 1993.


|
|
[10]
|
Z. Ditzian, On global inverse theorems of Szász and Baskakov operators, Canad. J. Math., 31 (1979), 255-263.
doi: 10.4153/CJM-1979-027-2.


|
|
[11]
|
H. Feng, S. Z. Hou, L. Y. Wei and D. X. Zhou, CNN models for readability of Chinese texts, Mathematical Foundations of Computing, 5 (2022), 351-362.
doi: 10.3934/mfc.2022021.

|
|
[12]
|
H. H. Gonska, Quantitative Korovkin-type theorems on simultaneous approximation, Math. Z., 186 (1984), 419-433.
doi: 10.1007/BF01174895.


|
|
[13]
|
V. Gupta, Convergence estimates of certain exponential type operators, Springer Proceedings in Mathematics and Statistics, 306 (2020), 47-55.
doi: 10.1007/978-981-15-1153-0_4.


|
|
[14]
|
V. Gupta, M. López-Pellicer and H. M. Srivastava, Convergence estimates of a family of approximation operators of exponential type, Filomat, 34 (2020), 4329-4341.
doi: 10.2298/FIL2013329G.


|
|
[15]
|
X. Guo, L. X. Li and Q. Wu, Modeling interactive components by coordinate kernel polynomial models, Mathematical Foundations of Computing, 3 (2020), 263-277.
doi: 10.3934/mfc.2020010.

|
|
[16]
|
V. Gupta and G. Tachev, Approximation with Positive Linear Operators and Linear Combinations, , Series: Developments in Mathematics, Volume 50, Springer, Cham, 2017.
doi: 10.1007/978-3-319-58795-0.


|
|
[17]
|
A. Holhoş, The rate of approximation of functions in an infinite interval by positive linear operators, Stud. Univ. Babeş-Bolyai Math., 55 (2010), 133-142.


|
|
[18]
|
S. Y. Huang, Y. L. Feng and Q. Wu, Learning theory of minimum error entropy under weak moment conditions, Anal. Appl., 20 (2022), 121-139.
doi: 10.1142/S0219530521500044.


|
|
[19]
|
M. Ismail and C. P. May, On a family of approximation operators, J. Math. Anal. Appl., 63 (1978), 446-462.
doi: 10.1016/0022-247X(78)90090-2.


|
|
[20]
|
C. P. May, Saturation and Inverse theorems for combinations of a class of exponential type operators, Canad. J. Math., 28 (1976), 1224-1250.
doi: 10.4153/CJM-1976-123-8.


|
|
[21]
|
F. Ozsarac and T. Acar, Reconstruction of Baskakov operators preserving some exponential functions, Mathematical Methods in the Applied Sciences, 42 (2019), 5124-5132.
doi: 10.1002/mma.5228.


|
|
[22]
|
R. Păltănea, Optimal estimates with moduli of continuity, Results Math., 32 (1997), 318-331.
doi: 10.1007/BF03322143.


|
|
[23]
|
K. Sato, Global approximation theorems for some exponential-type operators, J. Approx. Theory, 32 (1981), 32-46.
doi: 10.1016/0021-9045(81)90020-4.


|
|
[24]
|
G. Tachev, V. Gupta and A. Aral, Voronovskaja's theorem for functions with exponential growth, Georgian Math. J., 27 (2020), 459-468.
doi: 10.1515/gmj-2018-0041.


|
|
[25]
|
V. Totik, Uniform approximation by exponential-type operators, J. Math. Anal. Appl., 132 (1988), 238-246.
doi: 10.1016/0022-247X(88)90057-1.


|







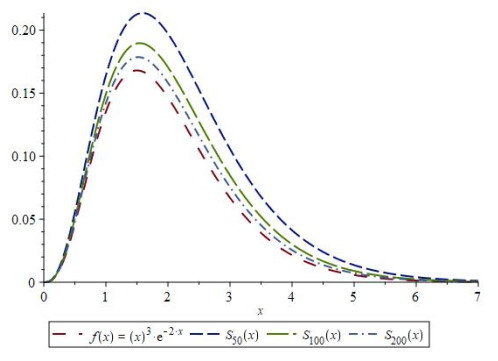
 Download:
Download: