Abstract
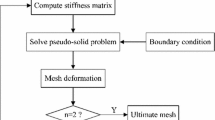
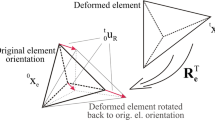
Deformation tools constitute an important topic in computer animation. This paper shows how we can give an exact value to the basic parameters in a dynamical elastic deformation system based on finite elements for 2D objects. We look for an optimal value for the time step ( Δt ) in the dynamical system, and an optimal area for the basic square finite element of the object. Fine time step adjustment is important to reduce the computational cost of the system, and guarantee the realistic look of the result (final deformation of the object). Then several results from different physical conditions are compared, in order to find a good system of measuring the difference between them. Finally, using this measurement parameter we can relate the size of the finite elements with the error between several deformations of the same object. The deformations are rendered using the Open Inventor application (VRML).
Supported by the project TIC98-0302 of CICYT.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Mascaró, M., Mir, A., Perales, F.: Modelado y animación de objetos deformables basado en la teoría de la elasticidad. CEIG 1997, 95–110 (1997)
Mascaró, M., Mir, A., Perales, F.: Visualización de deformaciones dinámicas mediante elementos finitos triangulares y cuadrangulares. CEIG 1999, 47–61 (1999)
Terzopoulos, D., et al.: Elastically Deformable Models. In: Computer Graphics (Proc. Siggraph), vol. 21(4), pp. 205–214 (1987)
Terzopoulos, D., Fleischer, K.: Deformable Models. Visual Computer 21(4), 306–331 (1988)
Terzopoulos, D., Witkin, A., Kass, M.: Dynamic 3D models with local and global deformations: Deformable superquadratics. IEEE Transactions on PAMI 13(7), 703–714 (1991)
Palmer, P., Mir, A., González, M.: Simulació Dinàmica i Deformacions. Memoria de investigación. Universidad de las Islas Baleares. Junio (1998)
Aono, M.: A Wrinkle Propagation Model for Cloth. In: Proc. CG. Int’l, pp. 95–115. Springer, Heidelberg (1990)
Thalmann, N.M., Yang, Y.: Techniques for Cloth Animation. In: M-Thalmann, N., Thalmann, D. (eds.) New Trends in Animation and Visualization, pp. 243–256. John Wiley & Sons, Chichester (1991)
Serón, F.J., Badal, J., Sabadell, F.J.: A numerical laboratory for simulation and visualization of seismic wavefields. Geophysical Prospecting 44, 603–642 (1996)
Provot, X.: Deformation Constraints in a Mass-Spring Model to Describe Rigid Cloth Behavior. INRIA (1995)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2000 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Mascaró, M., Mir, A., Perales, F. (2000). Elastic Deformations Using Finite Element Methods in Computer Graphic Applications. In: Nagel, HH., Perales López, F.J. (eds) Articulated Motion and Deformable Objects. AMDO 2000. Lecture Notes in Computer Science, vol 1899. Springer, Berlin, Heidelberg. https://doi.org/10.1007/10722604_4
Download citation
DOI: https://doi.org/10.1007/10722604_4
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-67912-7
Online ISBN: 978-3-540-44591-3
eBook Packages: Springer Book Archive