Skip to main content
Springer Nature Link
Log in

Least–Squares Approximation by Pythagorean Hodograph Spline Curves Via an Evolution Process

  • Conference paper
Geometric Modeling and Processing - GMP 2006 (GMP 2006)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 4077))

Included in the following conference series:

  • 1554 Accesses

Abstract

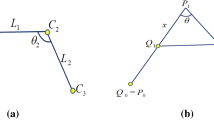
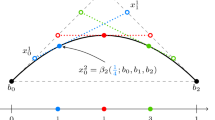
The problem of approximating a given set of data points by splines composed of Pythagorean Hodograph (PH) curves is addressed. In order to solve this highly non-linear problem, we formulate an evolution process within the family of PH spline curves. This process generates a one–parameter family of curves which depends on a time–like parameter t. The best approximant is shown to be a stationary point of this evolution. The evolution process – which is shown to be related to the Gauss–Newton method – is described by a differential equation, which is solved by Euler’s method.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Alhanaty, M., Bercovier, M.: Curve and surface fitting and design by optimal control methods. Computer–Aided Design 33, 167–182 (2001)

    Article  MATH  Google Scholar 

  2. Aigner, M., Jüttler, B.: Hybrid curve fitting. FSP Industrial Geometry, Report no. 2 (2005), available at: http://www.ig.jku.at

  3. Farouki, R.T.: Pythagorean-hodograph curves. In: Handbook of computer aided geometric design, pp. 405–427. North-Holland, Amsterdam (2002)

    Chapter  Google Scholar 

  4. Farouki, R.T., Kuspa, B.K., Manni, C., Sestini, A.: Efficient solution of the complex quadratic tridiagonal system for \(C\sp 2\) PH quintic splines. Numer. Algorithms 27(1), 35–60 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  5. Farouki, R.T., Manjunathaiah, J., Jee, S.: Design of rational cam profiles with pythagorean-hodograph curves. Mech. and Mach. Theory 33(6), 669–682 (1998)

    Article  MATH  Google Scholar 

  6. Farouki, R.T., Saitou, K., Tsai, Y.-F.: Least-squares tool path approximation with Pythagorean-hodograph curves for high-speed CNC machining. In: The mathematics of surfaces, VIII (Birmingham, 1998), Info. Geom., Winchester, pp. 245–264 (1998)

    Google Scholar 

  7. Hoff, K.E., Keyser, J., Lin, M., Manocha, D., Culver, T.: Fast computation of generalized Voronoi diagrams using graphics hardware. In: SIGGRAPH 1999, New York, pp. 277–286. ACM Press/Addison-Wesley (1999)

    Google Scholar 

  8. Hoschek, J., Lasser, D.: Fundamentals of computer aided geometric design. A K Peters, Wellesley (1993)

    MATH  Google Scholar 

  9. Kubota, K.K.: Pythagorean triplets in unique factorization domains. Amer. Math. Monthly 79, 503–505 (1972)

    Article  MATH  MathSciNet  Google Scholar 

  10. Kass, M., Witkin, A., Terzopoulos, D.: Snakes: active contour models. Int. J. Comp. Vision 1, 321–331 (1987)

    Article  Google Scholar 

  11. Moon, H.P., Farouki, R.T., Choi, H.I.: Construction and shape analysis of PH quintic Hermite interpolants. Comput. Aided Geom. Design 18(2), 93–115 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  12. Meek, D.S., Walton, D.J.: Geometric Hermite interpolation with Tschirnhausen cubics. J. Comput. Appl. Math. 81(2), 299–309 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  13. Pottmann, H., Leopoldseder, S.: A concept for parametric surface fitting which avoids the parametrization problem. Comp. Aided Geom. Design 20, 343–362 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  14. Pottmann, H., Leopoldseder, S., Hofer, M.: Approximation with active B-spline curves and surfaces. In: Proc. Pacific Graphics, pp. 8–25. IEEE Press, Los Alamitos (2002)

    Google Scholar 

  15. Pottmann, H., Leopoldseder, S., Hofer, M., Steiner, T., Wang, W.: Industrial geometry: recent advances and appl. in CAD. Comp.-Aided Design 37, 751–766 (2005)

    Article  Google Scholar 

  16. Rogers, D., Fog, N.: Constrained B-spline curve and surface fitting. Computer–Aided Design 21, 641–648 (1989)

    Article  MATH  Google Scholar 

  17. Šír, Z., Jüttler, B.: Constructing acceleration continuous tool paths using Pythagorean hodograph curves. Mech. and Mach. Theory 40(11), 1258–1272 (2005)

    Article  MATH  Google Scholar 

  18. Speer, T., Kuppe, M., Hoschek, J.: Global reparametrization for curve approximation. Comput. Aided Geom. Design 15, 869–877 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  19. Wang, W., Pottmann, H., Liu, Y.: Fitting B-spline curves to point clouds by squared distance minimization. ACM Transactions on Graphics 25(2) (2006)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Johannes Kepler University Linz, Austria

    M. Aigner, Z. Šír & B. Jüttler

Authors
  1. M. Aigner
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Z. Šír
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. B. Jüttler
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Seoul National University, Korea

    Myung-Soo Kim

  2. Carnegie Mellon University, Pittsburgh, PA, USA

    Kenji Shimada

Rights and permissions

Reprints and permissions

Copyright information

© 2006 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Aigner, M., Šír, Z., Jüttler, B. (2006). Least–Squares Approximation by Pythagorean Hodograph Spline Curves Via an Evolution Process. In: Kim, MS., Shimada, K. (eds) Geometric Modeling and Processing - GMP 2006. GMP 2006. Lecture Notes in Computer Science, vol 4077. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11802914_4

Download citation

  • DOI: https://doi.org/10.1007/11802914_4

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-36711-6

  • Online ISBN: 978-3-540-36865-6

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics