Skip to main content
Springer Nature Link
Log in

Stochastic Shortest Paths Via Quasi-convex Maximization

  • Conference paper
Algorithms – ESA 2006 (ESA 2006)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 4168))

Included in the following conference series:

Abstract

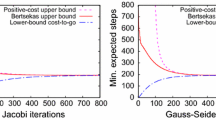
We consider the problem of finding shortest paths in a graph with independent randomly distributed edge lengths. Our goal is to maximize the probability that the path length does not exceed a given threshold value (deadline). We give a surprising exact n Θ(logn) algorithm for the case of normally distributed edge lengths, which is based on quasi-convex maximization. We then prove average and smoothed polynomial bounds for this algorithm, which also translate to average and smoothed bounds for the parametric shortest path problem, and extend to a more general non-convex optimization setting. We also consider a number other edge length distributions, giving a range of exact and approximation schemes.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Ackermann, H., Newman, A., Röglin, H., Vöcking, B.: Decision making based on approximate and smoothed pareto curves. In: Deng, X., Du, D.-Z. (eds.) ISAAC 2005. LNCS, vol. 3827, pp. 675–684. Springer, Heidelberg (2005)

    Chapter  Google Scholar 

  2. Bertsekas, D.: Dynamic Programming and Optimal Control, 2nd edn., vol. II. Athena Scientific, Belmont (2001)

    MATH  Google Scholar 

  3. Boyan, J., Mitzenmacher, M.: Improved results for route planning in stochastic transportation networks. In: Proc. of Symposium of Discrete Algorithms (2001)

    Google Scholar 

  4. Carstensen, P.: The complexity of some problems in parametric linear and combinatorial programming. Ph.D. Thesis, Mathematics Dept., U. of Michigan, Ann Arbor, Mich. (1983)

    Google Scholar 

  5. Dean, B., Goemans, M., Vondrak, J.: Approximating the stochastic knapsack: The benefit of adaptivity. In: Proceedings of FOCS, pp. 208–217 (2004)

    Google Scholar 

  6. Fan, Y., Kalaba, R., Moore, I.J.E.: Arriving on time. Journal of Optimization Theory and Applications (forthcoming)

    Google Scholar 

  7. Goel, A., Indyk, P.: Stochastic load balancing and related problems. In: Proceedings of the 40th Symposium on Foundations of Computer Science (1999)

    Google Scholar 

  8. Horst, R., Pardalos, P.M., Thoai, N.V.: Introduction to Global Optimization. Kluwer Academic Publishers, Dordrecht (2000)

    MATH  Google Scholar 

  9. Karger, D., Motwani, R., Ramkumar, G.D.S.: On approximating the longest path in a graph. Algorithmica 18, 82–98 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  10. Kelner, J.A., Spielman, D.A.: A randomized polynomial-time simplex algorithm for linear programming. Electronic Colloquium on Computational Complexity (156) (2005)

    Google Scholar 

  11. Kleinberg, J., Rabani, Y., Tardos, É.: Allocating bandwidth for bursty connections. SIAM Journal on Computing 30(1), 191–217 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  12. Loui, R.P.: Optimal paths in graphs with stochastic or multidimentional weights. Communications of the ACM 26, 670–676 (1983)

    Article  MATH  MathSciNet  Google Scholar 

  13. Miller-Hooks, E.D., Mahmassani, H.S.: Least expected time paths in stochastic, time-varying transportation networks. Transportation Science 34, 198–215 (2000)

    Article  MATH  Google Scholar 

  14. Mirchandani, P., Soroush, H.: Optimal paths in probabilistic networks: A case with temporary preferences. Computers and Operations Research 12(4), 365–381 (1985)

    Article  MATH  MathSciNet  Google Scholar 

  15. Mote, J., Murthy, I., Olson, D.: A parametric approach to solving bicriterion shortest path problems. European Journal of Operational Research 53, 81–92 (1991)

    Article  MATH  Google Scholar 

  16. Mulmuley, K., Shah, P.: A lower bound for the shortest path problem. Journal of Computer and System Sciences 63(2), 253–267 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  17. Nikolova, E., Brand, M., Karger, D.R.: Optimal route planning under uncertainty. In: Proceedings of International Conference on Automated Planning and Scheduling (2006)

    Google Scholar 

  18. Nikolova, E., Kelner, J.A.: On the hardness and smoothed complexity of low-rank quasi-concave minimization (May 2006) (manuscript)

    Google Scholar 

  19. Pallottino, S., Scutella, M.G.: Shortest path algorithms in transportation models: Classical and innovative aspects. Technical Report TR-97-06, Universita di Pisa Dipartimento di Informatica, Pisa, Italy (1997)

    Google Scholar 

  20. Papadimitriou, C.H., Yannakakis, M.: Shortest paths without a map. Theoretical Computer Science 84, 127–150 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  21. Papadimitriou, C.H., Yannakakis, M.: On limited nondeterminism and the complexity of the V-C dimension. Journal of Computer and System Sciences 53(2), 161–170 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  22. Polychronopoulos, G.H., Tsitsiklis, J.N.: Stochastic shortest path problems with recourse. Networks 27(2), 133–143 (1996)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. MIT CSAIL, MERL, Harvard University, Cambridge, MA

    Evdokia Nikolova, Jonathan A. Kelner, Matthew Brand & Michael Mitzenmacher

Authors
  1. Evdokia Nikolova
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jonathan A. Kelner
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Matthew Brand
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Michael Mitzenmacher
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Microsoft Research, One Microsoft Way, Redmond, WA 98052, USA and School of Computer Science, Tel-Aviv University, 69978, Tel-Aviv, Israel

    Yossi Azar

  2. Department of Computer Science, University of Leicester, University Road, LE1 7RH, Leicester, UK

    Thomas Erlebach

Rights and permissions

Reprints and permissions

Copyright information

© 2006 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Nikolova, E., Kelner, J.A., Brand, M., Mitzenmacher, M. (2006). Stochastic Shortest Paths Via Quasi-convex Maximization. In: Azar, Y., Erlebach, T. (eds) Algorithms – ESA 2006. ESA 2006. Lecture Notes in Computer Science, vol 4168. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11841036_50

Download citation

  • DOI: https://doi.org/10.1007/11841036_50

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-38875-3

  • Online ISBN: 978-3-540-38876-0

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics