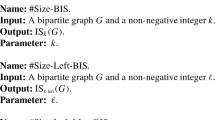Abstract
Many parameterized problems (as the clique problem or the dominating set problem) ask, given an instance and a natural number k as parameter, whether there is a solution of size k. We analyze the relationship between the complexity of such a problem and the corresponding maximality (minimality) problem asking for a solution of size k maximal (minimal) with respect to set inclusion. As our results show maximality problems may increase the parameterized complexity, while “in terms of the W-hierarchy” minimality problems do not increase the complexity.
This is a preview of subscription content, log in via an institution to check access.
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Arvind, V., Raman, V.: Approximation Algorithms for Some Parameterized Counting Problems. In: Bose, P., Morin, P. (eds.) ISAAC 2002. LNCS, vol. 2518, pp. 453–464. Springer, Heidelberg (2002)
Chang, C.C., Keisler, H.J.: Model Theory. In: Studies in Logic and Mathematical Foundations, vol. 73. North-Holland, Amsterdam (1990)
Chen, Y., Flum, J., Grohe, M.: Bounded nondeterminism and alternation in parameterized complexity theory. In: Proceedings of the 18th IEEE Conference on Computational Complexity CCC 2003, pp. 13–29 (2003)
Downey, R.G., Fellows, M.R.: Parameterized Complexity. Springer, Heidelberg (1999)
Downey, R.G., Fellows, M.R., Regan, K.: Descriptive complexity and the W-hierarchy. In: Proof Complexity and Feasible Arithmetic. AMS-DIMACS Series, vol. 39, pp. 119–134 (1998)
Eiter, T., Gottlob, G.: Identifying the minimal transversals of a hypergraph and related problems. SIAM Journal on Computing 24(6), 1278–1304 (1995)
Fernau, H.: Parameterized algorithms: A graph-theoretic approach. Habilitationsschrift, Universität Tübingen, Tübingen, Germany (2005)
Flum, J., Grohe, M.: Fixed-parameter tractability, definability, and model checking. SIAM Journal on Computing 31(1), 113–145 (2001)
Flum, J., Grohe, M.: The parameterized complexity of counting problems. SIAM Journal on Computing 33(4), 892–922 (2005)
Flum, J., Grohe, M.: Model-checking problems as a basis for parameterized intractability. Logical Methods in Computer Science 1(1) (2004)
Flum, J., Grohe, M.: Parameterized Complexity Theory. Springer, Heidelberg (2006)
Flum, J., Grohe, M., Weyer, M.: Bounded fixed-parameter tractability and \(\mbox{log}^{2}n\) nondeterministic bits. Journal of Computer and System Sciences 72, 34–71 (2006)
Khot, S., Raman, V.: Parameterized complexity of finding subgraphs with hereditary properties. Theoretical Computer Science 289(2), 997–1008 (2002)
Ritter, C.: Fagin-Definierbarkeit. Diplomarbeit, Universität Freiburg (2005)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2006 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Chen, Y., Flum, J. (2006). The Parameterized Complexity of Maximality and Minimality Problems. In: Bodlaender, H.L., Langston, M.A. (eds) Parameterized and Exact Computation. IWPEC 2006. Lecture Notes in Computer Science, vol 4169. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11847250_3
Download citation
DOI: https://doi.org/10.1007/11847250_3
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-39098-5
Online ISBN: 978-3-540-39101-2
eBook Packages: Computer ScienceComputer Science (R0)