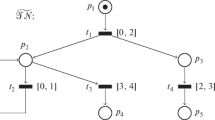
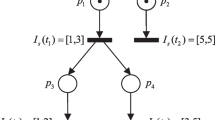
Abstract
Several recent papers investigate the relative expressiveness of Timed Automata and Time Petri Nets, two widespread models for realtime systems. It has been shown notably that Timed Automata and Bounded Time Petri Nets are equally expressive in terms of timed language acceptance, but that Timed Automata are strictly more expressive in terms of weak timed bisimilarity. This paper compares Timed Automata with Bounded Time Petri Nets extended with static Priorities, and shows that two large subsets of these models are equally expressive in terms of weak timed bisimilarity.
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Alur, R.: Timed automata. In: Halbwachs, N., Peled, D.A. (eds.) CAV 1999. LNCS, vol. 1633, pp. 8–22. Springer, Heidelberg (1999)
Alur, R., Dill, D.L.: A theory of timed automata. TCS 126, 183–235 (1994)
Bérard, B., Cassez, F., Haddad, S., Roux, O.H., Lime, D.: Comparison of the Expressiveness of Timed Automata and Time Petri Nets. In: Pettersson, P., Yi, W. (eds.) FORMATS 2005. LNCS, vol. 3829, pp. 211–225. Springer, Heidelberg (2005)
Berthomieu, B., Diaz, M.: Modeling and verification of time dependent systems using time Petri nets. IEEE Trans. on Software Engineering 17(3), 259–273 (1991)
Berthomieu, B., Menasche, M.: An enumerative approach for analyzing time Petri nets. IFIP Congress Series 9, 41–46 (1983)
Berthomieu, B., Vernadat, F.: State class constructions for branching analysis of time Petri nets. In: Garavel, H., Hatcliff, J. (eds.) TACAS 2003. LNCS, vol. 2619, pp. 442–457. Springer, Heidelberg (2003)
Bornot, S., Sifakis, J., Tripakis, S.: Modeling urgency in timed systems. In: de Roever, W.-P., Langmaack, H., Pnueli, A. (eds.) COMPOS 1997. LNCS, vol. 1536, pp. 103–129. Springer, Heidelberg (1998)
Bouyer, P., Haddad, S., Reynier, P.-A.: Extended timed automata and time Petri nets. In: Proc. of 6th Int. Conf. on Application of Concurrency to System Design (ACSD 2006), Turku, Finland. IEEE Computer Society Press, Los Alamitos (2006)
Cassez, F., Roux, O.H.: Structural translation from time petri nets to timed automata. Journal of Systems and Software (forthcoming, 2006)
D’Argenio, P.R., Gebremichael, B.: The coarsest congruence for timed automata with deadlines contained in bisimulation. In: Abadi, M., de Alfaro, L. (eds.) CONCUR 2005. LNCS, vol. 3653, pp. 125–140. Springer, Heidelberg (2005)
Haar, S., Kaiser, L., Simonot-Lion, F., Toussaint, J.: Equivalence of Timed State Machines and safe Time Petri Nets. In: Proc. of the 6th Workshop on Discrete Events Systems (WODES 2002), Zaragoza, Spain, pp. 119–124 (October 2002)
Hack, M.: Petri net languages. TR 159. MIT, Cambridge, Mass (1976)
Larsen, K.G., Pettersson, P., Yi, W.Y.: Model-checking for real-time systems. In: Reichel, H. (ed.) FCT 1995. LNCS, vol. 965, pp. 62–88. Springer, Heidelberg (1995)
Lime, D., Roux, O.H.: State class timed automaton of a time Petri net. In: 10th International Workshop on Petri Nets and Performance Models (PNPM 2003), Urbana, USA, September 2003, pp. 124–133. IEEE Computer Society, Los Alamitos (2003)
Merlin, P.M., Farber, D.J.: Recoverability of communication protocols: Implications of a theoretical study. IEEE Tr. Comm. 24(9), 1036–1043 (1976)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2006 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Berthomieu, B., Peres, F., Vernadat, F. (2006). Bridging the Gap Between Timed Automata and Bounded Time Petri Nets. In: Asarin, E., Bouyer, P. (eds) Formal Modeling and Analysis of Timed Systems. FORMATS 2006. Lecture Notes in Computer Science, vol 4202. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11867340_7
Download citation
DOI: https://doi.org/10.1007/11867340_7
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-45026-9
Online ISBN: 978-3-540-45031-3
eBook Packages: Computer ScienceComputer Science (R0)