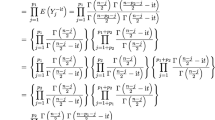Skip to main content
SpringerLink
Log in
Book cover

Data Analysis and Decision Support pp 113–121Cite as

ANOVA Models with Generalized Inverses

  • Chapter

  • 2332 Accesses

Abstract

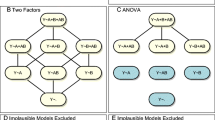
The 2-way ANOVA model is analyzed with generalized matrix or ginverses. We derive the co-called OLS− and OLS+ estimators of the rank deficient ANOVA model. The new g-inverses lead to two simple effects in a two-way ANOVA model: column means and adjusted row means or vice versa: row means and adjusted column means. For the F- Test this parameterizations is invariant.

This is a preview of subscription content, log in via an institution.

Chapter
USD   29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
USD   84.99
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
USD   109.99
Price excludes VAT (USA)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Learn about institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

References

  • DODGE, Y. and MAJUMDAR, D. (1979): An algorithm for finding least square generalized inverses for classification models with arbitrary patterns. J. Statist. Comput. Simul., 9, 1–17.

    MathSciNet  Google Scholar 

  • GRAYBILL, F.A. (1976): Theory and Application of the Linear Model, Wadsworth & Brooks/Cole, Pacific Grove, California.

    Google Scholar 

  • MAGNUS, J.R. and NEUDECKER, H. (1988): Matrix Differential Calculus with Applications in Statistics and Econometrics, Wiley, Chichester.

    Google Scholar 

  • POIRIER, D.J. (1995): Intermediate Statistics and Econometrics, The MIT Press, Cambridge, Mass.

    Google Scholar 

  • POLASEK, W. and LIU, S. (1997): MANOVA models: A Bayesian analysis, University of Basel, mimeo.

    Google Scholar 

  • POLASEK, W. and POETZELBERGER, K. (1994): Robust Bayesian methods in simple ANOVA models. J. Statist. Planning and Inference, 40, 295–311.

    Article  Google Scholar 

  • PRESS, S.J. (1989): Bayesian Statistics: Principles, Models and Applications, Wiley, New York.

    Google Scholar 

  • RAO, C.R. (1973): Linear Statistical Inference and Applications, 2nd edn., Wiley, New York.

    Google Scholar 

  • SCHEFFÉ, H. (1959): The Analysis of Variance, Wiley, New York.

    Google Scholar 

  • SEARLE, S.R., CASELLA, G., and MCCULLOCH, C.E. (1992): Variance Components, Wiley, New York.

    Google Scholar 

  • SEBER, G.A.F. (1984): Multivariate Observations, Wiley, New York.

    Google Scholar 

  • SMITH, A.F.M. (1973): A general Bayesian linear model. J. Royal Stat. Soc. B., 35, 67–75.

    MATH  Google Scholar 

  • THEIL (1971): Principle fo Econometrics. Wiley, New York.

    Google Scholar 

  • TUKEY, J.W. (1977): Exploratory Data Analysis, Addison-Wesley, Reading, Mass.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Advanced Studies, Stumpergasse 56, A-1060, Vienna, Austria

    Wolfgang Polasek

  2. Centre for Mathematics and its Applications, Mathematical Sciences Institute, Australian National University, Canberra, ACT, 0200, Australia

    Shuangzhe Liu

Authors
  1. Wolfgang Polasek
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shuangzhe Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Institute of Business Administration and Economics, Brandenburg University of Technology Cottbus, Konrad-Wachsmann-Allee 1, 03046, Cottbus, Germany

    Daniel Baier (Chair of Marketing and Innovation Management) (Chair of Marketing and Innovation Management)

  2. Department of Business Administration and Economics, Bielefeld University, Universitätsstr. 25, 33615, Bielefeld, Germany

    Reinhold Decker (Chair of Marketing) (Chair of Marketing)

  3. Computer Based New Media Group (CGNM), Institute for Computer Science, University of Freiburg, Georges-Köhler-Allee 51, 79110, Freiburg, Germany

    Lars Schmidt-Thieme

Rights and permissions

Reprints and permissions

Copyright information

© 2005 Springer-Verlag Berlin · Heidelberg

About this chapter

Cite this chapter

Polasek, W., Liu, S. (2005). ANOVA Models with Generalized Inverses. In: Baier, D., Decker, R., Schmidt-Thieme, L. (eds) Data Analysis and Decision Support. Studies in Classification, Data Analysis, and Knowledge Organization. Springer, Berlin, Heidelberg. https://doi.org/10.1007/3-540-28397-8_14

Download citation

Publish with us

Policies and ethics

Search

Navigation