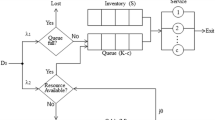
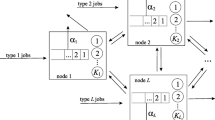
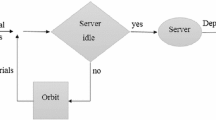
Abstract
In this paper we investigate some relations between the Petri net formalism and the queueing networks with blocking. This type of queueing network models are used to represent systems with finite capacity resource constraints, such as production, communication and computer systems. Various blocking mechanisms have been defined in the literature to represent the different behaviours of real systems with limited resources.
We show that the representation of these queueing networks by means of Generalized Stochastic Petri Nets offers the possibility of using results developed within the Petri net framework. In particular, we investigate product form equilibrium distributions for queueing networks with blocking by means of structural Petri net results. More precisely, we use the notion of implicit places. With this concept we characterise a class of queueing networks with blocking having interesting properties. For each queueing network of this class there exists another model with the same performance measures and exhibiting product form equilibrium distribution.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
M. Ajmone Marsan, G. Balbo, G. Conte, S. Donatelli, and G. Franceschinis. Modelling with Generalized Stochastic Petri Nets. John Wiley & Sons, 1995.
S. Balsamo and V. De Nitto-Personé. Closed queueing networks with finite capacities: Blocking types, product-form solution and performance indices. Performance Evaluation, 12:85–102, 1991.
S. Balsamo and V. De Nitto-Personé. A survey of product-form queueing networks with blocking and their equivalences. Annals of Operation Research, 48:31–61, 1994.
F. Baskett, K. M. Chandy, R. R. Muntz, and F. Palacios. Open, closed and mixed networks of queues with different classes of customers. Journal of the ACM, 22(2):248–260, April 1975.
B. Baynat and Y. Dallery. A ufified view of product-form approximation techniques for general closed queueing networks. Performance Evaluation, 18:205–224, 1993.
J. P. Buzen. Computational algorithms for closed queueing networks with exponential servers. Communications of the ACM, 16(9):527–531, September 1973.
J. M. Colom and M. Silva. Improving the linearly based characterization of P/T nets. In Proc. 10th Intern. Conference on Application and Theory of Petri Nets, pages 52–73, Bonn, Germany, June 1989.
W. J. Gordon and G. F. Newell. Cyclic queueing systems with restricted queues. Operations Research, 15:266–278, 1967.
M. Gribaudo and M. Sereno. GSPN semantics for queueing networks with blocking. In Proc. 7th Intern. Workshop on Petri Nets and Performance Models, Saint Malo, France, June 1997. IEEE-CS Press.
J. R. Jackson. Jobshop-like queueing systems. Management Science, 10(1):131–142, October 1963.
L. Kleinrock. Queueing Systems Volume I: Theory. Wiley, New York, NY, 1975.
S. Kundu and I. F. Akyldiz. Deadlock free buffer allocation in closed queueing networks. Queueing Systems, 4:47–56, 1989.
T. Murata. Petri nets: properties, analysis, and applications. Proceedings of the IEEE, 77(4):541–580, April 1989.
R. O. Onvural. Survey of closed queueing networks with blocking. ACM Computing Surveys, 22:83–121, 1990.
H. G. Perros. Queueing Networks with Blocking. Oxford, 1994.
M. Reiser and S. S. Lavenberg. Mean value analysis of closed multichain queueing networks. Journal of the ACM, 27(2):313–322, April 1980.
P. J. Schweitzer. A survey of mean value analysis, its generalizations, and applications, for networks of queues. Technical report, University of Rochester, Rochester, USA, Dec 1990.
M. Silva. Las Redes de Petri en la Automatica y la Informatica. Ed. AC, Madrid, Spain, 1985. In Spanish.
N. van Dijk. A simple bounding methodology for non-product-form queueing networks with blocking. In Proc. First International Workshop on Queueing Networks with Finite Capacity, Raleigh, North Carolina, USA, May 1988.
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 1998 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Gribaudo, M., Sereno, M. (1998). On the Use of Structural Petri Net Analysis for Studying Product Form Equilibrium Distributions of Queueing Networks with Blocking. In: Desel, J., Silva, M. (eds) Application and Theory of Petri Nets 1998. ICATPN 1998. Lecture Notes in Computer Science, vol 1420. Springer, Berlin, Heidelberg. https://doi.org/10.1007/3-540-69108-1_14
Download citation
DOI: https://doi.org/10.1007/3-540-69108-1_14
Published:
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-64677-8
Online ISBN: 978-3-540-69108-2
eBook Packages: Springer Book Archive