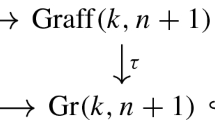Abstract
The Jacobi set is a useful descriptor of mutual behavior of functions defined on a common domain. We introduce the piecewise linear Jacobi set for general vector fields on simplicial complexes. This definition generalizes the definition of the Jacobi set for gradients of functions introduced by Edelsbrunner and Harer.
This work was supported by the Ministry of Education and Science of the Republic of Kazakhstan (program 0115PK03029) and Russian Foundation for Basic Research (grant 15-01-01671a).
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Similar content being viewed by others
References
Edelsbrunner, H., Harer, J., Natarajan, V., Pascucci, V.: Local and global comparison of continuous functions. In: Proceedings of 16th IEEE Conference on Visualization, pp. 275–280. IEEE Computer Society (2004). https://doi.org/10.1109/VISUAL.2004.68
Wolpert, N.: An exact and efficient approach for computing a cell in an arrangement of quadrics. Ph.D. thesis, Universität des Saarlandes (2002)
Wolpert, N.: Jacobi curves: computing the exact topology of arrangements of non-singular algebraic curves. In: Di Battista, G., Zwick, U. (eds.) ESA 2003. LNCS, vol. 2832, pp. 532–543. Springer, Heidelberg (2003). https://doi.org/10.1007/978-3-540-39658-1_49
Edelsbrunner, H., Harer, J.: Jacobi sets of multiple morse functions. In: Cucker, F., DeVore, R., Olver, P., Süli, E. (eds.) Foundations of Computational Mathematics, Minneapolis 2002. London Mathematical Society Lecture Note Series, pp. 37–57. Cambridge University Press, Cambridge (2004). https://doi.org/10.1017/CBO9781139106962.003
Novikov, S., Taimanov, I.: Modern Geometric Structures and Fields. American Mathematical Society, Providence (2006)
Natarajan, N.S.: Simplification of jacobi sets. In: Pascucci, V., Tricoche, X., Hagen, H., Tierny, J. (eds.) Topological Methods in Data Analysis and Visualization: Theory, Algorithms, and Applications. MATHVISUAL, pp. 91–102. Springer, Heidelberg (2011). https://doi.org/10.1007/978-3-642-15014-2_8
Bhatia, H., Wang, B., Norgard, G., Pascucci, V., Bremer, P.T.: Local, smooth, and consistent jacobi set simplification. Comput. Geom.: Theory Appl. 48(4), 311–332 (2015). https://doi.org/10.1016/j.comgeo.2014.10.009
NOAA Operational Model Archive and Distribution System. Data Transfer: NCEP GFS Forecasts (0.25 degree grid). http://nomads.ncep.noaa.gov/cgi-bin/filter_gfs_0p25_1hr.pl?dir=%2Fgfs.2018110100
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2019 Springer Nature Switzerland AG
About this paper
Cite this paper
Adilkhanov, A.N., Pavlov, A.V., Taimanov, I.A. (2019). Discrete Analog of the Jacobi Set for Vector Fields. In: Marfil, R., Calderón, M., Díaz del Río, F., Real, P., Bandera, A. (eds) Computational Topology in Image Context. CTIC 2019. Lecture Notes in Computer Science(), vol 11382. Springer, Cham. https://doi.org/10.1007/978-3-030-10828-1_1
Download citation
DOI: https://doi.org/10.1007/978-3-030-10828-1_1
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-030-10827-4
Online ISBN: 978-3-030-10828-1
eBook Packages: Computer ScienceComputer Science (R0)