Skip to main content
Springer Nature Link
Log in

Software of Linear and Geometrically Non-linear Problems Solution Under Spatial Loading of Rods of Complex Configuration

  • Conference paper
  • First Online:
Intelligent Human Computer Interaction (IHCI 2020)

Part of the book series: Lecture Notes in Computer Science ((LNISA,volume 12615))

Included in the following conference series:

Abstract

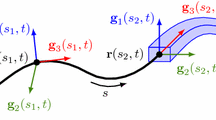
A software has been developed for calculating linear and geometrically nonlinear problems of rods under spatial loading. The software system structure for calculating linear problems of spatial loading of rods of complex configuration was improved by the R-function method (RFM) and successive approximations . Based on the developed software, the oscillation processes of spatially loaded rods in linear statements, the statics and dynamics of spatially loaded rods in linear and geometrically nonlinear statements were investigated; the problems of constrained torsion of a prismatic body of arbitrary section with different cavities were solved.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

References

  1. Anarova, S.: Mathematical modeling of the stress-strain state of elastic prismatic bodies of arbitrary section. Monograph, Tashkent, Navruz (2017)

    Google Scholar 

  2. Kabulov, V.K., Anarova, Sh.A.: Comparison of the cavity effect on the stress state of prismatic bodies of arbitrary cross-section in the problems of constrained torsion. J. AS RUz 6, 7–10 (2003)

    Google Scholar 

  3. Kurmanbaev, B.: Unified technology for constructing discrete models of three-dimensional static and dynamic problems of elastic and inelastic bodies, algorithms and their solutions, software and numerical analysis of results. Dis.Doc. Phys.-Math. Sci. - Tashkent (1992)

    Google Scholar 

  4. Nazirov, Sh.A., Piskorsky, L.F.: Complex of software tools for calculating and optimizing plate structures of a complex shape. In: Algorithms: Collection of scientific works. - Tashkent, IK AN RUz, vol. 81, pp. 41–54

    Google Scholar 

  5. Nazirov, Sh.A., Sadikov, Kh.S.: Complex of software tools for solving boundary value problems by variational methods. In: Algorithms: Collection of scientific works. - Tashkent, IK AN RUz, no. 65, pp. 38–48 (1988)

    Google Scholar 

  6. Nazirov, Sh.A., Nuraliev, F.M, Amanov, O.T.: The structure of the software complex for solving boundary value problems for systems of partial differential equations. Uzbek Mag. “Probl. Inform. Energy” – Tashkent 6, 40–43 (1998)

    Google Scholar 

  7. Nazirov, Sh.A., Anarova, Sh.A.: Modeling the stress-strain state of prismatic bodies of arbitrary cross-section in problems of constrained torsion. Uzbek Mag. “Probl. Inform. Energy” –Tashkent 3, 22–25 (2003)

    Google Scholar 

  8. Nazirov, Sh.A., Abduazizov, A.A.: Description of a set of programs for calculating radio-electronic devices. In: Issues of Calculated and Applied Mathematics: Collection of Scientific Works. - Tashkent, RPP and AIC Center, no. 128, pp. 64–84 (2012)

    Google Scholar 

  9. Nazirov, Sh.A., Nuraliyev, F.M.: Anarova, Sh.A.: Study of numeric convergence of the method of R - functions in problems of constraint torsion. Am. J. Comput. Appl. Math. 2(4), 89–196 (2012). https://doi.org/10.5923/j.ajcam.20120204.07

  10. Anarova, Sh.A., Nuraliev, F.M.: Study of stressed state of elastic prismatic bodies of arbitrary section with a cavity in problems of constraint torsion. Int. J. Sci. Innov. Math. Res. (IJSIMR) 3(2), 1–15 (2015). ISSN: 347–307X (Print) & ISSN: 2347–3142. www.arcjournals.org

  11. Anarova, Sh.A., Nuraliyev, F.M.: Usmonov, B.Sh., Chulliyev, Sh.I.: Numerical solution of the problem of spatially loaded rods in linear and geometrically nonlinear statements. Int. J. Eng. Technol. 7(4), 4563–4569 (2018). www.sciencepubco.com/index.php/doi:10.14419/ijet.v7i4.22669

  12. Anarova, Sh., Ismoilov, Sh.: Mathematical simulation of stress-strain state of loaded rods with account of transverse bending. J. Phys.: Conf. Ser. 1260, 102002 (2019). https://doi.org/10.1088/1742-6596/1260/10/102002

  13. Anarova, S., Isomidinov, A., Ismoilov, S.: Numerical analysis of vibration processes of spatially loaded rods. Probl. Comput. Appl. Math. Tashkent 5, 29–45 (2019)

    Google Scholar 

  14. Anarova, Sh.A., Abdirozikov, O.Sh.: Mathematical and numerical model of thermo elastic plates with complex form. Mukhammad al-Khorazmiy avlodlari ilmiy-amaliy va axborot tahliliy jurnal. Tashkent 5(10), 121–124 (2019)

    Google Scholar 

  15. Nuraliev, F., Anarova, Sh., Amanov, O., Jumaev S. & Abdirozikov O.: Mathematical model and computational experiments for the calculation of three-layer plates of complex configuration. J. Phys.: Conf. Ser. 1546, 012094 (2020). https://doi.org/10.1088/17426596/1546/1/01204

Download references

Author information

Authors and Affiliations

  1. Tashkent University of Information Technologies, 100200, Tashkent, Republic of Uzbekistan

    Sh. A. Anarova

  2. Namangan Engineering-Construction Institute, 160103, Namangan, Republic of Uzbekistan

    SH. M. Ismoilov

  3. Information and Communication Technology and Communication MOD, 111811, Tashkent, Uzbekistan

    O. Sh. Abdirozikov

Authors
  1. Sh. A. Anarova
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. SH. M. Ismoilov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. O. Sh. Abdirozikov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Sh. A. Anarova , SH. M. Ismoilov or O. Sh. Abdirozikov .

Editor information

Editors and Affiliations

  1. Woosong University, Daejeon, Korea (Republic of)

    Madhusudan Singh

  2. Dongseo University, Busan, Korea (Republic of)

    Dae-Ki Kang

  3. Keimyung University, Daegu, Korea (Republic of)

    Jong-Ha Lee

  4. Indian Institute of Information Technoloy, Allahabad, India

    Uma Shanker Tiwary

  5. Hankuk University of Foreign Studies, Yongin, Korea (Republic of)

    Dhananjay Singh

  6. Pukyong National University, Busan, Korea (Republic of)

    Wan-Young Chung

Rights and permissions

Reprints and permissions

Copyright information

© 2021 Springer Nature Switzerland AG

About this paper

Check for updates. Verify currency and authenticity via CrossMark

Cite this paper

Anarova, S.A., Ismoilov, S.M., Abdirozikov, O.S. (2021). Software of Linear and Geometrically Non-linear Problems Solution Under Spatial Loading of Rods of Complex Configuration. In: Singh, M., Kang, DK., Lee, JH., Tiwary, U.S., Singh, D., Chung, WY. (eds) Intelligent Human Computer Interaction. IHCI 2020. Lecture Notes in Computer Science(), vol 12615. Springer, Cham. https://doi.org/10.1007/978-3-030-68449-5_38

Download citation

  • DOI: https://doi.org/10.1007/978-3-030-68449-5_38

  • Published:

  • Publisher Name: Springer, Cham

  • Print ISBN: 978-3-030-68448-8

  • Online ISBN: 978-3-030-68449-5

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics