Abstract
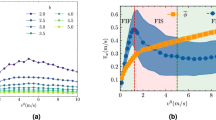
Clogging of granular materials and jamming of pedestrian crowds occur because of the formation of arches at bottlenecks. We propose a simple microscopic model that is able to reproduce oscillation phenomena due to formation and destabilization of arches in 2-dimensional flows. The dynamics of particles in front of a bottleneck is described by a one-dimensional stochastic cellular automaton on a semicircular geometry. The model predicts the existence of a critical bottleneck size for jamless particle flows and allows to determine the dependence of the jamming probability on the system size. The model can also be studied analytically and the results are in good agreement with simulations.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Visser, T., Wysocki, A., Rex, M., Löwen, H., Royall, C.P., Imhof, A., van Blaaderen, A.: Lane formation in driven mixtures of oppositely charged colloids. Soft Matter 7, 2352 (2011)
Helbing, D., Farkas, I., Vicsek, T.: Simulating dynamical features of escape panic. Nature (London), 407, 487 (2000)
Helbing, D., Buzna, L., Johansson, A., Werner, T.: Self-Organized Pedestrian Crowd Dynamics: Experiments, Simulations, and Design Solutions. Transp. Sci. 39, 1 (2005)
To, K., Lai, P.-Y., Pak, H.K.: Jamming of Granular Flow in a Two-Dimensional Hopper. Phys. Rev. Lett. 86, 71 (2001)
Janda, A., Zuriguel, I., Garcimartín, A., Pugnaloni, L.A., Maza, D.: Jamming and critical outlet size in the discharge of a two-dimensional silo. Europhys. Lett. 84, 44002 (2008)
Mankoc, C., Garcimartin, A., Zuriguel, I., Maza, D., Pugnaloni, L.A.: Role of vibrations in the jamming and unjamming of grains discharging from a silo. Phys. Rev. E 80, 011309 (2009)
Zuriguel, I., Garcimartín, A., Maza, D., Pugnaloni, L.A., Pastor, J.M.: Jamming during the discharge of granular matter from a silo. Phys. Rev. E 71, 051303 (2005)
Helbing, D., Johansson, A., Mathiesen, J., Jensen, M.H., Hansen, A.: Jamming of Granular Flow in a Two-Dimensional Hopper. Phys. Rev. Lett. 97, 168001 (2006)
Janda, A., Maza, D., Garcimartín, A., Kolb, E., Lanuza, J., Clément, E.: Unjamming a granular hopper by vibration. Europhys. Lett. 87, 24002 (2009)
Masuda, T., Nishinari, K., Schadschneider, A.: Critical Bottleneck Size for Jamless Particle Flows in Two Dimension. Phys. Rev. Lett. 112, 138701 (2014)
Schütz, G.M.: Exactly Sovable Models for Many-Body Systems. In: Domb, C., Lebowitz, J.L. (eds.) Phase Transitions and Critical Phenomena, vol. 19. Academic Press (2001)
Schadschneider, A., Chowdhury, D., Nishinari, K.: Stochastic Transport in Complex Systems: From Molecules to Vehicles. Elsevier, Amsterdam (2010)
Derrida, B., Evans, M.R., Hakim, V., Pasquier, V.: Exact solution of a 1d asymmetric exclusion model using a matrix formulation. J. Phys. A26, 1493 (1993)
Krebs, K., Sandow, S.: Matrix product eigenstates for one-dimensional stochastic models and quantum spin chains. J. Phys. A30, 3165 (1997)
Klauck, K., Schadschneider, A.: On the ubiquity of matrix-product states in one-dimensional stochastic processes with boundary interactions. Physica A271, 102 (1999)
Garcimartín, A., Zuriguel, I., Pugnaloni, L.A., Janda, A.: Shape of jamming arches in two-dimensional deposits of granular materials. Phys. Rev. E 82, 031306 (2010)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2014 Springer International Publishing Switzerland
About this paper
Cite this paper
Masuda, T., Nishinari, K., Schadschneider, A. (2014). Cellular Automaton Approach to Arching in Two-Dimensional Granular Media. In: Wąs, J., Sirakoulis, G.C., Bandini, S. (eds) Cellular Automata. ACRI 2014. Lecture Notes in Computer Science, vol 8751. Springer, Cham. https://doi.org/10.1007/978-3-319-11520-7_32
Download citation
DOI: https://doi.org/10.1007/978-3-319-11520-7_32
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-11519-1
Online ISBN: 978-3-319-11520-7
eBook Packages: Computer ScienceComputer Science (R0)