Skip to main content
Springer Nature Link
Log in

Systolic Dissemination in the Arrowhead Family

  • Conference paper
Cellular Automata (ACRI 2014)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 8751))

Included in the following conference series:

  • 3132 Accesses

Abstract

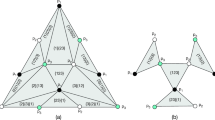
Although cellular automata (CA) are usually driven by a local rule, global communications are often needed either to synchronize a process or to share common data. However, these communications must be carried out from the nearest-neighbor, local transition. Such disseminations are named “systolic” herein: this metaphor is borrowed from the eponymous cellular architectures. The core of this study is the topology of the “arrowhead” family underlying the CA network in the hexagonal tessellation. The graphs of this family, directed or undirected, are Cayley graphs, or graphs of groups and are therefore vertex-transitive. As a consequence, the local rule is the same within the whole network. Two types of dissemination are presented: a (one–to–all) broadcasting and a (all–to–all) gossiping. For each type, a 3–port, directed scheme and a 6–port, undirected scheme are derived from construction. It is shown that the complexity of these algorithms is the graph diameter, either directed or undirected, according to the case study.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Moore, E.F.: The firing squad synchronization problem. In: Moore, E.F. (ed.) Sequential Machines, Selected Papers, pp. 213–214. Addison-Wesley, Reading (1964)

    Google Scholar 

  2. Umeo, H.: Firing squad synchronization problem in cellular automata. In: Encyclopedia of Complexity and Systems Science, pp. 3537–3574 (2009)

    Google Scholar 

  3. Désérable, D.: Propagative mode in a lattice-grain CA: time evolution and timestep synchronization. In: Sirakoulis, G.C., Bandini, S. (eds.) ACRI 2012. LNCS, vol. 7495, pp. 20–31. Springer, Heidelberg (2012)

    Chapter  Google Scholar 

  4. Désérable, D., Dupont, P., Hellou, M., Kamali-Bernard, S.: Cellular automata in complex matter. Complex Systems 20(1), 67–91 (2011)

    MathSciNet  Google Scholar 

  5. Chopard, B., Droz, M.: Cellular automata modeling of physical systems. Cambridge University Press, Cambridge (1998)

    Book  MATH  Google Scholar 

  6. Fraigniaud, P., Lazard, E.: Methods and problems of communication in usual networks. Discrete Applied Mathematics 53(1-3), 79–133 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  7. Kung, H.T.: Why systolic architectures? Computer 15(1), 37–46 (1982)

    Article  Google Scholar 

  8. Liestman, A.L., Richards, D.: Perpetual gossiping. Par. Proc. Lett. 3(4), 347–355 (1993)

    Article  MathSciNet  Google Scholar 

  9. Hromkovič, J., Klasing, R., Unger, W., Wagener, H.: The complexity of systolic dissemination of information in interconnection networks. In: Cosnard, M., Ferreira, A., Peters, J. (eds.) CFCP 1994. LNCS, vol. 805, pp. 235–249. Springer, Heidelberg (1994)

    Google Scholar 

  10. Flammini, M., Pérennes, S.: Lower bounds on systolic gossip. Information and Computation 196(2), 71–94 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  11. Chen, M.-S., Shin, K.G., Kandlur, D.D.: Addressing, routing and broadcasting in hexagonal mesh multiprocessors. IEEE Trans. Comp. 39(1), 10–18 (1990)

    Article  Google Scholar 

  12. Morillo, P., Comellas, F., Fiol, M.A.: Metric problems in triple loop graphs and digraphs associated to an hexagonal tessellation of the plane, TR 05-0286 (1986)

    Google Scholar 

  13. Albader, B., Bose, B., Flahive, M.: Efficient communication algorithms in hexagonal mesh interconnection networks. J. LaTeX Class Files 6(1), 1–10 (2007)

    Google Scholar 

  14. Désérable, D.: Minimal routing in the triangular grid and in a family of related tori. In: Lengauer, C., Griebl, M., Gorlatch, S. (eds.) Euro-Par 1997. LNCS, vol. 1300, pp. 218–225. Springer, Heidelberg (1997)

    Chapter  Google Scholar 

  15. Désérable, D.: Hexagonal Bravais–Miller routing of shortest path (unpublished)

    Google Scholar 

  16. Désérable, D.: Broadcasting in the arrowhead torus. Computers and Artificial Intelligence 16(6), 545–559 (1997)

    MathSciNet  Google Scholar 

  17. Heydemann, M.-C., Marlin, N., Pérennes, S.: Complete rotations in Cayley graphs. European J. Combinatorics 22(2), 179–196 (2001)

    Article  MATH  Google Scholar 

  18. Désérable, D.: A versatile two-dimensional cellular automata network for granular flow. SIAM J. Applied Math. 62(4), 1414–1436 (2002)

    Article  MATH  Google Scholar 

  19. Cottenceau, G., Désérable, D.: Open environment for 2d lattice-grain CA. In: Bandini, S., Manzoni, S., Umeo, H., Vizzari, G. (eds.) ACRI 2010. LNCS, vol. 6350, pp. 12–23. Springer, Heidelberg (2010)

    Chapter  Google Scholar 

  20. Désérable, D., Masson, S., Martinez, J.: Influence of exclusion rules on flow patterns in a lattice-grain model. In: Kishino (ed.) Powders & Grains, pp. 421–424. Balkema (2001)

    Google Scholar 

  21. Ediger, P., Hoffmann, R., Désérable, D.: Routing in the triangular grid with evolved agents. J. Cellular Automata 7(1), 47–65 (2012)

    MATH  Google Scholar 

  22. Ediger, P., Hoffmann, R., Désérable, D.: Rectangular vs triangular routing with evolved agents. J. Cellular Automata 8(1-2), 73–89 (2013)

    Google Scholar 

  23. Hoffmann, R., Désérable, D.: All-to-all communication with cellular automata agents in 2D grids: topologies, streets and performances. J. Supercomputing 69(1), 70–80 (2014)

    Article  Google Scholar 

  24. Dally, W.J., Seitz, C.L.: The torus routing chip. Dist. Comp. 1, 187–196 (1986)

    Article  Google Scholar 

  25. Xiang, Y., Stewart, I.A.: Augmented k–ary n–cubes. Information Sciences 181(1), 239–256 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  26. Désérable, D.: A family of Cayley graphs on the hexavalent grid. Discrete Applied Math. 93, 169–189 (1999)

    Article  MATH  Google Scholar 

  27. Grünbaum, B., Shephard, G.C.: Tilings and patterns. Freeman & Co., NY (1987)

    MATH  Google Scholar 

  28. Grossman, I., Magnus, W.: Groups and their graphs. New Mathematical Library, vol. 14. Random House, New-York (1964)

    MATH  Google Scholar 

  29. Harary, F.: Graph theory. Addison-Wesley, Reading (1969)

    Google Scholar 

  30. Désérable, D.: Embedding Kadanoff’s scaling picture into the triangular lattice. Acta Phys. Polonica B Proc. Suppl. 4(2), 249–265 (2011)

    Article  Google Scholar 

  31. Mandelbrot, B.B.: The fractal geometry of nature. Freeman and Cie (1982)

    Google Scholar 

  32. Sierpiński, W.: On a curve every point of which is a point of ramification. Prace Mat.–Fiz. 27, 77–86 (1916), et Acad. Pol. Sci. II, 99–106 (1975)

    Google Scholar 

  33. Désérable, D.: Arrowhead and diamond diameters (unpublished)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. INSA - Institut National des Sciences Appliquées, 20 Avenue des Buttes de Coësmes, 35043, Rennes, France

    Dominique Désérable

Authors
  1. Dominique Désérable
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Dept. of Applied Computer Science, AGH University of Science and Technology, al. Mickiewicza 30, 30-059, Krakow, Poland

    Jarosław Wąs

  2. Dept. of Electrical and Computer Engineering, Democritus University of Thrace, 67100, Xanthi, Greece

    Georgios Ch. Sirakoulis

  3. CSAI - Complex Systems and Artificial Intelligence Research Center, University of Milano-Bicocca, 20126, Milan, Italy

    Stefania Bandini

Rights and permissions

Reprints and permissions

Copyright information

© 2014 Springer International Publishing Switzerland

About this paper

Cite this paper

Désérable, D. (2014). Systolic Dissemination in the Arrowhead Family. In: Wąs, J., Sirakoulis, G.C., Bandini, S. (eds) Cellular Automata. ACRI 2014. Lecture Notes in Computer Science, vol 8751. Springer, Cham. https://doi.org/10.1007/978-3-319-11520-7_9

Download citation

  • DOI: https://doi.org/10.1007/978-3-319-11520-7_9

  • Publisher Name: Springer, Cham

  • Print ISBN: 978-3-319-11519-1

  • Online ISBN: 978-3-319-11520-7

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics