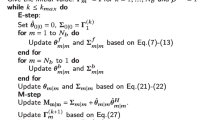Abstract
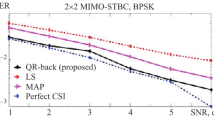
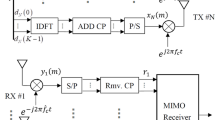
The Estimation of MIMO Channels becomes a tedious task in the non-stationary environment. This is because of pilot overhead and corresponding interference. To reduce this pilot overhead, QR decomposition is proposed in the literature. However, higher the rate of the QR decomposition will result in a computationally intensive system. In this paper, we propose a two stage estimation solution to reduce the complexity as well as to eliminate the interference arising out of pilot overhead. Advantages of this paper can be seen as separation of channel impulse response and interference, elimination of the interference arising out of pilot overhead, and reduction in computational complexity.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Similar content being viewed by others
References
Jiang, W., Asai, Y., Onizawa, T., Aikawa, S.: Fast algorithm for decision feedback equalization in multiple input multiple output channel. In: Vehicular Technology Conference, vol. 5, pp. 2423–2428 (2006)
Komninakis, C., Fraqouli, C., Sayed, A.H., Wesel, R.D.: Multi-input multi-output fading channel tracking and equalization using Kalman estimation. IEEE Trans. Sig. Process. 50, 1065–1076 (2002)
Fechtel, S.A., Meyr, H.: An investigation of channel estimation and equalization techniques for moderately rapid fading HF-channels. ICC 2, 768–772 (1991)
Tong, J.: An adaptive channel tracking method for MIMO-OFDM systems. In: ICCCAS, vol. 1, pp. 354–358 (2004)
Zia, A.: Channel identification and tracking using alternating projections. Statistical Signal Processing, pp. 430-433 (2003)
Schafhuber, D., Matz, G., Hlawatsch, F.: Kalman tracking of time varying channels in wireless MIMO-OFDM systems. Sign. Syst. Comput. 2, 1261–1265 (2003)
Oberli, C.: Maximum likelihood tracking algorithms for MIMO-OFDM. Commun. 4, 2468–2472 (2004)
Deng, K., Yin, Q., Meng, Y.: Blind symbol detection for multiple-input multiple-output systems via particle filtering. IEEE Int. Conf. Acoust. Speech Sig. Process. 3, 1041–1044 (2005)
Cescato, D., Bölcskei, H.: Algorithms for interpolation-based QR decomposition in MIMO-OFDM systems. IEEE Trans. Signal Process. 59(4), 1719–1733 (2011)
Po-Lin, C., Lin-Zheng, H., Li-Wei, C., Yuan-Hao, H.: Interpolation-based QR decomposition and channel estimation processor for MIMO-OFDM system. IEEE Transact. Circuits Syst. I 58(5), 1129–1141 (2011)
Bouchired, S., Ibnkahla, M., Roviras, D., Castanie, F.: Equalization of satellite UMTS channels using neural network devices. In: Proceedings of IEEE, ICASSP 1999, Phoenix, May 1999
Lin, H., Yamashita, K.: Hybrid simplex genetic algorithm for blind equalization using RBF networks. Math. Comput. Simul. 59, 293–304 (2002)
Han, S., Pedrycz, W., Changwook, H.: Nonlinear channel blind equalization using hybrid genetic algorithm with simulated annealing. Math. Comput. Model. 41, 697–709 (2005)
Lucky, R.W.: Automatic equalization of digital communication. Bell Syst. Tech. J 44, 547–588 (1965)
George, D.A., Bowen, R.R., Storey, J.R.: An adaptive decision feedback equalizer. IEEE Transact. Commun. Technol. (COM) 19, 281–293 (1971)
Gor, L: Complexity Reduction In: Multiple Input Multiple Output Algorithms, Ph.d. thesis. Victoria University (2007)
Fischer, R.F.H., Windpassinger, C., Stierstorfer, C., Siegl, C., Schenk, A., Abay, U.: Lattice-reduction-aided MMSE equalization and the successive estimation of correlated data. AEU – Int. J. Electron. Commun. 65(8), 688–693 (2011)
Oliver, J., Aravind, R., Prabhu, K.M.M.: A krylov: Subspace based low-rank channel estimation in OFDM systems. Sig. Process. 90(6), 1861–1872 (2010)
Panda, R.N., Padhy, S.K., Prasad, S., Panigrahi, S.P.: Reduced complexity dynamic systems using approximate control moments. Circuits Syst. Sig. Process. 31(5), 1731–1744 (2012)
Gao, J., Zhu, X., Nandi, A.K.: Independent component analysis for multiple-input multiple output wireless communication systems. Sig. Process. 91(4), 607–623 (2011)
Huang, Z., Tsai, P.: Efficient implementation of QR decomposition for Gigabit MIMO-OFDM systems. IEEE Transact. Circuits Syst. I 58(10), 2531–2542 (2011)
Widrow, B., Stearns, S.: Adaptive Signal Processing. Prentice-Hall Inc., Murray Hill (1985)
Li, H.Y., Fu, H.L., Guan, A.H.: Game theoretic power control algorithm in cognitive radio networks. J. Chongqing Univ. Posts Telecommun.: Nat. Sci. 22(6), 756–760 (2010)
Zhao, C.S., Kwak, K.: Joint sensing time and power allocation in cooperatively cognitive networks. IEEE Commun. Lett. 14(2), 163–165 (2010)
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2015 Springer International Publishing Switzerland
About this paper
Cite this paper
Panda, R.N., Padhy, S.K., Panigrahi, S.P. (2015). Complexity Reduction Using Two Stage Tracking. In: Panigrahi, B., Suganthan, P., Das, S. (eds) Swarm, Evolutionary, and Memetic Computing. SEMCCO 2014. Lecture Notes in Computer Science(), vol 8947. Springer, Cham. https://doi.org/10.1007/978-3-319-20294-5_71
Download citation
DOI: https://doi.org/10.1007/978-3-319-20294-5_71
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-20293-8
Online ISBN: 978-3-319-20294-5
eBook Packages: Computer ScienceComputer Science (R0)